Parabola 2 Question 32
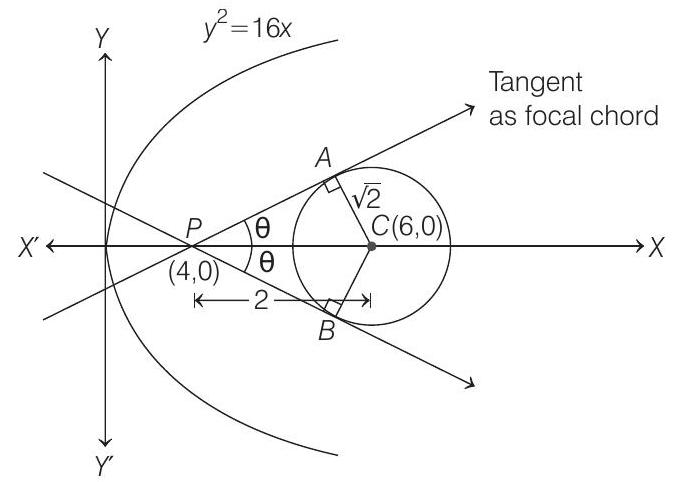
11. The focal chord to $y^{2}=16 x$ is tangent to $(x-6)^{2}+y^{2}=2$, then the possible values of the slope of this chord are
(2003, 1M)
(a) ${-1,1}$
(b) ${-2,2}$
(c) ${-2,1 / 2}$
(d) ${2,-1 / 2}$
Show Answer
Solution:
- Here, the focal chord of $y^{2}=16 x$ is tangent to circle $(x-6)^{2}+y^{2}=2$.
$\Rightarrow$ Focus of parabola as $(a, 0)$ i.e. $(4,0)$
Now, tangents are drawn from $(4,0)$ to $(x-6)^{2}+y^{2}=2$.
Since, $P A$ is tangent to circle.
$\therefore \tan \theta=$ slope of tangent $=\frac{A C}{A P}=\frac{\sqrt{2}}{\sqrt{2}}=1$, or $\quad \frac{B C}{B P}=-1$
$\therefore$ Slope of focal chord as tangent to circle $= \pm 1$
