Parabola 2 Question 21
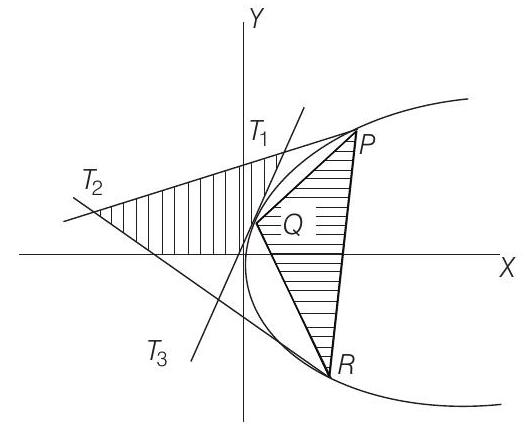
21. Consider the parabola
(2011)
Show Answer
Solution:
- As, we know area of
Topic 3 Equation of Normal and Properties






