Ellipse 1 Question 3
3. Let $S$ and $S^{\prime}$ be the foci of an ellipse and $B$ be any one of the extremities of its minor axis. If $\Delta S^{\prime} B S$ is a right angled triangle with right angle at $B$ and area $\left(\Delta S^{\prime} B S\right)=8 sq$ units, then the length of a latus rectum of the ellipse is
(a) $2 \sqrt{2}$
(b) $4 \sqrt{2}$
(c) 2
(d) 4
(2019 Main, 12 Jan II)
Show Answer
Answer:
Correct Answer: 3. (d)
Solution:
- Let the ellipse be $\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}=1$.
Then, according to given information, we have the following figure.
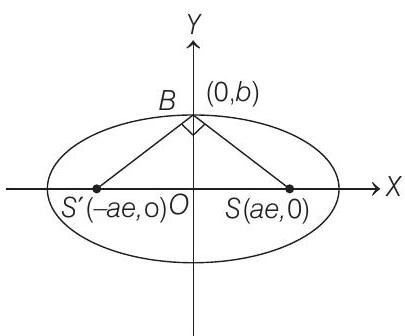
Clearly, slope of line $S B=\frac{b}{-a e}$ and slope of line $S^{\prime} B=\frac{b}{a e}$
$\because$ Lines $S B$ and $S^{\prime} B$ are perpendicular, so
$ \frac{b}{-a e} \cdot \frac{b}{a e}=-1 $
$[\because$ product of slopes of two
$ \Rightarrow \quad b^{2}=a^{2} e^{2} $
Also, it is given that area of $\Delta S^{\prime} B S=8$
$\therefore \quad \frac{1}{2} a^{2}=8$
$ \left[\because S^{\prime} B=S B=a \text { because } S^{\prime} B+S B=2 a \text { and } S^{\prime} B=S B\right] $
$\Rightarrow \quad a^{2}=16 \Rightarrow a=4$
$\because \quad e^{2}=1-\frac{b^{2}}{a^{2}}=1-e^{2}$
[from Eq. (i)]
$\Rightarrow \quad 2 e^{2}=1$
$\Rightarrow \quad e^{2}=\frac{1}{2}$
From Eqs. (i) and (iii), we get
$ \begin{array}{cc} & b^{2}=a^{2} \frac{1}{2}=16 \frac{1}{2} \quad \text { [using Eq. (ii)] } \\ \Rightarrow \quad & b^{2}=8 \end{array} $
Now, length of latus rectum $=\frac{2 b^{2}}{a}=\frac{2 \times 8}{4}=4$ units
