SATHEE: Complex Numbers 4 Question 9

Complex Numbers 4 Question 9

9. Suppose $z_{1}, z_{2}, z_{3}$ are the vertices of an equilateral triangle inscribed in the circle $| z | = 2$ . If $z_{1} = 1 + i \sqrt{3}$ , then $z_{2} = \dots, z_{3} = \dots$ .

(1994, 2M)

Show Answer

Answer:

Correct Answer: 9. $z_{2} = - 2, z_{3} = 1 - i \sqrt{3}$

Solution:

$z_{1} = 1 + i \sqrt{3} = r (\cos θ + i \sin θ)$

[let]

$\Rightarrow r \cos θ = 1, r \sin θ = \sqrt{3}$

$\Rightarrow r = 2$ and $θ = π / 3$

So, $z_{1} = 2 (\cos π / 3 + \sin π / 3)$

Since, $| z_{2} | = | z_{3} | = 2$

[given]

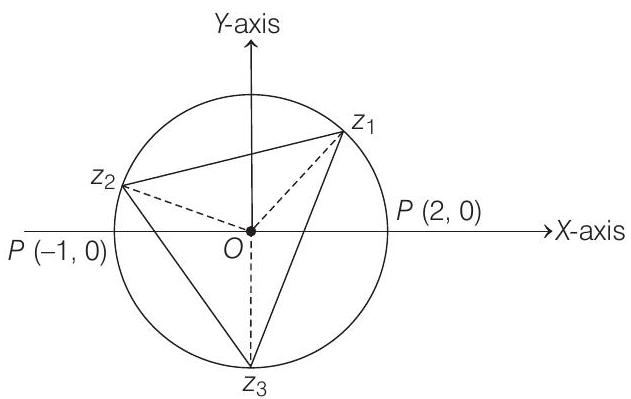
Now, the triangle $z_{1}, z_{2}$ and $z_{3}$ being an equilateral and the sides $z_{1} z_{2}$ and $z_{1} z_{3}$ make an angle $2 π / 3$ at the centre.

Therefore, $∠ P O z_{2} = \frac{π}{3} + \frac{2 π}{3} = π$

and $∠ P O z_{3} = \frac{π}{3} + \frac{2 π}{3} + \frac{2 π}{3} = \frac{5 π}{3}$

Therefore, $z_{2} = 2 (\cos π + i \sin π) = 2 (- 1 + 0) = - 2$

and $z_{3} = 2 \cos \frac{5 π}{3} + i \sin \frac{5 π}{3} = 2 \frac{1}{2} - i \frac{\sqrt{3}}{2} = 1 - i \sqrt{3}$

Alternate Solution

Whenever vertices of an equilateral triangle having centroid is given its vertices are of the form $z, z ω, z ω^{2}$ .

$∴$ If one of the vertex is $z_{1} = 1 + i \sqrt{3}$ , then other two vertices are $(z_{1} ω), (z_{1} ω^{2})$ .

$\begin{array}{lrl} \Rightarrow & (1 + i \sqrt{3}) \frac{(- 1 + i \sqrt{3})}{2}, (1 + i \sqrt{3}) \frac{(- 1 - i \sqrt{3})}{2} \\ \Rightarrow & \frac{- (1 + 3)}{2}, - \frac{(1 + i^{2} (\sqrt{3})^{2} + 2 i \sqrt{3})}{2} \\ \Rightarrow & - 2, - \frac{(- 2 + 2 i \sqrt{3})}{2} = 1 - i \sqrt{3} \\ ∴ & z_{2} = - 2 and z_{3} = 1 - i \sqrt{3} \end{array}$

Complex Numbers 4 Question 9

9. Suppose $z_{1}, z_{2}, z_{3}$ are the vertices of an equilateral triangle inscribed in the circle $| z | = 2$ . If $z_{1} = 1 + i \sqrt{3}$ , then $z_{2} = \dots, z_{3} = \dots$ .

Answer:

Alternate Solution

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Complex Numbers 4 Question 9

9. Suppose z1,z2,z3 are the vertices of an equilateral triangle inscribed in the circle |z|=2. If z1=1+i3, then z2=…,z3=….

Answer:

Alternate Solution

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Menu

9. Suppose $z_{1}, z_{2}, z_{3}$ are the vertices of an equilateral triangle inscribed in the circle $| z | = 2$ . If $z_{1} = 1 + i \sqrt{3}$ , then $z_{2} = \dots, z_{3} = \dots$ .