SATHEE: Circle 5 Question 22

Circle 5 Question 22

22. Through a fixed point $(h, k)$ secants are drawn to the circle $x^{2} + y^{2} = r^{2}$ . Show that the locus of the mid-points of the secants intercepted by the circle is $x^{2} + y^{2} = h x + k y . (1983, 5 M)$

Show Answer

Answer:

Correct Answer: 22. $x^{2} + y^{2} = h x + k y$

Solution:

Given, circle is $x^{2} + y^{2} = r^{2}$

Equation of chord whose mid point is given, is $T = S_{1} \Rightarrow x x_{1} + y y_{1} - r^{2} = x_{1}^{2} + y_{1}^{2} - r^{2}$

It also passes through $(h, k) h x_{1} + k y_{1} = x_{1}^{2} + y_{1}^{2}$

$∴$ Locus of $(x_{1}, y_{1})$ is

$x^{2} + y^{2} = h x + k y$

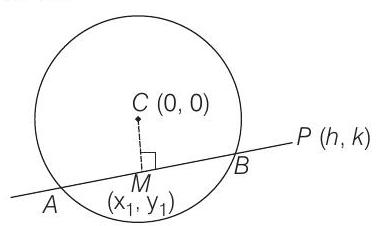
Alternate Solution

Let $M$ be the mid-point of chord $A B$ .

$\begin{array}{ll} \Rightarrow & C M ⊥ M P \\ \Rightarrow & (slope of C M) \cdot (slope of M P) = - 1 \\ \Rightarrow & \frac{y_{1}}{x_{1}} \cdot \frac{k - y_{1}}{h - x_{1}} = - 1 \\ \Rightarrow & k y_{1} - y_{1}^{2} = - h x_{1} + x_{1}^{2} \\ Hence, required locus is x^{2} + y^{2} = h x + k y \end{array}$

Circle 5 Question 22

22. Through a fixed point $(h, k)$ secants are drawn to the circle $x^{2} + y^{2} = r^{2}$ . Show that the locus of the mid-points of the secants intercepted by the circle is $x^{2} + y^{2} = h x + k y . (1983, 5 M)$

Answer:

Alternate Solution

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Circle 5 Question 22

22. Through a fixed point (h,k) secants are drawn to the circle x2+y2=r2. Show that the locus of the mid-points of the secants intercepted by the circle is x2+y2=hx+ky.(1983,5M)

Answer:

Alternate Solution

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Menu

22. Through a fixed point $(h, k)$ secants are drawn to the circle $x^{2} + y^{2} = r^{2}$ . Show that the locus of the mid-points of the secants intercepted by the circle is $x^{2} + y^{2} = h x + k y . (1983, 5 M)$