Chemical Kinetics - Result Question 40
####40. For a first order reaction $A(g) \longrightarrow 2 B(g)+C(g)$ at constant volume and $300 K$, the total pressure at the beginning $(t=0)$ and at time $t$ are $p _0$ and $p _t$, respectively. Initially, only $A$ is present with concentration $[A] _0$, and $t _{1 / 3}$ is the time required for the partial pressure of $A$ to reach $1 / 3^{rd}$ of its initial value. The correct option(s) is (are) (Assume that all these gases behave as ideal gases)
(2018 Adv.) (a)
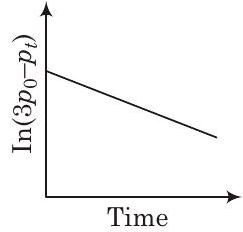
(c)
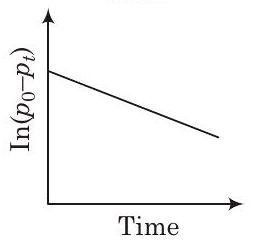
(b)
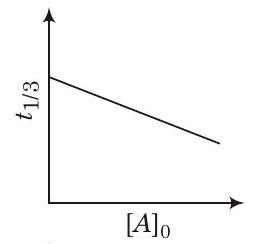
(d)
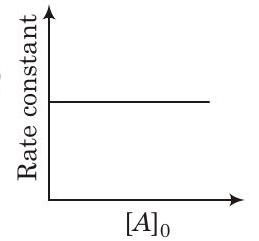
Show Answer
Answer:
Correct Answer: 40. $(a, d)$
Solution:
- Given for the reaction (at $T=300 K$ and constant volume $=V$ )
$$ \begin{array}{rrrc} & A(g) & \longrightarrow & 2 B(g)+C(g) \ \text { at } t=0 & p _0 & - & - \ \text { at } t=t & p _0-x & 2 x & x \ \text { at } t=t _{1 / 3} & {\left[p _0-\frac{2 p _0}{3}\right]=\frac{p _0}{3}} & \frac{4 p _0}{3} & \frac{2 p _0}{3} \end{array} $$
We can calculate,
$$ \begin{aligned} & p _t=p _0-x+2 x+x=p _0+2 x \ & \text { or } \quad 2 x=p _t-p _0 \quad \text { or } \quad x=\frac{p _t-p _0}{2} \end{aligned} $$
Now for first order reaction,
$$ t=\frac{1}{k} \ln \frac{p _0}{\left(p _0-x\right)} $$
Putting the value of $x$ in the equation,
$$ t=\frac{1}{k} \ln \frac{p _0}{p _0-\left(\frac{p _t-p _0}{2}\right)}=\frac{1}{k} \ln \frac{2 p _0}{2 p _0-p _t+p _0} $$
$$ \begin{aligned} & \text { or } k t=\ln \frac{2 p _0}{\left(3 p _0-p _t\right)} \ & \text { or } \quad k t=\ln 2 p _0-\ln \left(3 p _0-p _t\right) \ & \text { or } \quad \ln \left(3 p _0-p _t\right)=-k t+\ln 2 p _0 \end{aligned} $$
It indicates graph between $\ln \left(3 p _0-p _t\right) v s$ ’ $t$ ’ will be a straight line with negative slope, so option (a) is correct
$$ t _{1 / 3}=\frac{1}{k} \ln \frac{p _0}{p _0 / 3}=\frac{1}{k} \ln 3 $$
It indicates $t _{1 / 3}$ is independent of initial concentration so, option (b) is incorrect.
Likewise, rate constant also does not show its dependence over initial concentration. Thus, graph between rate constant and $[A] _0$ will be a straight line parallel to $X$-axis






