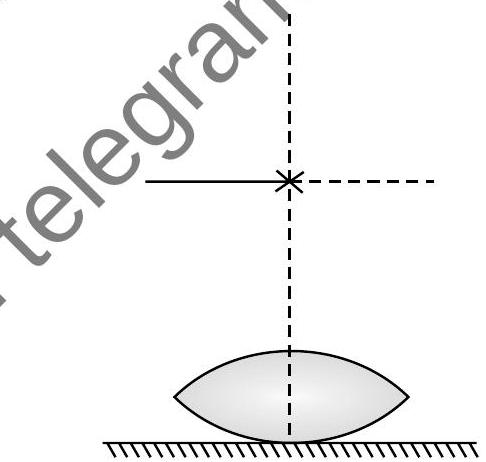
SATHEE: ray-optics-and-optical-instruments Question 35

ray-optics-and-optical-instruments Question 35

Question: Q. 4. A symmetric biconvex lens of radius of curvature $R$ and made of glass of refractive index 1.5 , is placed on a layer of liquid placed on top of a plane mirror as shown in the figure. An optical needle with its tip on the principal axis of the lens is moved along the axis until its real, inverted image coincides with the needle itself. The distance of the needle from the lens is measured to be $x$ . On removing the liquid layer and repeating the experiment, the distance is found to be $y$ . Obtain the expression for the refractive index of the liquid in terms of $x$ and $y$ .

[Delhi & OD 2018; Delhi Comptt. 2014]

Show Answer

Solution:

Ans. Lens maker’s formula $1 / 2$ Formula for ‘combination of lenses’ $1 / 2$ Obtaining the expression for $μ$

(a) Let $μ_{1}$ denote the refractive index of the liquid. When the image of the needle coincides with the lens itself; its distance from the lens, equals the relevant focal length.

With liquid layer present, the given set up, is equivalent to a combination of the given (convex) lens and a concavo plane/plano concave ’liquid lens’.

We have $\frac{1}{f} = (μ - 1) (\frac{1}{R_{1}} - \frac{1}{R_{2}})$

and

$\frac{1}{f} = (\frac{1}{f_{1}} + \frac{1}{f_{2}})$

as per the given data,

$\begin{aligned} \frac{1}{f_{2}} & = \frac{1}{y} = (1 \cdot 5 - 1) (\frac{1}{R} - \frac{1}{(- R)}) 1 / 2 & = \frac{1}{R} \end{aligned}$

$∴ \frac{1}{x} = (μ_{l} - 1) (- \frac{1}{R}) + \frac{1}{y} = \frac{- μ_{l}}{y} + \frac{2}{y} 1 / 2$

$\begin{aligned} ∴ \frac{μ_{1}}{y} = \frac{2}{y} - \frac{1}{x} = (\frac{2 x - y}{x y}) & or μ_{l} = (\frac{2 x - y}{x}) \end{aligned}$

[CBSE Marking Scheme 2018]

Detailed Answer :

Given, Radius of curvature of convex lens is $R$ and $μ$ $= 1.5$

When lens is placed on a layer of water then focal length of combination is $f$ . Thus the first measurement gives the focal length of combination i.e. $f = x$ .

In second measurement, we get the focal length $f_{1}$ of convex lens i.e., $f_{1} = y$ , therefore the focal length of plano convex lens of water $f_{2}$ is given as

$\begin{aligned} (i) & \Rightarrow \frac{1}{f_{1}} + \frac{1}{f_{2}} & = \frac{1}{f} \Rightarrow & \frac{1}{f_{2}} = \frac{1}{f} - \frac{1}{f_{1}} = \frac{1}{x} - \frac{1}{y} = \frac{y - x}{x y} \Rightarrow & f_{2} = \frac{x y}{y - x} \end{aligned}$

For plano convex lens of liquid of refractive index $μ_{l}$

$\frac{1}{f_{2}} = (μ_{l} - 1) (\frac{1}{R_{1}} - \frac{1}{R_{2}}) = (μ_{l} - 1) (- \frac{1}{R} - \frac{1}{\infty})$

$\Rightarrow \frac{1}{f_{2}} = - \frac{(μ_{l} - 1)}{R}$

$\Rightarrow μ_{l} = 1 - \frac{R}{f_{2}}$

Now from eqn. (i) and (ii),

$μ_{l} = 1 - \frac{R}{x y / (y - x)} = 1 - \frac{R (y - x)}{x y}$

Now again for plano convex lens of air, if image coincide at $2 f_{1}$ then for case II we have

$\begin{matrix} (iv) & \begin{array}{ll} \Rightarrow & 2 f_{1} = R \Rightarrow & f_{1} = \frac{R}{2} again & y = 2 f_{1} = 2 \times \frac{R}{2} \Rightarrow & y = R \end{array} \end{matrix}$

Now from eqn. (iii) and (iv),

$\begin{aligned} μ_{l} = 1 - \frac{y (y - x)}{x y} = 1 - \frac{y - x}{x} & μ_{l} = \frac{2 x - y}{x} \end{aligned}$

This is the required expression for the refractive index of liquid.

Ray Optics And Optical Instruments

ray-optics-and-optical-instruments Question 35

Detailed Answer :

Table of Contents

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Ray Optics And Optical Instruments

ray-optics-and-optical-instruments Question 35

Detailed Answer :

Table of Contents

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Menu