Solution:

Ans.

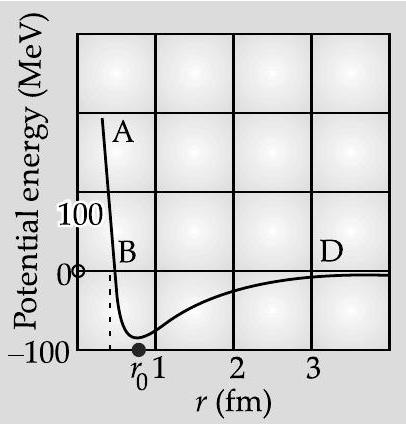
For $r<O B$, force is repulsive.

For $r>O B$, force is attractive.

(i) Nuclear forces are very strong. They are much stronger as compared to gravitational or coulomb forces.

(ii) They are chafgeindependent forces.

1

[CBSE Marking Scheme 2012]

TOPIC-2

Radioactivity and Nuclear Reactor

Revision Notes

• Radioactivity: When atoms become very heavy, neutrons are unable to bind and some nucleons keep on leaving the nucleus. These atoms/elements are known as radioactive elements and process of spontaneous ejection of nucleons or radiations is known as radioactivity.

There are three types of radiodactive decay in nature.

• $\alpha$-decay : In this decay $\alpha$-particles $\left({ }_{2}^{4} \mathrm{He}\right.$ nucleus) eject out.
• $\beta$-decay : In this decay electrons or positrons ( particles with the same mass as electrons, but with a charge exactly opposite to that of electron) eject out.
• $\gamma$-decay : In this high energy photons are emitted but no loss in $Z$ & $A$.

$>$ Properties of $\alpha$-rays :

• $\alpha$-rays consist of doubly ionised helium atoms.
• After $\alpha$-decay a nucleus is transformed into another nucleus.

Examples :

$$ \begin{gathered} { }{Z}^{A} \mathrm{X} \rightarrow{ }{\mathrm{Z}-2}^{A-4} \mathrm{Y}+{ }{2}^{4} \mathrm{He} \ { }{92}^{238} \mathrm{U} \rightarrow{ }{90}^{234} \mathrm{Th}+{ }{2}^{4} \mathrm{He} \end{gathered} $$

• The difference between the initial mass energy product and the final mass energy of the decay products is called the $Q$ value of the process or the disintegration energy. Thus, the $Q$ value of an alpha decay can be expressed as

Properties of $\alpha$-rays :

$$ Q=\left(m_{X}-m_{Y}-m_{\mathrm{He}}\right) c^{2} $$

• $\alpha$-rays are deflected by the electric field and the magnetic field.
• The velocity of $\alpha$-rays is about $10 %$ of the velocity of light.
• $\alpha$-rays affect the photographic plate.
• In $\alpha$-decay; mass number of daughter nucleus changes by 4 units and atomic number changes by 2 units.

Properties of $\beta$-rays :

• $\beta$-rays consist of fast moving electrons or positrons.
• In beta minus $\left(\beta^{-}\right)$decay, an electron is emitted by the nucleus. In this a neutron is converted into proton and electron and electron is emitted out along with antineutrino. Hence there is no change in mass number and +1 change in atomic number.

Example :

$$ { }{15}^{32} \mathrm{P} \rightarrow{ }{16}^{32} \mathrm{~S}+{ }{-1} e^{0}+\bar{v} \quad\left(T{1 / 2}=14.3 d\right) $$

• In beta plus $\left(\beta^{+}\right)$decay, a positron is emitted by the nucleus. In this a proton is converted into neutron and positron is emitted out along with neutrino. Hence there is no change in mass number and -1 change in atomic number.

Example :

$$ { }{11}^{22} \mathrm{Na} \rightarrow{ }{10}^{22} \mathrm{Ne}+{ }{1} e^{0}+v \quad\left(T{1 / 2}=2.6 y\right) $$

• In these equations $v, \bar{v}$ are known as neutrino and anti neutrino particles respectively. They have no charge, approximately no mass and unreactive.
• $\beta$-rays are deflected by the electric field and the magnetic field.
• The velocity of $\beta$-rays is up to $90 %$ of the velocity of light.
• $\beta$-rays affect the photographic plate.

Properties of $\gamma$-rays

• There are energy levels in nucleus also.
• When a excited state nucleus makes a transition to a lower energytate, it radiate electromagnetic radiation. As the energy differences between levels in a nucleus are of theorder of $\mathrm{MeV}$, the emitted photons are of $\mathrm{MeV}$ energies and are called gamma rays.
• Most radionuclides after an alpha decay or a beta decay leave the daughter nucleus in an excited state. Then this excited daughter nucleus come to ground state by fatiating $\gamma$-rays.
• $\gamma$-rays are photons of very short wavelength of the order of $10^{-11} \mathrm{~m}$ to $10^{-13} \mathrm{~m}$.
• $\gamma$-rays carry no charge and hence are not deflected by the electric field and the magnetic field.
• $\gamma$-rays move with the speed of light.
• $\gamma$-rays affect the photographic plate.

Laws of Radioactive decay :

• Rate of decay

$$ \frac{\Delta N}{\Delta t} \propto N $$

where, $N=$ number of nuclei in the sample

For very small time interval

$$ \frac{d N}{d t}=-\lambda N $$

where, $\lambda$ is disintegration constant

Integrating on bothsides,

$$ \begin{equation*} N(t)=N_{0} e^{-\lambda t} \tag{i} \end{equation*} $$

• Rate of disintegration, Differentiating $e q^{n}$ (i)

$$ \begin{array}{ll} \text { or } & R=\lambda N_{0} e^{-\lambda t} \ \text { where, } & R=\lambda N \ & R=\frac{-d N}{d t} \end{array} $$

Often rate of disintegration $R(=-d N / d t)$ is more important than $N$ itself. It gives us the number of nuclei decaying per unit time.

Alternative form of law of Radioactive decay:

$$ R=R_{0} e^{-\lambda t} $$

where, $R_{0}$ is the radioactive decay rate at time $t=0$, and $R$ is the rate at any subsequent time $t$.

The SI unit for rate of radioactive decay is becquerel. One becquerel $(\mathrm{Bq})$ is one decay per second.

Measurement of life of radionuclide :

$$ 1 \text { curie }=3.7 \times 10^{10} \mathrm{~Bq} $$

• Half lifetime $\left(T_{1 / 2}\right.$ : It is the time period in which both $N$ and $R$ reduce half of initial quantity.

$$ T_{1 / 2}=\frac{\ln 2}{\lambda}=\frac{0.693}{\lambda} $$

D Mean life $(\tau)$ : It is the time at which both $N$ and $R$ have been reduced to $e^{-1}$ of their initial values.

$$ \tau=1 / \lambda $$

Relation between half life time and mean life :

$$ T_{1 / 2}=\frac{\ln 2}{\lambda}=\tau \ln 2 $$

We may also derive from above formulae of half lifetime and radioactive decay rate that

$$ \frac{N}{N_{0}}=\left(\frac{1}{2}\right)^{n} $$

where, $n=\frac{t}{T_{1 / 2}}$

Nuclear Energy by artificial Fission and Fusion processes

• Fission : When a heavy nucleus is broken into two smaller nuclei, the process is known as fission. In this process huge amount of energy is released.

When a neutron was bombarded on a uranium target, the uranium nucleus broke into two nearly equal fragments releasing huge amount of energy.

• Some combination of products of above reaction are

$$ \begin{aligned} & { }{0}^{1} n+{ }{92}^{235} \mathrm{U} \rightarrow{ }{92}^{236} \mathrm{U} \rightarrow{ }{56}^{144} \mathrm{Ba}+{ }{36}^{89} \mathrm{Kr}+3{ }{0}^{1} n \ & { }{0}^{1} n+{ }{92}^{235} \mathrm{U} \rightarrow{ }{92}^{236} \mathrm{U} \rightarrow{ }{51}^{133} \mathrm{Ba}+{ }{41}^{99} \mathrm{Nb}+4{ }{0}^{1} n \ & { }{0}^{1} n+{ }{92}^{235} \mathrm{U} \rightarrow{ }{54}^{140} \mathrm{Xe}+{ }{38}^{94} \mathrm{Sr}+2{ }_{0}^{1} n \end{aligned} $$

• The energy released (the $Q$ value ) in the fission reaction of nuclei like uranum is of the order of $200 \mathrm{MeV}$ per fissioning nucleus.

Nuclear Reactor :

• When ${ }{92}^{235} \mathrm{U}$ undergoes a fission after bombarded by a neutron, it also releases an extra neutron. This extra neutron is then available for initiating fission of another ${ }{92}^{235} \mathrm{U}$. Hence this is a chain reaction. Controllable

form of chain reaction is used in nuclear reactor.

• Reason for not having a chain reaction in efficient way
• Poor concentration of Uranium

Solution : Enrichment of ore.

• Speed of neutrons is very high hence theypenetrate through Uranium, without having fission reaction.

Solution : Convert high energy neutron to thermal neutron by collision with moderator.

• Controlling the rate of reaction.

Solution : Insertion of controlrods so that they absorb excess neutrons and rate of reaction $\mathrm{K}$ is equal to one.

Benefits of Nuclear reactor :

• Huge energy from small quantity of uranium. It’s a good replacement of coal reactor.

Risks of Nuclear reactor :

• Uncontrollable blast
• Radiation leakage Oranium waste is also radioactive.

Nuclear Fusion: Two light nuclei (low Binding Energy per Nucleon) join and form one nucleus of higher Binding Energy per Nucleon, energy is released. This process is known as Fusion.

Some Examples of nuclear fusion are

$$ \begin{aligned} & { }{1}^{1} \mathrm{H}+{ }{1}^{1} \mathrm{H} \rightarrow{ }{1}^{2} \mathrm{H}+e^{+}+v+0.42 \mathrm{MeV} \ & { }{1}^{2} \mathrm{H}+{ }{1}^{2} \mathrm{H} \rightarrow{ }{2}^{3} \mathrm{He}+n+3.27 \mathrm{MeV} \ & { }{1}^{2} \mathrm{H}+{ }{1}^{2} \mathrm{H} \rightarrow{ }{1}^{3} \mathrm{H}+{ }{1}^{1} \mathrm{H}+4.03 \mathrm{MeV} \end{aligned} $$

Stars have fusion energy.

• Fusion process gives more energy than fission process. In the above examples of fusion and fission, energy from one unit mass by fusion is $6.7 \mathrm{MeV}$ while from fission it is $1 \mathrm{MeV}$

Advantages of Nuclear fusion reactor :

• It’s a clean fuel. No radioactive wastage in this process.
• Hydrogen is available in plenty.

Problems of Nuclear fusion reactor:

• Cannot be stopped unless the whole stock is burnt.
• Storage of hydrogen plasma.

Hydrogen bomb is uncontrollable nuclear fusion reaction.

Thermal nuclear fusion reaction in Sun : Fusion of hydrogen nuclei into helium nuclei is the source of energy of all stars including our sun.

$$ \begin{equation*} { }{1}^{1} \mathrm{H}+{ }{1}^{1} \mathrm{H} \rightarrow{ }_{1}^{2} \mathrm{H}+e^{+}+v+0.42 \mathrm{MeV} \tag{i} \end{equation*} $$

$$ \begin{align*} e^{+}+e^{-} & \rightarrow \gamma+\gamma+1.02 \mathrm{MeV} \tag{ii}\ { }{1}^{2} \mathrm{H}+{ }{1}^{1} \mathrm{H} & \rightarrow{ }{2}^{3} \mathrm{He}+\gamma+5.49 \mathrm{MeV} \tag{iii}\ { }{2}^{3} \mathrm{H}+{ }{2}^{3} \mathrm{H} & \rightarrow{ }{2}^{4} \mathrm{He}+{ }{1}^{1} \mathrm{H}+{ }{1}^{1} \mathrm{H}+12.86 \mathrm{MeV} \tag{iv} \end{align*} $$

The combined effect of above reactions is

$$ 4{ }{1}^{1} \mathrm{H}+2 e^{-} \rightarrow{ }{2}^{4} \mathrm{He}+2 v+6 \gamma+26.7 \mathrm{MeV} $$

Know the Formulae

Law of Radioactive decay :

Rate of disintegration

$$ N=N_{o} e^{-\lambda t} $$

or

$$ \begin{aligned} & R=\lambda N_{o} e^{-\lambda t} \ & R=\lambda N \end{aligned} $$

Alternative form of law of Radioactive decay:

Measurement of life of radionuclide :

$$ R=R_{0} e^{-\lambda t} $$

Half lifetime $\left(T_{1 / 2}\right)$ :

$$ T_{1 / 2}=\frac{\ln 2}{\lambda}=\frac{0.693}{\lambda} $$

Mean life :

$$ \tau=\frac{1}{\lambda} $$

$$ T_{1 / 2}=\frac{\ln 2}{\lambda \lambda}=\tau \ln 2 $$

? Objective Type Questions

where, $n=\frac{t}{T_{1 / 2}}$