Solution:

Ans. (i) Ampere’s circuital law states that the line integral

of magnetic field around a closed path is $\mu_{0}$ times o

total current enclosed by the path, $\oint \vec{B} \cdot \overrightarrow{d l}=\mu_{0} I$

where,

$B=$ magnetic field

$d l=$ infinitesimal segment of the path

$\mu_{0}=$ permeability of free space.

$I=$ enclosed electric current by the path

Magnefic field at a point will not depend on the

shape of Amperian loop and will remain same at every point on the loop.

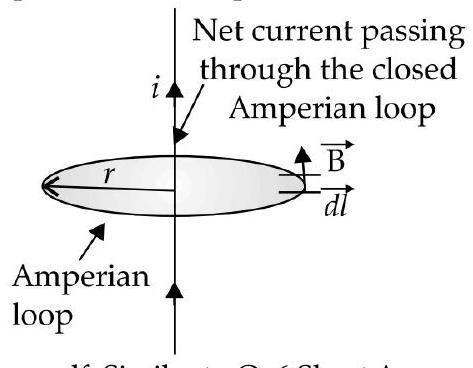
(ii) Try yourself, Similar to Q. 6 Short Answer Type-II

AI] Q. 2. (i) State Ampere’s circuital law. Use this law to obtain the expression for the magnetic field inside an air cored toroid of average radius , having ’ $n$ ’ turns per unit length and carrying a steady current $I$.

(ii) An observer to the left of a solenoid of $N$ turns each of cross section area ’ $A$ ’ observes that a steady current $I$ in it flows in the clockwise direction. Depict the magnetic field lines due to the solenoid specifying its polarity and show that it acts as a bar magnet of magnetic moment $m=$ NIA.

R U [Delhi I, II, III 2015]

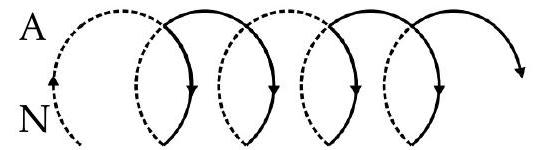
Ans.(i) Line integral of magnetic field over a closed loop is equal to the $\mu_{0}$ times the total current passing through the surface enclosed by the loop.

Alternatively : $\quad \oint \vec{B} \cdot \overrightarrow{d l}=\mu_{0} I$

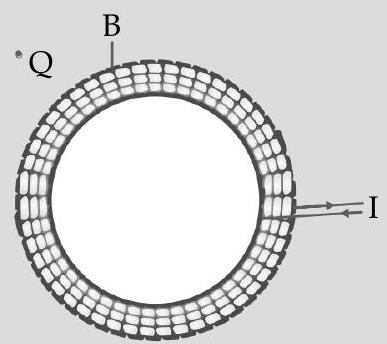
(a)

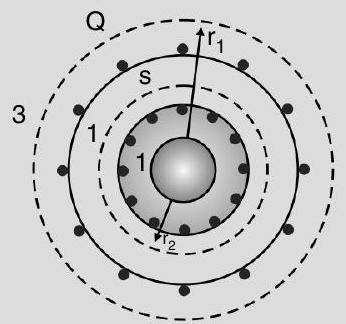
(b)

Let the current flowing through each turn of the toroid be $I$. The total number of turns equals $n$. $(2 \pi r)$ where, $n$ is the number of turns per unit length.

Applying Ampere’s circuital law, for the Amperian loop, for interior points.

$$ \begin{array}{rlrl} & & \oint \vec{B} \cdot \overrightarrow{d l} & =\mu_{0}(n \cdot 2 \pi r l) \ & B \times 2 \pi r & =\mu_{0} n 2 \pi r I \end{array} $$

(ii)

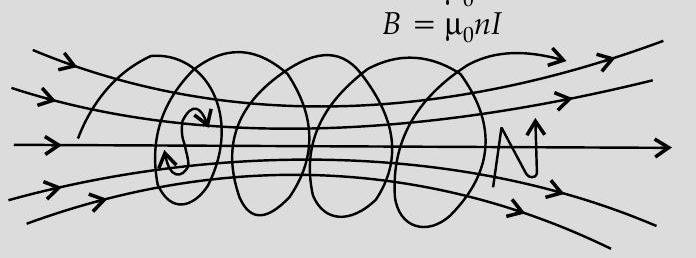
The solenoid contains $N$ loops, each carrying a current $I$. Therefore, each loop acts as a magnetic dipole. The magnetic moment for a current $I$, flowing in loop of area (vector) $A$ is given by $m=I A$

The magnetic moments of all loops are aligned along the same direction. Hence, net magnetic moment equals to NIA.

Ans. (i) Ampere’s circuital law deters that the cloned-losp intigral of the

the current passing through the loop.

$$ \text { Mathematically, } \oint \vec{B} \cdot \vec{d}=\mu_{0} I $$

Total incoming current $=$ Total out going lurrent

$\therefore I_{\text {rut }}=0$

For loop II,

Applying ampere’s circuital law.

The magnetic files lines will be, as shown $\therefore$ Field line best in from North.

heme end B is Noria end $A$ is South

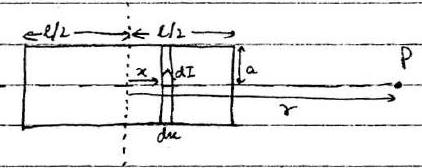
Consider a solenoid as shown Now tate an elementary lop of width che.

for this loop, yield at $P$,

$$ d B= $$

then cement in each torn = I. then of no. of turn per vent length =n current in pathourn = cement in each torn = $I$. . of turn per vent length cement in paction =

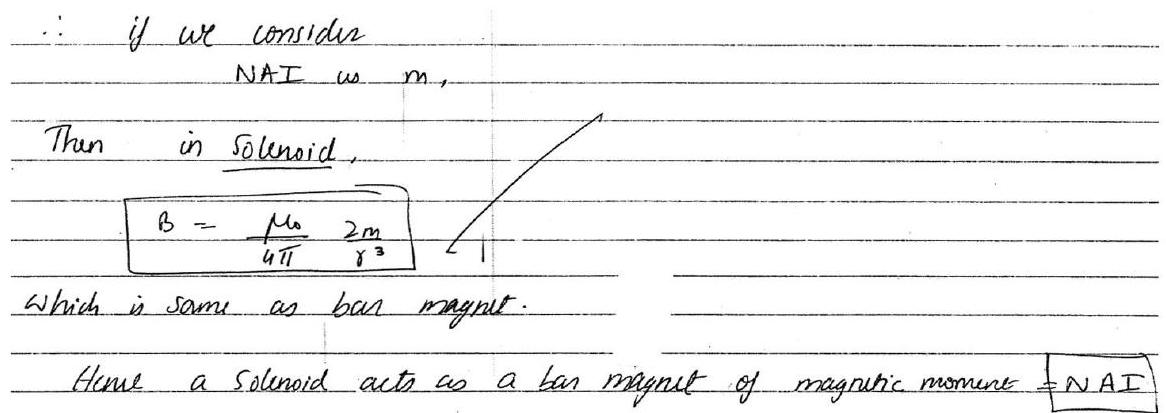
then no. of torn per unit length cement is each torn $=I$.

$$ \begin{aligned} d B & =\frac{\mu_{0} n I d x a^{2}}{2\left(a^{2}+(x-x)^{2}\right)^{3 / 2}} \ & \because r \gg x, a \ & \therefore\left(a^{2}+(x-x)^{2}\right)^{3 / 2} \approx r^{3} \ \int_{0}^{B} d B & =\int_{-4 L} \frac{\mu_{0} n I d x a^{2}}{2 r^{3}} \ B & =\frac{\mu_{0} n I a^{2} l}{2 r^{3}} \ & =\frac{\mu_{1}}{4 \pi} \frac{2 n I l \pi a^{2}}{r^{3}}=\frac{\mu_{0}}{4 \pi} 2 \times \frac{\mathrm{NAI}}{r^{3}} \end{aligned} $$

$$ \begin{aligned} {[\because n \times l} & =N \ \pi a^{2} & =A] \end{aligned} $$

for a bar magnet.

$$ B=\frac{\mu}{4 \pi} \frac{2 m}{r^{3}} \text { (on avis) } $$

[Topper’s Answer 2015]