moving-charges-and-magnetism Question 25
Question: Q. 1. Find the condition under which the charged particles moving with different speeds in the presence of electric and magnetic field vectors can be used to select charged particles of a particular speed.
R [O.D. I, II, III 2017]
Show Answer
Solution:
Ans. (i) For directions of $\vec{E}, \vec{B}, \vec{v}$
(ii) For magnitudes of $\vec{E}, \vec{B}, \vec{v}$
(i) The velocity $\vec{v}$ of the charged particles, and the $\vec{E}$ and $\vec{B}$ vectors, should be mutually perpendicular. $1 / 2$ Also the forces on $q$, due to $\vec{E}$ and $\vec{B}$ must be oppositely directed. (Also accept if the student draws a diagram to show the directions.)
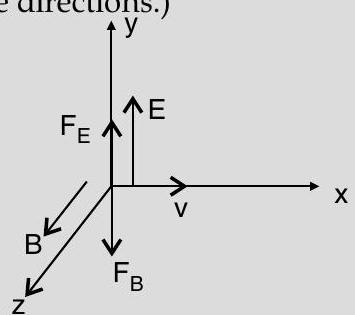
(ii) $q E=q v B$
$1 / 2$
$v=\frac{E}{B}$
$1 / 2$
[Alternatively, The student may write :
Force due to electric field $=q \vec{E}$
Force due to magnetic field $=q(\vec{v} \times \vec{B})$
The required condition is
$$ \begin{align*} q \vec{E} & =-q(\vec{v} \times \vec{B}) \ {[\text { or } \vec{E}} & =-(\vec{v} \times \vec{B})=(\vec{B} \times \vec{v})] \end{align*} $$
(Note : Award 1 mark only if the student just writes :
“The forces, on the charged particle, due to the electric and magnetic fields, must be equal and opposite to each other”)
[CBSE Marking Scheme 2017]
Detailed Answer :
If a charged particle travels in crossed electric and magnetic field, then both fields are perpendicular to each other.
Force on the particle will be :
$$ \begin{aligned} & \vec{F}=\overrightarrow{F_{E}}+\overrightarrow{F_{B}} \ & \vec{F}=\overrightarrow{q E}+q(\vec{v} \times \vec{B}) \end{aligned} $$
As $F_{B}$ is $180^{\circ}$ to $F_{E}$, so
$$ \begin{aligned} & \vec{F}=\overrightarrow{q E}-q(\vec{v} \times \vec{B}) \ & \vec{F}=q\left(E-v_{v} B\right) \hat{j} \end{aligned} $$
Now to select a particle, making resultant force as zero, hence
Now,
$$ 0=q(E-v B) \hat{j} $$
Hence,
$$ \begin{aligned} E-v B & =0 \ E & =v B \end{aligned} $$
Hence,
$$ v=\frac{E}{B} $$
