electrostatic-potential-and-capacitance Question 49
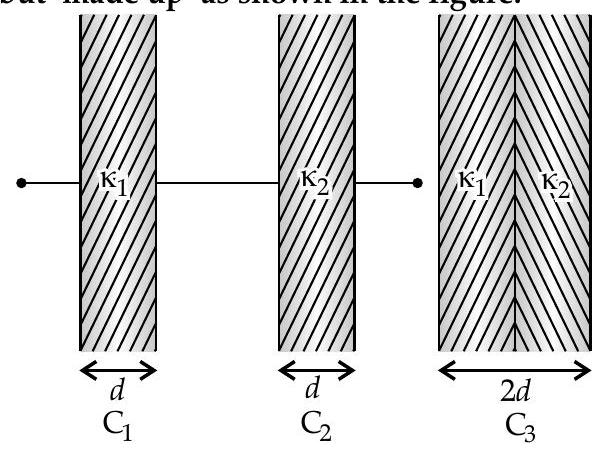
Question: Q. 25. The capacitors $C_{1}$ and $C_{2}$ having plates of area $A$ each, are connected in series, as shown. Compare the capacitance of this combination with the capacitor $C_{3}$, again having plates of area $A$ each, but ‘made up’ as shown in the figure.
Show Answer
Solution:
Ans. We have
U] [CBSE SQP 2013]
$$ \begin{aligned} & \text { and } \quad C_{2}=\frac{A \varepsilon_{0} \kappa_{2}}{d} \ & \therefore \quad C_{e q}=\frac{C_{1} C_{2}}{C_{1}+C_{2}}=\frac{A \varepsilon_{0}}{d}\left(\frac{\kappa_{1} \kappa_{2}}{\kappa_{1}+\kappa_{2}}\right) \quad 1 / 2 \end{aligned} $$
Now, capacitor $\mathrm{C}{3}$ can be considered as made up of two capacitors $C{1}$ and $C_{2}$, each of plate area $A$ and separation $d$, connected in series.
$$ \begin{aligned} & \text { We have } \quad C_{1}{ }^{\prime}=\frac{A \varepsilon_{0} \kappa_{1}}{d} \ & \text { and } \quad C_{2}{ }^{\prime}=\frac{A \varepsilon_{0} K_{2}}{d} \ & \Rightarrow \quad C_{3}=\frac{\mathrm{C}{1}{ }^{\prime} \mathrm{C}{2}^{\prime}}{\mathrm{C}{1}{ }^{\prime}+\mathrm{C}{2}{ }^{\prime}}=\frac{A \varepsilon_{0}}{d}\left(\frac{\kappa_{1} \kappa_{2}}{\kappa_{1}+\kappa_{2}}\right) \end{aligned} $$
$$ \therefore \quad \frac{C_{3}}{C_{e q}}=1 $$
Hence, the net capacitance of the combination is equal to that of $C_{3}$.
