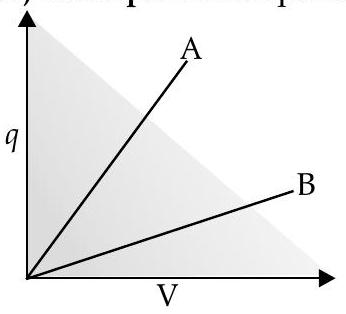
SATHEE: electrostatic-potential-and-capacitance Question 31

electrostatic-potential-and-capacitance Question 31

Question: Q. 6. The given graph shows variation of charge $q$ versus potential difference $V$ for two capacitors $C_{1}$ and $C_{2}$ . Both the capacitors have same plate separation but plate area of $C_{2}$ is greater than that of $C_{1}$ . Which line ( $A$ or $B$ ) corresponds to $C_{1}$ and why?

[Comptt. O.D. I, II, III 2014]

Show Answer

Solution:

Ans. For $C_{1}$

Line $B$

Since slope $(q / V)$ of Line $B$ is lesser than that of Line $A$ .

[CBSE Marking Scheme 2014] 1 (AI Q. 1. Two identical parallel plate capacitors $A$ and $B$ are connected to a battery of $V$ volts with the switch $S$ closed. The switch is now opened and the free space between the plates of the capacitors is filled with a dielectric of dielectric constant $κ$ . Find the ratio of the total electrostatic energy stored in both capacitors before and after the introduction of the dielectric.

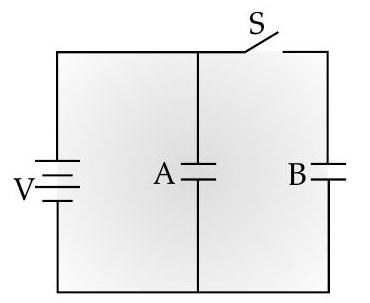
U [O.D. I, II, III 2017]

Ans. Formula for energy stored Energy stored before $1 / 2$

Energy stored after

Ratio

$Energy stored = \frac{1}{2} C V^{2} = (\frac{1}{2} \frac{Q^{2}}{C}) 1 / 2$

Net capacitance with switch $S$ closed

$\begin{aligned} = C + C = 2 C Energy stored & = \frac{1}{2} \times 2 C \times V^{2} = C V^{2} \end{aligned}$

After the switch $S$ is opened, capacitance of each capacitor $= κ \times C$

$∴$ Energy stored in capacitor $A$

$A = \frac{1}{2} κ C V^{2}$

For capacitor $B$ ,

$\begin{aligned} Energy stored & = \frac{1}{2} \frac{Q^{2}}{κ C} = \frac{1}{2} \frac{C^{2} V^{2}}{κ C} & = \frac{1}{2} \frac{C V^{2}}{κ} ∴ Total Energy stored & = \frac{1}{2} κ C V^{2} + \frac{C V^{2}}{κ} & = \frac{1}{2} C V^{2} (\frac{κ^{2} + 1}{κ}) Required ratio & = \frac{2 C V^{2} κ}{C V^{2} (κ^{2} + 1)} & = \frac{2 κ}{(κ^{2} + 1)} \end{aligned}$

[CBSE Marking Scheme 2017]

Electrostatic Potential And Capacitance

electrostatic-potential-and-capacitance Question 31

Table of Contents

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Electrostatic Potential And Capacitance

electrostatic-potential-and-capacitance Question 31

Table of Contents

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Menu