electric-charges-and-fields Question 29
Question: Q. 2. (i) Derive the expression for electric field at a point on the equatorial line of an electric dipole.
(ii) Two point charges $+4 \mu \mathrm{C}$ and $+1 \mu \mathrm{C}$ are separated by distance of $2 \mathrm{~m}$ in air. Find the point on the line joining charges at which the net electric field of the system is zero? U [O.D. (Comptt.) I, II, III 2017]
Show Answer
Solution:
Ans. (i) Derivation of expression for electric field.
(ii) Finding point on line joining charges 2
[CBSE Marking Scheme, 2017]
Detailed Answer :
(i) Try yourself, Similar to Q. 1 (i), Short Answer Type Questions-II.
(ii) Two point charges $q_{A}=+4 \mu \mathrm{C}$ and $q_{B}=+1 \mu \mathrm{C}$ are located $2 \mathrm{~m}$ apart in air as shown. $P$ be a point, at a distance $x$ from $A$, where electric field of the system is zero.
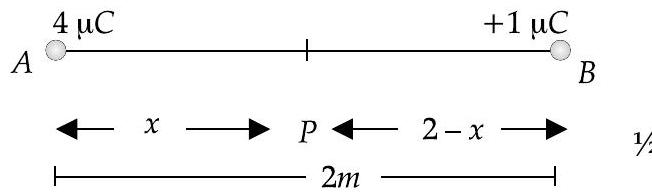
Distance between the two charges, $A B=2 \mathrm{~m}$
$\therefore \quad \mathrm{PA}=x \mathrm{~m}, \mathrm{~PB}=(2-x) \mathrm{m}$
Electric field at point $P$ caused by $+4 \mu \mathrm{C}$ charge,
$$ E_{1}=\frac{4 \times 10^{-6}}{4 \pi \varepsilon_{0}(P A)^{2}} \mathrm{~N} / \mathrm{C} \text { along } P A \quad 1 / 2 $$
Magnitude of electric field at point $P$ caused by $+1 \mu \mathrm{C}$ charge
$E_{2}=\frac{+1 \times 10^{-6}}{4 \pi \varepsilon_{0}(P B)^{2}} \mathrm{~N} / \mathrm{C}$ along $P B$
Now, $\frac{4 \times 10^{-6}}{4 \pi \varepsilon_{0}(x)^{2}}=\frac{+1 \times 10^{-6}}{4 \pi \varepsilon_{0}(2-x)^{2}}$
(Since net electric field at $P$ is zero) $\mathbf{1}$ $(2-x)^{2} 4=(x)^{2} 1$
$16-16 x+3 x^{2}=0$
$4(4-x)-3 x(4-x)=0$
$(4-3 x)(4-x)=0$
$x=\frac{4}{3}, x=4$, possible point on the line will be $x=\frac{4}{3} 1 / 2$
AI Q. 3. (a) Derive an expression for the electric field $E$ due to a dipole of length ’ $2 a^{\prime}$ at a point distant $r$ from the centre of the dipole on the axial line.
(b) Draw a graph of $E$ versus $r$ for $r»a$.
(c) If this dipole were kept in a uniform external electric field $E_{0}$, diagrammatically represent the position of the dipole in stable and unstable equilibrium and write the expressions for the torque acting on the dipole in both the cases.
R [O.D. Set III 2017]
| Ans. $n)$ | $\xrightarrow{\vec{D}}$ |
|---|---|
| $-1-\leq-10$ | |
| $a \quad a \quad E-q$ | |
| 二. | |
| $\infty$ (n) | $\xrightarrow{\mathrm{p}}$ |
| $-1-1-10$ | |
| $i$ a $+q \quad E-q$ | |
| $\therefore 1-x-1$ | |
| Considee a depole haverig depole momenc | |
| P. Ececteic fceld due to $-q$ at the pocnt $P$ is along $p Q$. |
|
| $E=1 q$ along $P B$ | |
| $-q \quad 4 \pi \varepsilon_{0}(\gamma+a)^{2}$ | |
| Fectsid fceld due to tq at the pocnl $P$ | |
| $\therefore$ along $P A$ ? | |
| $E_{t a}=\frac{1}{4 \pi \varepsilon_{0}} \frac{q}{(\gamma-a)^{2}} a \operatorname{long} p A$ |
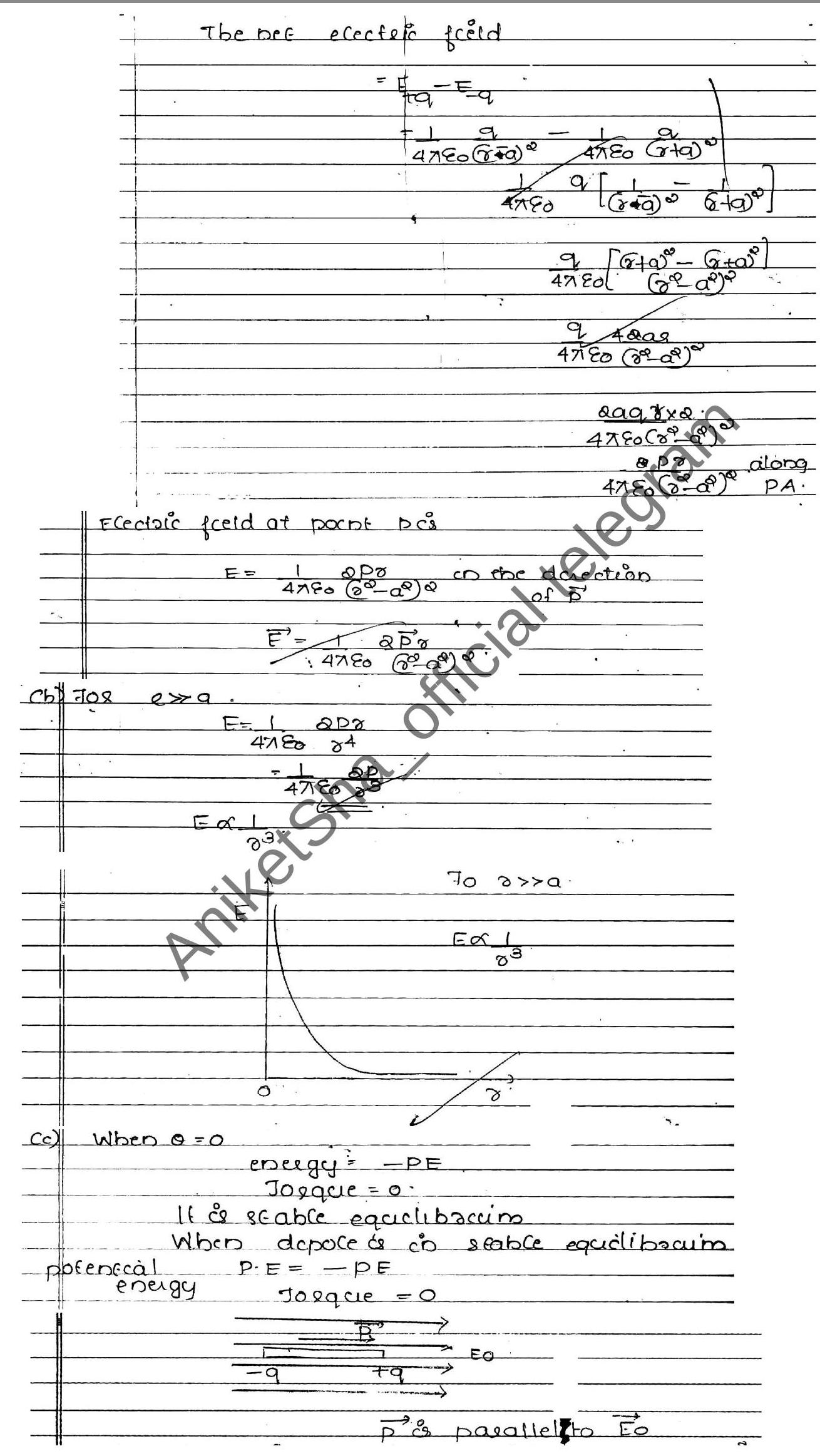
[Topper’s Answer 2017]
