electric-charges-and-fields Question 22
Question: Q. 5. Two thin concentric and coplanar spherical shells, of radii
(i)
(ii)
(iii)
A [Comptt. Delhi, 2016]
Show Answer
Solution:
Ans.
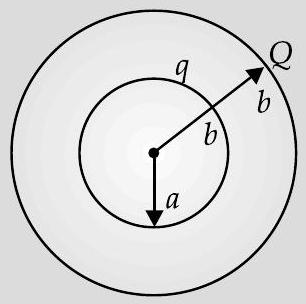
(i) For
Point lies inside both the spherical shells. Hence,
(ii) For
Point is outside the spherical shell of radius ’
1
(iii) For
Point is outside of both the spherical shells. Total effective charge at the centre equals
[CBSE Marking Scheme, 2016]






