dual-nature-of-radiation-and-matter Question 35
Question: Q. 11.8, Page 70]
Show Answer
Solution:
Ans. Correct option : (c)
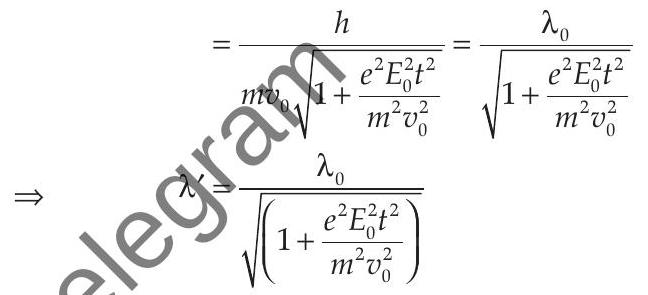
Explanation : According to the problem de-Broglie wavelength of electron at time $t=0$ is $\lambda_{0}=\frac{h}{m v_{0}}$
Electrostatic force on electron in electric field is
$\vec{F}{e}=-e \vec{E}=-e E{0} \hat{j}$
The acceleration of electron, $\vec{a}=\frac{\vec{F}}{m}=-\frac{e E_{0}}{m} \hat{j}$
It is acting along negative $y$-axis.
The initial velocity of electron along $x$-axis, $v_{x_{0}}=v_{0} \hat{i}$ This component of velocity will remain constant as there is no force on electron in this direction. Now considering $y$-direction. Initial velocity of electron along $y$-axis, $v_{y 0}=0$ Velocity of electron after time $t$ along $y$-axis
$$ v_{y}=0+\left(-\frac{e E_{0}}{m} \hat{j}\right) t=-\frac{e E_{0}}{m} t \hat{j} $$
Magnitude of velocity of electron after time $t$ is
$$ \begin{aligned} v & =\sqrt{v_{x}^{2}+v_{y}^{2}}=\sqrt{v_{0}^{2}+\left(\frac{-e E_{0}}{m} t\right)^{2}} \ \Rightarrow \quad & =v_{0} \sqrt{1+\frac{e^{2} E_{0}^{2} t^{2}}{m^{2} v_{0}^{2}}} \end{aligned} $$
de-Broglie wavelength, at $t=0$ is $\lambda_{0}=\frac{h}{m v_{0}}$
de-Broglie wavelength at $t=t$ is $\lambda^{\prime}=\frac{h}{m v}$
