SATHEE: current-electricity Question 46

current-electricity Question 46

Question: Q. 7. The galvanometer, in each of the two given circuits, does not show any deflection. Find the ratio of the resistors 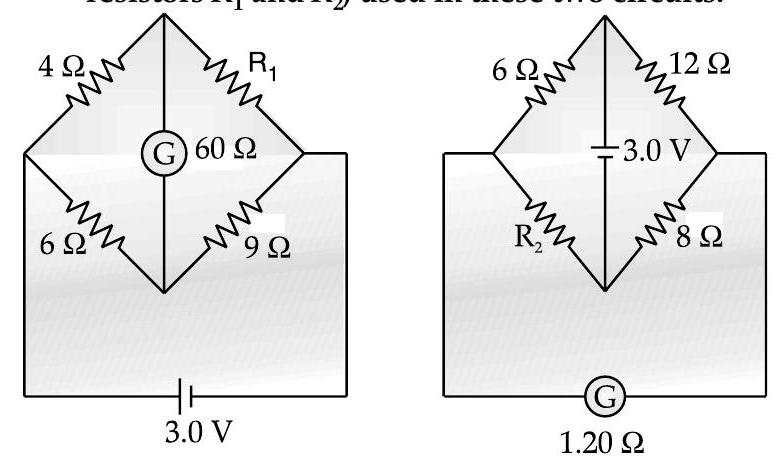$R_{1}$ and $R_{2}$ , used in these two circuits.

A [CBSE SQP 2013]

Show Answer

Solution:

Ans. For circuit 1, we have, (from the Wheatstone bridge balance condition),

$\begin{aligned} \frac{4}{R_{1}} & = \frac{6}{9} R_{1} & = 6 Ω \end{aligned}$

In circuit 2, the interchange of the positions of the battery and the galvanometer, does not change the (Wheatstone Bridge) balance condition.

$\begin{array}{ll} ∴ & \frac{R_{2}}{8} = \frac{6}{12} or & R_{2} = 4 Ω ∴ & \frac{R_{1}}{R_{2}} = \frac{6}{4} = \frac{3}{2} \end{array}$

$or R_{2} = 4 Ω 1 / 2$

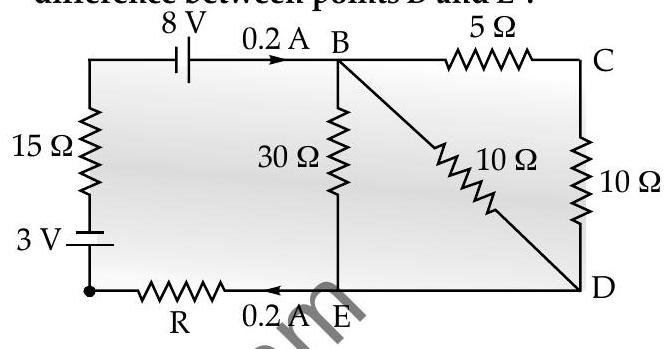
[AI Q. 8. Calculate the value of the resistance $R$ in the circuit shown in the figure so that the current in the circuit is $0.2 A$ . What would be the potential difference between points $B$ and $E$ ?

A [O.D. I, II, III 2012]

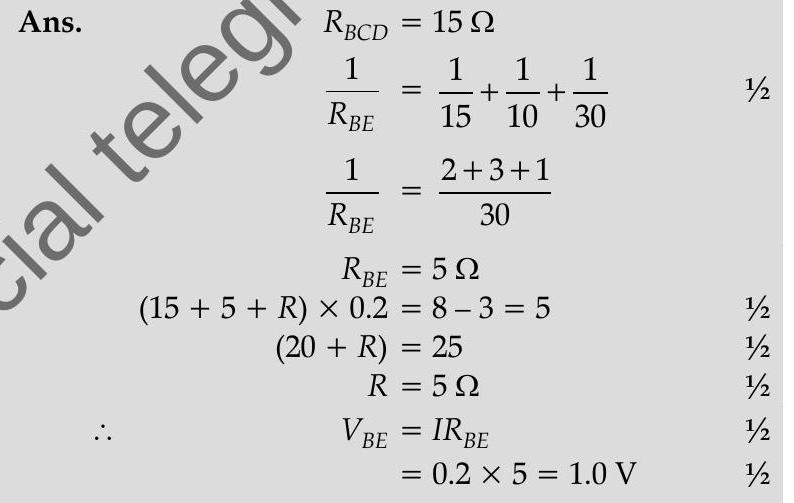
Ans.

$\frac{1}{R_{B E}} = \frac{1}{15} + \frac{1}{10} + \frac{1}{30}$

$\begin{aligned} \frac{1}{R_{B E}} & = \frac{2 + 3 + 1}{30} & R_{B E} & = 5 Ω & (15 + 5 + R) \times 0.2 & = 8 - 3 = 5 & 1 / 2 (20 + R) & = 25 & 1 / 2 R & = 5 Ω & 1 / 2 V_{B E} & = I R_{B E} & 1 / 2 & = 0.2 \times 5 = 1.0 V & 1 / 2 \end{aligned}$

$x$

$‘2$

Long Answer Type Questions

[AI Q. 1. (i) State the two Kirchhoff’s laws. Explain briefly how these rules are justified.

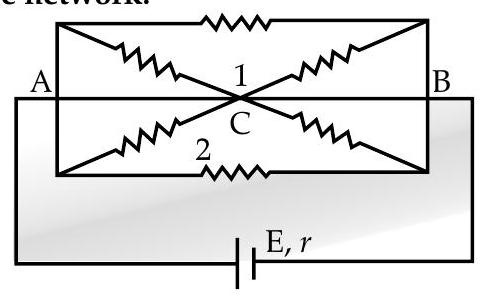
(ii) The current is drawn from a cell of emf $E$ and internal resistance $r$ connected to the network of resistors each of resistance $r$ as shown in the figure. Obtain the expression for (i) the current drawn from the cell and (ii) the power consumed in the network.

R [Delhi Compt I, II, III 2017]

Ans. (i) Try yourself Similar to Q. 1, Short Answer Type Question-I

(ii) Equivalent resistance of the loop

$R = \frac{r}{3}$

[CBSE Marking Scheme, 2012]

Hence current drawn from the cell

$I = \frac{E}{\frac{r}{3} + r} = \frac{3 E}{4 r}$

Power consumed, $P = I^{2} R$

$\begin{aligned} = \frac{9 E^{2}}{16 r^{2}} \times \frac{4 r}{3} & = \frac{3 E^{2}}{4 r} \end{aligned}$

$\begin{aligned} \frac{1}{R_{1}} & = \frac{1}{r} + \frac{1}{2 r} \frac{1}{R_{1}} & = \frac{2 + 1}{2 r} ∴ R_{1} & = \frac{2 r}{3} \end{aligned}$

Further since both mesh 1 and 2 are similar,

$∴ R_{2} = \frac{2 r}{3}$

Now combining resistances $R_{1}$ and $R_{2}$ , we get :

$\begin{aligned} \frac{1}{R} & = \frac{1}{R_{1}} + \frac{1}{R_{2}} ∴ \frac{1}{R} & = \frac{1}{\frac{2 r}{3}} + \frac{1}{\frac{2 r}{3}} \end{aligned}$

(As $R_{1} | | R_{2}$ )

$\begin{matrix} (1) & ∴ R = \frac{r}{3} \end{matrix}$

As the circuit is in series with internal resistance, then resultant resistance will be :

$r^{'} = r + \frac{r}{3} = \frac{4 r}{3}$

Hence the current drawn from the cell will be :

$\begin{matrix} (1) & I = \frac{E}{r^{'}} = \frac{3 E}{4 r} \end{matrix}$

Now power consumed by the network :

$P = I^{2} r^{'}$

$= {(\frac{3 E}{4 r})}^{2} \times \frac{4 r}{3}$

$∴$ Power, $P = \frac{3 E^{2}}{4 r}$

Current Electricity

current-electricity Question 46

Long Answer Type Questions

Table of Contents

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Current Electricity

current-electricity Question 46

Long Answer Type Questions

Table of Contents

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Menu