current-electricity Question 45
Question: Q. 4. The reading of the (ideal) ammeter, in the circuit shown here, is equal too.
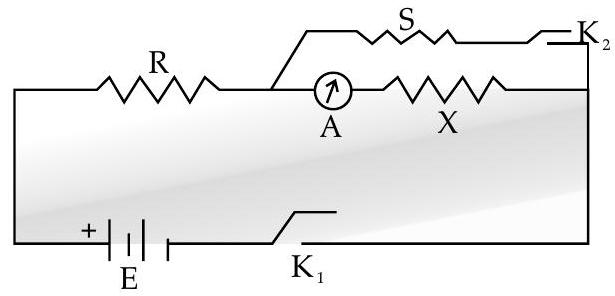
(i) $I$ when key $K_{1}$ is closed but key $K_{2}$ is open.
(ii) $\frac{I}{2}$ when both keys $K_{1}$ and $K_{2}$ are closed.
Find the expression of the resistance of $X$ in terms of the resistances of $R$ and $S$.
U] [Delhi Comptt. 2016]
Show Answer
Solution:
Ans. (i) Current $I$ when $K_{2}$ is open
$$ I=\frac{E}{R+X} $$
(ii) Current $I^{\prime}$ when $K_{2}$ is closed
$$ \left.I^{\prime}=\frac{E}{R+(S X}\right) \frac{E(S+X)}{R(S+X)+S X} \quad 1 / 2 $$
Current flowing through $X$,
$$ \begin{aligned} \frac{I}{2} & =\frac{I^{\prime} S}{S+X}=\frac{E S}{R(S+X)+S X} \ \Rightarrow \quad \frac{E}{2(R+X)} & =\frac{E S}{R(S+X)+S X} \ \Rightarrow \quad 2(R+X) S & =R(S+X)+S X \ 2 R S+2 X S & =R S+R X+S X \ R S & =R X-S X \ X & =\frac{R S}{(R-S)} \end{aligned} $$
$$ \begin{array}{rlrl} +9-3-\frac{3}{2} \times 2 & =V_{A D} \ \Rightarrow \quad V_{A D} & =3 \mathrm{~V} \ {[\text { Alternatively through path } A F D]} \ \frac{3}{2} \times 2 & =V_{A D} \ \Rightarrow \quad V_{A D} & =3 \mathrm{~V} \ \Rightarrow \quad[C B S E & \text { Marking Scheme, 2012] } \end{array} $$
