Solution:

Ans. (i)

(ii) Amplitude Modulation : When a wave is superimposed on a high frequency carrier wave in a manner that the frequency of modulated wave is same as that of the carrier wave, but its amplitude is made proportional to the instantaneous amplitude of the audio frequency modulating voltage, the process is called amplitude modulation (AM).

Let the instantaneous carrier voltage $\left(e_{c}\right)$ and modulating voltage $\left(e_{m}\right)$ be represented by

$$ \begin{align*} e_{c} & =E_{c} \sin \omega_{c} t \tag{i}\ e_{m} & =E_{m} \sin \omega_{m} t \tag{ii} \end{align*} $$

Thus, in amplitude modulation, amplitude A of modulated wave is made proportional to the instantaneous modulating voltage $e_{m}$

i.e., $\quad A=E_{c}+k e_{m}$

where, $k$ is a constant of proportionality.

In amplitude modulation, the proportionality constant $k$ is made equal to unity. Therefore, maximum positive amplitude of AM wave is given by

$$ \begin{equation*} A=E_{c}+e_{m}=E_{c}+E_{m} \sin \omega_{m} t \tag{iv} \end{equation*} $$

It is called top envelope.

The maximum negative amplitude of AM wave is given by

$$ \begin{align*} -A & =-E_{c}-e_{m} \ & =-\left(E_{c}+E_{m} \sin \omega_{m} t\right) \tag{v} \end{align*} $$

This is called bottom envelope.

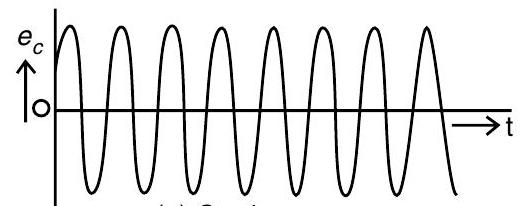
(a) Carrier wave

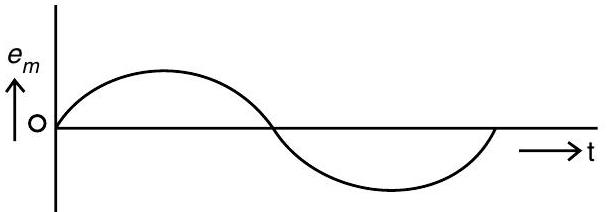
(b) Modulating Signal

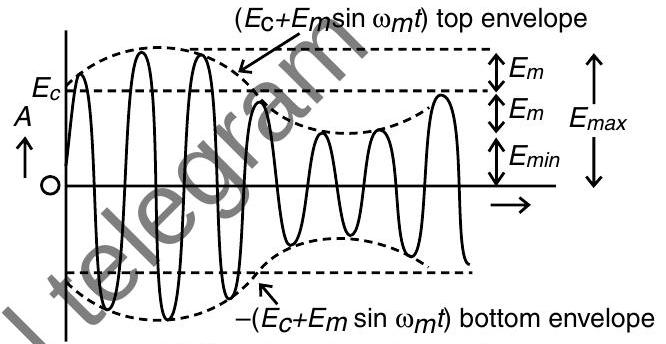
(c) Amplitude modulated wave

(a) Describe briefly three factors which justify the need for modulation of audio frequency signals over long distances in communication. (b) Draw the waveforms of (i) carrier wave, (ii) a modulating signal and (iii) amplitude modulated wave.

[CBSE Comptt- 2018]

Ans. (a) Describing the three factor 3

(b) Drawing the wave forms

2

(a) It is necessary to modulate the audio frequency signals because of the following three reasons :

(i) Size of the antenna or aerial $1 / 2$ This size needs to be comparable to the wavelength of the signal. it would be unmanageably large for audio frequency signals.

(ii) Effective power readiated

$1 / 2$

Power radiated, being proportional to $\left(\frac{l}{\lambda}\right)^{2}$ would be very small for a audio frequency signal.

(iii) Mixing up of different signals

The audible frequency range is quite small. Hence if transformation is done at audio frequencies, the chances of mixing up of different signals are very high.

$1 / 2$

(b) The required wave forms are as shown

(i) Carrier wave

(ii) Modulating Signal

(iii) Amplitude Modulated wave

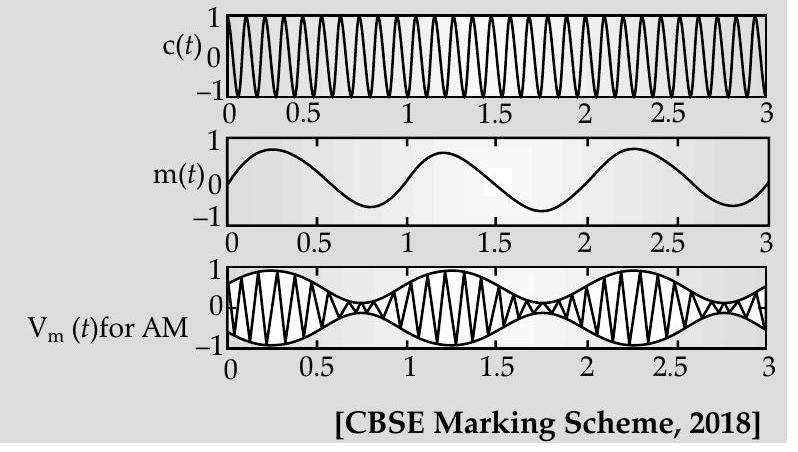
Detailed Answer :

(a) For a long distance Transmission of message signal we need modulation because of following reasons.

(i) Low energy: The message signals when converted to e.m. waves, do not have the sufficient energy to travel up to long distance, because of their low frequency. Hence these message signals are modulated with high frequency carrier signals before being send because carrier signals have high energy for long distance transmission.

(ii) Size of Antenna: For the effective Transmission by an antenna , the size of antenna should be at least of the size $\frac{\lambda}{4}$, where $\lambda$ is the wavelength of signal to be send. Thus for an em wave of audio signal of frequency is $20 \mathrm{kHz}$, we need an antenna of size nearly $3.75 \mathrm{~km}$, which is practicâlly impossible. Hence, these low frequency signals first modulated to high frequency signals before transmission to get the proper size of antenna. (iii) Mixing of signals : When number of signals are transmitted simultaneously, all these signals will get mixed up and at the end we get mixed signal, which is very difficult to separate. Therefore to remove this limitation, transmission is done at high frequency and a band of frequency is allotted to each user at the end, as done for radio and TV channels.