atoms Question 31
Question: Q. 1. (i) In Rutherford scattering experiment, draw the trajectory traced by $\alpha$-particles in the coulomb field of target nucleus and explain how this led to estimate the size of the nucleus.
(ii) Describe briefly how wave nature of moving electrons was established experimentally.
(iii) Estimate the ratio of de-Broglie wavelengths associated with deuterons and $\alpha$-particles when they are accelerated from rest through the same accelerating potential $V$.
Show Answer
Solution:
Ans. (i) The trajectory, traced by the $\alpha$-particles in the Coulomb field of target nucleus, has the form shown below.
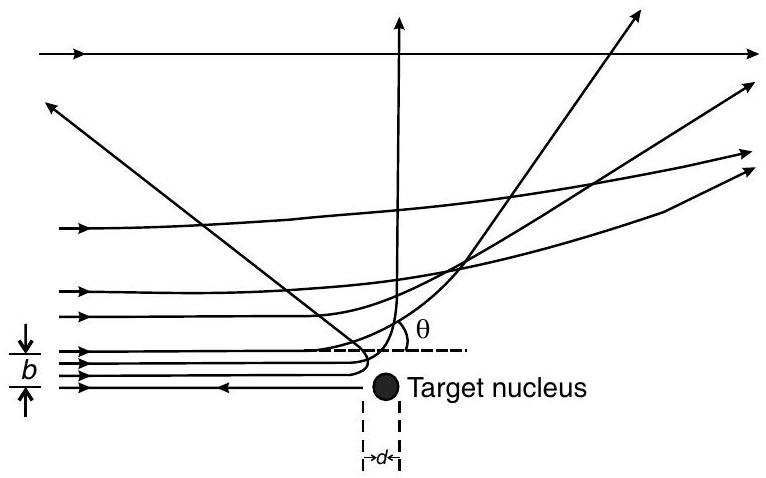
The size of the nucleus was estimated by observing the distance $(d)$ of closest approach, of the $\alpha$-particles. This distance is given by:
$$ \begin{equation*} d=\frac{1}{4 \pi \varepsilon_{0}} \cdot \frac{2 e Z e}{K} \tag{1} \end{equation*} $$
where, $K=$ kinetic energy of the $\alpha$-particles when they are far away from the target nuclei.
(ii) The wave nature of moving electrons was established through the Davisson-Germer experiment.
In this experiment, it was observed that a beam of electrons, when scattered by a nickel target, showed ‘maxima’ in certain directions; (like the ‘maxima observed in interference/diffraction experinents with light.)
$$ \begin{align*} \lambda & =\frac{h}{p} \tag{iii}\ \lambda & =\frac{h}{m v} \ \lambda & =\frac{h}{\sqrt{2 m q V}} \ \frac{\lambda_{d}}{\lambda_{\alpha}} & =\sqrt{\frac{m_{a} q_{\alpha}}{m_{d} q_{d}}} \end{align*} $$
$$ \frac{\lambda_{d}}{\lambda_{\alpha}}=\sqrt{\frac{4 \times 2}{2 \times 1}}=2 $$
