Polynomials Question 30
Question: Q. 3. Basketball and soccer are played with a spherical ball. Even though an athlete dribbles the ball in both sports, a basketball player uses his hands and a soccer player uses his feet. Usually, soccer is played outdoors on a large field and basketball is played indoor on a court made out of wood. The projectile (path traced) of soccer ball and basketball are in the form of parabola representing quadratic polynomial.
[CBSE QB, 2021]
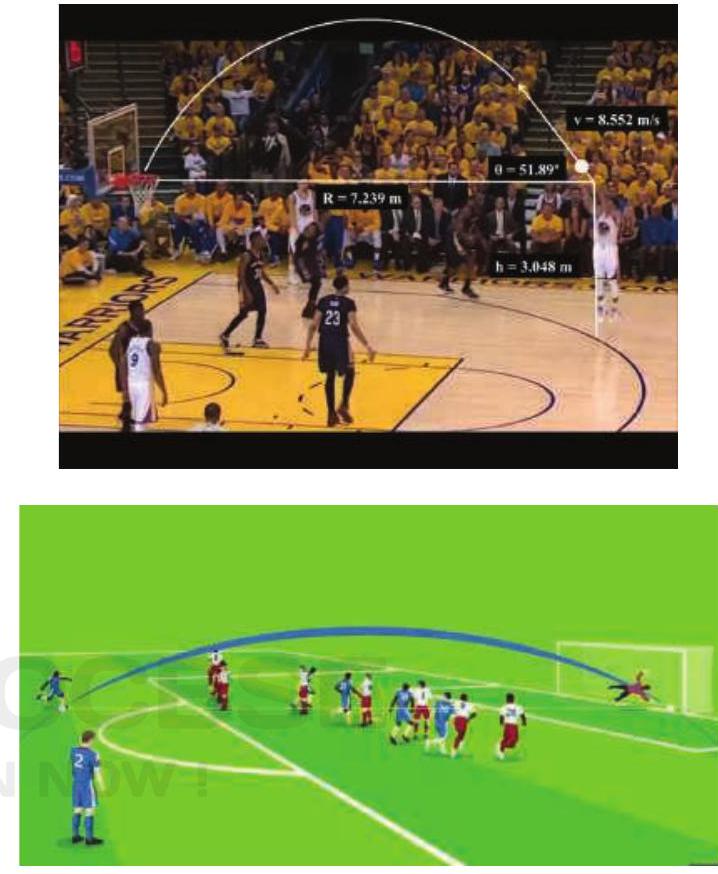
(i) The shape of the path traced shown is (a) Spiral (b) Ellipse (c) Linear (d) Parabola
Show Answer
Solution:
Correct option: (d).
(ii) The graph of parabola opens upwards, if
(a)
(b)
(c)
(d)
Sol. Correct option: (c).
(iii) Observe the following graph and answer
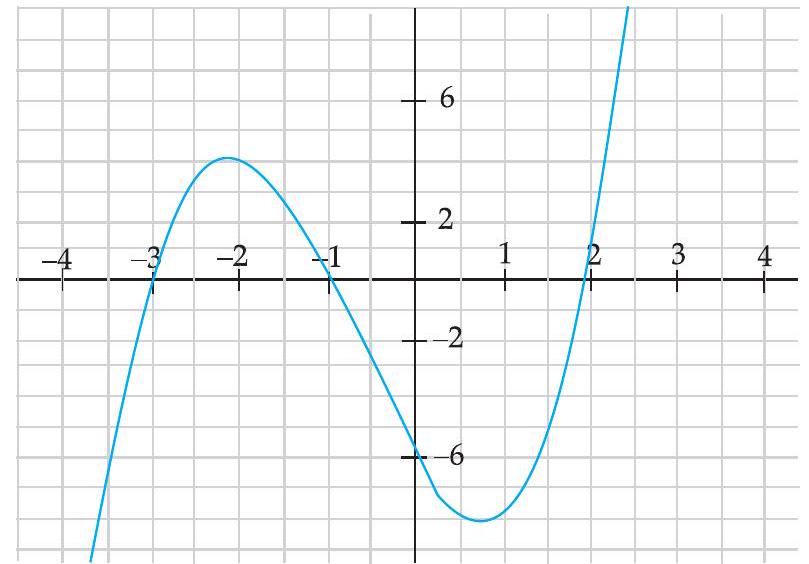
In the above graph, how many zeroes are there for the polynomial?
(a) 0
(b) 1
(c) 2
(d) 3
Sol. Correct option: (d).
Explanation: The number of zeroes of polynomial is the number of times the curve intersects the
Here, the polynomial meets the
(iv) The three zeroes in the above shown graph are
(a)
(b)
(c)
(d)
Sol. Correct option: (c).
(v) What will be the expression of the polynomial?
(a)
(b)
(c)
(d)
Sol. Correct option: (a).
Explanation: Since, the three zeroes
Hence, the expression is
$$ \begin{aligned} & =\leftx^{2}+x+3 x+3\right \ & =x^{3}+4 x^{2}+3 x-2 x^{2}-8 x-6 \ & =x^{3}+2 x^{2}-5 x-6 \end{aligned} $$
[II Q. 4. Applications of Parabolas: Highway Overpasses/ Underpasses
A highway underpass is parabolic in shape.

Shape of Cross Slope :
a. Paraboilc camber
Parabola
A parabola is the graph that results from
Parabolas are symmetric about a vertical line known as the Axis of Symmetry.
The Axis of Symmetry runs through the maximum or minimum point of the parabola which is called the vertex.
C + AE [CBSE SQP, 2020-21]
(i) If the highway overpass is represented by
(a)
(b)
(c)
(d)
Sol. Correct option:
(b)
[CBSE Marking Scheme, 2020]
Detailed Solution:
(ii) The highway overpass is represented graphically. Zeroes of a polynomial can be expressed graphically. Number of zeroes of polynomial is equal to number of points where the graph of polynomial:
(a) Intersects
(b) Intersects Y-axis
(c) Intersects
(d) None of the above
Sol. Correct option: (a)
Explanation: We know that the number of zeroes of polynomial is equal to number of points where the graph of polynomial intersects X-axis.
(iii) Graph of a quadratic polynomial is a:
(a) straight line
(b) circle
(c) parabola
(d) ellipse
Sol. Correct option: (c)
Explanation: Here, the given graph of a quadratic polynomial is a parabola.
1
(iv) The representation of Highway Underpass whose one zero is 6 and sum of the zeroes is 0 , is:
(a)
(b)
(c)
(d)
Sol. Correct option: (b)
[CBSE Marking Scheme, 2020]
Detailed Solution:
(v) The number of zeroes that polynomial
(a) 1
(b) 2
(c) 0
(d) 3
Sol. Correct option: (c)
Explanation: We have,
i.e., It has no factorisation.
Hence no real value of










