Thermodynamics and Thermochemistry 1 Question 16
16. An ideal gas undergoes isothermal compression from $5 \mathrm{~m}^{3}$ to $1 \mathrm{~m}^{3}$ against a constant external pressure of $4 \mathrm{Nm}^{-2}$. Heat released in this process is used to increase the temperature of 1 mole of Al. If molar heat capacity of $\mathrm{Al}$ is $24 \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1}$, the temperature of $\mathrm{Al}$ increases by
(2019 Main, 10 Jan II)
(a) $\frac{3}{2} \mathrm{~K}$
(b) $1 \mathrm{~K}$
(c) $2 \mathrm{~K}$
(d) $\frac{2}{3} \mathrm{~K}$
17 A process has $\Delta H=200 \mathrm{~J} \mathrm{~mol}^{-1}$ and $\Delta S=40 \mathrm{JK}^{-1} \mathrm{~mol}^{-1}$. Out of the values given below, choose the minimum temperature above which the process will be spontaneous
(2019 Main, 10 Jan I)
(a) $20 \mathrm{~K}$
(b) $4 \mathrm{~K}$
(c) $5 \mathrm{~K}$
(d) $12 \mathrm{~K}$
18 The entropy change associated with the conversion of $1 \mathrm{~kg}$ of ice at $273 \mathrm{~K}$ to water vapours at $383 \mathrm{~K}$ is
(Specific heat of water liquid and water vapour are $4.2 \mathrm{~kJ}$ $\mathrm{K}^{-1} \mathrm{~kg}^{-1}$ and $2.0 \mathrm{kJK}^{-1} \mathrm{~kg}^{-1}$; heat of liquid fusion and vapourisation of water are $334 \mathrm{~kJ} \mathrm{~kg}^{-1}$ and $2491 \mathrm{kJkg}^{-1}$ respectively $). \quad(\log 273=2.436, \quad \log 373=2.572$, $\log 383=2.583)$
(2019 Main, 9 Jan II)
(a) $9.26 \mathrm{~kJ} \mathrm{~kg}^{-1} \mathrm{~K}^{-1}$
(b) $8.49 \mathrm{~kJ} \mathrm{~kg}^{-1} \mathrm{~K}^{-1}$
(c) $7.90 \mathrm{~kJ} \mathrm{~kg}^{-1} \mathrm{~K}^{-1}$
(d) $2.64 \mathrm{~kJ} \mathrm{~kg}^{-1} \mathrm{~K}^{-1}$
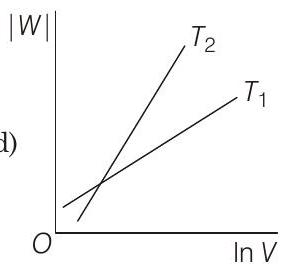
19 Consider the reversible isothermal expansion of an ideal gas in a closed system at two different temperatures $T_{1}$ and $T_{2}$ $\left(T_{1}<T_{2}\right)$. The correct graphical depiction of the dependence of work done $(W)$ on the final volume $(V)$ is
(2019 Main, 9 Jan I)
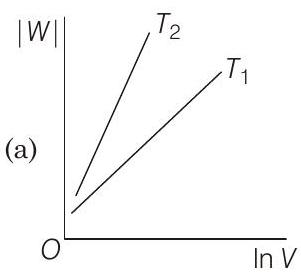
(b)
(d)
Show Answer
Solution:
- It is an irreversible isothermal compression of an ideal gas.
(i) $d E=d q+p\left(V_{f}-V_{i}\right)$
where, $d E=$ Internal energy change
$$ \begin{aligned} d q & =\text { amount of heat released } \ \Rightarrow \quad 0 & =d q+p\left(V_{f}-V_{i}\right) \end{aligned} $$
$[\because d E=0$ for an isothermal process $]$
$\Rightarrow d q=-4(1-5)=16 \mathrm{~J}$
(ii) $\quad d q=n \times C \times \Delta T$ (for $\mathrm{Al}$ )
$\Rightarrow 16 \mathrm{~J}=1 \mathrm{~mol} \times 24 \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1} \times \Delta T$
$\Rightarrow \Delta T=\frac{16}{24} \mathrm{~K}=\frac{2}{3} \mathrm{~K}$






