Chemical and Ionic Equilibrium 1 Question 27
28. For a reaction, $A \rightleftharpoons P$, the plots of $[A]$ and $[P]$ with time at temperatures $T_{1}$ and $T_{2}$ are given below.
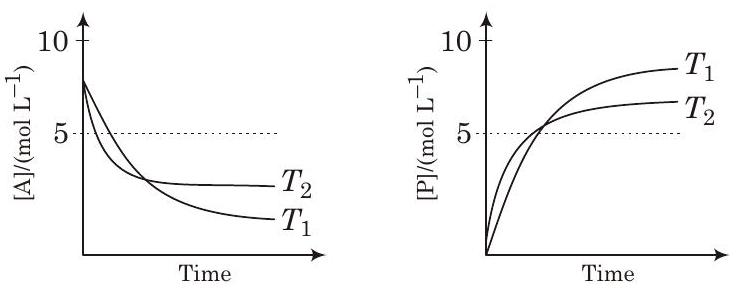
If $T_{2}>T_{1}$, the correct statement(s) is are
(Assume $\Delta H^{\ominus}$ and $\Delta S^{\ominus}$ are independent of temperature and ratio of $\ln K$ at $T_{1}$ to $\ln K$ at $T_{2}$ is greater than $T_{2} / T_{1}$. Here $H, S, G$ and $K$ are enthalpy, entropy, Gibbs energy and equilibrium constant, respectively.)
(2018 Adv.)
(a) $\Delta H^{\ominus}<0, \Delta S^{\ominus}<0$
(b) $\Delta G^{\ominus}<0, \Delta H^{\ominus}>0$
(c) $\Delta G^{\ominus}<0, \Delta S^{\ominus}<0$
(d) $\Delta G^{\ominus}<0, \Delta S^{\ominus}>0$
Show Answer
Solution:
- For the reaction, $A \rightleftharpoons P$
Given,
$$ \begin{array}{r} T_{1}<T_{2} \ \frac{\ln K_{1}}{\ln K_{2}}>\frac{T_{2}}{T_{1}} \end{array} $$
It shows, On increasing the temperature, $K$ decreases so reaction is exothermic i.e., $\Delta H^{\circ}<0$
Besides, graph shows $K>1$
So
$$ \Delta G^{\mathrm{o}}<0 $$
Now from equation (i)
$$ \begin{array}{ll} & T_{1} \ln K_{1}>\mathrm{T}{2} \ln K{2} \ & -\Delta G^{\mathrm{o}}>-\Delta G^{\mathrm{o}} \ \text { Likewise } & \left(-\Delta H^{\mathrm{o}}+T_{1} \Delta S^{\mathrm{o}}\right)>\left(-\Delta H^{\mathrm{o}}+T_{2} \Delta S^{\mathrm{o}}\right) \ \text { or simply } & T_{1} \Delta S^{\mathrm{o}}>T_{2} \Delta S^{\mathrm{o}} \ \text { So, } & \left(T_{2}-T_{1}\right) \Delta S^{\circ}<0 \ \therefore & \Delta S^{\mathrm{o}}<0 \end{array} $$
In other words, increase of $\Delta G$ with increase in temperature is possible only when $\Delta S^{\circ}<0$. Hence, options (a) and (c) are correct.






