Chapter7 System Of Particles And Rotational Motion
Chapter 7
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
MCQ I
7.1 For which of the following does the centre of mass lie outside the body?
(a) A pencil
(b) A shotput
(c) A dice
(d) A bangle
7.2 Which of the following points is the likely position of the centre of mass of the system shown in Fig. 7.1?
(a) $\mathrm{A}$
(b) $\mathrm{B}$
(c) $\mathrm{C}$
(d) $\mathrm{D}$
7.3 A particle of mass $m$ is moving in $y z$-plane with a uniform velocity $v$ with its trajectory running parallel to +ve $y$-axis and intersecting
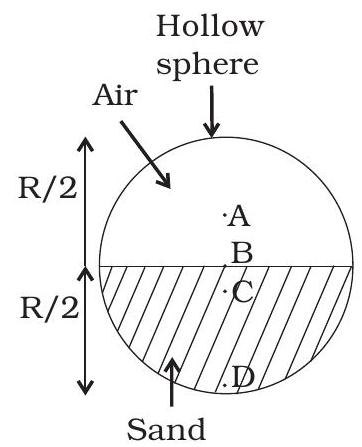
Fig. 7.1
$z$-axis at $z=a$ (Fig. 7.2). The change in its angular momentum about the origin as it bounces elastically from a wall at $y=$ constant is:
(a) $m v a \hat{\mathbf{e}}_{x}$
(b) $2 m v a \hat{\mathbf{e}}_{x}$
(c) $y m v \hat{\mathbf{e}}_{x}$
(d) $2 y m v \hat{\mathbf{e}}_{x}$
7.4 When a disc rotates with uniform angular velocity, which of the
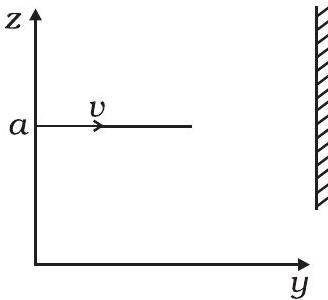
Fig. 7.2 following is not true?
(a) The sense of rotation remains same.
(b) The orientation of the axis of rotation remains same.
(c) The speed of rotation is non-zero and remains same.
(d) The angular acceleration is non-zero and remains same.
7.5 A uniform square plate has a small piece $Q$ of an irregular shape removed and glued to the centre of the plate leaving a hole behind (Fig. 7.3). The moment of inertia about the $\mathrm{z}$-axis is then

Fig. 7.3
(a) increased
(b) decreased
(c) the same
(d) changed in unpredicted manner.
7.6 In problem 7.5, the $\mathrm{CM}$ of the plate is now in the following quadrant of $x-y$ plane,
(a) I
(b) II
(c) III
(d) IV
7.7 The density of a non-uniform rod of length $1 \mathrm{~m}$ is given by $\rho(x)=a\left(1+b x^{2}\right)$
where $\mathrm{a}$ and $\mathrm{b}$ are constants and $o \leq x \leq 1$.
The centre of mass of the rod will be at
(a) $\frac{3(2+b)}{4(3+b)}$
(b) $\frac{4(2+b)}{3(3+b)}$
(c) $\frac{3(3+b)}{4(2+b)}$
(d) $\frac{4(3+b)}{3(2+b)}$
7.8 A Merry-go-round, made of a ring-like platform of radius $\mathrm{R}$ and mass $\mathrm{M}$, is revolving with angular speed $\omega$. A person of mass M is standing on it. At one instant, the person jumps off the round, radially away from the centre of the round (as seen from the round). The speed of the round afterwards is
(a) $2 \omega$
(b) $\omega$
(c) $\frac{\omega}{2}$
(d) 0
MCQ II
7.9 Choose the correct alternatives:
(a) For a general rotational motion, angular momentum $\mathbf{L}$ and angular velocity $\boldsymbol{\omega}$ need not be parallel.
(b) For a rotational motion about a fixed axis, angular momentum $\mathbf{L}$ and angular velocity $\boldsymbol{\omega}$ are always parallel.
(c) For a general translational motion, momentum $\mathbf{p}$ and velocity $\mathbf{v}$ are always parallel.
(d) For a general translational motion, acceleration a and velocity $\mathbf{v}$ are always parallel.
7.10 Figure 7.4 shows two identical particles 1 and 2, each of mass $m$, moving in opposite directions with same speed $\mathbf{v}$ along parallel lines. At a particular instant, $\mathbf{r_1}$ and $\mathbf{r_2}$ are their respective position vectors drawn from point $A$ which is in the plane of the parallel lines . Choose the correct options:
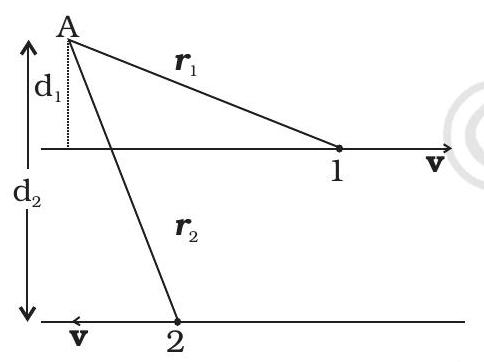
Fig. 7.4
(a) Angular momentum $\boldsymbol{l_1}$ of particle 1 about $\mathrm{A}$ is $\boldsymbol{l_1}=\operatorname{mvd}_{1}$
(b) Angular momentum $\boldsymbol{l_2}$ of particle 2 about $\mathrm{A}$ is $\boldsymbol{l_2}=\mathbf{m v r}_{2}$
(c) Total angular momentum of the system about A is $\boldsymbol{l}=m v\left(\boldsymbol{r_1}+\boldsymbol{r_2}\right)$
(d) Total angular momentum of the system about A is $\boldsymbol{l}=m v\left(d_{2}-d_{1}\right) \otimes$
represents a unit vector coming out of the page.
$\otimes$ represents a unit vector going into the page.
7.11 The net external torque on a system of particles about an axis is zero. Which of the following are compatible with it?
(a) The forces may be acting radially from a point on the axis.
(b) The forces may be acting on the axis of rotation.
(c) The forces may be acting parallel to the axis of rotation.
(d) The torque caused by some forces may be equal and opposite to that caused by other forces.
System of Particles and Rotational Motion
7.12 Figure 7.5 shows a lamina in $x-y$ plane. Two axes $z$ and $z^{\prime}$ pass perpendicular to its plane. A force $\mathbf{F}$ acts in the plane of lamina at point $\mathrm{P}$ as shown. Which of the following are true? (The point $\mathrm{P}$ is closer to $Z^{\prime}$-axis than the $\mathrm{z}$-axis.)
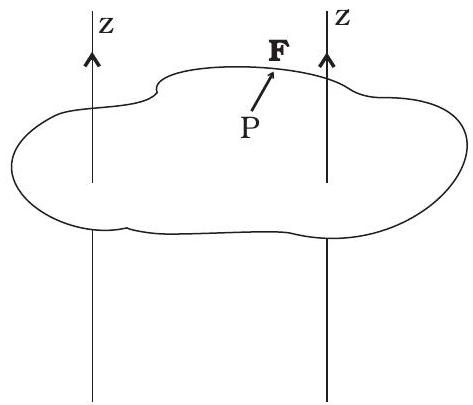
Fig. 7.5
(a) Torque $\tau$ caused by $\mathbf{F}$ about $z$ axis is along $-\hat{\mathbf{k}}$.
(b) Torque $\tau^{\prime}$ caused by $\mathbf{F}$ about $z^{\prime}$ axis is along $-\hat{\mathbf{k}}$.
(c) Torque $\tau$ caused by $\mathbf{F}$ about $z$ axis is greater in magnitude than that about $\mathrm{z}$ axis.
(d) Total torque is given be $\tau=\tau+\tau^{\prime}$.
7.13 With reference to Fig. 7.6 of a cube of edge $a$ and mass $m$, state whether the following are true or false. ( $\mathrm{O}$ is the centre of the cube.)

Fig. 7.6
(a) The moment of inertia of cube about $z$-axis is $I_{z}=I_{x}+I_{y}$
(b) The moment of inertia of cube about $z^{\prime}$ is
$$ I_{z}^{\prime}=I_{z}+\frac{m a^{2}}{2} $$
(c) The moment of inertia of cube about $z^{\prime \prime}$ is
$$ =I_{z}+\frac{m a^{2}}{2} $$
(d) $I_{x}=I_{y}$
VSA
7.14 The centre of gravity of a body on the earth coincides with its centre of mass for a ‘small’ object whereas for an ’extended’ object it may not. What is the qualitative meaning of ‘small’ and ’extended’ in this regard?
For which of the following the two coincides? A building, a pond, a lake, a mountain?
7.15 Why does a solid sphere have smaller moment of inertia than a hollow cylinder of same mass and radius, about an axis passing through their axes of symmetry?
7.16 The variation of angular position $\theta$, of a point on a rotating rigid body, with time $t$ is shown in Fig. 7.7. Is the body rotating clock-wise or anti-clockwise?

Fig. 7.7
7.17 A uniform cube of mass $m$ and side $a$ is placed on a frictionless horizontal surface. A vertical force $\boldsymbol{F}$ is applied to the edge as shown in Fig. 7.8.

Fig. 7.8
Match the following (most appropriate choice):
| Column I | Column II |
|---|---|
| (a) $m g / 4<F<m g / 2$ | (i) Cube will move up. |
| (b) $F>m g / 2$ | (ii) Cube will not exhibit motion. |
| (c) $F>m g$ | (iii) Cube will begin to rotate and slip at A. |
| (d) $F=m g / 4$ | (iv) Normal reaction effectively at $a / 3$ from $A$, no motion. |
7.18 A uniform sphere of mass $m$ and radius $R$ is placed on a rough horizontal surface (Fig. 7.9). The sphere is struck horizontally at a height $h$ from the floor. Match the following:

Fig. 7.9
| Column I | Column II |
|---|---|
| (a) $h=R / 2$ | (i) Sphere rolls without slipping with a constant velocity and no loss of energy. |
| (b) $h=R$ | (ii) Sphere spins clockwise, loses energy by friction. |
| (c) $h=3 R / 2$ | (iii) Sphere spins anti-clockwise, loses energy by friction. |
| (d) $h=7 \mathrm{R} / 5$ | (iv) Sphere has only a translational motion, looses energy by friction. |
7.19 The vector sum of a system of non-collinear forces acting on a rigid body is given to be non-zero. If the vector sum of all the torques due to the system of forces about a certain point is found to be zero, does this mean that it is necessarily zero about any arbitrary point?
7.20 A wheel in uniform motion about an axis passing through its centre and perpendicular to its plane is considered to be in mechanical (translational plus rotational) equilibrium because no net external force or torque is required to sustain its motion. However, the particles that constitute the wheel do experience a centripetal acceleration directed towards the centre. How do you reconcile this fact with the wheel being in equilibrium?
How would you set a half-wheel into uniform motion about an axis passing through the centre of mass of the wheel and perpendicular to its plane? Will you require external forces to sustain the motion?
7.21 A door is hinged at one end and is free to rotate about a vertical axis (Fig. 7.10). Does its weight cause any torque about this axis? Give reason for your answer.
7.22 (n-1)$ equal point masses each of mass $m$ are placed at the vertices of a regular n-polygon. The vacant vertex has a position vector a with respect to the centre of the polygon. Find the position vector of centre of mass.
LA
7.23 Find the centre of mass of a uniform (a) half-disc, (b) quarter-disc.
7.24 Two discs of moments of inertia $I_{1}$ and $I_{2}$ about their respective axes (normal to the disc and passing through the centre), and rotating with angular speed $\omega_{1}$ and $\omega_{2}$ are brought into contact face to face with their axes of rotation coincident.
(a) Does the law of conservation of angular momentum apply to the situation? why?
(b) Find the angular speed of the two-disc system.
(c) Calculate the loss in kinetic energy of the system in the process.
(d) Account for this loss.
7.25 A disc of radius $R$ is rotating with an angular speed $\omega_{o}$ about a horizontal axis. It is placed on a horizontal table. The coefficient of kinetic friction is $\mu_{k}$.
(a) What was the velocity of its centre of mass before being brought in contact with the table?
(b) What happens to the linear velocity of a point on its rim when placed in contact with the table?
(c) What happens to the linear speed of the centre of mass when disc is placed in contact with the table?
(d) Which force is responsible for the effects in (b) and (c).
(e) What condition should be satisfied for rolling to begin?
(f) Calculate the time taken for the rolling to begin.
7.26 Two cylindrical hollow drums of radii $R$ and $2 R$, and of a common height $h$, are rotating with angular velocities $\omega$ (anti-clockwise) and $\omega$ (clockwise), respectively. Their axes, fixed are parallel and in a horizontal plane separated by $(3 R+\delta)$. They are now brought in contact $(\delta \rightarrow 0)$.
(a) Show the frictional forces just after contact.
(b) Identify forces and torques external to the system just after contact.
(c) What would be the ratio of final angular velocities when friction ceases?
Exemplar Problems-Physics
7.27 A uniform square plate $\mathrm{S}$ (side $\mathrm{c}$ ) and a uniform rectangular plate $\mathrm{R}$ (sides b, a) have identical areas and masses (Fig. 7.11).
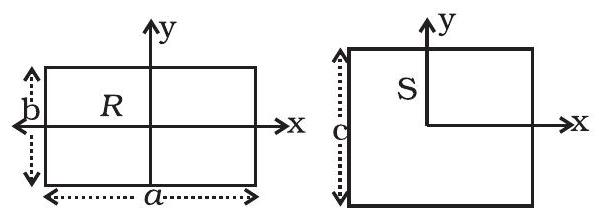
Fig. 7.11
Show that
(i) $I_{x R} / I_{x S}<1$; (ii) $I_{y R} / I_{y S}>1$; (iii) $I_{z R} / I_{z S}>1$.
7.28 A uniform disc of radius $R$, is resting on a table on its rim. The coefficient of friction between disc and table is $\mu$ (Fig 7.12). Now the disc is pulled with a force $\mathbf{F}$ as shown in the figure. What is the maximum value of $\mathbf{F}$ for which the disc rolls without slipping?
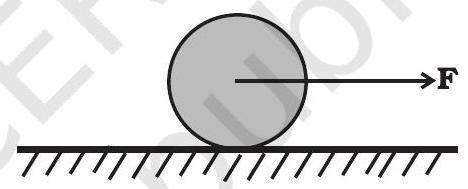
Fig. 7.12
Solutions 7
7.1 (d)
7.2 (c)
7.3 The initial velocity is $v_i=v \hat{e_y}$ and, after reflection from the wall, the final velocity is $v_f=-v \hat{e_y}$. The trajectory is described as $r=y \hat{e_y}+a \hat{e_Z}$. Hence the change in angular momentum is $r \times m\left(v_f-v_i\right)=2 m v a \hat{e_x}$. Hence the answer is (b).
7.4 (d)
7.5 (b)
7.6 (c)
7.7 When $\mathrm{b} \rightarrow 0$, the density becomes uniform and hence the centre of mass is at $x=0.5$. Only option (a) tends to 0.5 as $\mathrm{b} \rightarrow 0$.
7.8 (b) $\omega$
7.9 (a), (c)
7.10 (a), (d)
7.11 All are true.
7.12 (a) False, it is along $\hat{\mathbf{k}}$.
(b) True
(c) True
(d) False, there is no sense in adding torques about 2 different axes.
7.13 (a) False, perpendicular axis theorem is applicable only to a lamina.
(b) True
(c) False, $z$ and $z$ " are not parallel axes.
(d) True.
7.14 When the vertical height of the object is very small as compared to earth’s radius, we call the object small, otherwise it is extended.
(a) Building and pond are small objects.
(b) A deep lake and a mountain are examples of extended objects.
7.15 $I=\sum m_{i} r_{i}^{2}$. All the mass in a cylinder lies at distance $R$ from the axis of symmetry but most of the mass of a solid sphere lies at a smaller distance than $R$.
7.16 Positive slope indicates anticlockwise rotation which is traditionally taken as positive.
7.17 (a) ii, (b) iii, (c) i, (d) iv
7.18 (a) iii, (b) iv (c) ii (d) i.
7.19 No. Given $\sum_{i} \mathbf{F}_{i} \neq 0$
The sum of torques about a certain point ’ 0
$\sum_i \mathbf{r_i} \times \mathbf{F_i}=0$
The sum of torques about any other point $\mathrm{O}^{\prime}$,
$\sum_i\left(\mathbf{r_i}-\mathbf{a}\right) \times \mathbf{F_i}=\sum_i \mathbf{r_i} \times \mathbf{F_i}-\mathbf{a} \times \sum_i F_i$
Here, the second term need not vanish.
7.20 The centripetal acceleration in a wheel arise due to the internal elastic forces which in pairs cancel each other; being part of a symmetrical system.
In a half wheel the distribution of mass about its centre of mass (axis of rotation) is not symmetrical. Therefore, the direction of angular momentum does not coincide with the direction of angular velocity and hence an external torque is required to maintain rotation.
7.21 No. A force can produce torque only along a direction normal to itself as $\boldsymbol{\tau}=\mathbf{r} \times \mathbf{f}$. So, when the door is in the $x y$-plane, the torque produced by gravity can only be along $\pm \boldsymbol{Z}$ direction, never about an axis passing through $y$ direction.
7.22 Let the C.M. be ‘b’. Then, $\frac{(n-1) m b+m a}{m n}=0 \Rightarrow b=-\frac{1}{n-1} a$
7.23 (a) Surface density $\sigma=\frac{2 M}{\pi a^{2}}$
$$ \bar{x}=\frac{\int x d m}{\int d m}=\int_{r=0}^{a} \int_{\theta=0}^{\pi} r \cos \theta \sigma r d r d \theta=\int_{r=0}^{a} \int_{\theta=0}^{\pi} \sigma r d r d \theta $$
$$ =\frac{\int_0^a r^2 d r \sin \theta|x_0}{\int_0^a r d r \int_0^{\pi} d \theta}=0 $$
$\bar{y}=\frac{\int y d m}{\int d m}=\int_{\theta=0}^{\pi} \int_{r=0}^{a} r \sin \theta \sigma r d r d \theta \int_{r=0}^{a} \int_{\theta=0}^{\pi} \sigma r d r d \theta$
$=\frac{\int_{0}^{a} r^{2} d r \int_{\theta=0}^{\pi} \sin \theta d \theta}{\int_{0}^{a} r d r \int_{0}^{\pi} d \theta}=\frac{a^{3}}{3} \frac{[-\cos \theta]_{0}^{\pi}}{\left(a^{2} / 2\right) \pi}=\frac{a}{3} \frac{4}{\pi}=\frac{4 a}{3 \pi}$.
(b) Same procedure, as in (a) except $\theta$ goes from 0 to $\pi / 2$ and
$$ \sigma=\frac{4 M}{\pi a^{2}} $$
7.24 (a) Yes, because there is no net external torque on the system. External forces, gravitation and normal reaction, act through the axis of rotation, hence produce no torque.
(b) By angular momentum conservation
$$ \begin{aligned} & I \omega=I_{1} \omega_{1}+I_{2} \omega_{2} \ & \therefore \omega=\frac{I_{1} \omega_{1}+I_{2} \omega_{2}}{I_{1}+I_{2}} \end{aligned} $$
(c) $K_{f}=\frac{1}{2}\left(I_{1}+I_{2}\right) \frac{\left(I_{1} \omega_{1}+I_{2} \omega_{2}\right)^{2}}{\left(I_{1}+I_{2}\right)^{2}}=\frac{1}{2} \frac{\left(I_{1} \omega_{1}+I_{2} \omega_{2}\right)^{2}}{I_{1}+I_{2}}$
$$ \begin{aligned} & K_{i}=\frac{1}{2}\left(I_{1} \omega_{1}^{2}+I_{2} \omega_{2}^{2}\right) \ & \Delta K=K_{f}-K_{i}=-\frac{I_{1} I_{2}}{2\left(I_{1}+I_{2}\right)}\left(\omega_{1}-\omega_{2}\right)^{2} \end{aligned} $$
(d) The loss in kinetic energy is due to the work against the friction between the two discs.
7.25 (a) Zero (b) Decreases (c) Increases (d) Friction (e) $v_{c m}=R \omega$.
(f) Acceleration produced in centre of mass due to friction:
$$ a_{c m}=\frac{F}{m}=\frac{\mu_{k} m g}{m}=\mu_{k} g $$
Angular acceleration produced by the torque due to friction,
$$ \begin{aligned} & \alpha=\frac{\tau}{I}=\frac{\mu_{k} m g R}{I} \ & \therefore v_{c m}=u_{c m}+a_{c m} t \Rightarrow v_{c m}=\mu_{k} g t \ & \text { and } \omega=\omega_{o}+\alpha t \Rightarrow \omega=\omega_{o}-\frac{\mu_{k} m g R}{I} t \end{aligned} $$
For rolling without slipping,
$$ \begin{aligned} & \frac{v_{c m}}{R}=\omega_{o}-\frac{\mu_{K} m g R}{I} t \ & \frac{\mu_{K} g t}{R}=\omega_{O}-\frac{\mu_{K} m g R}{I} t \end{aligned} $$
$$ t=\frac{R \omega_{o}}{\mu_{k} g\left(1+\frac{m R^{2}}{I}\right)} $$
7.26
(a)

$\mathbf{F} \uparrow$ force on left drum (upward)
$\mathbf{F} \downarrow$ force on right drum (downward) (b) $\quad F^{\prime}=F=F^{\prime \prime}$ where $F$ and $F^{\prime \prime}$ and external forces through support. $\mathrm{F}_{\text {net }}=0$
External torque $=F \times 3 R$, anticlockwise.
(c) Let $\omega_{1}$ and $\omega_{2}$ be final angular velocities (anticlockwise and clockwise respectively)

Finally there will be no friction.
Hence, $R \omega_{1}=2 R \omega_{2} \Rightarrow \frac{\omega_{1}}{\omega_{2}}=2$
7.27 (i) Area of square $=$ area of rectangle $\Rightarrow c^{2}=a b$
$$ \frac{I_{x R}}{I_{x S}} \times \frac{I_{y R}}{I_{y S}}=\frac{b^{2}}{c^{2}} \times \frac{a^{2}}{c^{2}}=\left(\frac{a b}{c^{2}}\right)^{2}=1 $$
(i) and (ii) $\quad \frac{I_{y R}}{I_{y S}}>\frac{I_{x R}}{I_{x S}} \Rightarrow \frac{I_{y R}}{I_{y S}}>1$ and $\frac{I_{x R}}{I_{x S}}<1$.
(iii)
$$ \begin{aligned} & I_{z r}-I_{Z S} \propto\left(a^{2}+b^{2}-2 c^{2}\right) \ & =a^{2}+b^{2}-2 a b>0 \ \therefore & \left(I_{z R}-I_{z S}\right)>0 \ \therefore & \frac{I_{z R}}{I_{z S}}>1 . \end{aligned} $$
7.28 Let the accelaration of the centre of mass of disc be ’ $a$ ‘, then
$M a=F-f$
The angular accelaration of the disc is $\alpha=a / R$. (if there is no sliding).
Then
$$ \begin{align*} & \left(\frac{1}{2} M R^{2}\right) \alpha=R f \tag{2}\ & \Rightarrow M a=2 f \end{align*} $$
Thus, $f=F / 3$. Since there is no sliding,

$\Rightarrow f \leq \mu m g$
$\Rightarrow F \leq 3 \mu M g$.






