Straight Line -

It is a curve such that every point on the line segment joining any two points on it lies on it. Equation of straight line is always of first degree on $\mathrm{x}$ and $\mathrm{y}$.

General equation of straight line is $\mathrm{ax}+\mathrm{by}+\mathrm{c}=0$

Where a,b,c are any real numbers not all zero.

Slope of a line

The trigonometrical tangent of an angle that a line makes with the positive direction of the $\mathrm{x}$ axis in anticlockwise direction is called the slope or gradient of the line Normally slope is denoted by $\mathrm{m}=\tan \theta$.

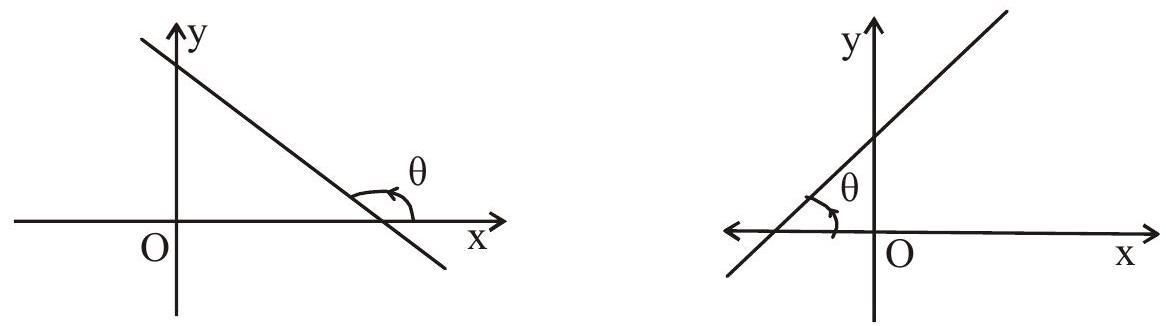
Points to remember

(i) Slope of a line parallel to $\mathrm{x}$-axis is zero and perpendicular to $\mathrm{x}$-axis is undefined.

(ii) If slope is positive then it makes acute angle with positive direction of $\mathrm{x}$-axis and if it is negative, then it will make obtuse angle with positive direction of $x$-axis.

(iii) If a line is equally inclined to the axes, that it will make angle of ${45}^{\circ }$ or ${135}^{\circ }$, with $x$ axis. Hence slope of line is $\pm 1$.

(iv) It three points $\mathrm{A},\mathrm{B},\mathrm{C}$ are collinear then slope of $\mathrm{AB}=$ slope of $\mathrm{BC}=$ slope of $\mathrm{AC}$

(v) Slope of the line joining two points $(x_1,y_1)$ and $(x_2,y_2)$ is $m={\displaystyle \frac{y_1-y_2}{x_1-x_2}}$ or ${\displaystyle \frac{y_2-y_1}{x_2-x_1}}$

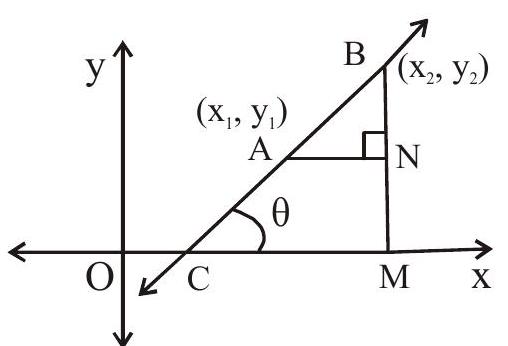
(vi) Equation of $x$-axis is $y=0$

(vii) Equation of $\mathrm{y}$-axis is $\mathrm{x}=0$

(viii) Equation of line parallel to $\mathrm{x}$-axis is $\mathrm{y}=\pm \mathrm{a}$, where $\mathrm{a}$ is any real number

(ix) Equation of line parallel to $\mathrm{y}$-axis (perpendicular to $\mathrm{x}$-axis) is $\mathrm{x}=\pm \mathrm{a}$, where $\mathrm{a}$ is any real number

Angle between two lines

The angle $\theta$ between the lines having slopes ${\mathrm{m}}_1$ and ${\mathrm{m}}_2$ is given by

$\tan \theta =\left|{\displaystyle \frac{{\mathrm{m}}_1-{\mathrm{m}}_2}{1+{\mathrm{m}}_1{\mathrm{m}}_2}}\right|=\pm \left({\displaystyle \frac{{\mathrm{m}}_1-{\mathrm{m}}_2}{1+{\mathrm{m}}_1{\mathrm{m}}_2}}\right)$

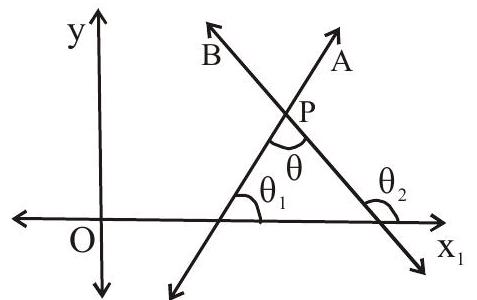
Where ${\mathbf{m}}_1=\mathbf{t}\mathbf{a}\mathbf{n}{\theta }_1,{\mathbf{m}}_2=\mathbf{t}\mathbf{a}\mathbf{n}{\theta }_2$

(i) When two lines are parallel then their slopes are equal ie. $m_1=m_2$

(ii) When two lines are perpendicular then the product of their slope is -1

i.e. ${\mathrm{m}}_1{\mathrm{m}}_2=-1$ or $1+{\mathrm{m}}_1{\mathrm{m}}_2=0$

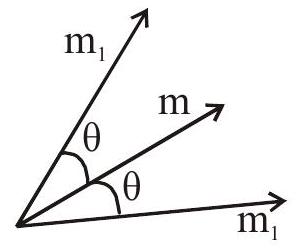
A line equally inclined with two lines

Let the two lines with slopes ${\mathrm{m}}_1$ and ${\mathrm{m}}_2$ be equally inclined to a line with slope $\mathrm{m}$ then $\left({\displaystyle \frac{{\mathrm{m}}_1-\mathrm{m}}{1+{\mathrm{m}}_1\mathrm{m}}}\right)=\pm \left({\displaystyle \frac{{\mathrm{m}}_2-\mathrm{m}}{1+{\mathrm{m}}_2\mathrm{m}}}\right)$

Special form of a line

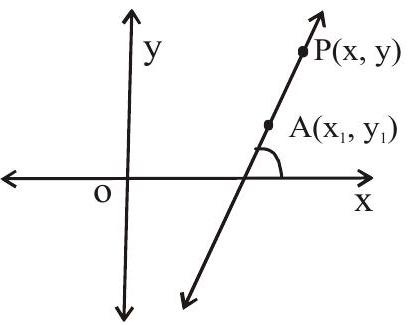
1. Point slope form

The equation of a line which passes through the point $({\mathrm{x}}_1,{\mathrm{y}}_1)$ and has the slope $\mathrm{m}$ is $\mathrm{y}-{\mathrm{y}}_1=\mathrm{m}(\mathrm{x}-$ ${\mathrm{x}}_1)$

2. Two point form

The equation of a line passing through two points $({\mathrm{x}}_1,{\mathrm{y}}_1)$ and $({\mathrm{x}}_2,{\mathrm{y}}_2)$ is

$y-y_1={\displaystyle \frac{y_2-y_1}{x_2-x_1}}(x-x_1)$

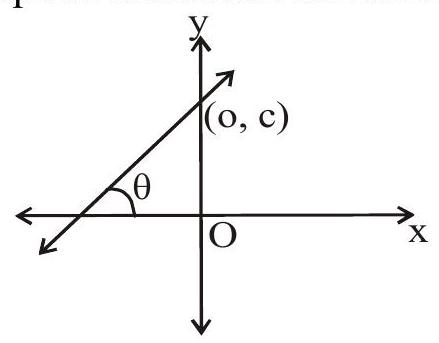
3. Slope and y intercept form [Non Vertical Line]

The equation of a line with slope $m$ that makes an intercept $c$ on $y$-axis is $y=mx+c$

Intercept form

The equation of a straight line which cuts off intercepts $\mathrm{a}$ and $\mathrm{b}$ on $\mathrm{x}$-axis and $\mathrm{y}$-axis respectively is given by

${\displaystyle \frac{\mathrm{x}}{\mathrm{a}}}+{\displaystyle \frac{\mathrm{y}}{\mathrm{b}}}=1$

${\displaystyle \frac{-x}{a}}+{\displaystyle \frac{y}{b}}=1$

${\displaystyle \frac{\mathrm{x}}{\mathrm{a}}}+{\displaystyle \frac{\mathrm{y}}{\mathrm{b}}}=-1$

${\displaystyle \frac{\mathrm{x}}{\mathrm{a}}}-{\displaystyle \frac{\mathrm{y}}{\mathrm{b}}}=1$

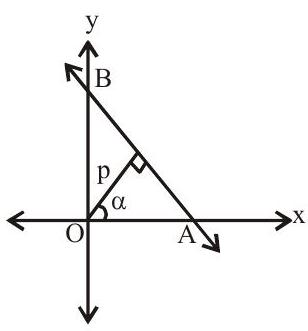
Normal form (perpendicular form)

The equation of a straight line upon which the length of the perpendicular from the origin is $\mathrm{p}$ and the perpendicular makes an angle $\alpha$ with the positive direction of $\mathrm{x}$-axis is given by $\mathrm{x}\cos \alpha +\mathrm{y}\sin \alpha =\mathrm{p}$

Note Here $\mathrm{p}$ is always taken as positive and $\alpha$ is measured from positive direction of $\mathrm{x}$-axis in anticlockwise direction between 0 and $2\pi$

Distance form or Symmetric form or Parametric Form

The equation of a straight line passing through the point $\left({\mathrm{x}}_{1,}{\mathrm{y}}_1\right)$ and making an angle $\theta$ with the positive direction of $\mathrm{x}$-axis is given by

${\displaystyle \frac{\mathrm{x}-{\mathrm{x}}_1}{\cos \theta }}={\displaystyle \frac{\mathrm{y}-{\mathrm{y}}_1}{\sin \theta }}=\mathrm{r}$

Where $\mathrm{r}$ is the distance of the point $(\mathrm{x},\mathrm{y})$ from the point $({\mathrm{x}}_1,{\mathrm{y}}_1)$

Parametric form

From the above equation we get

$\begin{array}{rl}& x-x_1=r\cos \theta ,y-y_1=r\sin \theta \\ & x=x_1+r\cos \theta ,y=y_1+r\sin \theta \end{array}$

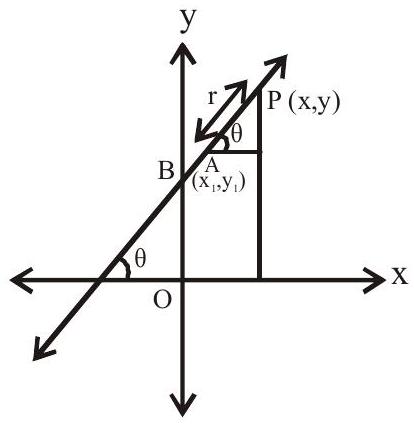
The coordinates $(\mathrm{x},\mathrm{y})$ of any point on the line at a distance $\mathrm{r}$ from the point $\mathrm{A}({\mathrm{x}}_1,{\mathrm{y}}_1)$ can be taken as

$({\mathrm{x}}_1+\mathrm{r}\cos \theta ,{\mathrm{y}}_1+\mathrm{r}\sin \theta )$

Where the line is inclined at an angle $\theta$ with positive direction of $\mathrm{x}$-axis.

1. If $\mathrm{P}$ is on the right side of $\mathrm{A}({\mathrm{x}}_1,{\mathrm{y}}_1)$ then $\mathrm{r}$ is positive and if $\mathrm{p}$ is on the left side of $\mathrm{A}({\mathrm{x}}_1,{\mathrm{y}}_1)$ then $r$ is negative.

2. At a given distance $\mathrm{r}$ from the point $({\mathrm{x}}_1,{\mathrm{y}}_1)$ on the line ${\displaystyle \frac{\mathrm{x}-{\mathrm{x}}_1}{\cos \theta }}={\displaystyle \frac{\mathrm{y}-{\mathrm{y}}_1}{\sin \theta }}$, there are two points viz; $({\mathrm{x}}_1+\mathrm{r}\cos \theta ,{\mathrm{y}}_1+\mathrm{r}\sin \theta )$ and $({\mathrm{x}}_1-\mathrm{r}\cos \theta ,{\mathrm{y}}_1-\mathrm{r}\sin \theta )$

1. Distance of a line from a point

Length of perpendicular (distance) from the point $(x_1,y_1)$ to the line $ax+by+c=0$ is given by $p={\displaystyle \frac{|a{x}_1+b{y}_1+c|}{\sqrt{a^2+b^2}}}$

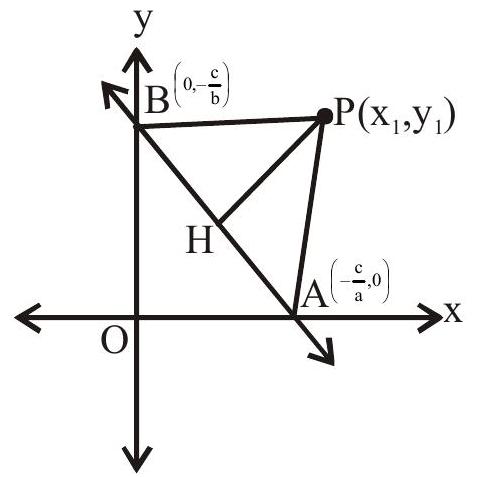
Length of perpendicular from the origin to the line $ax+by+c=0$ is given by

$\mathrm{p}={\displaystyle \frac{|\mathrm{c}|}{\sqrt{{\mathrm{a}}^2+{\mathrm{b}}^2}}}(\because ({\mathrm{x}}_1,{\mathrm{y}}_1)$ is $(0,0))$

2. Distance between two parallel lines

The distance between two parallel lines $ax+by+c_1=0$ and $ax+by+c_2=0$ is given by

$\mathrm{p}={\displaystyle \frac{|{\mathrm{c}}_1-{\mathrm{c}}_2|}{\sqrt{{\mathrm{a}}^2+{\mathrm{b}}^2}}}$

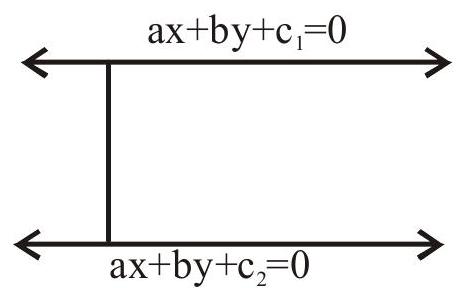
Note that coefficient of $\mathrm{x}$ and $\mathrm{y}$ of both equation must be same.

3. Area of a parallelogram

Area of a parallelogram $\mathrm{ABCD}=2$ area of $\mathrm{△}\mathrm{ABD}$

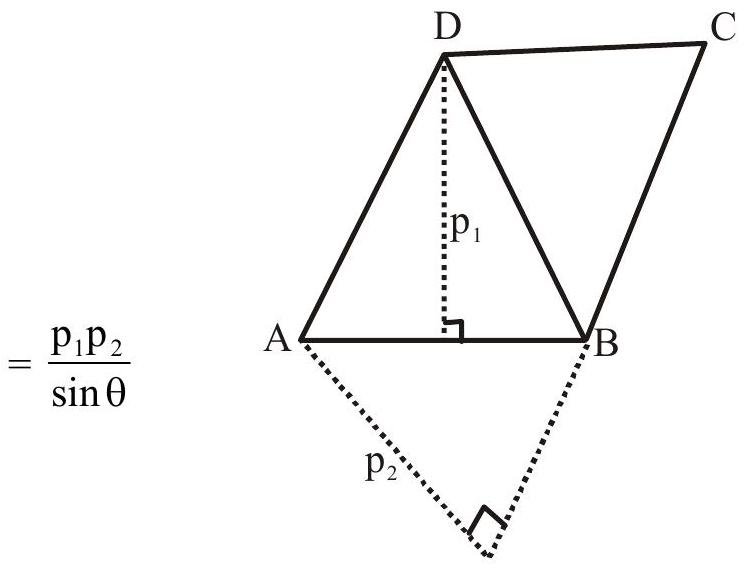
If the sides of a parallelogram be ${\mathrm{a}}_1\mathrm{x}+{\mathrm{b}}_1\mathrm{y}+{\mathrm{c}}_1=0;{\mathrm{a}}_1\mathrm{x}+{\mathrm{b}}_1\mathrm{y}+{\mathrm{d}}_1=0:{\mathrm{a}}_2\mathrm{x}+{\mathrm{b}}_2\mathrm{y}+{\mathrm{c}}_2=0,{\mathrm{a}}_2\mathrm{x}+{\mathrm{b}}_2\mathrm{y}+{\mathrm{d}}_2$

$=0$ area of parallelogram $=\left|{\displaystyle \frac{(c_1-d_1)(c_2-d_2)}{\left|\begin{array}{ll}{a}_1& b_1\\ a_2& b_2\end{array}\right|}}\right|$

Area of Rhombus

In case of a rhombus ${\mathrm{p}}_1={\mathrm{p}}_2$, area of rhombus $={\displaystyle \frac{{\mathrm{p}}_1{}^2}{\sin \theta }}$

Also area of rhombus $={\displaystyle \frac{1}{2}}{\mathrm{d}}_1{\mathrm{d}}_2$ where ${\mathrm{d}}_1,{\mathrm{d}}_2$ are the lengths of two perpendicular diagonals

Area of a triangle

Area of a triangle whose vertices are $({\mathrm{x}}_1,{\mathrm{y}}_1),({\mathrm{x}}_2,{\mathrm{y}}_2)$ and $({\mathrm{x}}_3,{\mathrm{y}}_3)$ is

$\begin{array}{rl}& {\displaystyle \frac{1}{2}}|{\mathrm{x}}_1({\mathrm{y}}_2-{\mathrm{y}}_3)+{\mathrm{x}}_2({\mathrm{y}}_3-{\mathrm{y}}_1)+{\mathrm{x}}_3({\mathrm{y}}_2-{\mathrm{y}}_1)|\\ & \text{ Or }{\displaystyle \frac{1}{2}}\left|\begin{array}{ll}{\mathrm{x}}_1& {\mathrm{y}}_1\\ {\mathrm{x}}_2& {\mathrm{y}}_2\\ {\mathrm{x}}_3& {\mathrm{y}}_3\\ {\mathrm{x}}_1& {\mathrm{y}}_1\end{array}\right|\\ & ={\displaystyle \frac{1}{2}}[({\mathrm{x}}_1{\mathrm{y}}_2+{\mathrm{x}}_2{\mathrm{y}}_3+{\mathrm{x}}_3{\mathrm{y}}_1)-({\mathrm{x}}_2{\mathrm{y}}_1+{\mathrm{x}}_3{\mathrm{y}}_2+{\mathrm{x}}_1{\mathrm{y}}_3)\mid \end{array}$

1. If area of a triangle is given then use $\pm$ sign.

2. If three points $\mathrm{A},\mathrm{B},\mathrm{C}$ are collinear then area of triangle $\mathrm{ABC}$ is zero.

3. If $a_{1}x+b_{1}y+c_1=0;a_{2}x+b_{2}y+c_2=0$ and $a_{3}x+b_{3}y+c_3=0$ are the three sides of a triangle, then the area of the triangle is given by

$\mathrm{\Delta }={\displaystyle \frac{1}{2{\mathrm{C}}_1{\mathrm{C}}_2{\mathrm{C}}_3}}{\left|\begin{array}{lll}{\mathrm{a}}_1& {\mathrm{b}}_1& {\mathrm{c}}_1\\ {\mathrm{a}}_2& {\mathrm{b}}_2& {\mathrm{c}}_2\\ {\mathrm{a}}_3& {\mathrm{b}}_3& {\mathrm{c}}_3\end{array}\right|}^2$

where ${\mathrm{C}}_1={\mathrm{a}}_2{\mathrm{b}}_3-{\mathrm{a}}_3{\mathrm{b}}_2$

$C_2=a_3{b}_1-a_1{b}_3$

${\mathrm{C}}_1,{\mathrm{C}}_2,{\mathrm{C}}_3\text{ are cofactors of }{\mathrm{c}}_1,{\mathrm{c}}_2$ & ${\mathrm{c}}_3$

Area of polygon

The area of polygon whose vertices are $({\mathrm{x}}_1,{\mathrm{y}}_1),\left({\mathrm{x}}_2{\mathrm{y}}_2\right)\dots \dots \dots \dots .\left({\mathrm{x}}_{\mathrm{n}1}{\mathrm{y}}_{\mathrm{n}}\right)$

$={\displaystyle \frac{1}{2}}\left|\begin{array}{cc}{\mathrm{x}}_1& {\mathrm{y}}_1\\ {\mathrm{x}}_2& {\mathrm{y}}_2\\ \cdot & \\ \cdot & \\ \cdot & \\ {\mathrm{x}}_{\mathrm{n}}& {\mathrm{y}}_{\mathrm{n}}\\ {\mathrm{x}}_1& {\mathrm{y}}_1\end{array}\right|={\displaystyle \frac{1}{2}}[({\mathrm{x}}_1{\mathrm{y}}_2+{\mathrm{x}}_2{\mathrm{y}}_3+{\mathrm{x}}_3{\mathrm{y}}_4\dots \dots .{\mathrm{x}}_{\mathrm{n}}{\mathrm{y}}_1)-({\mathrm{x}}_2{\mathrm{y}}_1+{\mathrm{x}}_3{\mathrm{y}}_2+{\mathrm{x}}_4{\mathrm{y}}_3\dots \dots {\mathrm{x}}_1{\mathrm{y}}_{\mathrm{n}})$

General Equation of straight line

First degree equation of the form

$ax+by+c=0$ where $a,b,c$ not all zero represent equation of straight line.

Reducing general equation to slope intercept form

Given equation is $\mathrm{ax}+\mathrm{by}+\mathrm{c}=0$

Rewrite the equation by $=-\mathrm{ax}-\mathrm{c}$

divide by $\mathrm{b}$ we get $\mathrm{y}={\displaystyle \frac{-\mathrm{a}}{\mathrm{b}}}\mathrm{x}{\displaystyle \frac{-\mathrm{c}}{\mathrm{b}}}$

It look like $\mathrm{y}=\mathrm{mx}+\mathrm{c}$

$\begin{array}{rl}& \text{ slope }={\displaystyle \frac{-a}{b}}=-\left({\displaystyle \frac{\text{ Coefficient of }x}{\text{ Coefficient of }y}}\right)=m\\ & y-\text{ intereept }={\displaystyle \frac{-c}{b}}=-\left({\displaystyle \frac{\text{ Constant }}{\text{ Coefficient of }y}}\right)\end{array}$

Reducing to intercept form

Given equation is $\mathrm{ax}+\mathrm{by}+\mathrm{c}=0$

Rewrite the equation $ax+by=-c$

${\displaystyle \frac{x}{-c/a}}+{\displaystyle \frac{y}{-c/b}}=1$

It look like ${\displaystyle \frac{\mathrm{x}}{\mathrm{a}}}+{\displaystyle \frac{\mathrm{y}}{\mathrm{b}}}=1$,

So intercepts are $-\mathrm{c}/\mathrm{a}$ & $\mathrm{c}/\mathrm{b}$ on $\mathrm{x}$-axis and $\mathrm{y}$-axis respectively.

Reduce to normal form

Given equation be $\mathrm{ax}+\mathrm{by}+\mathrm{c}=0$

Re write the equation $ax+by=-c$

$-ax-by=c$

Keeping constant term postive

Divide by $\sqrt{{\mathrm{a}}^2+{\mathrm{b}}^2}$ we get $-{\displaystyle \frac{\mathrm{a}}{\sqrt{{\mathrm{a}}^2+{\mathrm{b}}^2}}}\mathrm{x}-{\displaystyle \frac{\mathrm{b}}{\sqrt{{\mathrm{a}}^2+{\mathrm{b}}^2}}}\mathrm{y}={\displaystyle \frac{\mathrm{c}}{\sqrt{\mathrm{a}2+\mathrm{b}2}}}$

It look like $\mathrm{x}\cos \alpha +\mathrm{y}\sin \alpha =\mathrm{p}$

Where $\cos \alpha ={\displaystyle \frac{-\mathrm{a}}{\sqrt{{\mathrm{a}}^2+{\mathrm{b}}^2}}},\sin \alpha ={\displaystyle \frac{-\mathrm{b}}{\sqrt{{\mathrm{a}}^2+{\mathrm{b}}^2}}}($ or $)\tan \alpha ={\displaystyle \frac{\mathrm{b}}{\mathrm{a}}}$

$\because$ distance of a line from the origin is always positive

$\therefore {\displaystyle \frac{\mathrm{c}}{\sqrt{{\mathrm{a}}^2+{\mathrm{b}}^2}}}=\mathrm{p}$ is positive.

Image of a point in different cases

i. The image of a point with respect to the line mirror

Image of $\mathrm{A}({\mathrm{x}}_1,{\mathrm{y}}_1)$ with respect to line mirror $\mathrm{ax}+\mathrm{by}+\mathrm{c}=0$ be $\mathrm{B}({\mathrm{x}}_2,{\mathrm{y}}_2)$ is given by

${\displaystyle \frac{{\mathrm{x}}_2-{\mathrm{x}}_1}{\mathrm{a}}}={\displaystyle \frac{{\mathrm{y}}_2-{\mathrm{y}}_1}{\mathrm{b}}}={\displaystyle \frac{2({\mathrm{ax}}_1+{\mathrm{by}}_1+\mathrm{c})}{{\mathrm{a}}^2+{\mathrm{b}}^2}}$

$M$ is the foot of prependicular from $A$ on $ax+by+c=0$

ii. The image of a point with respect to $x$-axis:-

Let $\mathrm{A}({\mathrm{x}}_1,{\mathrm{y}}_1)$ be any point and $\mathrm{B}({\mathrm{x}}_2,{\mathrm{y}}_2)$ its image after reflection in the $\mathrm{x}$-axis then. is the mid point of $A$ & $B)$

$\mathrm{M}$ is the foot of perpendicular from $\mathrm{A}$ on $\mathrm{x}$-axis

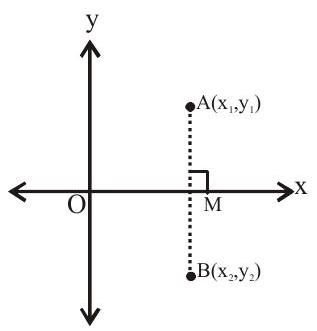
iii. The image of a point with respect to $y$-axis:then.

Let $\mathrm{A}({\mathrm{x}}_1,{\mathrm{y}}_1)$ be any point and $\mathrm{B}({\mathrm{x}}_2,{\mathrm{y}}_2)$ its image after reflection in the mirror $\mathrm{y}$-axis

$x_2=-x_1$ & $y_1=y_2(N$ is the mid point of $A$ & $B)$

$\mathrm{N}$ is the foot of perpendicular from $\mathrm{A}$ on $\mathrm{y}$-axis

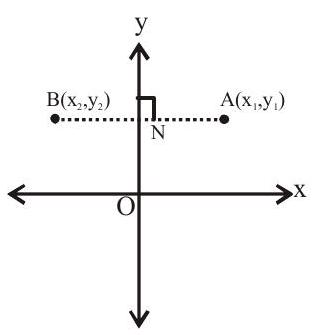
iv. The image of a point with respect to the origin:-

Let $A\left(x_1{y}_1\right)$ be any point $B\left(x_2{y}_2\right)$ be its image after reflection through the origin then.

$x_2=-x_1$ & $y_2=-y_1(O$ is the mid point of $A$ & $B)$

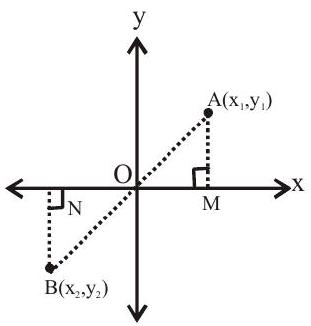
v. The image of a point with respect to the line $y=x$

Let $\mathrm{A}\left({\mathrm{x}}_{1,}{\mathrm{y}}_1\right)$ be any point and $\mathrm{B}({\mathrm{x}}_2,{\mathrm{y}}_2)$ be its image after reflection in the line $\mathrm{y}=\mathrm{x}$ then.

${\mathrm{y}}_2={\mathrm{x}}_1$ and ${\mathrm{x}}_2={\mathrm{y}}_1(\mathrm{M}$ is the mid point of $A$ & $B)$

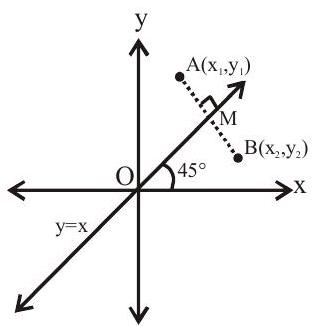
vi The image of a point with respect to the line $\mathbf{y}=\mathbf{x}\mathbf{t}\mathbf{a}\mathbf{n}\theta$

Let $\mathrm{A}({\mathrm{x}}_1,{\mathrm{y}}_1)$ be any point and $\mathrm{B}({\mathrm{x}}_2,{\mathrm{y}}_2)$ be its image after reflection in the line $\mathrm{y}=\mathrm{x}\tan \theta$ or $\mathrm{y}=\mathrm{mx}$ then.

${\mathrm{x}}_2={\mathrm{x}}_1\cos 2\theta +{\mathrm{y}}_1\sin 2\theta$ (M is the mid point of A & B)

${\mathrm{y}}_2={\mathrm{x}}_1\cos 2\theta -{\mathrm{y}}_1\sin 2\theta$

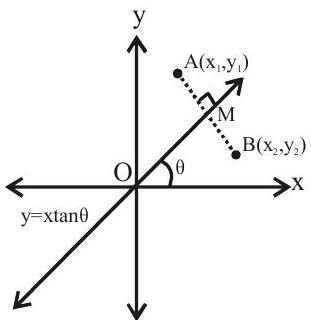
Line parallel and perpendicular to given line

Given equation of straight line be $ax+by+c=0$

A line parallel to given line is $\mathrm{ax}+\mathrm{by}+\mathrm{d}=0$ only constant term changes.

A line perpendicular to given line is $bx-ay+k=0$

Here change coordinate of $x$ as coordinate of $y$ & coordinate of $y$ as negative of coordi nate of $x$ and constant term changes

It the lines ${\mathrm{a}}_1\mathrm{x}+{\mathrm{b}}_1\mathrm{y}+{\mathrm{c}}_1=0$ and ${\mathrm{a}}_2\mathrm{x}+{\mathrm{b}}_2\mathrm{y}+{\mathrm{c}}_2=0$ are prependecular then ${\mathrm{a}}_1{\mathrm{a}}_2+{\mathrm{b}}_1{\mathrm{b}}_2=0$

i. Coincident, if ${\displaystyle \frac{{\mathrm{a}}_1}{{\mathrm{a}}_2}}={\displaystyle \frac{{\mathrm{b}}_1}{{\mathrm{b}}_2}}={\displaystyle \frac{{\mathrm{c}}_1}{{\mathrm{c}}_2}}$

ii. Parallel, if ${\displaystyle \frac{{\mathrm{a}}_1}{{\mathrm{a}}_2}}={\displaystyle \frac{{\mathrm{b}}_1}{{\mathrm{b}}_2}}\ne {\displaystyle \frac{{\mathrm{c}}_1}{{\mathrm{c}}_2}}$

iii. Intersecting, if ${\displaystyle \frac{{\mathrm{a}}_1}{{\mathrm{a}}_2}}\ne {\displaystyle \frac{{\mathrm{b}}_1}{{\mathrm{b}}_2}}$

1. Concurrent lines

The three given lines are concurrent if they meet at one point.

i. Find the point of intersection of any two lines by solving them simultaneously. If this point satisfies the third equation also then the given lines are concurrent.

ii. Let three lines be

${\mathrm{L}}_1={\mathrm{a}}_1\mathrm{x}+{\mathrm{b}}_1\mathrm{y}+{\mathrm{c}}_1=0{\mathrm{L}}_2={\mathrm{a}}_2\mathrm{x}+{\mathrm{b}}_2\mathrm{y}+{\mathrm{c}}_2=0$ and

${\mathrm{L}}_3={\mathrm{a}}_3\mathrm{x}+{\mathrm{b}}_3\mathrm{y}+{\mathrm{c}}_3=0$ are concurrent if

$\left|\begin{array}{lll}{\mathrm{a}}_1& {\mathrm{b}}_1& {\mathrm{c}}_1\\ {\mathrm{a}}_2& {\mathrm{b}}_2& {\mathrm{c}}_2\\ {\mathrm{a}}_3& {\mathrm{b}}_3& {\mathrm{c}}_3\end{array}\right|=0$

iii. The three lines ${\mathrm{L}}_1=0,{\mathrm{L}}_2=0$ and ${\mathrm{L}}_3=0$ are concurrent if there exist constants $\ell ,\mathrm{m}$ and $n$ not all zero at the same time, such that

$\ell {\mathrm{L}}_1+{\mathrm{mL}}_2+{\mathrm{nL}}_3=0$

2. Equations of angle bisectors between two lines

The equations of the bisectors of the angles between the lines $a_{1}x+b_{1}y+c_1=0$ and ${\mathrm{a}}_2\mathrm{x}+{\mathrm{b}}_2\mathrm{y}+{\mathrm{c}}_2=0$

are given by ${\displaystyle \frac{|a_{1}x+b_{1}y+c_1|}{\sqrt{a_1^2+b_1^2}}}=\pm {\displaystyle \frac{a_{2}x+b_{2}y+c_2}{\sqrt{a_2^2+b_2^2}}}$

i. Any point on a bisector is equidistant from the given lines.

ii. Locus of points which are equidistant from the two intersecting lines is an angle bisector.

iii. Bisectors are perpendicular to each other.

iv. Equation of the bisector of the acute and of obtuse angle between two lines.

Let $a_{1}x+b_{1}y+c_1=0\dots \dots \dots (1)$

and $a_{2}x+b_{2}y+c_2=0\dots \dots \dots (2)$

${\mathrm{c}}_1>0,{\mathrm{c}}_2>0$ then the equation

${\displaystyle \frac{{\mathrm{a}}_1\mathrm{x}+{\mathrm{b}}_1\mathrm{y}+{\mathrm{c}}_1}{\sqrt{{\mathrm{a}}_1^2+{\mathrm{b}}_1^2}}}=+{\displaystyle \frac{{\mathrm{a}}_2\mathrm{x}+{\mathrm{b}}_2\mathrm{y}+{\mathrm{c}}_2}{\sqrt{{\mathrm{a}}_2^2+{\mathrm{b}}_2^2}}}$

is the bisector of the acute or obtuse angle between the lines $1$ & $2$ according as ${\mathrm{a}}_1{\mathrm{a}}_2+{\mathrm{b}}_1{\mathrm{b}}_2<0$ or $>0$

again ${\displaystyle \frac{a_1\mathrm{x}+{\mathrm{b}}_1\mathrm{y}+{\mathrm{c}}_1}{\sqrt{{\mathrm{a}}_1^2+{\mathrm{b}}_1^2}}}=-{\displaystyle \frac{{\mathrm{a}}_2\mathrm{x}+{\mathrm{b}}_2\mathrm{y}+{\mathrm{c}}_2}{\sqrt{{\mathrm{a}}_2^2+{\mathrm{b}}_2^2}}}$

is the bisector of the acute or obtuse angle between the lines $1$ & $2$ according as ${\mathrm{a}}_1{\mathrm{a}}_2+{\mathrm{b}}_1{\mathrm{b}}_2>0$ or $<0$

3. Position of two points relative to a given line

Let the line be $\mathrm{L}=\mathrm{ax}+\mathrm{by}+\mathrm{c}=0$

$\mathrm{P}({\mathrm{x}}_1,{\mathrm{y}}_1)$ and $\mathrm{A}({\mathrm{x}}_2,{\mathrm{y}}_2)$ are two given points.

i. If ${\mathrm{ax}}_1+b{\mathrm{by}}_1+\mathrm{c}$ and ${\mathrm{ax}}_2+\mathrm{by}{\mathrm{y}}_2+\mathrm{c}$ both are of the same sign and hence ${\displaystyle \frac{a{x}_1+b{y}_1+c}{a{x}_2+b{y}_2+c}}>0$ then the points $\mathrm{P}$ and $\mathrm{Q}$ lie on same side of line $\mathrm{ax}+\mathrm{by}+\mathrm{c}=0$

ii. If ${\mathrm{ax}}_1+{\mathrm{by}}_1+\mathrm{c}$ and ${\mathrm{ax}}_2+{\mathrm{by}}_2+\mathrm{c}$ are of opposite sign and hence ${\displaystyle \frac{a{x}_1+b{y}_1+c}{a{x}_2+b{y}_2+c}}<0$ then the points $\mathrm{P}$ and $\mathrm{Q}$ lie on opposite side of the line $\mathrm{ax}+\mathrm{by}+\mathrm{c}=0$

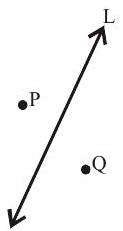
iii. If origin lie on line then the line is known as origin side.

iv. A point $\left(x_1{y}_1\right)$ will lie on origin side of the line $ax+by+c=0$ if $a{x}_1+b{y}_1+c$ and $c$ have same sign.

v. A point $\left({\mathrm{x}}_{1,}{\mathrm{y}}_1\right)$ will lie on non-origin side of the line $\mathrm{ax}+\mathrm{by}+\mathrm{c}=0$ if ${\mathrm{ax}}_1+{\mathrm{by}}_1+\mathrm{c}$ and $\mathrm{c}$ have opposite sign

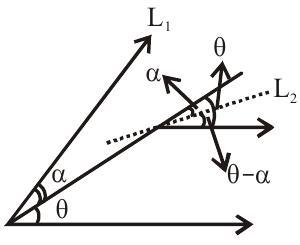
Family of straight lines

Let ${\mathrm{L}}_1={\mathrm{a}}_1\mathrm{x}+{\mathrm{b}}_1\mathrm{y}+{\mathrm{c}}_1=0$ and ${\mathrm{L}}_2={\mathrm{a}}_2\mathrm{x}+{\mathrm{b}}_2\mathrm{y}+{\mathrm{c}}_2=0$

Then the general equation of any straight line passing through the point of intersection of lines $L_1$ and $L_2$ is given by $L_1+\lambda L_2=0$ where $\lambda$ is any real number.

Equations of straight lines through $(x_1,y_1)$ making angle $\alpha$ with $y=mx+c$

$y-y_1={\displaystyle \frac{m+\tan \alpha }{1-m\tan \alpha }}(x-x_1)$ and $y-y_1={\displaystyle \frac{m-\tan \alpha }{1+m\tan \alpha }}(x-x_1)$

Standard points of a triangle

1. Centroid or centre of gravity :-

The centroid of a triangle is the point of intersection of its median’s. the centroid divides the median in the ratio 2:1 (vertex:base).

Coordinates of G are $G({\displaystyle \frac{x_1+x_2+x_3}{3}},{\displaystyle \frac{y_1+y_2+y_3}{3}})$

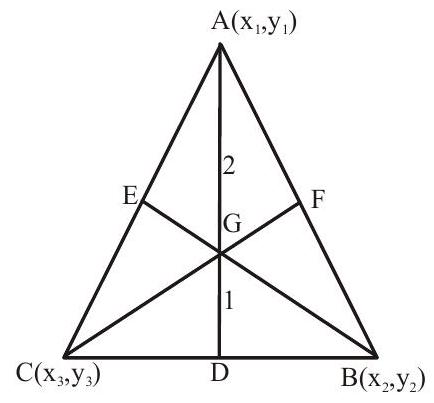
In isosceles triangle median to the equal sides are equal in length and in equilateral triangle all medians are equal in length.

Equations of median can be obtained by using two point form with vertex and the mid point of opposite side.

2. Circumcentre:-

The circumcentre of a triangle is the point of intersection of the perpendicular bisectors of the sides of a triangle.

Coordinates of $\mathrm{O}$ can be obtained from the equation.

${\mathrm{OA}}^2={\mathrm{OB}}^2={\mathrm{OC}}^2$

If angles $\mathrm{A},\mathrm{B},\mathrm{C}$ and vertices $\mathrm{A}({\mathrm{x}}_1,{\mathrm{y}}_1),\mathrm{B}({\mathrm{x}}_2,{\mathrm{y}}_2)$ and $\mathrm{C}({\mathrm{x}}_3,{\mathrm{y}}_3)$ of a $\mathrm{△}\mathrm{ABC}$ are given; Then its circumcentre is given by $\{{\displaystyle \frac{x_1\sin 2A+x_2\sin 2B+x_3\sin 2C}{\sin 2A+\sin 2B+\sin 2C}},{\displaystyle \frac{y_1\sin 2A+y_2\sin 2B+y_3\sin 2C}{\sin 2A+\sin 2B+\sin 2C}}\}$

The circumcentre of a right-angled triangle is the mid-point of its hypotenuse. Therefore the mid-point of hypotenuse is equidstant from its vertices

$\mathrm{AM}=\mathrm{BM}=\mathrm{CM}$

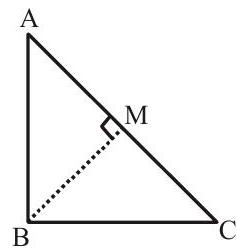
The circumcentre of the triangle formed by $(0,0),({\mathrm{x}}_1,{\mathrm{y}}_1)$ and $({\mathrm{x}}_2,{\mathrm{y}}_2)$ is

$({\displaystyle \frac{{\mathrm{y}}_2({\mathrm{x}}_1^2+{\mathrm{y}}_1^2)-{\mathrm{y}}_1({\mathrm{x}}_2^2+{\mathrm{y}}_2^2)}{2({\mathrm{x}}_1{\mathrm{y}}_2-{\mathrm{x}}_2{\mathrm{y}}_1)}},{\displaystyle \frac{{\mathrm{x}}_2({\mathrm{x}}_1^2+{\mathrm{y}}_1^2)-{\mathrm{x}}_1({\mathrm{x}}_2^2+{\mathrm{y}}_2^2)}{2({\mathrm{x}}_2{\mathrm{y}}_1-{\mathrm{x}}_1{\mathrm{y}}_2)}})$

3. Incentre of a triangle :-

The point of intersection of the internal bisecters of the angles of a

triangle is called the incentre of the triangle.

The coordinates of the incentre of a triangle with vertices $({\mathrm{x}}_1,{\mathrm{y}}_1),({\mathrm{x}}_2,{\mathrm{y}}_2)$ and $({\mathrm{x}}_3,{\mathrm{y}}_3)$ are $({\displaystyle \frac{{\mathrm{ax}}_1+{\mathrm{bx}}_2+{\mathrm{cx}}_3}{\mathrm{a}+\mathrm{b}+\mathrm{c}}},{\displaystyle \frac{{\mathrm{ay}}_1+{\mathrm{by}}_2+{\mathrm{cy}}_3}{\mathrm{a}+\mathrm{b}+\mathrm{c}}})$

Where $a,b,c$ are lengths of sides of triangle

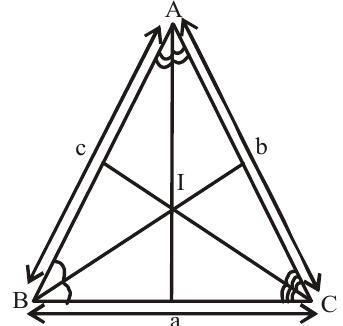
The incentre of the triangle formed by $(0,0),(a,0),(0,b)$ is $({\displaystyle \frac{ab}{a+b+\sqrt{a^2+b^2}}},{\displaystyle \frac{ab}{a+b+\sqrt{a^2+b^2}}})$

4. Orthocentre :-

The orthocentre of a triangle is a point intersection of altitudes.

i. Take the equations of any two sides of a triangle. find the eqnations the lines perpendicular to those lines and passing through the opposite vertices. solve these two equations we get orthocentre of the triangle.

ii. If angles $\mathrm{A},\mathrm{B},\mathrm{C}$ and vertices $\mathrm{A}({\mathrm{x}}_1,{\mathrm{y}}_1)\mathrm{B}({\mathrm{x}}_2,{\mathrm{y}}_2)$ and $\mathrm{C}({\mathrm{x}}_3,{\mathrm{y}}_3)$ of a $\mathrm{△}\mathrm{ABC}$ are given

then orthocentre of $\mathrm{△}\mathrm{ABC}$ is given by

$({\displaystyle \frac{x_1\tan A+x_2\tan B+x_3\tan C}{\tan A+\tan B+\tan C}},{\displaystyle \frac{y_1\tan A+y_2\tan B+y_3\tan C}{\tan A+\tan B+\tan C}})$

iii. If any two lines out of three lines $\mathrm{AB},\mathrm{BC},\mathrm{CA}$, are perpendicular, then orthocentre is the point of intersection of two perpendicular lines.

iv. The orthocentre of the triangle with verties $(0,0),({\mathrm{x}}_1,{\mathrm{y}}_1)$ and $({\mathrm{x}}_2,{\mathrm{y}}_2)$ is

$(({\mathrm{y}}_1-{\mathrm{y}}_2)\left({\displaystyle \frac{{\mathrm{x}}_1{\mathrm{x}}_2-{\mathrm{y}}_1{\mathrm{y}}_2}{{\mathrm{x}}_2{\mathrm{y}}_1-{\mathrm{x}}_1{\mathrm{y}}_2}}\right),({\mathrm{x}}_1-{\mathrm{x}}_2)\left({\displaystyle \frac{{\mathrm{x}}_1{\mathrm{x}}_2+{\mathrm{y}}_1{\mathrm{y}}_2}{{\mathrm{x}}_1{\mathrm{y}}_2-{\mathrm{x}}_2{\mathrm{y}}_1}}\right))$

v. The orthocentre $(\mathrm{O})$, centroid $(\mathrm{G})$ and circumcentre $(\mathrm{C})$ of any triangle lie in a straight line and $\mathrm{G}$ divides the join of $\mathrm{O}$ and $\mathrm{C}$ in the ratio $2:1$

vi. In an equilateral triangle,orthocentre, centroid, circumcentre and incentre conside.

5. Coordinates of nine point circle :-

If a circle passes through the foot of perpendicular (D,E,F) mid points of sides $\mathrm{BC},\mathrm{CA},\mathrm{AB}$ respectively $(\mathrm{H},\mathrm{I},\mathrm{J})$ and mid points of the line joining the orthocentre $\mathrm{O}$ to the angular points A,B,C (K,L,M) thus the nine points D,E,F H,I,J, K,L,M all lie on a circle, This circle is known as nine point circle and its centre is called nine point centre.

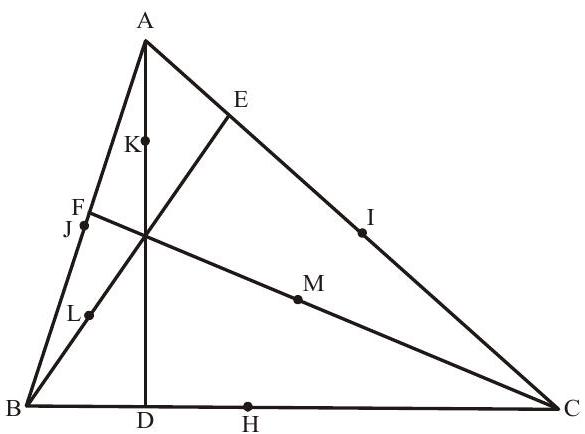
1. The orthocentre (0), nine point centre (N) centroid (G) and circumcentre (C) all lie in the same line i.e. ONGC (oil natural gas corporation)

2. The nine point centre bisects the join of orthocentre (O) and circumcentre (C)

3. The radius of nine point circle is half the radius of circumcircle.

Examples:

1. If the centroid and circumcentre of a triangle are $(3,3)$ and $(6,2)$ respectively, then the orthocentre is

(a) $(-3,5)$

(b) $(-3,1)$

(c) $(3,-1)$

(d) $(9,5)$

Show Answer

Solution: Centroid, circumcentre and orthocenter are collinear such that centroid divides the circumcenter and orthocentre in the ratio 1:2. Ans(-3,5).

2. If the algebraic sum of the perpendicular distances from the point $(2,0),(0,2)$ and $(1,1)$ to a variable straight line be zero, then the line passes through the point

(a) $(3,3)$

(b) $(1,1)$

(c) $(1,-1)$

(d) $(-1,-1)$

Show Answer

Solution: Let equation be $\mathrm{ax}+\mathrm{by}+\mathrm{c}=0$

A.T.Q. ${\displaystyle \frac{2a+ob+c}{\sqrt{a^2+b^2}}}+{\displaystyle \frac{0+2b+c}{\sqrt{a^2+b^2}}}+{\displaystyle \frac{a+b+c}{\sqrt{a^2+b^2}}}=0$

$\Rightarrow 3\mathrm{a}+3\mathrm{b}+3\mathrm{c}=0\Rightarrow \mathrm{a}+\mathrm{b}+\mathrm{c}=0$

This shows that $ax+by+c=0$ passes through $(1,1)$.

3. The area enclosed by $2|x|+3|y|\le 6$ is

(a) 3 Sq-units

(b) 12 Sq.units

(c) 9 Sq.units

(d) 24 Sq.units

Show Answer

Solution: $2x+3y\le 6,x\ge 0,y\ge 0$

$\begin{array}{ll}2x-3y\le 6,& x\ge 0,y\le 0\\ -2x+3y& \le 6,x\le 0,y\ge 0\\ -2x-3y& \le 6,x\le 0,y\le 0\end{array}$

Form a rhombus with diagonals $4$ & $6$.

Area $={\displaystyle \frac{1}{2}}\times 4\times 6=12$ sq. units.

4. The number of integer values of $m$ for which the $x$-coordinate of the point of intersection of the lines $3x+4y=9$ and $y=mx+1$ is also an integer is

(a) 2

(b) 0

(c) 4

(d) 1

Show Answer

Solution: Point of intersection $({\displaystyle \frac{5}{3+4m}},{\displaystyle \frac{3+9m}{3+4m}})$

$x$-coordinate is an integer when $3+4\mathrm{m}=\pm 1$ or $\pm 5$.

$\mathrm{m}=-1,-2,-{\displaystyle \frac{1}{2}},{\displaystyle \frac{1}{2}}$

Hence there are two values of $\mathrm{m}$.

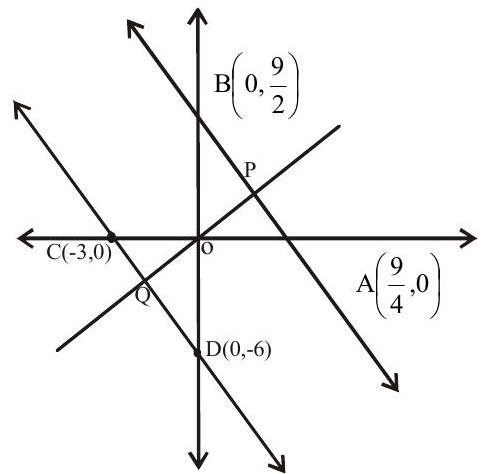
5. A straight line through the origin meets the parallel lines $4x+2y=9$ and $2x+y+6=0$ at points $P$ & $\mathrm{Q}$ respectively. Then the point $\mathrm{O}$ divides the segment $\mathrm{PQ}$ in the ratio

(a) $1:2$

(b) $3:4$

(c) $2:1$

(d) $4:3$

Show Answer

Solution: Clearly ${}_{\mathrm{\Delta }}\mathrm{OPA}\sim \mathrm{\Delta }\mathrm{OQC}$.

$\Rightarrow {\displaystyle \frac{\mathrm{OP}}{\mathrm{OQ}}}={\displaystyle \frac{\mathrm{OA}}{\mathrm{OC}}}={\displaystyle \frac{9/4}{3}}={\displaystyle \frac{3}{4}}$

6. A family of lines is given by $(1+2\lambda )\mathrm{x}+(1-\lambda )\mathrm{y}+\lambda =0,\lambda$ being the parameter. The line belonging to this family at the maximum distance from the $\mathrm{pt}(1,4)$ is

(a) $4x-y+1=0$

(b) $33x+12y+7=0$

(c) $12x+33y=7$

(d) None of these.

Show Answer

Solution: $x+y+\lambda (2x-y+1)=0$

The required line is $y=-{\displaystyle \frac{1}{3}}={\displaystyle \frac{4-{\displaystyle \frac{1}{3}}}{1+{\displaystyle \frac{1}{3}}}}(x+{\displaystyle \frac{1}{3}})\Rightarrow 12x+33y=7$.

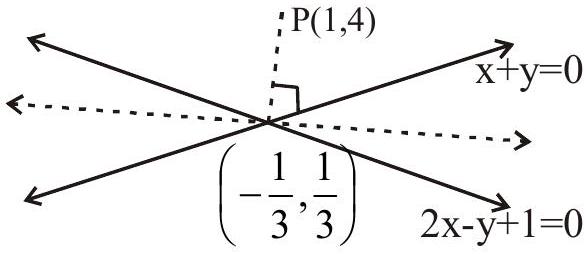
7. The four sides of a quadrilateral are given by the equation $xy(x-2)(y-3)=0$. The equation of the line parallel to $x-4y=0$ that divides the quadrilateral in two equal areas is

(a) $\mathrm{x}-4\mathrm{y}+5=0$

(b) $\mathrm{x}-4\mathrm{y}-5=0$

(c) $4y=x+1$

(d) $4y+1=x$.

Show Answer

Solution: ar.of $\mathrm{OADE}={\displaystyle \frac{1}{2}}$ ar $\mathrm{OABC}$

${\displaystyle \frac{1}{2}}(\mathrm{OE}+\mathrm{AD})\times \mathrm{OA}={\displaystyle \frac{1}{2}}\times \mathrm{OA}\times \mathrm{AB}$

${\displaystyle \frac{1}{2}}(-{\displaystyle \frac{\lambda }{4}}+{\displaystyle \frac{2-\lambda }{4}})\times 2={\displaystyle \frac{1}{2}}\times 2\times 3$

${\displaystyle \frac{2-2\lambda }{4}}=3$

$\therefore \lambda =-5$

$\therefore$ Equation is $\mathrm{x}-4\mathrm{y}+5=0$

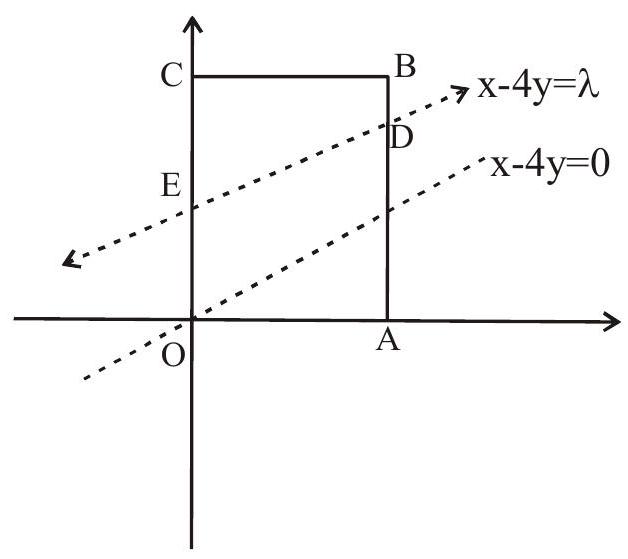
Exercise

1. A triangle $\mathrm{ABC}$ with vertices $\mathrm{A}(-1,0),\mathrm{B}(-2,{\displaystyle \frac{3}{4}})$ & $\mathrm{C}(-3,-{\displaystyle \frac{7}{6}})$ has its orthocenter $\mathrm{H}$.

Then the orthocentre of triangle $\mathrm{BCH}$ will be

(a) $(-1,0)$

(b) $-3,-2)$

(c) $(1,3)$

(d) $(-1,2)$

Show Answer

Answer: a

2. The points $\mathrm{A}(0,0),\mathrm{B}(\cos \alpha ,\sin \alpha )$ and $\mathrm{C}(\cos \beta ,\sin \beta )$ are the vertices of a right angled triangle if

(a) $\sin \left({\displaystyle \frac{\alpha +\beta }{2}}\right)={\displaystyle \frac{1}{\sqrt{2}}}$

(b) $\cos \left({\displaystyle \frac{\alpha +\beta }{2}}\right)={\displaystyle \frac{1}{\sqrt{2}}}$

(c) $\cos \left({\displaystyle \frac{\alpha -\beta }{2}}\right)={\displaystyle \frac{1}{\sqrt{2}}}$

(d) $\sin \left({\displaystyle \frac{\alpha +\beta }{2}}\right)=-{\displaystyle \frac{1}{\sqrt{2}}}$

Show Answer

Answer: c

3. Set of values of $\alpha$ for which the point $(\alpha ,{\alpha }^2-2)$ lies inside the triangle formed by the lines $\mathrm{x}+\mathrm{y}=1,\mathrm{y}=\mathrm{x}+1$ and $\mathrm{y}=-1$ is

(a) $({\displaystyle \frac{1-\sqrt{13}}{2}},-1)\mathrm{U}(1,{\displaystyle \frac{-1+\sqrt{13}}{2}})$

(b) $(1,\sqrt{13})$

(c) $(-\sqrt{13},-1)$

(d) None

Show Answer

Answer: a

4. Area of the parallelogram formed by the lines $y=mx,y=mx+1,y=nx+1$ equals

(a) ${\displaystyle \frac{|\mathrm{m}+\mathrm{n}|}{(\mathrm{m}-\mathrm{n}{)}^2}}$

(b) ${\displaystyle \frac{2}{|m+n|}}$

(c) ${\displaystyle \frac{1}{|m+n|}}$

(d) ${\displaystyle \frac{1}{|m-n|}}$

Show Answer

Answer: d

5. $\mathrm{A}$ and $\mathrm{B}$ are fixed points. The vertex $\mathrm{C}$ of $\mathrm{△}\mathrm{ABC}$ moves such that $\cot \mathrm{A}+\cot \mathrm{B}=$ constant. The locus of $C$ is a straight line

(a) perpendicular to $\mathrm{AB}$

(b) parallel to $\mathrm{AB}$

(c) inclined at an angle $(\mathrm{A}-\mathrm{B})$ to $\mathrm{AB}$

(d) None of these.

Show Answer

Answer: b

6. The area of the figure formed by $\mathrm{a}|\mathrm{x}|+\mathrm{b}|\mathrm{y}|+\mathrm{c}=0$ is

(a) ${\displaystyle \frac{{\mathrm{c}}^2}{|\mathrm{ab}|}}$

(b) ${\displaystyle \frac{2{\mathrm{c}}^2}{|\mathrm{ab}|}}$

(c) ${\displaystyle \frac{c^2}{2|ab|}}$

(d) None of these

Show Answer

Answer: b

7. The orthocentre, circumcentre, centroid and incentre of the triangle formed by the line $\mathrm{x}+\mathrm{y}$ $=$ a with the coordinate axes lie on

(a) $x^2+y^2=1$

(b) $y=x$

(c) $y=2x$

(d) $y=3x$

Show Answer

Answer: b

8. Two points $(a,3)$ and $(5,b)$ are the opposite vertices of a rectangle. If the other two vertices lie on the line $y=2x+c$ which passes through the point $(a,b)$ then the value of $c$ is

(a) -7

(b) -4

(c) 0

(d) 7

Show Answer

Answer: a