Coordinate Geometry-i Circles (Lecture-01)
BASIC CONCEPTS
Circle : A circle is the locus of points which are equidistant from a fixed point and lies on the same plane.
Fixed point is called centre of a circle and constant distance is called radius of the circle
STANDARD EQUATION OF A CIRCLE
The equation of a circle with the centre at
If centre is at the origin and radius is
GENERALEQUATION OF A CIRCLE
centre
CONDITIONS FOR A SECOND DEGREE EQUATION TO REPRESENT A CIRCLE
(i) coefficient of
(ii)
If
If
If
EQUATION OF CIRCLE IN VARIOUS FORMS
1. Equation of circle with centre (h.k) and passes through origin. is
Note that when a circle passes through origin the constant term must be zero
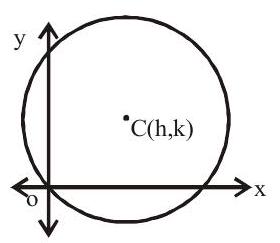
2. If the circle touches
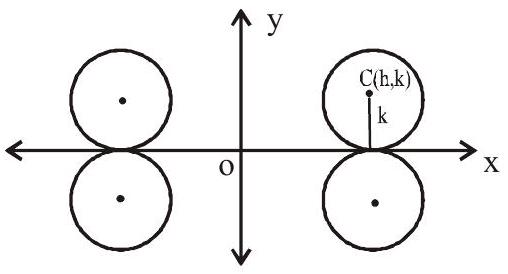
3. If the circle touches
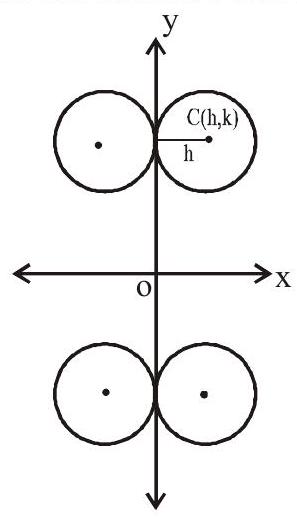
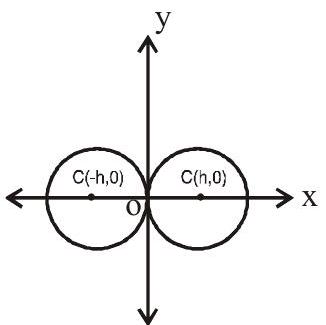
4. If the circle touches both the axes then its equation is

5. If the circle touches

6. If the circle touches
7. If the circle passes through origin and cuts intercepts
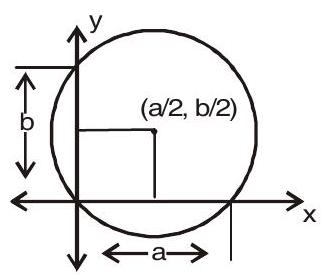
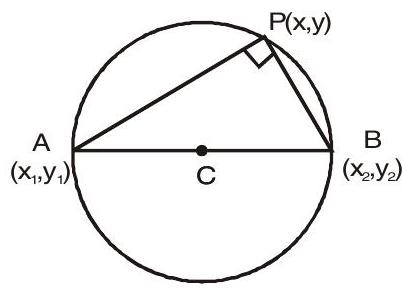
8. Equation of Circle on a given Diameter
If
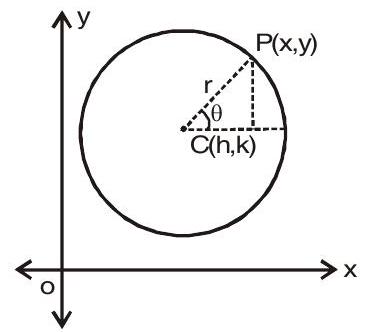
9. Parametric form of Circle
Where
In particular coordinates of any point on the circle
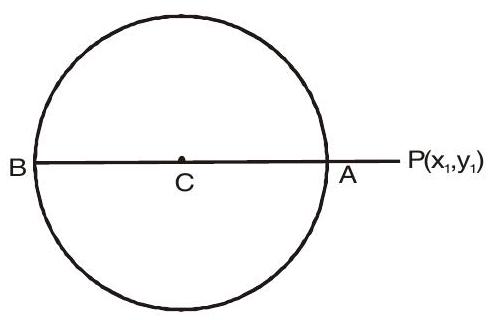
10. Intercept made on the axes by a circle
Let the equation of circle is
Position of a point with respect to a circle .
let the circle is
Point
(or)

Maximum and Minimum Distance of a point from the circle
Let the circle
The maximum and minimum distance from
Line and a circle
Let
(i)

(ii)
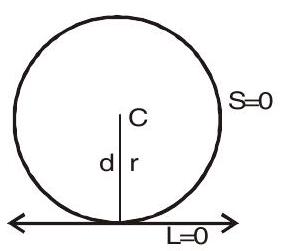
(iii)
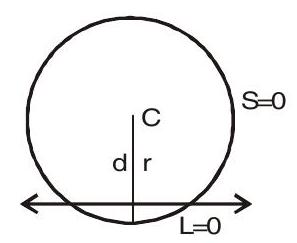
(iv)

If
(i)
(ii)
(iii)
Equations of Tangents
Point form :
Equation of the tangent to the circle
Parametric forms
Equation of the tangent to the circle
Slope form
The equation of a tangent of slope
The coordinates of the point of contact are
(i) Condition for a line
(ii) Condition that the line
(iii) Equation of tangent to the circle
(iv) The line
(v) If the line
(vi) If the line
Tangents from a point outside the circle
If circle is
Squaring
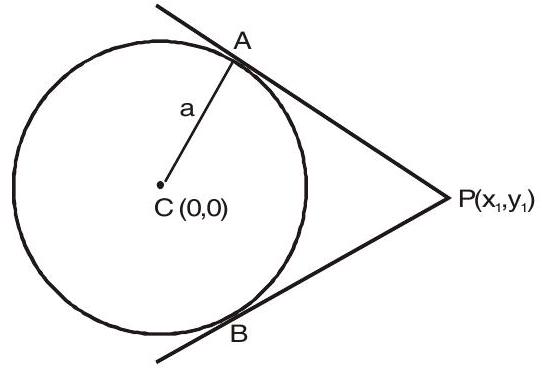
We get two equations of tangents.
Let the circle be
Let the slope of the tangent is
Now find the distance of this line from the centre
Length of the tangent from a point to a circle.
Let the circle be

Power of point with respect to a circle
The power of

Note :
(i) The power of the point outside the circle is positive
(ii) The power of the point on the circle is zero
(iii) The power of the point inside the circle is negative
Pair of tangents
The equation of the pair of tangents drawn from the point
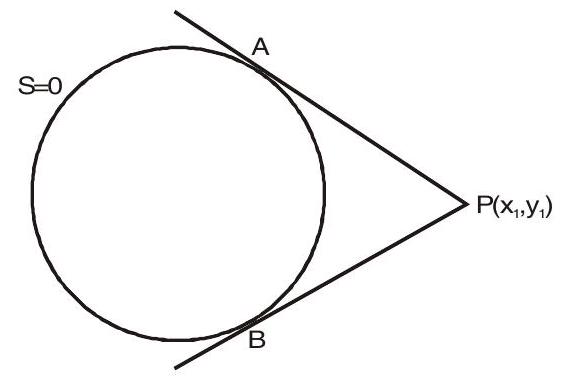
Where
Note : The pair of tangents from
Normal to a circle at a given point
The normal of a circle at any point is a straight line which is perpendicular to the tangent at the point and always passes through the centre of the circle.
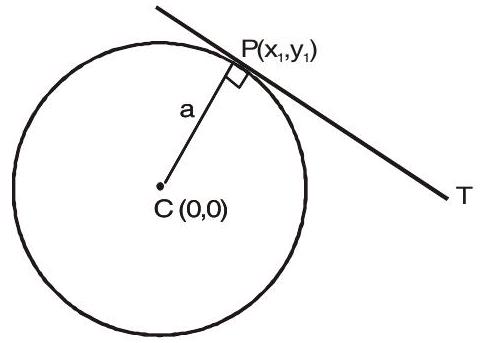
Point form
To find the equation of normal to the circle
Since we know that normal passes through the centre of a circle. So we get two points on normal using two point form of a line we get the equation of normal as
or
To find normal at
then according to determinant
Write first two rows as
Then normal at
If equation of circle is
here
If equation of circle is
here
Then
Chord of contact
Let the equation of circle be
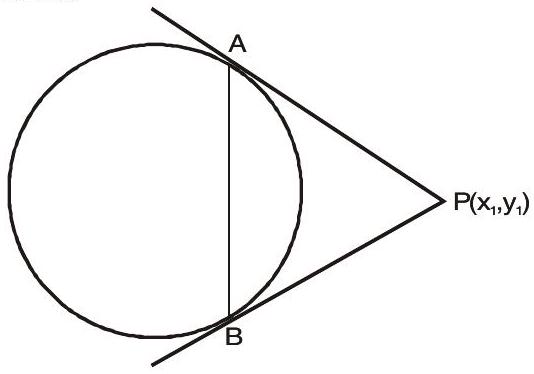
Equation of the chord bisected at a given point
Let the equation of circle be
Equation of chord
If the equation of circle be
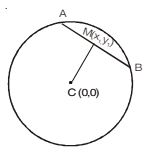
Director circle and its equation
The locus of the point of intersection of two perpendicular tangents to a given circle is known as its director circle.
Equation of Director Circle
Let
Squaring we get
or
Intersection of two circles, common Tangents to two circles
Let the two circles be
with centres
Different cases of intersection of two circles
Case I When
ie., distance between the centre is greater than sum of the radii.
In this case there are 4 common tangents can be drawn.
Two direct common tangents (circles lies on the same side of the tangent)
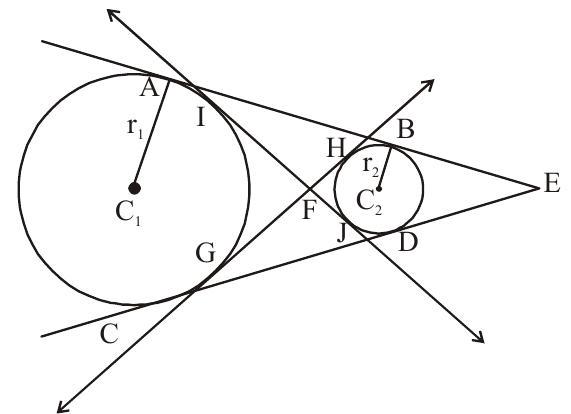
Two indirect (Transverse) common tangents (circles lies opposite side of the tangent) GFH & IFJ.
Note that centres of two circles and point of intersection of tangents are collinear also
To find the equations of common tangents.
Let us assume equation of tangent of any circle in slope form be
Points
Substitnde the values of
Case II When
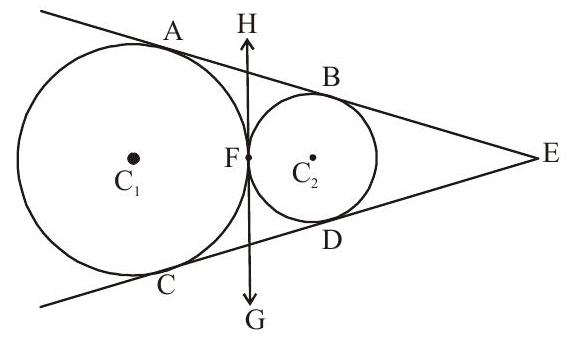
ie., distance between the centre is equal to sum of the radii.
In this case there are 3 common tangents 2 direct common tangent and one transverse common tangent
The equation of tangent at point
Coordinate of
Case III When
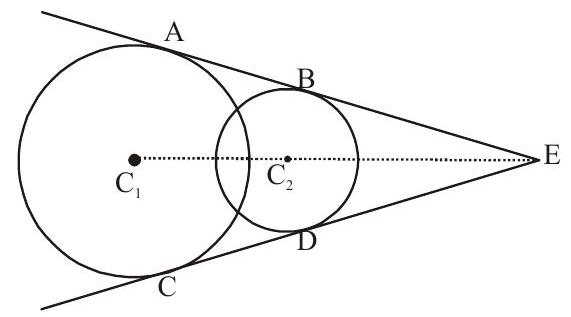
ie,. Distance between the centre is less than sum of the radii.
In this case only two direct common tangents are possible.
Case IV When
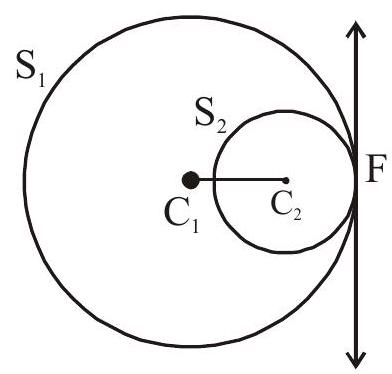
ie., distance between the centre is equal to difference of the radii.
Then the two circles touch each other internally.
In this case only one direct common tangent is possible.
Equation of common tangent is
Case V When
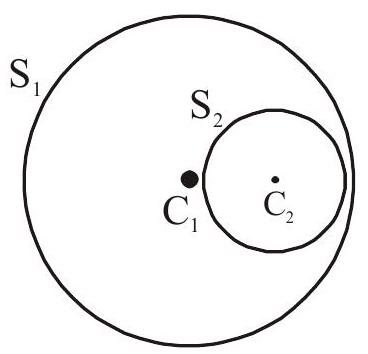
ie., distance between the centre is less than the difference of the radii. Then one circle contains the other
In this case there is no real Common tangents.
Length of direct common tangent if
length of direct common tangent
length of transverse common tangent
Where
Common Chord of two circles
The common chord joining the point of intersection of two circles is called their common chord .IfS
Let the equations of circles are
Then equation of common chord

Length of common chord is
Note :
(i) Common chord
(ii) Circle on the common chord a diameter then centre of the circle passing through A and B lie on the common chord of the two circle
(iii) If the length of common chord is zero then the two circles touch each other and the common chord be comes the common tangent to the two circles at the point of contact.
Angle of intersection of two circles
Let the two circles
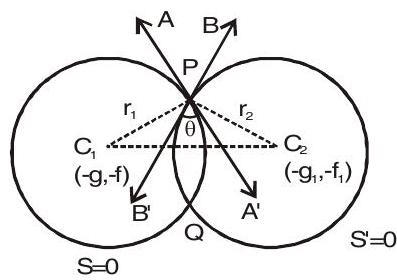
Let
ie.
Hence
Now in
If the angle between the circles is 90 ie.,
It is a condition for two circles to be orthogonal
Radical axis
The radical axis of two circles is the locus of a point which moves such that the lengths of the tangents drawn from it to the two circles are equal.

Consider
Let
or
Equation of radical axis is
Properties of radical axis
1. Radical axis is perpendicular to the line joining the centres of the given circles.
(Slope of radical axis)
2. The radical axis bisects common tangents of two circles
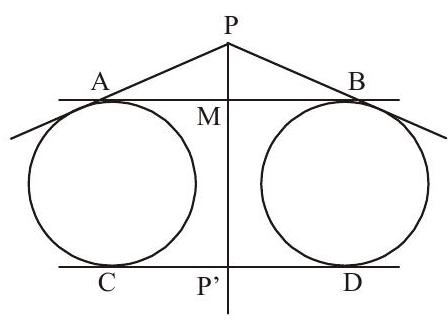
As
Hence radical axis bisects the common tangents
3. If two circles cut a third circle orthogonally, then the radical axis of the two circles will pass through the centre of the third circle, or the locus of the centre of a circle cutting two given circles orthogonally is the radical axis of the given two circles
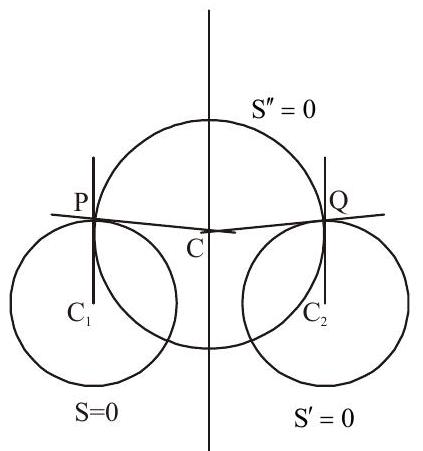
Hence
4. The position of the radical axis of the two circles geometrically

Radical Centre
The radical axes of three circles, taken in pairs, meet in a point, which is called their radical centre.
Let the three circles be
OL, OM, ON be radical axes of the pair sets of circles
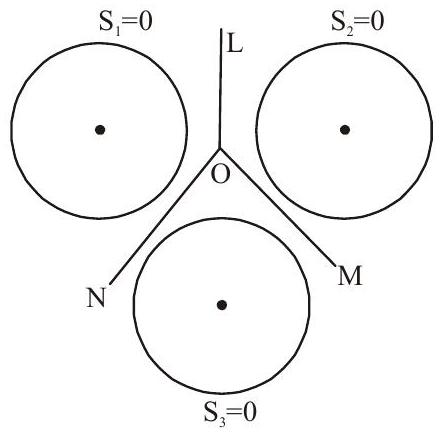
Equations of OL,
Family of lines passes through point of intersection of lines
If 
3. The radical centre of three given circles will be the centre of a fourth circle which cuts all the three circles orthogonally. and the radius of the fourth circle is the length of tangent drawn from radical centre of the three given circles to any of these circles
Co - Axial System of Circles
A system of circles or family of circles, every pair of which have the same radical axis are called co-axial circles
1. The equation of family of co-axial circles when the equation of radical axis and one circle are given

Then equation of co-axial circle is
2. The equation of co-axial system of a circles where the equation of any two circles of the system are
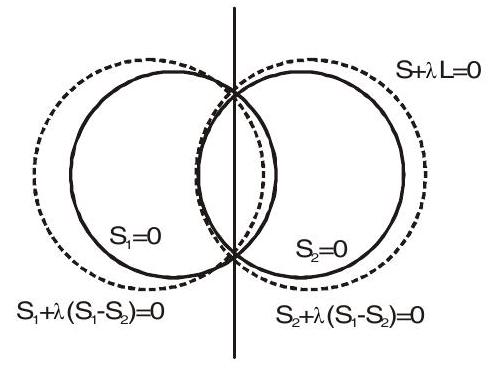
respectively is
and
3. The equation of a system of co-axial circles in the simplest form is
The common radical axis is the
The equation of system of co-axial circles in the simplest form is
The common radical axis is the 
1. Find the equation of tangent to the circle
Show Answer
Solution:
Equation of tangent of
2. Find the equations of the tangents to the circle
Show Answer
Solution:
Since tangents make an angle of
radius of circle
we know equation of tangent to a circle
or
3. Show that the line
Show Answer
Solution:
Since the line
Equation of tangent to a circle at
4. Find the equation of the normal to the circle
Show Answer
Solution:
Equation of normal at
Slope of this equation is
Slope of
Since given that normal is parallel to
It is the equation of normal
5. Show that the line
Show Answer
Solution:
Centre and radius of circle
is
If the distance of a line
Let point of contact be
and given line
(1) and (2) are idenfical then comparing (1) and (2) we get
Solving these two equations of
6. The angle between a pair of tangents from a point
Show Answer
Solution:
Let the coordinate of
circle is
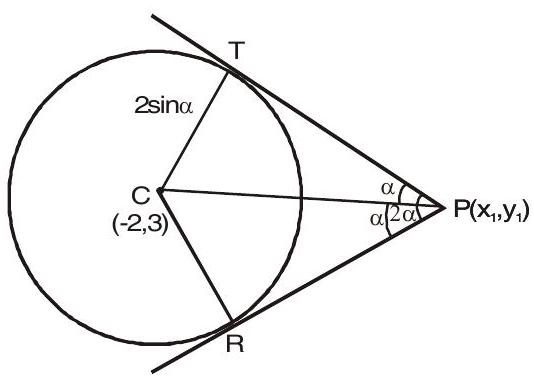
Distance of
In
or
Squaring
7. If the length of tangent from
Show Answer
Solution:
According to the question
Squaring both side
Divide by 3 we get
8. The chord of contact of tangents drawn from a point on the circle
Show Answer
Solution:
Let
It is a tangent to the circle
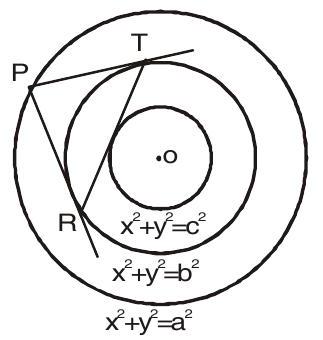
EXERCISE
1. The points
(a) 0
(b) 1
(c) 2
(d) None of these
Show Answer
Answer: b2. If the line
(a) a
(b)
(c)
(d)
Show Answer
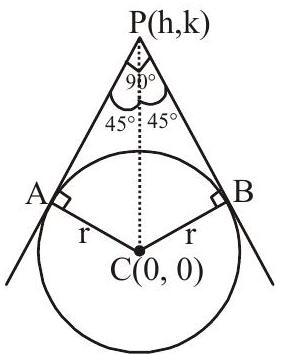
Answer: b3. Equation of incircle of equilateral triangle
(a)
(b)
(c)
(d) None of these
Show Answer
Answer: a4. A variable circle having radius ’
(a)
(b)
(c)
(d)
Show Answer
Answer: a5. The locus of the centre of the circle which cuts a chord of length 2 a from the positive
(a)
(b)
(c)
(d)
Show Answer
Answer: c6. The number of circle having radius 5 and passing through the points
(a) one
(b) two
(c) four
(d) infinite
Show Answer
Answer: b7. The equation of the smallest circle passing through the intersection of the line
(a)
(b)
(c)
(d) None of these
Show Answer
Answer: b8. The number of the points on the circle
(a) 1
(b) 2
(c) 3
(d) None of these
Show Answer
Answer: d9. The locus of the mid-point of a chord of the circle
(a)
(b)
(c)
(d)
Show Answer
Answer: b10. The area of the triangle formed by joining the origin to the points of intersection of the line
(a) 3
(b) 4
(c) 5
(d) 6
Show Answer
Answer: c11. If
(a) 11
(b) -11
(c) 24
(d) None of these
Show Answer
Answer: b12. A variable circle passes through the fixed point A(p.q) and touches
(a)
(b)
(c)
(d)
Show Answer
Answer: a13. If the lines
(a)
(b)
(c)
(d)
Show Answer
Answer: a14. The lines
(a)
(b)
(c)
(d)
Show Answer
Answer: c15. The equation of circle which passes through the origin and cuts off intercepts 5 and 6 from the positive parts of the axes respectively, is
(a)
(b)
(c)
(d)










