TRIGONOMETRY TRIANGLES - 3 (Properties of Triangles - Problem Solving)
Properties and Solutions of Triangles
Notation :
Vertices A,B,C
Sides $\mathrm{a}, \mathrm{b}, \mathrm{c}$
Centroid G (Point of intersection of medians )
Orthocentre O (Point of intersection of altitudes)
Circumcentre S (Point of intersection of perpendicular bisectors of the sides)
Incentre I (Point of intersection of internal bisectors of the angles)
Excentres $\mathrm{I} _{1}, \mathrm{I} _{2}, \mathrm{I} _{3}$ (Point of intersection of internal bisector of an angle & external bisectors of the other two angles).
Circumradius $\mathrm{R}$ (radius of circle with centre $\mathrm{S}$ and passing through the vertices)
Inradius $r$ (radius of circle with centre I and touching the sides)
Exradii $\mathrm{r} _{1}, \mathrm{r} _{2}, \mathrm{r} _{3}$ ( radii of circles with centres $\mathrm{I} _{1}, \mathrm{I} _{2}, \mathrm{I} _{3}$ respectively and touching the sides)
Semiperimeter $\mathrm{s}=\frac{\mathrm{a}+\mathrm{b}+\mathrm{c}}{2}$
Area of triangle $\Delta$
Concepts and Formula
1. Sine law
In any triangle $A B C, \frac{a}{\sin A}=\frac{b}{\sin B}=\frac{c}{\sin C}$
2. Cosine law
In any triangle $A B C$
-
$\quad \cos \mathrm{A}=\frac{\mathrm{b}^{2}+\mathrm{c}^{2}-\mathrm{a}^{2}}{2 \mathrm{bc}}$ or $\mathrm{a}^{2}=\mathrm{b}^{2}+\mathrm{c}^{2}-2 \mathrm{bc} \cos \mathrm{A}$
-
$\quad \cos \mathrm{B}=\frac{\mathrm{c}^{2}+\mathrm{a}^{2}-\mathrm{b}^{2}}{2 \mathrm{ac}}$ or $\mathrm{b}^{2}=\mathrm{c}^{2}+\mathrm{a}^{2}-2 \mathrm{ca} \cos \mathrm{B}$
-
$\quad \cos \mathrm{C}=\frac{\mathrm{a}^{2}+\mathrm{b}^{2}-\mathrm{c}^{2}}{2 \mathrm{ab}}$ or $\mathrm{c}^{2}=\mathrm{a}^{2}+\mathrm{b}^{2}-2 \mathrm{ab} \cos \mathrm{C}$
3. Projection formula
-
$ \mathrm{a}=\mathrm{b} \cos \mathrm{C}+\mathrm{c} \cos \mathrm{B}$
-
$\mathrm{b}=\cos \mathrm{A}+\mathrm{a} \cos \mathrm{C}$
-
$ \mathrm{c}=\operatorname{acos} \mathrm{B}+\mathrm{b} \cos \mathrm{A}$
4. Napier’s analogy (Tangent rule)
-
$\tan \frac{\mathrm{B}-\mathrm{C}}{2}=\frac{\mathrm{b}-\mathrm{c}}{\mathrm{b}+\mathrm{c}} \cot \frac{\mathrm{A}}{2}$
-
$ \tan \frac{\mathrm{C}-\mathrm{A}}{2}=\frac{\mathrm{c}-\mathrm{a}}{\mathrm{c}+\mathrm{a}} \cot \frac{\mathrm{B}}{2}$
-
$\tan \frac{A-B}{2}=\frac{a-b}{a+b} \cot \frac{C}{2}$
5. Auxiliary formulae
(Trigonometrical ratios of half angles of a triangle)
-
$ \sin \frac{\mathrm{A}}{2}=\sqrt{\frac{(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}{\mathrm{bc}}} ; \sin \frac{\mathrm{B}}{2}=\sqrt{\frac{(\mathrm{s}-\mathrm{c})(\mathrm{s}-\mathrm{a})}{\mathrm{ca}}} ; \sin \frac{\mathrm{C}}{2}=\sqrt{\frac{(\mathrm{s}-\mathrm{a})(\mathrm{s}-\mathrm{b})}{\mathrm{ab}}} ;$
-
$\quad \cos \frac{\mathrm{A}}{2}=\sqrt{\frac{\mathrm{s}(\mathrm{s}-\mathrm{a})}{\mathrm{bc}}} ; \cos \frac{\mathrm{B}}{2}=\sqrt{\frac{\mathrm{s}(\mathrm{s}-\mathrm{b})}{\mathrm{ca}}} ; \cos \frac{\mathrm{C}}{2}=\sqrt{\frac{\mathrm{s}(\mathrm{s}-\mathrm{c})}{\mathrm{ab}}} ;$
-
$\tan \frac{\mathrm{A}}{2}=\sqrt{\frac{(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}{\mathrm{s}(\mathrm{s}-\mathrm{a})}} ; \tan \frac{\mathrm{B}}{2}=\sqrt{\frac{(\mathrm{s}-\mathrm{c})(\mathrm{s}-\mathrm{a})}{\mathrm{s}(\mathrm{s}-\mathrm{b})}} ; \tan \frac{\mathrm{C}}{2}=\sqrt{\frac{(\mathrm{s}-\mathrm{a})(\mathrm{s}-\mathrm{b})}{\mathrm{s}(\mathrm{s}-\mathrm{c})}} ;$
$\quad$ $\left(=\frac{\Delta}{\mathrm{s}(\mathrm{s}-\mathrm{a})}\right) \quad\left(=\frac{\Delta}{\mathrm{s}(\mathrm{s}-\mathrm{b})}\right) \quad\left(=\frac{\Delta}{\mathrm{s}(\mathrm{s}-\mathrm{c})}\right)$
6. Area of Triangle
-
Area of triangle $\mathrm{ABC} _{\Delta}=\sqrt{\mathrm{s}(\mathrm{s}-\mathrm{a})(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}$ (Hero’s formula)
-
$\Delta=\frac{1}{2} \mathrm{absin} \mathrm{C}=\frac{1}{2} \mathrm{bcsin} \mathrm{A}=\frac{1}{2} \mathrm{casin} \mathrm{B}$
-
$\Delta=\frac{1}{2} \frac{\mathrm{a}^{2} \sin \mathrm{B} \sin \mathrm{C}}{\sin (\mathrm{B}+\mathrm{C})}=\frac{1}{2} \frac{\mathrm{b}^{2} \sin \mathrm{C} \sin \mathrm{A}}{\sin (\mathrm{C}+\mathrm{A})}=\frac{1}{2} \frac{\mathrm{c}^{2} \sin \mathrm{A} \sin \mathrm{B}}{\sin (\mathrm{A}+\mathrm{B})}$
-
$\sin \mathrm{A}=\frac{2 \Delta}{\mathrm{bc}}=\frac{2}{\mathrm{bc}} \sqrt{\mathrm{s}(\mathrm{s}-\mathrm{a})(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}$
-
$ \sin \mathrm{B}=\frac{2 \Delta}{\mathrm{ca}}=\frac{2}{\mathrm{ca}} \sqrt{\mathrm{s}(\mathrm{s}-\mathrm{a})(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}$
-
$ \sin \mathrm{C}=\frac{2 \Delta}{\mathrm{ab}}=\frac{2}{\mathrm{ab}} \sqrt{\mathrm{s}(\mathrm{s}-\mathrm{a})(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}$
$\quad$ Also $\frac{\sin A}{a}=\frac{\sin B}{b}=\frac{\sin C}{c}=\frac{2 \Delta}{a b c}$
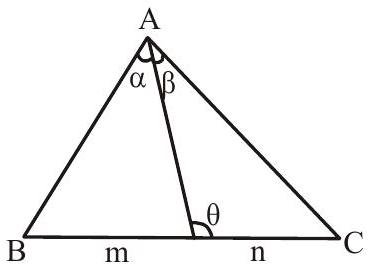
7. $m-n$ Rule
In any triangle,
$(\mathrm{m}+\mathrm{n}) \cot \theta=\mathrm{m} \cot \alpha-\mathrm{n} \cot \beta$
$ =n \cot B-m \cot C $
8. Circumcircle of a triangle
Circle passing through the angular point of a $\triangle \mathrm{ABC}$ is called its circumcircle. Its radius is denoted by R. The circum centre may lie within, outside or upon one of the sides of the triangle. In a right angled triangle the circum centre is the mid-point of hypotenuse.
-
$\mathrm{R}=\frac{\mathrm{a}}{2 \sin \mathrm{A}}=\frac{\mathrm{b}}{2 \sin \mathrm{B}}=\frac{\mathrm{c}}{2 \sin \mathrm{C}}$
-
$\mathrm{R}=\frac{\mathrm{abc}}{4 \Delta}$
9. Incircle of a triangle
The circle which touches the sides is called inscribed circle or incircle. Its radius is denoted by r.
-
$\mathrm{r}=\frac{\Delta}{\mathrm{s}}$
-
$\mathrm{r}=(\mathrm{s}-\mathrm{a}) \tan \frac{\mathrm{A}}{2}=(\mathrm{s}-\mathrm{b}) \tan \frac{\mathrm{B}}{2}=(\mathrm{s}-\mathrm{c}) \tan \frac{\mathrm{C}}{2}$
-
$\mathrm{r}=\frac{\mathrm{a} \sin \frac{\mathrm{B}}{2} \sin \frac{\mathrm{C}}{2}}{\cos \frac{\mathrm{A}}{2}}=\frac{\mathrm{b} \sin \frac{\mathrm{A}}{2} \sin \frac{\mathrm{C}}{2}}{\cos \frac{\mathrm{B}}{2}}=\frac{\mathrm{c} \sin \frac{\mathrm{B}}{2} \sin \frac{\mathrm{A}}{2}}{\cos \frac{\mathrm{C}}{2}}$
-
$\mathrm{r}=4 \mathrm{R} \sin \frac{\mathrm{A}}{2} \sin \frac{\mathrm{B}}{2} \sin \frac{\mathrm{C}}{2}$
10. Escribed circles of a triangle.
The circle which touches side $B C$ and two sides $A B \& A C$ produced of $\triangle A B C$ is called escribed circle opposite to the angle A. Its radius is denoted by $r _{1}$. Similarly $r _{2} \& r _{3}$ denote the radii of escribed circles opposite to angles B & C.
-
$\mathrm{r} _{1}=\frac{\Delta}{\mathrm{s}-\mathrm{a}} ; \mathrm{r} _{2}=\frac{\Delta}{\mathrm{s}-\mathrm{b}} ; \mathrm{r} _{3}=\frac{\Delta}{\mathrm{s}-\mathrm{c}}$
-
$\mathrm{r} _{1}=\mathrm{s} \tan \frac{\mathrm{A}}{2} ; \mathrm{r} _{2}=\mathrm{s} \tan \frac{\mathrm{B}}{2} ; \mathrm{r} _{3}=\mathrm{s} \tan \frac{\mathrm{C}}{2}$
-
$\mathrm{r} _{1}=\frac{\mathrm{a} \cos \frac{\mathrm{B}}{2} \cos \frac{\mathrm{C}}{2}}{\cos \frac{\mathrm{A}}{2}} ; \mathrm{r} _{2}=\frac{\mathrm{b} \cos \frac{\mathrm{A}}{2} \cos \frac{\mathrm{C}}{2}}{\cos \frac{\mathrm{B}}{2}} \quad \mathrm{r} _{3}=\frac{\mathrm{c} \cos \frac{\mathrm{B}}{2} \cos \frac{\mathrm{A}}{2}}{\cos \frac{\mathrm{C}}{2}}$
-
$r _{1}=4 R \sin \frac{A}{2} \cos \frac{B}{2} \cos \frac{C}{2} ; r _{2}=4 R \cos \frac{A}{2} \sin \frac{B}{2} \cos \frac{C}{2} ; r _{3}=4 R \cos \frac{A}{2} \cos \frac{B}{2} \sin \frac{C}{2}$
11. Orthocentre and Pedal triangle
-
The triangle MNP formed by joining the feet of the altitudes is called the pedal triangle
-
The distance of orthocentre $\mathrm{O}$ from vertices $\mathrm{A}, \mathrm{B}, \mathrm{C}$ are $2 \mathrm{R} \cos \mathrm{A}, 2 \mathrm{R} \cos \mathrm{B}$ and $2 \mathrm{R} \cos \mathrm{C}$
-
Distance of $\mathrm{O}$ from sides are $2 \mathrm{R} \cos \mathrm{B} \cos \mathrm{C}, 2 \mathrm{R} \cos \mathrm{C} \cos \mathrm{A}$ and $2 \mathrm{R} \cos \mathrm{A} \cos \mathrm{B}$.
-
$\operatorname{In} _{\triangle} \mathrm{MNP}$,
$\quad$ $ \begin{array}{ll} \angle \mathrm{M}=\pi-2 \mathrm{C} ; & \mathrm{MN}=\operatorname{acos} \mathrm{A} \\ \angle \mathrm{N}=\pi-2 \mathrm{~B} ; & \mathrm{NP}=\cos \mathrm{C} \\ \angle \mathrm{P}=\pi-2 \mathrm{~A} ; & \mathrm{MP}=\mathrm{b} \cos \mathrm{B} \end{array} $
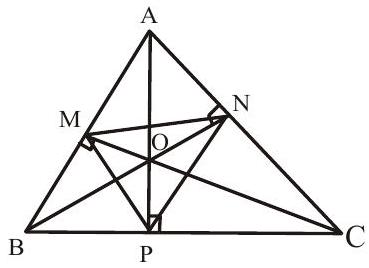
$\quad$i.e. sides of pedal triangles are a $\cos \mathrm{A}(\mathrm{R} \sin 2 \mathrm{~A})$, $\mathrm{b} \cos \mathrm{B}(\mathrm{R} \sin 2 \mathrm{~B})$ and $\cos \mathrm{C}(\mathrm{R} \sin 2 \mathrm{C})$.
- Circumradii of the triangles $\mathrm{OBC}, \mathrm{OCA} \& \mathrm{OAB}$ are equal.
12. Note :
-
Orthocentre of $\triangle \mathrm{ABC}$ is the incentre of pedal triangle MNP.
-
Incentre I of $\triangle \mathrm{ABC}$ is the orthocentre of $\Delta \mathrm{I} _{1}, \mathrm{I} _{2} \mathrm{I} _{3}$.
-
Centroid of $\triangle \mathrm{ABC}$ lies on the line joining the circumcentre to the orthocentre and divides it in the ratio $1: 2$
-
Circumcentre of the pedal triangle bisects the line joining the circumcentre of the triangle to the orthocentre.
13. Excentral Triangle.
The triangle formed by joining the three excentres $\mathrm{I} _{1}, \mathrm{I} _{2}, \& \mathrm{I} _{3}$ of $\triangle \mathrm{ABC}$ is called the excentral or excentric triangle.
Here $\mathrm{AI} _{1} \perp \mathrm{I} _{2} \mathrm{I} _{3}$
$\mathrm{BI}_ 2 \perp \mathrm{I}_ 1 \mathrm{I}_3$
$\& \quad \mathrm{CI} _{3} \perp \mathrm{I} _{1} \mathrm{I} _{2}$
So clearly $\Delta \mathrm{ABC}$ is pedal triangle of excentral triangle $\Delta \mathrm{I} _{1} \mathrm{I} _{2} \mathrm{I} _{3}$
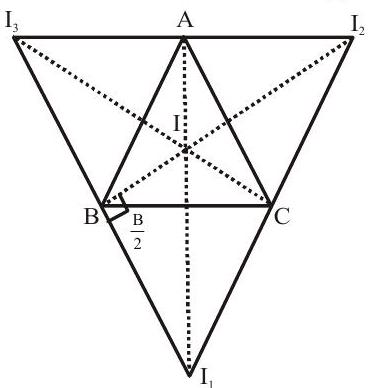
-
Sides of excentral trangle are $4 R \cos \frac{A}{2}, 4 R \cos \frac{B}{2}$ and $4 R \cos \frac{C}{2}$ and its angles are $\frac{\pi}{2}-\frac{\mathrm{A}}{2}, \frac{\pi}{2}-\frac{\mathrm{B}}{2}, \frac{\pi}{2}-\frac{\mathrm{C}}{2}$
-
$\mathrm{II} _{1}=4 \mathrm{R} \sin \frac{\mathrm{A}}{2} ; \mathrm{II} _{2}=4 \mathrm{R} \sin \frac{\mathrm{B}}{2} ; \mathrm{II} _{3}=4 \mathrm{R} \sin \frac{\mathrm{C}}{2}$
14. Nine point circle
Circle circumscribing the pedal triangle of a given triangle bisects the sides of the given triangle and also the line joining the vertices of the given triangle to the orthocentre of the given triangle. This circle is known as nine point circle.
i.e. Nine point circle passes through the mid point of the sides, feet of the perpendiculars and the mid points of the line joining the orthocentre to the angular points.
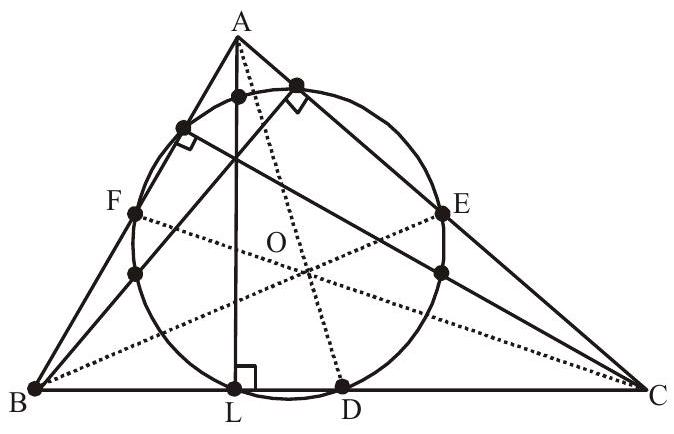
15. Distance between special points
- Distance between excentre and circumcentre
$\quad\mathrm{OI} _{1}=\mathrm{R} \sqrt{1+8 \sin \frac{\mathrm{A}}{2} \cos \frac{\mathrm{B}}{2} \cos \frac{\mathrm{C}}{2}}$
$\quad\mathrm{OI} _{2}=\mathrm{R} \sqrt{1+8 \cos \frac{\mathrm{A}}{2} \sin \frac{\mathrm{B}}{2} \cos \frac{\mathrm{C}}{2}}$
$\quad\mathrm{OI} _{3}=\mathrm{R} \sqrt{1+8 \cos \frac{\mathrm{A}}{2} \cos \frac{\mathrm{B}}{2} \sin \frac{\mathrm{C}}{2}}$
-
Distance between cirumcentre & orthocentre is $\sqrt{1-8 \cos A \cos B \cos C}$
-
Distance between cirumcentre & incentre is $\sqrt{\mathrm{R}^{2}-2 \mathrm{Rr}}$
-
Distance between incentre & orthocentre is $\sqrt{2 r^{2}-4 R^{2}} \cos A \cos B \cos C$
-
Perimeter $(\mathrm{P})$ and area $(\mathrm{A})$ of a regular polygon of $n$ sides inscribed in a circle of radius $r$ are given by $\mathrm{P}=2 \mathrm{nrsin} \frac{\pi}{\mathrm{n}}$ and $\mathrm{A}=\frac{1}{2} \mathrm{nr}^{2} \sin \frac{2 \pi}{\mathrm{n}}$.
-
Perimeter $(\mathrm{P})$ and area $(\mathrm{A})$ of a regular polygon of $n$ sides circumscribed about a given circle of radius $r$ are given by $P=2 n \tan \frac{\pi}{n}$ and $A=\frac{1}{2} n^{2} \tan \frac{2 \pi}{n}$
16. Length of medians (Apollonious rule)
$\mathrm{m} _{1}^{2}=\frac{\mathrm{b}^{2}+\mathrm{c}^{2}}{2}-\frac{\mathrm{a}^{2}}{4}$
$\mathrm{m} _{2}^{2}=\frac{\mathrm{c}^{2}+\mathrm{a}^{2}}{2}-\frac{\mathrm{b}^{2}}{4}$
$\mathrm{m} _{3}^{2}=\frac{\mathrm{a}^{2}+\mathrm{b}^{2}}{2}-\frac{\mathrm{c}^{2}}{4}$
Also $\mathrm{m} _{1}{ }^{2}+\mathrm{m} _{2}{ }^{2}+\mathrm{m} _{3}{ }^{2}=\frac{3}{4}\left(\mathrm{a}^{2}+\mathrm{b}^{2}+\mathrm{c}^{2}\right)$
If $\mathrm{A}=90^{\circ}$, then $\mathrm{m} _{2}{ }^{2}+\mathrm{m} _{3}{ }^{2}=5 \mathrm{~m} _{1}{ }^{2}$
Area $=\frac{4}{3} \sqrt{m\left(m-m _{1}\right)\left(m-m _{2}\right)\left(m-m _{3}\right)}$ where $2 m=m _{1}+m _{2}+m _{3}$.
17. Altitudes
$\mathrm{h} _{1}=\frac{2 \Delta}{\mathrm{a}}, \mathrm{h} _{2}=\frac{2 \Delta}{\mathrm{b}}, \mathrm{h} _{3}=\frac{2 \Delta}{\mathrm{c}}$,
Area is given by $\frac{1}{\Delta}=4 \sqrt{\frac{1}{\mathrm{~h}}\left(\frac{1}{\mathrm{~h}}-\frac{1}{\mathrm{~h} _{1}}\right)\left(\frac{1}{\mathrm{~h}}-\frac{1}{\mathrm{~h} _{2}}\right)\left(\frac{1}{\mathrm{~h}}-\frac{1}{\mathrm{~h} _{3}}\right)}$ where $\frac{2}{\mathrm{~h}}=\frac{1}{\mathrm{~h} _{1}}+\frac{1}{\mathrm{~h} _{2}}+\frac{1}{\mathrm{~h} _{3}}$
18. Length of internal bisectors of angles
$A, B, C$ are given by $\frac{2 b c}{b+c} \cos \frac{A}{2} ; \frac{2 c a}{c+a} \cos \frac{B}{2}$; $\frac{2 a b}{a+b} \cos \frac{C}{2}$ respectively
19. Some useful results.
-
$\mathrm{r} _{1}+\mathrm{r} _{2}=4 \mathrm{R} \cos ^{2} \frac{\mathrm{C}}{2}$
-
$r _{3}-r=4 R \sin ^{2} \frac{C}{2}$.
-
$\mathrm{r} _{1}+\mathrm{r} _{2}+\mathrm{r} _{3}-\mathrm{r}=4 \mathrm{R}$
-
$\operatorname{rr} _{1} \mathrm{r} _{2} \mathrm{r} _{3}=\Delta^{2}$
-
$\frac{1}{\mathrm{r} _{1}}+\frac{1}{\mathrm{r} _{2}}+\frac{1}{\mathrm{r} _{3}}=\frac{1}{\mathrm{r}}$
-
$\frac{1}{\mathrm{r}^{2}}+\frac{1}{\mathrm{r} _{1}{ }^{2}}+\frac{1}{\mathrm{r} _{2}{ }^{2}}+\frac{1}{\mathrm{r} _{3}{ }^{2}}=\frac{\mathrm{a}^{2}+\mathrm{b}^{2}+\mathrm{c}^{2}}{\Delta^{2}}$
-
$\quad s=\sqrt{r _{1} r _{2}+r _{2} r _{3}+r _{3} r _{1}}=\sqrt{\sum r _{1} r _{2}}$
-
$\Delta=\frac{\mathrm{r} _{1} \mathrm{r} _{2} \mathrm{r} _{3}}{\mathrm{~s}}=\frac{\mathrm{r} _{1} \mathrm{r} _{2} \mathrm{r} _{3}}{\sqrt{\sum \mathrm{r} _{1} \mathrm{r} _{2}}}$
-
$r=\frac{r _{1} r _{2} r _{3}}{\sum r _{1} r _{2}}$
-
$\mathrm{R}=\frac{\left(\mathrm{r} _{1}+\mathrm{r} _{2}\right)\left(\mathrm{r} _{2}+\mathrm{r} _{3}\right)\left(\mathrm{r} _{3}+\mathrm{r} _{1}\right)}{4 \sum \mathrm{r} _{1} \mathrm{r} _{2}}$
$\quad a=\frac{\mathrm{r} _{1}\left(\mathrm{r} _{2}+\mathrm{r} _{3}\right)}{\sqrt{\sum \mathrm{r} _{1} \mathrm{r} _{2}}} ; b=\frac{\mathrm{r} _{2}\left(\mathrm{r} _{3}+\mathrm{r} _{1}\right)}{\sqrt{\sum \mathrm{r} _{1} \mathrm{r} _{2}}} ; c=\frac{\mathrm{r} _{3}\left(\mathrm{r} _{3}+\mathrm{r} _{2}\right)}{\sqrt{\sum \mathrm{r} _{1} \mathrm{r} _{2}}}$
20. Solution of triangles
(a) Solution of a general triangle.
| Given | To find | Formulae used |
|---|---|---|
| a, b, c | A, B, C | $\bullet \quad$ Find $_{\Delta}=\sqrt{\mathrm{s}(\mathrm{s}-\mathrm{a})(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}$ |
| $\bullet \quad$ $\sin \mathrm{A}=\frac{2 \Delta}{\mathrm{bc}}, \sin \mathrm{B}=\frac{2 \Delta}{\mathrm{ca}}, \sin \mathrm{C}=\frac{2 \Delta}{\mathrm{ab}}$, | ||
| or $\tan \frac{\mathrm{A}}{2}=\frac{\Delta}{\mathrm{s}(\mathrm{s}-\mathrm{a})}$ etc. | ||
| or $\quad \cos A=\frac{b^2+c^2-a^2}{2 b c}$ etc. (if $\mathrm{a}, \mathrm{b}, \mathrm{c}$ are sides) |
||
| a, B, C | c, A, B | $\bullet \quad\frac{\mathrm{A}+\mathrm{B}}{2}=90^{\circ}-\frac{\mathrm{C}}{2} $ |
| $ \quad\&\tan \frac{\mathrm{A}-\mathrm{B}}{2}=\frac{\mathrm{a}-\mathrm{b}}{\mathrm{a}+\mathrm{b}} \cot \frac{\mathrm{C}}{2} $ | ||
| $ \bullet\quad \mathrm{c}=\frac{\mathrm{a} \sin \mathrm{C}}{\sin \mathrm{A}} \text { (sine rule) } $ | ||
| or $\mathrm{c}^2=\mathrm{a}^2+\mathrm{b}^2-2 \mathrm{ab\cos} \mathrm{C} \text { (cosine rule)} $ | ||
| a, A, B (or c, A, B) |
C, a, b | $\bullet \quad$$\mathrm{C}=180^{\circ}-(\mathrm{A}+\mathrm{B})$ |
| $\bullet \quad$ $\mathrm{b}=\frac{\mathrm{a} \sin \mathrm{B}}{\sin \mathrm{A}}, \mathrm{c}=\frac{\mathrm{a} \sin \mathrm{C}}{\sin \mathrm{A}}$ (sine rule) | ||
| (or $\mathrm{b}=\frac{\mathrm{c} \sin \mathrm{B}}{\sin \mathrm{C}}, \mathrm{a}$ $=\frac{\mathrm{c} \sin \mathrm{A}}{\sin \mathrm{C}}$ as the case may be) |
||
| a, b, A | c, B, C | $\bullet$ $\quad \sin B=\frac{b}{a} \sin A……..(i)$ |
| $\bullet$ $\quad\mathrm{C}=180^{\circ}-(\mathrm{A}+\mathrm{B})…….(ii)$ | ||
| $\bullet$$\quad\mathrm{c}=\frac{\mathrm{a} \sin \mathrm{C}}{\sin \mathrm{A}}……………….(iii)$ |

(b) Solution of a right angled triangle
Let $\angle \mathrm{C}=90^{\circ}$
| Given | To find | Formulae used |
|---|---|---|
| $\mathrm{a}, \mathrm{b}$ | $\mathrm{A}, \mathrm{B}, \mathrm{c}$ | $\bullet \mathrm{c}^{2}=\mathrm{a}^{2}+\mathrm{b}^{2}$ |
| (two sides) | $\bullet \tan ^{\mathrm{A}}=\frac{\mathrm{a}}{\mathrm{b}}\left(\right.$ or $\left.\tan \mathrm{B}=\frac{\mathrm{b}}{\mathrm{a}}\right)$ | |
| $\bullet \mathrm{B}=90^{\circ}-\mathrm{A}\left(\right.$ or $\left.\mathrm{A}=90^{\circ}-\mathrm{B}\right)$ | ||
| $\mathrm{c}, \mathrm{a}$ | $\mathrm{A}, \mathrm{B}, \mathrm{b}$ | $\bullet \mathrm{b}^{2}=\mathrm{c}^{2}-\mathrm{a}^{2}$ |
| (hypotenuse & | $\bullet \mathrm{SinA}=\frac{\mathrm{a}}{\mathrm{c}}$ | |
| one side) | $\bullet \mathrm{b}=\cos \mathrm{A}$ or $\mathrm{b}=\mathrm{a} \cot \mathrm{A}$ | |
| a, A | $\bullet \mathrm{B}=90^{\circ}-\mathrm{A}$ |
Solved examples
1. If in $\triangle \mathrm{ABC}, \mathrm{a}=6, \mathrm{~b}=3$ and $\cos (\mathrm{A}-\mathrm{B})=\frac{4}{5}$ then its area is
(a). 8
(b). 9
(c). 6
(d). None of these
Show Answer
Solution : Using Napier’s analogy,
$\tan \frac{A-B}{2}=\frac{a-b}{a+b} \cot \frac{C}{2}$ and $\tan \left(\frac{A-B}{2}\right)=\sqrt{\frac{1-\cos (A-B)}{1+\cos (A-B)}}$
$\Rightarrow \quad \frac{1}{3}=\frac{6-3}{6+3} \cot \frac{\mathrm{C}}{2}$ $\tan \left(\frac{\mathrm{A}-\mathrm{B}}{2}\right)=\sqrt{\frac{1-\frac{4}{5}}{1+\frac{4}{5}}}=\frac{1}{3}$
$\Rightarrow \quad \cot \frac{\mathrm{C}}{2}=1$
$\mathrm{C}=90^{\circ}$
$\therefore$ Area of $\Delta=\frac{1}{2} \mathrm{absinC}=\frac{1}{2} \cdot 6 \cdot 3 \cdot \sin 90^{\circ}=9$ square units
Answer: (b)
2. If the angles $\mathrm{A}, \mathrm{B}, \mathrm{C}$ are the solutions of the equation $\tan ^{3} \mathrm{x}-3 \mathrm{k} \cdot \tan ^{2} \mathrm{x}-3 \tan \mathrm{x}+\mathrm{k}=0$, then the $\triangle \mathrm{ABC}$ is
(a). isosceles
(b). equilateral
(c). acute angled
(d). None of these
Show Answer
Solution: $\tan \mathrm{A}, \tan \mathrm{B}, \tan \mathrm{C}$ are roots of the given equation
$\therefore \tan A+\tan B+\tan C=3 k, \tan A \tan B+\tan B \tan C+\tan C \tan A=-3$ and $\tan A \cdot \tan B \cdot \tan C=-k$
But $\tan A+\tan B+\tan C=\tan A \tan B \tan C$
$\Rightarrow 3 \mathrm{k}=-\mathrm{k} \Rightarrow 4 \mathrm{k}=0$ gives $\mathrm{k}=0$
$\therefore \tan \mathrm{Atan} \mathrm{B} \tan \mathrm{C}=0$
$\Rightarrow \tan \mathrm{A}=0$ or $\tan \mathrm{B}=0$ or $\tan \mathrm{C}=0$ which is not possible
Answer: (d)
3. In $\triangle \mathrm{ABC}$
$\sin ^{4} \mathrm{~A}+\sin ^{4} \mathrm{~B}+\sin ^{4} \mathrm{C}=\sin ^{2} \mathrm{~B} \sin ^{2} \mathrm{C}+2 \sin ^{2} \mathrm{C} \sin ^{2} \mathrm{~A}+2 \sin ^{2} \mathrm{~A} \sin ^{2} \mathrm{~B}$, then $\angle \mathrm{A}=$
(a). $\frac{\pi}{6}, \frac{5 \pi}{6}$
(b). $\frac{\pi}{6}, \frac{5 \pi}{3}$
(c). $\frac{5 \pi}{6}, \frac{\pi}{3}$
(d). None of these
Show Answer
Solution : Given equation is,
$\mathrm{a}^{4}+\mathrm{b}^{4}+\mathrm{c}^{4}=\mathrm{b}^{2} \mathrm{c}^{2}+2 \mathrm{c}^{2} \mathrm{a}^{2}+2 \mathrm{a}^{2} \mathrm{~b}^{2}$ (using sine rule)
$\Rightarrow a^{4}+b^{4}+c^{4}+2 b^{2} c^{2}-2 c^{2} a^{2}-2 a^{2} b^{2}=3 b^{2} c^{2}$.
$\left(\mathrm{b}^{2}+\mathrm{c}^{2}-\mathrm{a}^{2}\right)^{2}=3 \mathrm{~b}^{2} \mathrm{c}^{2}$
$\Rightarrow \frac{\left(\mathrm{b}^{2}+\mathrm{c}^{2}-\mathrm{a}^{2}\right)^{2}}{4 \mathrm{~b}^{2} \mathrm{c}^{2}}=\frac{3}{4}$
$\left(\frac{\mathrm{b}^{2}+\mathrm{c}^{2}-\mathrm{a}^{2}}{2 \mathrm{bc}}\right)^{2}=\left(\frac{\sqrt{3}}{2}\right)^{2}$
$\cos ^{2} \mathrm{~A}=\frac{3}{4} \Rightarrow \cos \mathrm{A}= \pm \frac{\sqrt{3}}{2}$
$\Rightarrow \mathrm{A}=\frac{\pi}{6}, \frac{5 \pi}{6}$
Answer: (a)
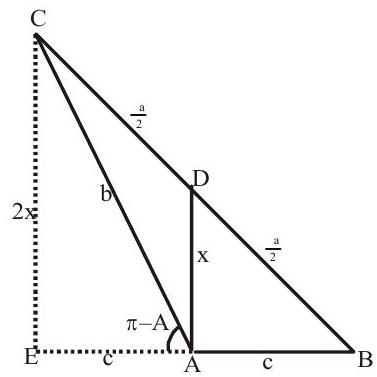
4. If the median of a triangle through $\mathrm{A}$ is perpendicular to $\mathrm{AB}$, then
(a). $2 \tan \mathrm{A}+\tan \mathrm{B}=0$
(b). $2 \tan \mathrm{A}-\tan \mathrm{B}=0$
(c). $\tan \mathrm{A}-2 \tan \mathrm{B}=0$
(d). $\tan \mathrm{A}+2 \tan \mathrm{B}=0$
Show Answer
Solution :
$ \begin{aligned} \tan \mathrm{A} & =-\tan (180-\mathrm{A}) \\ & =-2 \frac{\mathrm{x}}{\mathrm{c}} \\ & =-2\left(\frac{\mathrm{x}}{\mathrm{c}}\right) \\ & =-2 \tan \mathrm{B} \\ \tan \mathrm{A} & +2 \tan \mathrm{B}=0 \end{aligned} $
Answer: (d)
5. In $\triangle \mathrm{ABC}$, medians $\mathrm{AD}$ and $\mathrm{BE}$ are drawn. If $\mathrm{AD}=4, \angle \mathrm{DAB}=\frac{\pi}{6}$ and $\angle \mathrm{ABE}=\frac{\pi}{3}$, then the area of $\triangle \mathrm{ABC}$ is (in square units)
(a). $\frac{64}{3 \sqrt{3}}$
(b). $\frac{8}{3 \sqrt{3}}$
(c). $\frac{16}{3 \sqrt{3}}$
(d). $\frac{32}{3 \sqrt{3}}$
Show Answer
Solution :
$ \begin{aligned} & \text { In } \Delta \mathrm{ABG} \\ & \cos \frac{\pi}{6}=\frac{\mathrm{AG}}{\mathrm{AB}} \\ & \frac{\sqrt{3}}{2}=\frac{2}{3} \frac{\mathrm{AD}}{\mathrm{AB}}(\therefore \mathrm{AD}=4) \\ & \Rightarrow \frac{\sqrt{3}}{2}=\frac{2 \times 4}{3 \mathrm{AB}} \\ & \Rightarrow \mathrm{AB}=\frac{16}{3 \sqrt{3}} \end{aligned} $
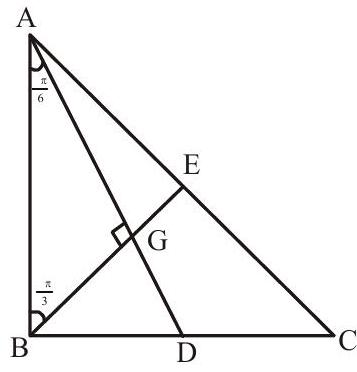
Area of ${ } _{\Delta} \mathrm{ABD}=\frac{1}{2} \mathrm{AB} \cdot \mathrm{AD} \cdot \sin \frac{\pi}{6}$
$ =\frac{1}{2} \cdot \frac{16}{3 \sqrt{3}} \cdot 4 \cdot \frac{1}{2}=\frac{16}{3 \sqrt{3}} \text { units }^{2} $
$\therefore$ Area of $\triangle \mathrm{ABC}=2$ area of $\triangle \mathrm{ABD}=\frac{32}{3 \sqrt{3}}$ units $^{2}$
Answer: (d)
6. In $\triangle \mathrm{ABC}, \mathrm{a}^{2}+\mathrm{b}^{2}+\mathrm{c}^{2}=\mathrm{ac}+\sqrt{3}$ ab, then the triangle is
(a). equilateral
(b). isosceles
(c). right angled
(d). None of these
Show Answer
Solution :
$\mathrm{a}^{2}+\mathrm{b}^{2}+\mathrm{c}^{2}-\mathrm{ac}-\sqrt{3} \mathrm{ab}=0$
$\left(\frac{a^{2}}{4}+c^{2}-a c\right)+\left(\frac{3 a^{2}}{4}+b^{2}-\sqrt{3} a b\right)=0$
$\Rightarrow\left(\frac{\mathrm{a}}{2}-\mathrm{c}\right)^{2}+\left(\frac{\sqrt{3} \mathrm{a}}{2}-\mathrm{b}\right)^{2}=0$
$\Rightarrow \frac{\mathrm{a}}{2}=\mathrm{c} \& \frac{\sqrt{3}}{2} \mathrm{a}=\mathrm{b}$ $\Rightarrow \mathrm{a}=2 \mathrm{c} \quad \& \mathrm{a}=\frac{2 \mathrm{~b}}{\sqrt{3}}$
$\mathrm{a}=2 \mathrm{c}=\frac{2 \mathrm{~b}}{\sqrt{3}}=\lambda$
$\therefore \mathrm{a}=\lambda, \mathrm{c}=\frac{\lambda}{2}, \mathrm{~b}=\frac{\sqrt{3} \lambda}{2}$
$\therefore \mathrm{a}^{2}=\mathrm{b}^{2}+\mathrm{c}^{2}$
$\therefore$ right angled
Answer: (c)
7. In $\triangle \mathrm{ABC}$, if $\cot \mathrm{A}+\cot \mathrm{B}+\cot \mathrm{C}=\sqrt{3}$, then show that the triangle is equilateral
Show Answer
Solution :
In $\triangle \mathrm{ABC}, \tan \mathrm{A}+\tan \mathrm{B}+\tan \mathrm{C}=\tan \mathrm{A} \tan B \tan \mathrm{C}$
$\Rightarrow \quad \cot B \cot \mathrm{C}+\cot C \cot \mathrm{A}+\cot B \cot \mathrm{A}=1………..(1)$
Let $\cot \mathrm{A}=\mathrm{x}, \cot \mathrm{B}=\mathrm{y}, \cot \mathrm{C}=\mathrm{z}$
given that $\mathrm{x}+\mathrm{y}+\mathrm{z}=\sqrt{3}$
Squaring,
$ \mathrm{x}^{2}+\mathrm{y}^{2}+\mathrm{z}^{2}+2 \mathrm{xy}+2 \mathrm{yz}+2 \mathrm{zx}=3 \times 1 $
$x^{2}+y^{2}+z^{2}+2 x y+2 y z+2 z x=3(x y+y z+z x)$ using $(1)$
i.e. $\quad x^{2}+y^{2}+z^{2}-x y-y z-z x=0$
$\Rightarrow \quad 2 x^{2}+2 y^{2}+2 z^{2}-2 x y-2 y z-2 z x=0$
$\Rightarrow \quad(\mathrm{x}-\mathrm{y})^{2}+(\mathrm{y}-\mathrm{z})^{2}+(\mathrm{z}-\mathrm{x})^{2}=0$
$\Rightarrow \quad x=y \& y=z \& z=x$
$\Rightarrow \quad \mathrm{x}=\mathrm{y}=\mathrm{z}$
$\Rightarrow \quad \cot \mathrm{A}=\cot \mathrm{B}=\cot \mathrm{C}$
$\Rightarrow \mathrm{A}=\mathrm{B}=\mathrm{C}$
$\Rightarrow \quad$ The triange is equilateral.
Practice questions
1. In $\triangle \mathrm{ABC}, \angle \mathrm{B}=\frac{\pi}{3}, \angle \mathrm{C}=\frac{\pi}{4}$, Let $\mathrm{D}$ divides $\mathrm{BC}$ internally in the ratio $1: 3$, then $\frac{\sin \angle \mathrm{BAD}}{\sin \angle \mathrm{CAD}}$ is equal to
(a). $\frac{1}{\sqrt{6}}$
(b). $\frac{1}{3}$
(c). $\frac{1}{\sqrt{3}}$
(d). $\sqrt{\frac{2}{3}}$
Show Answer
Answer: (a)2. If in $\triangle \mathrm{PQR}, \sin \mathrm{P}, \sin \mathrm{Q}, \sin \mathrm{R}$ are in A.P., then
(a). The altitudes are in A.P
(b). The medians are in G.P
(c). The altitudes are in H.P
(b). The medians are in A.P
Show Answer
Answer: (c)3. In ${ } _{\triangle} \mathrm{PQR}, \angle \mathrm{R}=\frac{\pi}{2}$, if $\tan \left(\frac{\mathrm{P}}{2}\right)$ and $\tan \left(\frac{\mathrm{Q}}{2}\right)$ are the roots of $\mathrm{ax}^{2}+\mathrm{bx}+\mathrm{c}=0(\mathrm{a} \neq 0)$, then
(a). $\mathrm{a}+\mathrm{b}=\mathrm{c}$
(b). $\mathrm{b}+\mathrm{c}=\mathrm{a}$
(c). $\mathrm{c}+\mathrm{a}=\mathrm{b}$
(d). $\mathrm{b}=\mathrm{c}$
Show Answer
Answer: (a)4. In $\triangle \mathrm{ABC}, 2 \mathrm{ac} \sin \frac{(\mathrm{A}-\mathrm{B}+\mathrm{C})}{2}=$
(a). $\mathrm{a}^{2}+\mathrm{b}^{2}-\mathrm{c}^{2}$
(b). $\mathrm{c}^{2}+\mathrm{a}^{2}-\mathrm{b}^{2}$
(c). $\mathrm{b}^{2}-\mathrm{c}^{2}-\mathrm{a}^{2}$
(d). $\mathrm{c}^{2}-\mathrm{a}^{2}-\mathrm{b}^{2}$
Show Answer
Answer: (b)5. In $\triangle \mathrm{ABC}$, let $\angle \mathrm{C}=\frac{\pi}{2}$. If $\mathrm{r}$ is the inradius and $\mathrm{R}$ is the circumradius of the triangle, then $2(r+R)$ is equal to
(a). $a+b$
(b). $\mathrm{b}+\mathrm{c}$
(c). $\mathrm{c}+\mathrm{a}$
(d). $\mathrm{a}+\mathrm{b}+\mathrm{c}$
Show Answer
Answer: (a)6. In radius of a circle which is inscribed in an isosceles triangle one of whose angle is $\frac{2 \pi}{3}$ is $\sqrt{3}$, then area of triangle is
(a). $4 \sqrt{3}$
(b). $12-7 \sqrt{3}$
(c). $12+7 \sqrt{3}$
(d). None of these
Show Answer
Answer: (c)7. If the angles $\mathrm{A}, \mathrm{B} \& \mathrm{C}$ of a triangle are in A.P and if $\mathrm{a}, \mathrm{b}$ and $\mathrm{c}$ denote the lengths of the sides opposite to $\mathrm{A}, \mathrm{B} \& \mathrm{C}$ respectively, then the value of the expression $\frac{\mathrm{a}}{\mathrm{c}} \sin 2 \mathrm{C}+\frac{\mathrm{c}}{\mathrm{a}} \sin 2 \mathrm{~A}$ is
(a). $\frac{1}{2}$
(b). $\frac{\sqrt{3}}{2}$
(c). $1$
(d). $\sqrt{3}$
Show Answer
Answer: (d)8. Read the following passage and answer the questions.
Consider the circle $\mathrm{x}^{2}+\mathrm{y}^{2}=9$ and the parabola $\mathrm{y}^{2}=8 \mathrm{x}$. They intersect at $P \& Q$ in the first and fourth quadrants, respectively. Tangents to the circle at $P \& Q$ intersect the $x$-axis at $R$ and tangents to the parabola at $\mathrm{P} \& \mathrm{Q}$ intersect the $\mathrm{x}$ axis at $\mathrm{S}$.
(i). The ratio of the areas of ${ } _{\Delta} \mathrm{PQS}$ and ${ } _{\Delta} \mathrm{PQR}$ is
(a). $1 : \sqrt{2}$
(b). $1 : 2$
(c). $1 : 40$
(d). $1 : 8$
Show Answer
Answer: (c)(ii). The radius of the circumcircle of the ${ } _{\Delta} \mathrm{PRS}$ is
(a). $5$
(b). $3 \sqrt{3}$
(c). $3 \sqrt{2}$
(d). $2 \sqrt{3}$
Show Answer
Answer: (b)(iii). The radius of the incircle of the triangle $\mathrm{PQR}$ is
(a). $4$
(b). $3$
(c). $\frac{8}{3}$
(d). $2$
Show Answer
Answer: (d)9. Internal bisector $\angle \mathrm{A}$ of $\triangle \mathrm{ABC}$ meets side $\mathrm{BC}$ at $\mathrm{D}$. A line drawn through $\mathrm{D}$ perpendicular to $\mathrm{AD}$ intersects the side $\mathrm{AC}$ at $\mathrm{E} \&$ side $\mathrm{AB}$ at $\mathrm{F}$. If $\mathrm{a}, \mathrm{b}, \mathrm{c}$ represent sides of $\triangle \mathrm{ABC}$, then
(a). $\mathrm{AE}$ is the $\mathrm{HM}$ of $\mathrm{b} \& \mathrm{c}$
(b). $\mathrm{AD}=\frac{2 \mathrm{bc}}{\mathrm{b}+\mathrm{c}} \cos \frac{\mathrm{A}}{2}$
(c). $\mathrm{EF}=\frac{4 \mathrm{bc}}{\mathrm{b}+\mathrm{c}} \sin \frac{\mathrm{A}}{2}$
(d). $\triangle \mathrm{AEF}$ is isosceles
Show Answer
Answer: (a, b, c, d)10. A straight line through the vertex $\mathrm{P}$ of a $\Delta \mathrm{PQR}$ intersects the side $\mathrm{QR}$ at the point $\mathrm{S}$ and the circumcircle of the triangle $\mathrm{PQR}$ at the point $\mathrm{T}$. If $\mathrm{S}$ is not the centre of the circumcircle, then
(a). $\frac{1}{\mathrm{PS}}+\frac{1}{\mathrm{ST}}<\frac{2}{\sqrt{\mathrm{QS} \times \mathrm{SR}}}$
(b). $\frac{1}{\mathrm{PS}}+\frac{1}{\mathrm{ST}}>\frac{2}{\sqrt{\mathrm{QS} \times \mathrm{SR}}}$
(c). $\frac{1}{\mathrm{PS}}+\frac{1}{\mathrm{ST}}<\frac{4}{\mathrm{QR}}$
(d). $\frac{1}{\mathrm{PS}}+\frac{1}{\mathrm{ST}}>\frac{4}{\mathrm{QR}}$
Show Answer
Answer: (b, d)11. In a $\triangle \mathrm{ABC}$ with fixed base $\mathrm{BC}$, the vertex $\mathrm{A}$ moves such that $\cos \mathrm{B}+\cos \mathrm{C}=4 \sin ^{2} \frac{\mathrm{A}}{2}$. If $\mathrm{a}, \mathrm{b}$ and $\mathrm{c}$ denote the lengths of the sides of the triangle opposite to the angles $\mathrm{A}, \mathrm{B}$ and $\mathrm{C}$ respectively, then
(a). $\mathrm{b}+\mathrm{c}=4 \mathrm{a}$
(b). $\mathrm{b}+\mathrm{c}=2 \mathrm{a}$
(c). locus of point $A$ is an ellipse
(d). locus of point $\mathrm{A}$ is a pair of straight line
Show Answer
Answer: (b, c)12. Match the following:
| Column I | Column II |
|---|---|
| (a). In $\triangle \mathrm{ABC}$, if $\frac{\cos \mathrm{A}}{\mathrm{a}}=\frac{\cos \mathrm{B}}{\mathrm{b}}=\frac{\cos \mathrm{C}}{\mathrm{c}}$ and the side $a=2$, then area of the triangle is | (p). $2 \sqrt{3}+\sqrt{6}$ |
| (b). In $\triangle A B C, a \geq b \geq c$, if $\frac{a^{3}+b^{3}+c^{3}}{\sin ^{3} A+\sin ^{3} B+\sin ^{3} C}=7$, then the maximum possible value of a is | (q). $\frac{1}{\sqrt{6}}$ |
| (c). Two sides of a triangle are given by the roots of the equation $x^{2}-2 \sqrt{3} x+2=0$. the angle between the sides is $\frac{\pi}{3}$.The perimeter of the triangle is | (r). $\sqrt[3]{7}$ |
| (s). $\sqrt{3}$ |
Show Answer
Answer: a $\rarr$ s; b $\rarr$ r; c$\rarr$ p13. In $\triangle \mathrm{ABC}$ if $\cos \mathrm{A}+\cos \mathrm{B}+\cos \mathrm{C}=\frac{7}{4}$, then $\frac{\mathrm{R}}{\mathrm{r}}=$
(a). $\frac{4}{3}$
(b). $\frac{3}{4}$
(c). $\frac{2}{3}$
(d). $\frac{3}{2}$
Show Answer
Answer: (a)14. In $\triangle \mathrm{ABC}$, sides a,b,c are in A.P and $\frac{2}{199}+\frac{2}{3 ! 7 !}+\frac{1}{5 ! 5 !}=\frac{8^{\mathrm{a}}}{(2 \mathrm{~b}) !}$ then the maximum value of $\tan A \tan B$ is
(a). $\frac{1}{2}$
(b). $\frac{1}{3}$
(c). $\frac{1}{4}$
(d). $\frac{1}{5}$
Show Answer
Answer: (b)15. If $\mathrm{A} _{1} \mathrm{~A} _{2} \mathrm{~A} _{3} \ldots \ldots \mathrm{A} _{\mathrm{n}}$ be a regular polygon of $\mathrm{n}$ sides and $\frac{1}{\mathrm{~A} _{1} \mathrm{~A} _{2}}=\frac{1}{\mathrm{~A} _{1} \mathrm{~A} _{3}}+\frac{1}{\mathrm{~A} _{1} \mathrm{~A} _{4}}$, then
(a). $\mathrm{n}=5$
(b). $\mathrm{n}=6$
(c). $\mathrm{n}=7$
(d). None of these










