Sine rule

1. $\frac{\sin A}{a}=\frac{\sin B}{b}=\frac{\sin C}{c}=k$

or $\frac{a}{\sin A}=\frac{b}{\sin B}=\frac{c}{\sin C}=\lambda$

Cosine rule

2. (i). $\quad \cos \mathrm{A}=\frac{\mathrm{b}^{2}+\mathrm{c}^{2}-\mathrm{a}^{2}}{2 \mathrm{bc}}$

(ii). $\quad \cos \mathrm{B}=\frac{\mathrm{c}^{2}+\mathrm{a}^{2}-\mathrm{b}^{2}}{2 \mathrm{ca}}$

(iii). $\quad \cos \mathrm{C}=\frac{\mathrm{a}^{2}+\mathrm{b}^{2}-\mathrm{c}^{2}}{2 \mathrm{ab}}$

Projection formulae

3. (i). $\quad b \cos \mathrm{C}+\mathrm{c} \cos \mathrm{B}=\mathrm{a}$

(ii). $\quad \mathrm{c} \cos \mathrm{A}+\mathrm{a} \cos \mathrm{C}=\mathrm{b}$

(iii). $\quad a \cos \mathrm{B}+\mathrm{b} \cos \mathrm{A}=\mathrm{c}$

Napier’s analogy

4. (i). $\quad \tan \left(\frac{\mathrm{B}-\mathrm{C}}{2}\right)=\frac{\mathrm{b}-\mathrm{c}}{\mathrm{b}+\mathrm{c}} \cot \frac{\mathrm{A}}{2}$

(ii). $\quad \tan \left(\frac{\mathrm{C}-\mathrm{A}}{2}\right)=\frac{\mathrm{c}-\mathrm{a}}{\mathrm{c}+\mathrm{a}} \cot \frac{\mathrm{B}}{2}$

(iii). $\quad \tan \left(\frac{\mathrm{A}-\mathrm{B}}{2}\right)=\frac{\mathrm{a}-\mathrm{b}}{\mathrm{a}+\mathrm{b}} \cot \frac{\mathrm{C}}{2}$

5. (a). (i). $\sin \frac{\mathrm{A}}{2}=\sqrt{\frac{(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}{\mathrm{bc}}}$

(ii). $\quad \sin \frac{\mathrm{B}}{2}=\sqrt{\frac{(\mathrm{s}-\mathrm{c})(\mathrm{s}-\mathrm{a})}{\mathrm{ca}}}$

(iii). $\quad \sin \frac{\mathrm{C}}{2}=\sqrt{\frac{(\mathrm{s}-\mathrm{a})(\mathrm{s}-\mathrm{b})}{\mathrm{ab}}}$

(b). (i). $\quad \cos \frac{\mathrm{A}}{2}=\sqrt{\frac{\mathrm{s}(\mathrm{s}-\mathrm{a})}{\mathrm{bc}}}$

(ii). $\quad \cos \frac{\mathrm{B}}{2}=\sqrt{\frac{\mathrm{s}(\mathrm{s}-\mathrm{b})}{\mathrm{ca}}}$

(iii). $\quad \cos \frac{\mathrm{C}}{2}=\sqrt{\frac{\mathrm{s}(\mathrm{s}-\mathrm{c})}{\mathrm{ab}}}$

(c). (i). $\quad \tan \frac{\mathrm{A}}{2}=\sqrt{\frac{(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}{\mathrm{s}(\mathrm{s}-\mathrm{a})}}$

(ii). $\quad \tan \frac{\mathrm{B}}{2}=\sqrt{\frac{(\mathrm{s}-\mathrm{c})(\mathrm{s}-\mathrm{a})}{\mathrm{s}(\mathrm{s}-\mathrm{b})}}$

(iii). $\quad \tan \frac{\mathrm{C}}{2}=\sqrt{\frac{(\mathrm{s}-\mathrm{a})(\mathrm{s}-\mathrm{b})}{\mathrm{s}(\mathrm{s}-\mathrm{c})}}$

(d). (i). $\quad \cot \frac{\mathrm{A}}{2}=\sqrt{\frac{\mathrm{s}(\mathrm{s}-\mathrm{a})}{(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}}$

(ii). $\quad \cot \frac{\mathrm{B}}{2}=\sqrt{\frac{\mathrm{s}(\mathrm{s}-\mathrm{b})}{(\mathrm{s}-\mathrm{c})(\mathrm{s}-\mathrm{a})}}$

(iii). $\quad \cot \frac{\mathrm{C}}{2}=\sqrt{\frac{\mathrm{s}(\mathrm{s}-\mathrm{c})}{(\mathrm{s}-\mathrm{a})(\mathrm{s}-\mathrm{b})}}$

(e). (i). $\quad \sin \mathrm{A}=\frac{2 \Delta}{\mathrm{bc}}=\frac{2}{\mathrm{bc}} \sqrt{\mathrm{s}(\mathrm{s}-\mathrm{a})(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}$

(ii). $\quad \sin \mathrm{B}=\frac{2 \Delta}{\mathrm{ca}}=\frac{2}{\mathrm{ca}} \sqrt{\mathrm{s}(\mathrm{s}-\mathrm{a})(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}$

(i). $\quad \sin \mathrm{C}=\frac{2 \Delta}{\mathrm{ab}}=\frac{2}{\mathrm{ab}} \sqrt{\mathrm{s}(\mathrm{s}-\mathrm{a})(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})}$

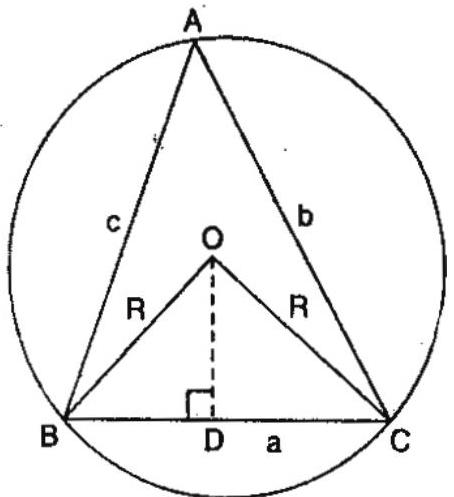
Radius of the circumcircle ‘R’

6. $\mathrm{R}=\frac{\mathrm{a}}{2 \sin \mathrm{A}}=\frac{\mathrm{b}}{2 \sin \mathrm{B}}=\frac{\mathrm{c}}{2 \sin \mathrm{C}}=\frac{\mathrm{abc}}{4 \Delta}$

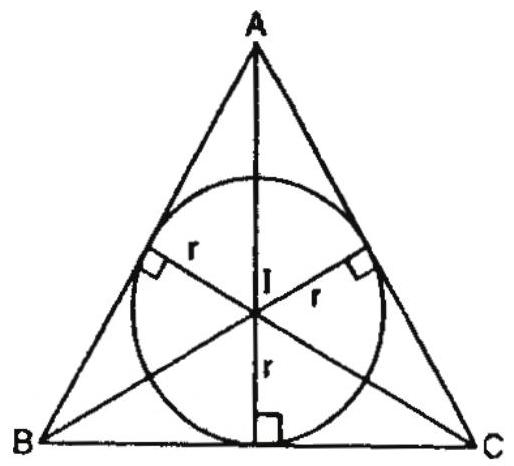
Radius of the incircle ‘$r$’

7. $\mathrm{r}=\frac{\Delta}{\mathrm{s}}=(\mathrm{s}-\mathrm{a}) \tan \frac{\mathrm{A}}{2}=(\mathrm{s}-\mathrm{b}) \tan \frac{\mathrm{B}}{2}=(\mathrm{s}-\mathrm{c}) \tan \frac{\mathrm{C}}{2}=4 \mathrm{R} \sin \frac{\mathrm{A}}{2} \sin \frac{\mathrm{B}}{2} \sin \frac{\mathrm{C}}{2}$

Radii of the excircles $r _{1}, r _{2}$ and $r _{3}$

8. (i). $\quad \mathrm{r} _{1}=\frac{\Delta}{\mathrm{s}-\mathrm{a}}=\mathrm{stan} \frac{\mathrm{A}}{2}=4 \mathrm{R} \sin \frac{\mathrm{A}}{2} \cos \frac{\mathrm{B}}{2} \cos \frac{\mathrm{C}}{2}$

(ii). $\quad \mathrm{r} _{2}=\frac{\Delta}{\mathrm{s}-\mathrm{b}}=\mathrm{s} \tan \frac{\mathrm{B}}{2}=4 \mathrm{R} \cos \frac{\mathrm{A}}{2} \sin \frac{\mathrm{B}}{2} \cos \frac{\mathrm{C}}{2}$

(iii). $\quad r _{3}=\frac{\Delta}{s-c}=s \tan \frac{C}{2}=4 R \cos \frac{A}{2} \cos \frac{B}{2} \sin \frac{C}{2}$

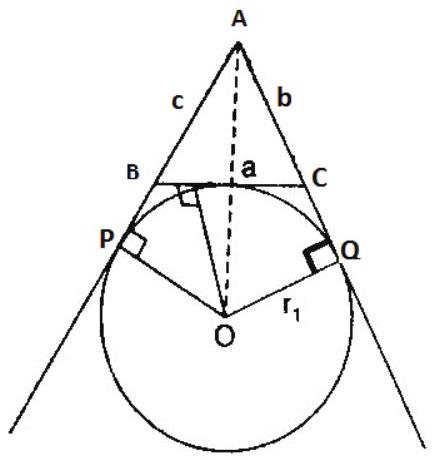
(iv). $\quad r _{1}=\frac{a \cos \frac{B}{2} \cos \frac{C}{2}}{\cos \frac{A}{2}}$ etc

(v). $\quad \mathrm{r} _{1}+\mathrm{r} _{2}+\mathrm{r} _{3}=\mathrm{r}+4 \mathrm{r}$

(vi). $\quad \frac{1}{\mathrm{r} _{1}}+\frac{1}{\mathrm{r} _{2}}+\frac{1}{\mathrm{r} _{3}}=\frac{1}{\mathrm{r}}$

(vii). $\quad \mathrm{r} _{1} \mathrm{r} _{2}+\mathrm{r} _{2} \mathrm{r} _{3}+\mathrm{r} _{3} \mathrm{r} _{1}=\mathrm{s}^{2}$

(viii). $\quad a \cos A+b \cos B+c \cos C=4 R \sin A \sin B \sin C$

(ix). $\quad a \cot \mathrm{A}+\mathrm{b} \cot \mathrm{B}+\mathrm{c} \cot \mathrm{C}=2(\mathrm{R}+\mathrm{r})$

Also, $r=\frac{a \sin \frac{B}{2} \sin \frac{C}{2}}{\cos \frac{A}{2}}=\frac{b \sin \frac{C}{2} \sin \frac{A}{2}}{\cos \frac{B}{2}}=\frac{c \sin \frac{A}{2} \sin \frac{B}{2}}{\cos \frac{C}{2}}$

9. If length of the median $\mathrm{AD}, \mathrm{BE}$ and $\mathrm{CF}$ are given, then sides can be determined by using the formula

$ \mathrm{a}^{2}=\frac{4}{3}\left(2 \mathrm{BE}^{2}+2 \mathrm{CF}^{2}-\mathrm{AD}^{2}\right) $

Similarly $\quad b^{2}=\frac{4}{3}\left(2 \mathrm{CF}^{2}+2 \mathrm{AD}^{2}-\mathrm{BE}^{2}\right)$

and $\mathrm{c}^{2}=\frac{4}{3}\left(2 \mathrm{AD}^{2}+2 \mathrm{BE}^{2}-\mathrm{CF}^{2}\right)$

10. Distance of the orthocentre from the vertex $A$ is $2 R \cos A$.

Pedal triangle

11. Triangle formed by joining the foot of perpendiculars drawn from vertices to opposite sides of a given tri-angle is called pedal triangle.

Length of the sides of pedal triangle of given $\triangle \mathrm{ABC}$ are $\mathrm{a} \cos \mathrm{A}, \mathrm{b} \cos \mathrm{B}$ and $\mathrm{c} \cos \mathrm{C}$ respectively. Angles of the pedal triangle are $180^{\circ}-2 \mathrm{~A}, 180^{\circ}-2 \mathrm{~B}$ and $180^{\circ}-2 \mathrm{C}$ respectively.

Excentric triangle

12. If $\mathrm{I} _{1}, \mathrm{I} _{2}$, and $\mathrm{I} _{3}$ are the centres of excircles, then $\Delta \mathrm{I} _{1} \mathrm{I} _{2} \mathrm{I} _{3}$ is called excentric triangle. $\mathrm{I} _{2}, \mathrm{~A}, \mathrm{I} _{3}$;

$\mathrm{I} _{3}, \mathrm{~B}, \mathrm{I} _{1}$ and $\mathrm{I} _{2}, \mathrm{C}, 1 _{2}$ are collinear. $\triangle \mathrm{ABC}$ is the pedal $\Delta$ of $\Delta \mathrm{I} _{1} \mathrm{I} _{2} \mathrm{I} _{3}$. Incentre ’ $\mathrm{I}$ ’ of the $\triangle \mathrm{ABC}$ will be orthocentre of $\Delta \mathrm{I} _{1} \mathrm{I} _{2} \mathrm{I} _{3}$.

13. If median $\mathrm{AD}$ inclined at angles $\alpha, \beta, \gamma$ with $\mathrm{BC}, \mathrm{CA}$ and $\mathrm{AB}$ respectively then

$\sin \alpha=\frac{b \sin C}{\sqrt{2 b^{2}+2 c^{2}-a^{2}}}$

$\sin \beta=\frac{\mathrm{a} \sin \mathrm{C}}{\sqrt{2 \mathrm{~b}^{2}+2 \mathrm{c}^{2}-\mathrm{a}^{2}}}$ and $\sin \gamma=\frac{\mathrm{a} \sin \mathrm{B}}{\sqrt{2 \mathrm{~b}^{2}+2 \mathrm{c}^{2}-\mathrm{a}^{2}}}$

14. Distance between orthocentre and circumcentre

$=R \sqrt{1-8 \cos A \cos B \cos C}$

15. Distance between circumcentre and incentre is

$R \sqrt{1-8 \sin \frac{\mathrm{A}}{2} \sin \frac{\mathrm{B}}{2} \sin \frac{\mathrm{C}}{2}}=\sqrt{\mathrm{R}(\mathrm{R}-2 \mathrm{r})}$

16. Distance between circumcentre and centre of excircles are

$\mathrm{OI} _{1} \mathrm{R} \sqrt{1+8 \sin \frac{\mathrm{A}}{2} \cos \frac{\mathrm{B}}{2} \cos \frac{\mathrm{C}}{2}}=\sqrt{\mathrm{R}\left(\mathrm{R}+2 \mathrm{r} _{1}\right)}$

$\mathrm{OI} _{2} \mathrm{R} \sqrt{1+8 \cos \frac{\mathrm{A}}{2} \sin \frac{\mathrm{B}}{2} \cos \frac{\mathrm{C}}{2}}=\sqrt{\mathrm{R}\left(\mathrm{R}+2 \mathrm{r} _{2}\right)}$

$\mathrm{OI} _{3} \mathrm{R} \sqrt{1+8 \cos \frac{\mathrm{A}}{2} \cos \frac{\mathrm{B}}{2} \sin \frac{\mathrm{C}}{2}}=\sqrt{\mathrm{R}\left(\mathrm{R}+2 \mathrm{r} _{3}\right)}$

17. Length of the angle bisectors

$A D=\frac{2 b c}{b+c} \cos \frac{A}{2}=\frac{b c \sin A}{b+c \sin \frac{A}{2}}$

$\mathrm{BE}=\frac{2 \mathrm{ca}}{\mathrm{c}+\mathrm{a}} \cos \frac{\mathrm{B}}{2}$ and $\mathrm{CF}=\frac{2 \mathrm{ab}}{\mathrm{a}+\mathrm{b}} \cos \frac{\mathrm{C}}{2}$

18. Area of quadrilateral $\mathrm{ABCD}$, if sum of a pair opposite angle is $2 \alpha$ is

$\sqrt{(s-a)(s-b)(s-c)(s-d)-a b c d \cos ^{2} \alpha}$

where $2 \mathrm{~s}=\mathrm{a}+\mathrm{b}+\mathrm{c}+\mathrm{d}$

19. $\mathrm{m}-\mathrm{n}$ theorem

$(\mathrm{m}+\mathrm{n}) \cot \theta=\mathrm{m} \cot \alpha-\mathrm{n} \cot \beta$

$(\mathrm{m}+\mathrm{n}) \cot \theta=\mathrm{n} \cot \mathrm{B}-\mathrm{m} \cot \mathrm{C}$

Some extra tips

1. $\quad$i. $\quad \sum \mathrm{a}^{3} \sin (\mathrm{B}-\mathrm{C})=0$

$\quad$ $\quad$ii. $\quad \sum \mathrm{a}^{3} \cos (\mathrm{B}-\mathrm{C})=3 \mathrm{abc}$

2. $\quad$(i). If $a \cos B=b \cos A$, then the triangle is isosceles

$\quad$ (ii). $\quad$If $a \cos \mathrm{A}=\mathrm{b} \cos \mathrm{B}$, then the triangle is isosceles or right angled

$\quad$ (iii). $\quad$If $\mathrm{a}^{2}+\mathrm{b}^{2}+\mathrm{c}^{2}=8 \mathrm{R}^{2}$, then the triangle is right angled

$\quad$ (iv). $\quad$If $\cos ^{2} \mathrm{~A}+\cos ^{2} \mathrm{~B}+\cos ^{2} \mathrm{C}=1$, then the triangle is right angled

$\quad$ (v). $\quad$If $\cos A=\frac{\sin B}{2 \sin C}$, then the triangle is isosceles

$\quad$ (vi). $\quad$If $\frac{\mathrm{a}}{\cos \mathrm{A}}=\frac{\mathrm{b}}{\cos \mathrm{B}}=\frac{\mathrm{c}}{\cos \mathrm{C}}$, then the triangle is equilateral

$\quad$ (vii). $\quad$If $\cos \mathrm{A}+\cos \mathrm{B}+\cos \mathrm{C}=\frac{3}{2}$, then the triangle is equilateral

$\quad$ (viii). $\quad$If $\sin \mathrm{A}+\sin \mathrm{B}+\sin \mathrm{C}=\frac{3 \sqrt{3}}{2}$, then the triangle is equilateral

$\quad$ (ix). $\quad$If $\tan A+\tan B+\tan C=3 \sqrt{3}$, then the triangle is equilateral

$\quad$ (x). $\quad$If $\cot A+\cot B+\cot C=\sqrt{3}$, then the triangle is equilateral

3. (a). The circumcentre lies (i) inside an acute angled triangle (ii) outside an obtuse angled triangle.

$\quad$ (b). The circumcircle of a right angled triangle is the mid point of the hypotenuse.

$\quad$ (c). The orthocentre of a right angled triangle is the vertex at the right angle.

4. Triangle is equilateral if any one of the following holds:

$\quad$ (a). $\mathrm{R}=2 \mathrm{r}$

$\quad$ (b). $r _{1}=r _{2}=r _{3}$

$\quad$ (c). $r: R: r _{1}=1: 2: 3$

5. Triangle is right angled if $r: R: r _{1}=2: 5: 12$

6. If $\mathrm{r} _{1}, \mathrm{r} _{2}, \mathrm{r} _{3}$ are in H.P. iff $\mathrm{a}, \mathrm{b}, \mathrm{c}$ are in A.P.

7. In an equilateral triangle:

$\quad$ (a). area $=\frac{\sqrt{3} \mathrm{a}^{2}}{4}$

$\quad$ (b). $ \mathrm{R}=\frac{\mathrm{a}}{\sqrt{3}}$

$\quad$ (c). $ r=\frac{R}{2}$

$\quad$ (d). $r _{1}=r _{2}=r _{3}=\frac{3 R}{2}$

$\quad$ e. $\mathrm{r}=\mathrm{R}=\mathrm{r} _{1}=1: 2: 3$

Examples

1. In triangle $\mathrm{ABC}, 2 \mathrm{ac} \sin \left(\frac{1}{2}(\mathrm{~A}-\mathrm{B}+\mathrm{C})\right.$ is equal to

(a). $\mathrm{a}^{2}+\mathrm{b}^{2}-\mathrm{c}^{2}$

(b). $\mathrm{c}^{2}+\mathrm{a}^{2}-\mathrm{b}^{2}$

(c). $\mathrm{b}^{2}-\mathrm{c}^{2}-\mathrm{a}^{2}$

(d). $\mathrm{c}^{2}-\mathrm{a}^{2}-\mathrm{b}^{2}$

2. Let $\mathrm{ABC}$ be a triangle such that $\angle \mathrm{ACB}=\frac{\pi}{6}$ and let $\mathrm{a}, \mathrm{b}$ and $\mathrm{c}$ denote the lengths of the side opposite to $\mathrm{A}, \mathrm{B}$ and $\mathrm{C}$, respectively. The values of $\mathrm{x}$ for which $\mathrm{a}=\mathrm{x}^{2}+\mathrm{x}+1, \mathrm{~b}=\mathrm{x}^{2}-1$ and $\mathrm{c}=2 \mathrm{x}+1$ is (are)

(a). $-(2+\sqrt{3})$

(b). $1+\sqrt{3}$

(c). $2+\sqrt{3}$

(d). $4 \sqrt{3}$

3. In $\triangle \mathrm{ABC}$, interval angle bisector of $\angle \mathrm{A}$ meets side $\mathrm{BC}$ in $\mathrm{D}$. $\mathrm{DE} \perp \mathrm{AD}$ meets $\mathrm{AC}$ in $\mathrm{E}$ and $\mathrm{AB}$ in F. Then

(a). $\mathrm{AE}$ is $\mathrm{HM}$ of $\mathrm{b}$ and $\mathrm{c}$

(b). $\mathrm{AD}=\frac{2 \mathrm{bc}}{\mathrm{b}+\mathrm{c}} \cos \frac{\mathrm{A}}{2}$

(c). $\mathrm{EF}=\frac{4 \mathrm{bc}}{\mathrm{b}+\mathrm{c}} \sin \frac{\mathrm{A}}{2}$

(d). $\triangle \mathrm{AEF}$ is isosceles

Show Answer

Solution:

In $\triangle \mathrm{AFE}$, we get $\mathrm{AF}=\mathrm{AE}$

$\therefore \triangle \mathrm{AFE}$ is an isosceles $\Delta$

$\operatorname{ar}(\triangle \mathrm{ABC})=\operatorname{ar}(\triangle \mathrm{ABD})+\operatorname{ar}(\Delta \mathrm{ADC})$

$=\frac{1}{2} \mathrm{bc} \sin \mathrm{A}=\frac{1}{2} \mathrm{c} \mathrm{AD} \sin \frac{\mathrm{A}}{2}+\frac{1}{2} \mathrm{bAD} \sin \frac{\mathrm{A}}{2}$

$2 \mathrm{bcsin} \frac{\mathrm{A}}{2} \cos \frac{\mathrm{A}}{2}=\mathrm{AD} \sin \frac{\mathrm{A}}{2}(\mathrm{~b}+\mathrm{c})$

$\mathrm{AD}=\frac{2 \mathrm{bc} \cos \frac{\mathrm{A}}{2}}{\mathrm{~b}+\mathrm{c}}$

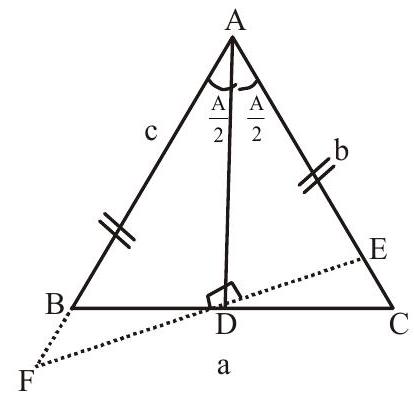
Also $\mathrm{AD}=\mathrm{AE} \cos \frac{\mathrm{A}}{2}$

$\mathrm{AE} \cos \frac{\mathrm{A}}{2}=\frac{2 \mathrm{bc} \cos \frac{\mathrm{A}}{2}}{\mathrm{~b}+\mathrm{c}}$

$\therefore \mathrm{AE}$ is $\mathrm{HM}$ of $\mathrm{b}$ and $\mathrm{c}$

Again $\mathrm{EF}=2 \mathrm{DE}=2 \mathrm{AD} \tan \frac{\mathrm{A}}{2}=\frac{2.2 \mathrm{bc} \cos \frac{\mathrm{A}}{2} \cdot \tan \frac{\mathrm{A}}{2}}{\mathrm{~b}+\mathrm{c}}$

$ =\frac{4 b c \sin \frac{A}{2}}{b+c} $

Hence option a, b, c, d are correct

4. In a triangle $\mathrm{ABC}$ with fixed base $\mathrm{BC}$, the vertex $\mathrm{A}$ moves such that $\cos \mathrm{B}+\cos \mathrm{C}=4 \sin ^{2} \frac{\mathrm{A}}{2}$

If $\mathrm{a}, \mathrm{b}$ and $\mathrm{c}$ denote the lengths of the sides of the triangle opposite to the angles $\mathrm{A}, \mathrm{B}$ and $\mathrm{C}$ respectively, Then

(a). $\mathrm{b}+\mathrm{c}=4 \mathrm{a}$

(b). $\mathrm{b}+\mathrm{c}=2 \mathrm{a}$

(c). locus of point $\mathrm{A}$ is an ellipse

(d). locus of point $\mathrm{A}$ is a pair of straight lines.

Practice questions

1. The roots of the equation $6 x^{2}-5 x+1=0$ are $\tan \frac{A}{2}$ and $\tan \frac{B}{2}$ where $A, B, C$ are the angles of a triangle, then

(a). $\mathrm{a}^{2}+\mathrm{b}^{2}>\mathrm{c}^{2}$

(b). $\mathrm{a}^{2}+\mathrm{b}^{2}=\mathrm{c}^{2}$

(c). $\mathrm{a}^{2}-\mathrm{b}^{2}=\mathrm{c}^{2}$

(d). None of these

2. If $\frac{\cos \mathrm{A}}{\mathrm{a}}=\frac{\cos \mathrm{B}}{\mathrm{b}}=\frac{\cos \mathrm{C}}{\mathrm{c}}$ and the side $\mathrm{a}=2$ then area of triangle is

(a). $1$

(b). $2$

(c). $\frac{\sqrt{3}}{2}$

(d). $\sqrt{3}$

3. If in a triangle $\mathrm{PQR}, \sin \mathrm{P}, \sin \mathrm{Q}, \sin \mathrm{R}$ are in $\mathrm{A} . \mathrm{P}$ then

(a). The altitudes are in A.P

(b). The altitudes are in H.P

(c). The medians are in G.P

(d). The medians are in A.P

4. If the sides $a, b, c$ of $\triangle A B C$ are in A.P then $\cot \frac{1}{2} \mathrm{~A}, \cot \frac{1}{2} \mathrm{~B}, \cot \frac{1}{2} \mathrm{C}$ are in

(a). A.P

(b). G.P

(c). H.P

(d). None of these

5. If radius of the incircle of a triangle with sides $5 p, 6 p$ and $5 p$ is 6 , then $p$ is equal to

(a). 4

(b). 6

(c). 8

(d). 10

6. In any $\triangle \mathrm{ABC}, \sum\left[\frac{\sin ^{2} \mathrm{~A}+\sin \mathrm{A}+1}{\sin \mathrm{A}}\right]$ is always greater than

(a). 9

(b). 3

(c). 27

(d). 10

7. If $\mathrm{c}^{2}=\mathrm{a}^{2}+\mathrm{b}^{2}$, then $4 \mathrm{~s}(\mathrm{~s}-\mathrm{a})(\mathrm{s}-\mathrm{b})(\mathrm{s}-\mathrm{c})=$

(a). $\mathrm{s}^{4}$

(b). $b^{2} \mathrm{c}^{2}$

(c). $\mathrm{c}^{2} \mathrm{a}^{2}$

(d). $\mathrm{a}^{2} \mathrm{~b}^{2}$

8. If $a, b, c, d$ be the sides of a quadrilateral, then the minimum value of $\frac{a^{2}+b^{2}+c^{2}}{d^{2}}$ is equal to

(a). $\frac{1}{2}$

(b). $\frac{1}{3}$

(c). $\frac{1}{4}$

(d). $1$

9. $\frac{\mathrm{r} _{1}}{\mathrm{bc}}+\frac{\mathrm{r} _{2}}{\mathrm{ca}}=\frac{\mathrm{r} _{3}}{\mathrm{ab}}=$

(a). $\frac{1}{2 \mathrm{R}}-\frac{1}{\mathrm{r}}$

(b). $2 \mathrm{R}-\mathrm{r}$

(c). $\mathrm{r}-2 \mathrm{R}$

(d). $\frac{1}{\mathrm{r}}-\frac{1}{2 \mathrm{R}}$

10. Passage

(a). $\frac{\mathrm{a}}{\sin \mathrm{A}}=2 \mathrm{R}, \mathrm{R}=\frac{\mathrm{abc}}{4 \Delta}$

(b). $\mathrm{r}=\frac{\Delta}{3}=(\mathrm{s}-\mathrm{a}) \tan \frac{\mathrm{A}}{2}=4 \mathrm{R} \sin \frac{\mathrm{A}}{2} \sin \frac{\mathrm{B}}{2} \sin \frac{\mathrm{C}}{2}$

(c). $\mathrm{r} _{1}=\frac{\Delta}{\mathrm{s}-\mathrm{a}}=\mathrm{s} \tan \frac{\mathrm{A}}{2}=4 \mathrm{R} \sin \frac{\mathrm{A}}{2} \cos \frac{\mathrm{B}}{2} \cos \frac{\mathrm{C}}{2}$

(d). If P be the orthocenter of a $\triangle \mathrm{ABC}$ and its distances PA from the vertex $\mathrm{A}$ and PL from the sides $\mathrm{BC}$ are $\mathrm{PA}=2 \mathrm{R} \cos \mathrm{A}, \mathrm{PL}=2 \mathrm{R} \cos \mathrm{B} \cos \mathrm{C}$

Answer the following questions based upon above passage

(i). If $r _{1}=2 r _{2}=3 r _{3}$, then

(a). $\frac{\mathrm{a}}{\mathrm{b}}=\frac{4}{5}$

(b). $\frac{\mathrm{a}}{\mathrm{b}}=\frac{5}{4}$

(c). $\frac{\mathrm{a}}{\mathrm{c}}=\frac{3}{5}$

(d). $\frac{\mathrm{a}}{\mathrm{c}}=\frac{5}{3}$

(ii). $\frac{1}{\mathrm{r} _{1}^{2}}+\frac{1}{\mathrm{r} _{2}^{2}}+\frac{1}{\mathrm{r} _{3}^{2}}+\frac{1}{\mathrm{r}^{2}}=$

(a). $\frac{\mathrm{a}^{2}+\mathrm{b}^{2}+\mathrm{c}^{2}}{\mathrm{~s}^{2}}$

(b). $\frac{\sum \mathrm{a}^{2}}{\Delta^{2}}$

(c). $4 \mathrm{R}$

(d). $4 \mathrm{r}$

(iii). $\frac{\mathrm{r} _{1}-\mathrm{r}}{\mathrm{a}}+\frac{\mathrm{r} _{2}-\mathrm{r}}{\mathrm{b}}=$

(a). $\frac{\mathrm{a}}{\mathrm{r} _{1}}$

(b). $\frac{\mathrm{b}}{\mathrm{r} _{2}}$

(c). $\frac{\mathrm{c}}{\mathrm{r} _{3}}$

(d). None of these

(iv). In a triangle $\mathrm{ABC}$, let $\angle \mathrm{c}=\frac{\pi}{2}$. If $\mathrm{r}$ is the inradius and $\mathrm{R}$ is the circumradius of the triangle then $2(r+R)$ is equal to

(a). $a+b$

(b). $\mathrm{b}+\mathrm{c}$

(c). $\mathrm{c}+\mathrm{a}$

(d). $\mathrm{a}+\mathrm{b}+\mathrm{c}$