TRIGONOMETRY EQUATIONS - 2 (Trigonometric Equations)
1. Trigonometric equation
An equation involving one or more trigonometrical ratios of unknown angles is called a trigonometrical equation.
2. Solution of trigonometric equation
A value of the unknown angle which satisfies the given equation is called a solution of the equation.
(a). Principal solution. The smallest numerical value, positive or negative for the angle of a trigonometrical equation is called its principal solution. If a positive angle as well as a negative angle of smallest value (and equal) are available, then we take the positive value as the principal values.
(i). The principal solution of $\sin \theta=\mathrm{k},|\mathrm{k}| \leq 1$ is the value of $\theta$ in the interval $\left[-\frac{\pi}{2}, \frac{\pi}{2}\right]$ which satisfy the equation.
(ii). The principal solution of $\cos \theta=\mathrm{k},|\mathrm{k}| \leq 1$ is the value of $\theta$ in the interval $[0, \pi]$ which satisfy the equation.
(iii). The principal solution of $\tan \theta=\mathrm{k},|\mathrm{k}|<\infty$, the value of $\theta$ in the interval $\left(-\frac{\pi}{2}, \frac{\pi}{2}\right)$, which satisfy the equation.
(b) General solution. Since all the trigonometric functions are many one onto, therefore, there are infinite values of $\theta$ for which trigonometric functions have the same value. Therefore, all such possible values of $\theta$ for which trigonometric ratios are same is known as general solutions of the variable angle $\theta$.
3. General solutions of trigonometric ratios
$\quad$ (i). If $\sin \theta=0$, then $\theta=n \pi, n \in Z$ (set of integers)
$\quad$ (ii). If $\cos \theta=0$, then $\theta=(2 n+1) \pi / 2, n \in Z$
$\quad$ (iii). If $\tan \theta=0$, then $\theta=n \pi, n \in Z$
$\quad$ (iv). If $\sin \theta=\sin \alpha$ then $\theta=\mathrm{n} \pi+(-1)^{\mathrm{n}} \alpha, \mathrm{n} \in \mathrm{Z}, \alpha \in\left[-\frac{\pi}{2}, \frac{\pi}{2}\right]$
$\quad$ (v). If $\cos \theta=\cos \alpha$, then $\theta=2 \mathrm{n} \pi \pm \alpha, n \in Z, \alpha \in[0, \pi]$
$\quad$ (vi). If $\tan \theta=\tan \alpha$, then $\theta=\mathrm{n} \pi+\alpha, \mathrm{n} \in \mathrm{Z} \alpha \in\left[-\frac{\pi}{2}, \frac{\pi}{2}\right]$
$\quad$ (vii). If $\sin \theta=1$, then $\theta=2 \mathrm{n} \pi+\frac{\pi}{2}, \mathrm{n} \in \mathrm{Z}$
$\quad$ (viii). If $\cos \theta=1$, then $\theta=2 \mathrm{n} \pi, \mathrm{n} \in \mathrm{Z}$
$\quad$ (ix). If $\sin ^{2} \theta=\sin ^{2} \alpha$ or $\cos ^{2} \theta=\cos ^{2} \alpha$ or $\tan ^{2} \theta=\tan ^{2} \alpha$ then $\theta=n \pi \pm \alpha, n \in Z$
$\quad$ (x). If $\sin (a \theta+b)=\sin \alpha$, then $\theta \neq n \pi+(-1)^{n}\left(\frac{a-b}{a}\right)$. First of all we have to write the general solution as such for $\mathrm{a} \theta+\mathrm{b}$ and then, find $\theta$ as $\mathrm{a} \theta+b=n \pi+(-1)^{\mathrm{n}} . \alpha$
$\quad$ $\Rightarrow \theta=\frac{1}{\mathrm{a}}\left[\mathrm{n} \pi+(-1)^{\mathrm{n}} \boldsymbol{\alpha}-\mathrm{b}\right]$
$\quad$ (xi). For $\mathrm{n} \in \mathrm{Z}, \sin \mathrm{n} \pi=0$ and $\cos \mathrm{n} \pi=(-1)^{\mathrm{n}}$
$\quad$ $\sin (\mathrm{n} \pi+\theta)=(-1)^{\mathrm{n}} \sin \theta$
$\quad$ $\cos (\mathrm{n} \pi+\theta)=(-1)^{\mathrm{n}} \cos \theta$
$\quad$ (xii). If $\mathrm{n}$ is an odd integer, then
$\quad$ $\sin \frac{\mathrm{n} \pi}{2}=(-1)^{\frac{\mathrm{n}-1}{2}} \cos \frac{\mathrm{n} \pi}{2}=0$
$\quad$ $\sin \left(\frac{\mathrm{n} \pi}{2}+\theta\right)=(-1)^{\frac{\mathrm{n}-1}{2}} \cos \theta$
$\quad$ $\cos \left(\frac{\mathrm{n} \pi}{2}+\theta\right)=(-1)^{\frac{\mathrm{n}+1}{2}} \sin \theta$
4. Important points to remember
$\quad$ (i). For equations of the type $\sin \theta=\mathrm{k}$ or $\cos \theta=\mathrm{k}$, one must check that $|\mathrm{k}| \leq 1$.
$\quad$ (ii). Avoid squaring the equation, if possible, because it may lead to extraneous solutions.
$\quad$ (iii). Do not cancel the common variable factor from the two sides of the equations which are in a product because we may loose some solutions.
$\quad$ (iv). The answer should not contain such values of $\theta$ which make any of the terms undefined or infinite.
$\quad$ (v). Check that denominator is not zero at any stage while solving equations.
$\quad$ (vi). (a). If $\tan \theta$ or $\sec \theta$ is involved in the equation, $\theta$ should not be odd multiple of $\frac{\pi}{2}$.
$\quad$(b). If $\cot \theta$ or $\operatorname{cosec} \theta$ is involved in the equation, $\theta$ should not be a multiple of $\pi$ or 0 .
$\quad$(vii). If two different trigonometric ratios, such as, $\tan \theta$ and $\sec \theta$ are involved then after solving we cannot apply the usual formulae for general solution, because periodicity of the functions are not same.
$\quad$(viii). If L.H.S. of the given trigonometric equation is always less than or equal to $\mathrm{k}$ and RHS is always greater than $\mathrm{k}$, then no solution exists. If both the sides are equal to $\mathrm{k}$ for same value of $\theta$, then solution exists and if they are equal for different value of $\theta$, then solution does not exist.
Examples
1. The general solution of the equation $\sum \cos \left(\mathrm{r}^{2} \mathrm{x}\right) \sin (\mathrm{rx})=\frac{1}{2}$ is
(a). $2 \mathrm{~m} \pi+\frac{\pi}{6}, \mathrm{~m} \in \mathrm{I}$
(b). $\frac{(4 \mathrm{~m}+1)}{\mathrm{n}(\mathrm{n}+1)} \frac{\pi}{2} \mathrm{~m} \in \mathrm{I}$
(c). $\frac{(4 \mathrm{~m}-1)}{\mathrm{n}(\mathrm{n}+1)} \frac{\pi}{2}, \mathrm{~m} \in \mathrm{I}$
(d). None of these
Show Answer
Solution:
$ \begin{aligned} & \sum _{\mathrm{r}=1}^{\mathrm{n}} \cos \left(\mathrm{r}^{2} \mathrm{x}\right) \sin (\mathrm{rx})=\frac{1}{2} \\ & =\sum _{\mathrm{r}=1}^{\mathrm{n}} 2 \cos \left(\mathrm{r}^{2} \mathrm{x}\right) \sin (\mathrm{rx})=1 \\ & =\sum _{\mathrm{r}=1}^{\mathrm{n}}\left[\sin \left(\mathrm{r}^{2}+\mathrm{r}\right) \mathrm{x}-\sin \left(\mathrm{r}^{2}-\mathrm{r}\right) \mathrm{x}\right]=1 \\ & =\sum _{\mathrm{r}=1}^{\mathrm{n}}[\sin \mathrm{r}(\mathrm{r}+1) \mathrm{x}-\sin \mathrm{r}(\mathrm{r}-1) \mathrm{x}]=1 \\ & =\sin 2 \mathrm{x}-\sin 0+\sin 6 \mathrm{x}-\sin 2 \mathrm{x}+\sin 12 \mathrm{x}-\sin 6 \mathrm{x}+\ldots \ldots \ldots \ldots .+\sin (\mathrm{n}(\mathrm{n}+1 \\ & =\sin [\mathrm{n}(\mathrm{n}+1) \mathrm{x}]-\sin 0=1 \\ & =\sin (\mathrm{n}(\mathrm{n}+1) \mathrm{x})=1 \\ & =\sin n(\mathrm{n}+1) \mathrm{x}=\sin \frac{\pi}{2} \\ & \mathrm{n}(\mathrm{n}+1) \mathrm{x}=\left(2 \mathrm{~m} \pi+\frac{\pi}{2}\right), \mathrm{m} \in \mathrm{I} \\ & \mathrm{x}=\frac{4 \mathrm{~m} \pi+\pi}{2 \mathrm{n}(\mathrm{n}+1)}, \mathrm{m} \in \mathrm{I} \\ & =\frac{(4 \mathrm{~m}+1)}{\mathrm{n}(\mathrm{n}+1)} \frac{\pi}{2}, \mathrm{~m} _{\in} \mathrm{I} \end{aligned} $
Option ‘b’ is correct
2. If $[\sin x]+[\sqrt{2} \cos x]=-3, x \in[0,2 \pi][[.]$ denotes the greatest integer function $]$, then $x$ belongs to
(a). $\left(\pi, \frac{5 \pi}{4}\right)$
(b). $\left[\pi, \frac{5 \pi}{4}\right]$
(c). $\left(\frac{5 \pi}{4}, 2 \pi\right)$
(d). $\left[\frac{5 \pi}{4}, 2 \pi\right]$
Show Answer
Solution:
$ \because[\sin x]+[\sqrt{2} \cos x]=-3 $
$[\sin x]=-1 \quad[\sqrt{2} \cos x]=-2$
$\therefore-1 \leq \sin x<0 \quad$ and $\quad-2 \leq \sqrt{2} \cos x<-1$
$ -\sqrt{2} \leq \cos x<\frac{-1}{\sqrt{2}} $
$-1 \leq \sin x<0$ and $-1 \leq \cos x<-\frac{1}{\sqrt{2}}(\because-1 \leq \cos x \leq 1)$
since both sinx and cosx are negative
i.e. $\mathrm{x} \in(\pi, 2 \pi)$ and $\mathrm{x} \in\left(\frac{3 \pi}{4}, \frac{5 \pi}{4}\right)$
$\therefore \mathrm{x} \in\left(\pi, \frac{5 \pi}{4}\right)$
Option ‘a’ is correct
3. The number of solutions of the equation $\sin x+2 \sin 2 x=3+\sin 3 x$ in the interval $[0, \pi]$ is
(a). 0
(b). 1
(c). 2
(d). 3
Show Answer
Solution:
We have
$\sin x+2 \sin 2 x=3+\sin 3 x$
$\sin 3 x-\sin x-2 \sin 2 x+3=0$
$2 \cos 2 x \sin x-4 \sin x \cos x+3=0$
$2 \sin x(\cos 2 x-2 \cos x)+3=0$
$2 \sin x\left(2 \cos ^{2} x-1-2 \cos x\right)+3=0$
$\sin x\left(4 \cos ^{2} x-4 \cos x-2\right)+3=0$
$\sin x\left\{(2 \cos x-1)^{2}-3\right\}+3=0$
$\sin x(2 \cos x+1)^{2}+3(1-\sin x)=0$
$\because 0 \leq \mathrm{x} \leq \pi$
$\because 0 \leq \sin x \leq 1$
$1-\sin x \geq 0$
$\sin x(2 \cos x-1)^{2} \geq 0$
$\therefore$ each term is equal to zero
$1-\sin x=0 \Rightarrow \sin x=1$
$\therefore \cos \mathrm{X}=0$
$\sin x(2 \cos x-1)^{2} \neq 0$
$\therefore$ No solution
4. The number of values of $\theta$ in the interval $\left(-\frac{\pi}{2}, \frac{\pi}{2}\right)$ suggesting the equation $(\sqrt{3})^{\sec ^{2} \theta}=\tan ^{4} \theta+2 \tan ^{2} \theta$ is
(a). 1
(b). 2
(c). 3
(d). None of these
Show Answer
Solution:
We have
$ \begin{aligned} (\sqrt{3})^{\sec ^{2} \theta} & =\tan ^{4} \theta+2 \tan ^{2} \theta \\ & =\left(1+\tan ^{2} \theta\right)^{2}-1 \\ & =\left(\sec ^{2} \theta\right)^{2}-1 \end{aligned} $
let $\sec ^{2} \theta=\mathrm{x} \therefore \mathrm{x} \geq 1$
$(\sqrt{3})^{x}=x^{2}-1$
graph of $y=(\sqrt{3})^{x} \& y=x^{2}-1$
intersect at one point
When $\mathrm{x}=2 ; \mathrm{y}=3$
$ \therefore \sec ^{2} \theta=2 $
$ \sec \theta= \pm \sqrt{2} $
$\therefore \theta$ takes two values in $\left(-\frac{\pi}{2}, \frac{\pi}{2}\right)$
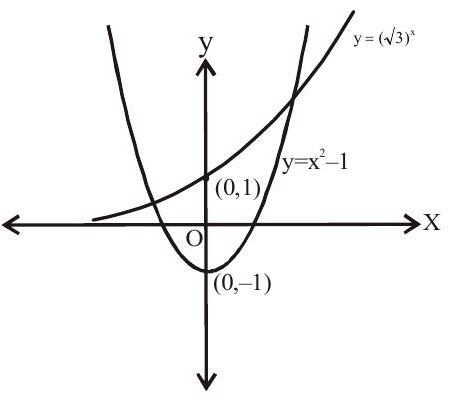
Option ‘b’ is correct
5. If $0 \leq x \leq \pi$ and $81^{\sin ^{2} x}+81^{\cos ^{2} x}=30$ then $x$ is equal to
(a). $\frac{\pi}{6}$
(b). $\frac{\pi}{2}$
(c). $\pi$
(d). $\frac{\pi}{4}$
Show Answer
Solution:
we have
$ \begin{aligned} & 81^{\sin ^{2} x}+81^{\cos ^{2} x}=30 \\ & 81^{\sin ^{2} x}+81^{1-\sin ^{2} x}=30 \\ & 81^{\sin ^{2} x}+\frac{81}{81^{\sin ^{2} x}}=30 \end{aligned} $
let $81^{\sin ^{2} x}=\mathrm{y}$
$ \begin{aligned} & y^{2}-30 y+81=0 \\ & y^{2}-27 y-3 y+81=0 \\ & (y-27)(y-3)=0 \\ & 81^{\sin ^{2} x}=27 \text { or } 3 \\ & 3^{4 \sin ^{2} x}=3^{3} \text { or } 3^{1} \\ & \therefore 4 \sin ^{2} x=3 \text { or } 1 \\ & \sin ^{2} x=\frac{3}{4} \text { or } \frac{1}{4} \\ & \sin x= \pm \frac{\sqrt{3}}{2} \text { or } \pm \frac{1}{2} \end{aligned} $
$\because 0 \leq \mathrm{x} \leq \pi \therefore \sin \mathrm{x}=\frac{\sqrt{3}}{2}$ or $\frac{1}{2}$
$\therefore \frac{\pi}{3}, \frac{2 \pi}{3}, \frac{\pi}{6}, \frac{5 \pi}{6}$
6. The equation a $\sin x+b \cos x=c$
where $|c|>\sqrt{a^{2}+b^{2}}$ has
(a). one solution
(b). two
(c). no solution
(d). infinite number of solution
Show Answer
Solution:
We have
$\operatorname{asin} x+b \cos x=c$
$ \frac{a}{\sqrt{a^{2}+b^{2}}} \sin x+\frac{b}{\sqrt{a^{2}+b^{2}}} \cos x=\frac{c}{\sqrt{a^{2}+b^{2}}} $
$ \begin{aligned} & \sin x \cos \alpha+\cos x \sin \alpha=\frac{c}{\sqrt{a^{2}+b^{2}}} \\ & \sin (x+\alpha)=\sin \beta>1 \because|c|>\sqrt{a^{2}+b^{2}} \text { i.e. } \frac{|c|}{\sqrt{a^{2}+b^{2}}}>1 \end{aligned} $
not possible
Oorrect option is c
Practice questions
1. If $\cos 2 \theta=(\sqrt{2+1})\left(\cos \theta \frac{1}{\sqrt{2}}\right)$, then $\theta=$
(a). $2 \mathrm{n} \pi$
(b). $2 \mathrm{n} \pi \pm \frac{\pi}{4}$
(c). $2 \mathrm{n} \pi \pm \frac{\pi}{3}$
(d). none
Show Answer
Answer: (b, c)2. It $3 \cos ^{2} \theta-2 \sqrt{3} \sin \theta \cos \theta-3 \sin ^{2} \theta=0$ then $\theta$ equals
(a). $\frac{n \pi}{2}+\frac{\pi}{6}$
(b). $\frac{n \pi}{2}-\frac{\pi}{6}$
(c). $\frac{n \pi}{2}+\frac{\pi}{3}$
(d). $\frac{n \pi}{2}-\frac{\pi}{3}$
Show Answer
Answer: (a)3. The general solution of $\sin x-3 \sin 2 x+\sin 3 x=\cos x-3 \cos 2 x+\cos 3 x$ is
(a). $\mathrm{n} \pi+\frac{\pi}{8}$
(b). $\frac{n \pi}{2}+\frac{\pi}{8}$
(c). $(-1)^{\mathrm{n}}\left(\frac{\mathrm{n} \pi}{2}\right)+\frac{\pi}{8}$
(d). $2 \mathrm{n} \pi+\cot ^{-1}\left(\frac{3}{2}\right)$
Show Answer
Answer: (b)4. The number of points of intersection of $2 y=1$ and $y=\cos x$ in $\frac{-\pi}{2} \leq x \leq \frac{\pi}{2}$ is
(a). 1
(b). 2
(c). 3
(d). 4
Show Answer
Answer: (b)5. The number of values of $x$ in the internal $[0,5 \pi]$ satisfying the equation $3 \sin ^{2} x-7 \sin x+2$ $=0$ is
(a). 0
(b). 5
(c). 6
(d). 10
Show Answer
Answer: (c)6. The sum of all the solutions of the equation $\cos \theta \cos \left(\frac{\pi}{3}+\theta\right) \cos \left(\frac{\pi}{3}-\theta\right)=\frac{1}{4}, \theta \in[0,6 \pi]$ is
(a). $15 \pi$
(b). $30 \pi$
(c). $\frac{100 \pi}{3}$
(d). none
Show Answer
Answer: (b)7. The number of values of $\theta \in[0,4 \pi]$ satisfying the equation $|\sqrt{3} \cos x-\sin x| \geq 2$ is
(a). 0
(b). 2
(c). 4
(d). 8
Show Answer
Answer: (c)8. If $\frac{1}{6} \sin \theta, \cos \theta, \tan \theta$ are in G.P., then $\theta$ equals
(a). $2 \mathrm{n} \pi \pm \frac{\pi}{3}$
(b). $2 \mathrm{n} \pi \pm \frac{\pi}{6}$
(c). $ n \pi+(-1)^{n} \frac{\pi}{3}$
(d). $n \pi+\frac{\pi}{3}$
Show Answer
Answer: (a)9. The number of solutions of the equation $\tan x+\sec x=2 \cos x$ lying in the internal $[0,2 \pi]$ is
(a). 0
(b). 1
(c). 2
(d). 3
Show Answer
Answer: (c)10. The general solution of equation $\sin ^{2} \theta \sec \theta+\sqrt{3} \tan \theta=0$ is
(a). $ \theta=\mathrm{n} \pi+(-1)^{\mathrm{n}+1} \frac{\pi}{3}$
(b). $ \theta=\mathrm{n} \pi$
(c). $ \theta=\mathrm{n} \pi+(-1)^{\mathrm{n}+1} \frac{\pi}{6}$
(d). $\theta=\frac{\mathrm{n} \pi}{2}$
Show Answer
Answer: (b)11. Passage
Solution of equations asin $x \pm b \cos x=c$.
General value satisfying two equations. $a \cos \theta \pm \mathrm{b} \sin \theta=\mathrm{c}, \theta$ satisfying two equations.
(a). The equation acos $\theta \pm \mathrm{b} \sin \theta=\mathrm{c}$ be first converted to $\mathrm{r} \cos (\theta \pm \alpha)=\mathrm{c}$ where $\mathrm{a}=\mathrm{r\cos} \alpha, \mathrm{b}=\mathrm{r\sin} \alpha$
(b). $\theta$ satisfying two equations
Find the common value of $\theta$ lying between 0 and $2 \pi$ and then add $2 n \pi$
Answer the following questions based upon above passage
(i). The number of intergral values of $k$ for which the equation $7 \cos x+5 \sin x=2 k+1$ has a solution is
(a). 4
(b). 8
(c). 10
(d). 12
Show Answer
Answer: (b)(ii). If $\cos 3 x+\sin \left(2 x-\frac{7 \pi}{6}\right)=-2$ then $x$ equals
(a). $\frac{\pi}{3}(6 m-1)$
(b). $\frac{\pi}{3}(6 m+1)$
(c). $ \frac{\pi}{3}(2 \mathrm{~m}+1)$
(d). none
Show Answer
Answer: (b)(iii). The values of $\mathrm{x}$ such that $-\pi<\mathrm{x}<\pi$ and satisfying the equation are given by $8^{\left[1+\left|\cos x^{x}+\cos ^{2} x+\cos ^{3} x\right|+\ldots+\operatorname{tos}\right]}=4^{3} x$ equals
(a). $\frac{\pi}{3}$
(b). $\frac{2 \pi}{3}$
(c). $\frac{-\pi}{3}$
(d). $\frac{-2 \pi}{3}$
Show Answer
Answer: (a, b, c, d)(iv). The number of solution of the equaiton $\mathrm{e}^{\sin x}-\mathrm{e}^{-\sin x}-4=0$ is
(a). 1
(b). 2
(c). 4
(d). 0










