STATISTICS
Measures of Central Tendency (Averages)
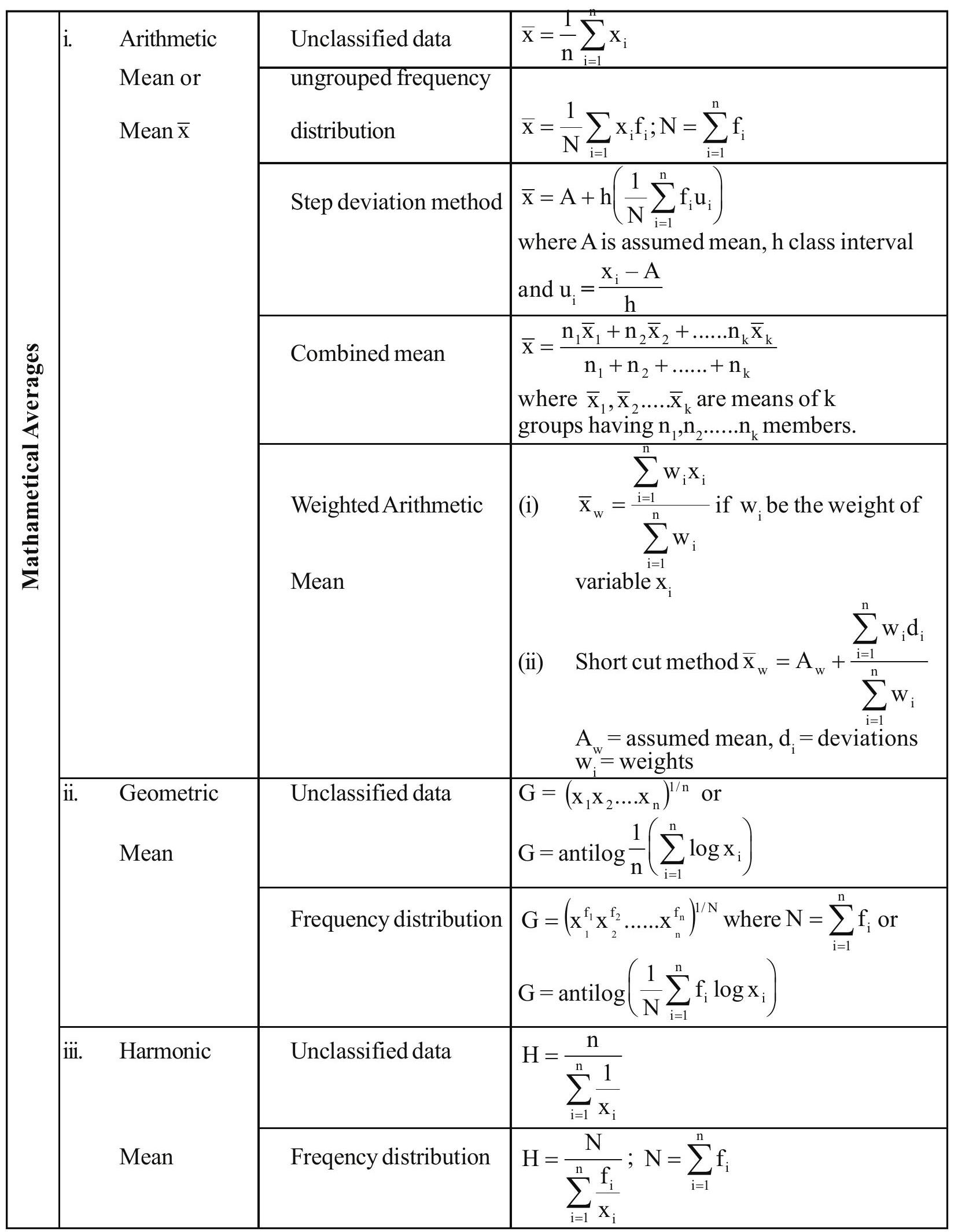

Note: Like median, the other partition values quartiles, deciles, percentiles etc can be determined.
i$^{\text{th}}$ quartile $Q _{i}$ is given by $Q _{i}=\ell+\frac{\frac{i N}{4}-c f}{f} \times h ; i=1,2,3$
Where symbols have same meanings as in median. Clearly, the second quartile $\mathrm{Q} _{2}$ is median.
First quartile is called lower quartile and the third quartile is upper quartile.
Measures of dispersion
The degree to which numerical data tend to spread about an average value is called variation or dispersion of the data. It measures the scatterendness of various observation about some central value.
| Range | Difference of the largest and smallest values | |
|---|---|---|
| Quartile Deviation Coefficient of quartile deviation | $\frac{Q _{3}-Q _{1}}{2}$ $\frac{Q _{3}-Q _{1}}{Q _{3}+Q _{1}}$ where $Q _{1}, Q _{3}$ are respectively the first & third quartiles. |
|
| Mean Devialion $(\delta)$ | Unclassified data | $\frac{1}{n} \sum_{i=1}^n\mid x-a\mid \text { where } a=A . M$ Median or Mode as the case may be |
| (M.D is least when measured from the median) | Ungrouped frequency distributon | M.D $=$ $\frac{1}{n} \sum _{i=1}^{n} f _{i}\left|x _{i}-a\right|$ where $a=A . M,$ Median or Mode as the case may be & $N=\sum _{i=1}^{n} f _{i}$ |
| Grouped frequency distribution |
M.D $=\frac{1}{n} \sum _{i=1}^{n} f _{i}\left|x _{i}-a\right|$ where $a=A . M$ Median or Mode as the case may be $\&$ $\mathrm{~N}=\sum _{\mathrm{i}=1}^{\mathrm{n}} \mathrm{f} _{\mathrm{i}}$ |
|
| Standard |
Unclassified data | $\left.\sigma=\sqrt{\frac{1}{n} \sum _{i=1}^{n}\left(x _{i}-\bar{x}\right)^{2}}=\sqrt[1]{\frac{1}{n} \sum _{i=1}^{n} x _{i}^{2}-\left(\frac{\sum _{i=1}^{n} x _{i}}{n}\right.}\right)^2$ |
| Deviation $(\sigma)$ | Ungrouped frequency distribution | $\sigma=\sqrt{\frac{1}{\mathrm{~N}}} \sum_ {\mathrm{i}=1}^{\mathrm{n}} \mathrm{f}_ {\mathrm{i}}\left(\mathrm{x}_ {\mathrm{i}}-\overline{\mathrm{x}}\right)^2$ $\begin{aligned} & \sigma=\sqrt{\frac{1}{N} \sum_{i=1}^n f_i x_i^2-\left(\frac{\sum_{i=1}^n f_i x_i}{N}\right)^2} \text { where } \\ & N=\sum_{i=1}^n f_i\end{aligned}$ |
| Grouped frequency | $\sigma=\mathrm{h} \sqrt{\frac{1}{\mathrm{~N}} \sum _{\mathrm{i}=1}^{\mathrm{n}} \mathrm{f} _{\mathrm{i}} \mathrm{u} _{\mathrm{i}}^{2}-\left(\frac{\sum _{\mathrm{i}=1}^{\mathrm{n}} \mathrm{f} _{\mathrm{i}} \mathrm{u} _{\mathrm{i}}}{\mathrm{N}}\right)^{2}}$ | |
| distribution | where, $\mathrm{N}=\sum _{\mathrm{i}=1}^{\mathrm{n}} \mathrm{f} _{\mathrm{i}} \& \mathrm{u} _{\mathrm{i}}=\frac{\mathrm{x} _{\mathrm{i}}-\mathrm{A}}{\mathrm{h}}$ $\mathrm{A} \rightarrow$ assumed mean $\mathrm{h} \rightarrow$ class interval |
|
| Root mean square | Unclassified data | $\mathrm{s}=\sqrt{\frac{1}{\mathrm{n}} \sum _{\mathrm{i}=1}^{\mathrm{n}}\left(\mathrm{x} _{\mathrm{i}}-\mathrm{a}\right)^{2}} ; \mathrm{a}$ is asumed mean |
| Ungrouped frequency distribution | $\mathrm{s}=\sqrt{\frac{1}{\mathrm{~N}} \sum _{\mathrm{i}=1}^{\mathrm{n}} \mathrm{f} _{\mathrm{i}}\left(\mathrm{x} _{\mathrm{i}}-\mathrm{a}\right)^{2}} ; \mathrm{N}=\sum _{\mathrm{i}=1}^{\mathrm{n}} \mathrm{f} _{\mathrm{i}}$ | |
| Grouped frequency distribution | $\mathrm{s}=\sqrt{\frac{1}{\mathrm{~N}} \sum _{\mathrm{i}=1}^{\mathrm{n}} \mathrm{f} _{\mathrm{i}}\left(\mathrm{x} _{\mathrm{i}}-\mathrm{a}\right)^{2}} ; \mathrm{N}=\sum _{\mathrm{i}=1}^{\mathrm{n}} \mathrm{f} _{\mathrm{i}}$ |
Note that $\mathrm{s}^{2}=\sigma^{2}+\mathrm{d}^{2}$ where $\mathrm{d}=\overline{\mathrm{x}}-\mathrm{a}$
Clearly s is least when $\mathrm{d}=0$ i.e. $\overline{\mathrm{x}}-\mathrm{a}$
i.e. root mean square devian is least when deviations are taken from $\bar{x}$.
Note: median can be determined from graph also. It is the abscissa of the point of intersection of “less than” ogive and “more than” ogive.
Note: i. The algebraic sum of the deviations of all the values of the variable from their mean is zero.
i.e. $\sum\left(\mathrm{x} _{\mathrm{i}}-\overline{\mathrm{x}}\right)=0$, for ungrouped distribution and $\sum \mathrm{f} _{\mathrm{i}}\left(\mathrm{x} _{\mathrm{i}}-\overline{\mathrm{x}}\right)=0$, for grouped distribution.
ii. The sum of the squares of the deviations of the variable is minimum when taken about arithmetic mean.
iii. Let $\mathrm{x} \& \mathrm{y}$ be two variables, $\mathrm{b}, \mathrm{c}$ two constants and $\mathrm{u}=\mathrm{bx}+\mathrm{cy}$. Then $\overline{\mathrm{u}}=\mathrm{b} \overline{\mathrm{x}}+\mathrm{cy}$ where $\bar{x} \& \bar{y}$ are A.M.S of $x _{i}{ }^{\prime} s \& y _{i}$ ’s, when $b=c=1, u=x+y \& \bar{u}=\bar{x}+\bar{y}$
i.e. The mean of sum of two variables is equal to sum of their means (it is true for more than two variables also)
Note :
i. Standard deviation is independent of shift of origin but depends upon change of scale.
i.e. if $y=\frac{x}{h}$, then $\sigma _{y}=\frac{\sigma x}{|h|}$
ii. Square of S.D., ie. $\sigma^{2}$ is called the variance.
iii. Coefficient of variation c.v. $=\frac{\sigma}{\overline{\mathrm{x}}} \times 100$.
The distribution for which the coefficent of variance is less is more consistent.
iv. S.D. $\sigma$ of the combined group of two groups having means $\bar{x} _{1}, \overline{\mathrm{x}} _{2} ;$ standard deviations $\sigma _{1}, \sigma _{2}$ and number of elements $n _{1}, n _{2}$ is given by
$\sigma^{2}=\frac{1}{\mathrm{n} _{1}+\mathrm{n} _{2}}\left(\mathrm{n} _{1}\left(\sigma _{1}^{2}+\mathrm{d} _{1}^{2}\right)+\mathrm{n} _{2}\left(\sigma _{2}^{2}+\mathrm{d} _{2}^{2}\right)\right)$ where $\mathrm{d} _{1}=\overline{\mathrm{x}} _{1}-\overline{\mathrm{x}}$ and $\mathrm{d} _{2}=\overline{\mathrm{x}} _{2}-\overline{\mathrm{x}}$
$\overline{\mathrm{x}}=\frac{\mathrm{n} _{1} \overline{\mathrm{x}} _{1}+\mathrm{n} _{2} \overline{\mathrm{x}} _{2}}{\mathrm{n} _{1}+\mathrm{n} _{2}}$ (combined mean)
Also $\sigma^{2} \leq(\text { Range })^{2}$
Symmetric and skew distribution
In a symmetrical distribution, mean, median and mode coincide. Here frequencies are symmetrically distributed on both sides of the central value. (ie. same number of frequencies are distributed at the same linear distance on either side of mode). The frequency curve is bell-shaped and mean = median = mode

In a skew distribution, the variation doesnot have symmetry.

Note: In a moderately skewed distribution, Mean $-$ Mode $=3($ Mean $-$ Median $)$
Solved examples
1. In any disrete series when all values are not same, the relation between M.D about mean and S.D is
(a). M.D $=$ S.D
(b). M.D $\geq$ S.D
(c). M.D $<$ S.D
(d). M.D $>$ S.D
Show Answer
Solution:
For a distribution $\left(\mathrm{x} _{\mathrm{i}}, \mathrm{f} _{\mathrm{i}}\right) \mathrm{i}=1,2, \ldots . \mathrm{n}$
$\sigma _{\mathrm{x}}^{2}=\frac{1}{\mathrm{~N}} \sum \mathrm{f} _{\mathrm{i}}\left(\mathrm{x} _{\mathrm{i}}-\overline{\mathrm{x}}\right)^{2} \& \mathrm{M} . \mathrm{D}=\frac{1}{\mathrm{~N}} \sum \mathrm{f} _{\mathrm{i}}\left|\mathrm{x} _{\mathrm{i}}-\overline{\mathrm{x}}\right|$
$\therefore \quad \sigma _{\mathrm{x}}^{2}-(\mathrm{M} . \mathrm{D})^{2}=\frac{1}{\mathrm{~N}} \sum \mathrm{f} _{\mathrm{i}} \mathrm{d} _{\mathrm{i}}{ }^{2}-\left(\frac{1}{\mathrm{~N}} \sum \mathrm{f} _{\mathrm{i}} \mathrm{d} _{\mathrm{i}}\right)^{2}$ where $\mathrm{d} _{\mathrm{i}}=\mathrm{x} _{\mathrm{i}}-\overline{\mathrm{x}}$
$=\quad \sigma _{\mathrm{d}}^{2}>0$
$\Rightarrow \quad \sigma _{\mathrm{x}}^{2}>(\text { M.D. })^{2}$
$\Rightarrow S.D. >M.D$
Answer: c
2. The A.M. of $n$ observation is $\bar{x}$. If the sum of $n-5$ observations is $a$, then the mean of remaining 5 observation is
(a). $\frac{\mathrm{n} \overline{\mathrm{x}}+\mathrm{a}}{5}$
(b). $\frac{\mathrm{n} \overline{\mathrm{x}}-\mathrm{a}}{5}$
(c). $n \bar{x}+a$
(d). none of these
Show Answer
Solution:
If $m$ is the mean of 5 observations, then
$\bar{x}=\frac{(n-5) \frac{a}{n-5}+5 m}{n-5+5}\left(\because \bar{x}=\frac{n _{1} \bar{x} _{1}+n _{2} \bar{x} _{2}}{n _{1}+n _{2}}\right)$
$\Rightarrow \mathrm{n} \overline{\mathrm{x}}=\mathrm{a}+5 \mathrm{~m}$
$\Rightarrow \mathrm{m}=\frac{\mathrm{n} \overline{\mathrm{x}}-\mathrm{a}}{5}$
Answer: b
3. If $\overline{\mathrm{x}} _{1}$ and $\overline{\mathrm{x}} _{2}$ are means of two distributions such that $\overline{\mathrm{x}} _{1}<\overline{\mathrm{x}} _{2}$ and $\overline{\mathrm{x}}$ is the combined mean, then
(a). $\overline{\mathrm{x}}<\overline{\mathrm{x}} _{1}$
(b). $\overline{\mathrm{x}}>\overline{\mathrm{x}} _{2}$
(c). $\overline{\mathrm{x}}=\frac{\overline{\mathrm{x}} _{1}+\overline{\mathrm{x}} _{2}}{2}$
(d). $\overline{\mathrm{x}} _{1}<\overline{\mathrm{x}}<\overline{\mathrm{x}} _{2}$
Show Answer
Solution:
If $\mathrm{n} _{1} \& \mathrm{n} _{2}$ are the number of items in two distributions having means $\overline{\mathrm{x}} _{1} \& \overline{\mathrm{x}} _{2}$.
$\overline{\mathrm{x}}=\frac{\mathrm{n} _{1} \overline{\mathrm{x}} _{1}+\mathrm{n} _{2} \overline{\mathrm{x}} _{2}}{\mathrm{n} _{1}+\mathrm{n} _{2}}$
$\therefore \quad \overline{\mathrm{x}}-\overline{\mathrm{x}} _{1}=\frac{\mathrm{n} _{1} \overline{\mathrm{x}} _{1}+\mathrm{n} _{2} \overline{\mathrm{x}} _{2}}{\mathrm{n} _{1}+\mathrm{n} _{2}}-\overline{\mathrm{x}} _{1}=\frac{\mathrm{n} _{2}\left(\overline{\mathrm{x}} _{2}-\overline{\mathrm{x}} _{1}\right)}{\mathrm{n} _{1}+\mathrm{n} _{2}}>0 \quad\left(\because \overline{\mathrm{x}} _{2}>\overline{\mathrm{x}} _{1}\right)$
$\Rightarrow \quad \overline{\mathrm{x}}>\overline{\mathrm{x}} _{1}$
Similary, $\overline{\mathrm{x}}-\overline{\mathrm{x}} _{2}=\frac{\mathrm{n} _{1}\left(\overline{\mathrm{x}} _{1}-\overline{\mathrm{x}} _{2}\right)}{\mathrm{n} _{1}+\mathrm{n} _{2}}<0$
$\Rightarrow \quad \overline{\mathrm{x}}<\overline{\mathrm{x}} _{2}$
$\therefore \quad \overline{\mathrm{x}} _{1}<\overline{\mathrm{x}}<\overline{\mathrm{x}} _{2}$
Answer: d
4. The mean of $\frac{{ }^{50} \mathrm{C} _{0}}{1}, \frac{{ }^{50} \mathrm{C} _{2}}{3}, \frac{{ }^{50} \mathrm{C} _{4}}{5} \ldots . . \frac{{ }^{50} \mathrm{C} _{50}}{51}$ is
(a). $\frac{2^{50}}{51}$
(b). $\frac{2^{49}}{51}$
(c). $\frac{2^{49}}{39 \times 17}$
(d). none of these
Show Answer
Solution:
$(1+x)^{50}+(1-x)^{50}=2\left\{\left\{^{50} \mathrm{C} _{0}+{ }^{50} \mathrm{C} _{2} \mathrm{x}^{2} \ldots \ldots+{ }^{50} \mathrm{C} _{50} \mathrm{x}^{50}\right\}\right.$
Integrating with limits 0 to 1
$\left.2\left\{{ }^{50} \mathrm{C} _{0} \mathrm{x}+{ }^{50} \mathrm{C} _{2} \frac{\mathrm{x}^{3}}{3}+\ldots .+{ }^{50} \mathrm{C} _{50} \frac{\mathrm{x}^{51}}{51}\right\} _{0}^{1}=\frac{(1+\mathrm{x})^{51}}{51}-\frac{(1+\mathrm{x})^{51}}{51}\right] _{0}^{1}$
${ }^{50} \mathrm{C} _{0}+\frac{{ }^{50} \mathrm{C} _{2}}{3}+\ldots \ldots . .+\frac{{ }^{50} \mathrm{C} _{50}}{51}=\frac{1}{2} \cdot \frac{2^{51}}{51}=\frac{2^{50}}{51}$
Mean $=\frac{2^{50}}{51 \times 26}=\frac{2^{49}}{39 \times 17}$
Answer: c
Practice questions
1. Mean of $n$ terms is $\bar{x}$. If these $x$ items are successively increased by $2,2^{2}, 2^{3}, \ldots \ldots . .2^{n}$, then the new mean is
(a). $\overline{\mathrm{x}}+\frac{2^{\mathrm{n}+1}}{\mathrm{n}}$
(b). $\bar{x}+\frac{2^{n+1}}{n}-\frac{2}{n}$
(c). $\overline{\mathrm{x}}+\frac{2^{\mathrm{n}}}{\mathrm{n}}$
(d). none of these
Show Answer
Answer: (b)2. The weighted A.M. of first $n$ natural number whose weights are equal is
(a). $\frac{\mathrm{n}+1}{2}$
(b). $\frac{2 \mathrm{n}+1}{2}$
(c). $\frac{2 \mathrm{n}+1}{3}$
(d). $\frac{(2 n+1)(n+1)}{6}$
Show Answer
Answer: (a)3. If $\mathrm{G}$ is the G.M. of the product of $\mathrm{k}$ sets of observations, with G.M.’s $\mathrm{G} _{1}, \mathrm{G} _{2} \ldots . . . \mathrm{G} _{\mathrm{k}}$ respectively, then $\mathrm{G}$ is equal to
(a). $\log \mathrm{G} _{1}+\log \mathrm{G} _{2}+\ldots \ldots . .+\log \mathrm{G} _{\mathrm{k}}$
(b). $\log \mathrm{G} _{1}+\log \mathrm{G} _{2} \ldots \ldots . .+\log \mathrm{G} _{\mathrm{k}}$
(c). $\mathrm{G} _{1} \mathrm{G} _{2} \ldots \ldots \mathrm{G} _{\mathrm{k}}$
(d). none of these
Show Answer
Answer: (c)4. The mean square deviation of $n$ observations $\mathrm{x} _{1}, \mathrm{x} _{2}, \ldots \ldots \mathrm{x} _{\mathrm{n}}$ about $-2$ and $2$ are $18$ and $10$ respectively. Then, S.D of the given set is
(a). 1
(b). 2
(c). 3
(d). 4
Show Answer
Answer: (c)5. A car owner buys petrol at ₹ 7.50, ₹ 8.00 and ₹ 8.50 per litre for the 3 successive years. If he spends ₹ 4,000 each year, then the average cost per litre of petrol is
(a). ₹ 8
(b). ₹ 8.25
(c). ₹ 7.98
(d). none of these
Show Answer
Answer: (c)6. The mean of the values $0,1,2, \ldots \ldots, n$ with the corresponding weights ${ }^{n} C _{0},{ }^{n} C _{1}, \ldots . .{ }^{n} C _{n}$ repectively is
(a). $\frac{2^{\mathrm{n}}}{\mathrm{n}+1}$
(b). $\frac{2^{\mathrm{n}+1}}{\mathrm{n}(\mathrm{n}+1)}$
(c). $\frac{\mathrm{n}+1}{2}$
(d). $\frac{n}{2}$
Show Answer
Answer: (d)7. The quartile deviation of daily wages (in Rs.) of 7 persons is given below:
$12, 7, 15, 10, 17, 17, 25$ is
(a). 14.5
(b). 3.5
(c). 9
(d). 4.5
Show Answer
Answer: (b)8. If a variable $x$ takes values $x _{i}$ such that $a \leq x _{i} \leq b$, for $i=1,2, \ldots,n ,$ Then
(a). $ \mathrm{a} \leq \operatorname{var}(\mathrm{x}) \leq \mathrm{b}$
(b). $ \mathrm{a}^{2} \leq \operatorname{var}(\mathrm{x}) \leq \mathrm{b}^{2}$
(c). $ \frac{\mathrm{a}^{2}}{4} \leq \operatorname{var}(\mathrm{x})$
(d). $(b-a)^{2} \geq \operatorname{var}(x)$
Show Answer
Answer: (d)9. For $(2n+1)$ observations $\mathrm{x} _{1},-\mathrm{x} _{1}, \mathrm{x} _{2},-\mathrm{x} _{2}, \ldots, \mathrm{x} _{\mathrm{n}},-\mathrm{x} _{\mathrm{n}}$ and 0 , where $\mathrm{x} _{\mathrm{i}}{ }^{\prime}$ s are different, let S.D. and M.D. denote standard deviation and mean deviaiton about median, then which is true
(a). S.D. $<$ M.D.
(b). S.D $>$ M.D.
(c). S.D. $=$ M.D.
(d). nothing can be said in general
Show Answer
Answer: (b)10. The $A M$ and variance of 10 observations are 10 and 4 respectively. Later it is discovered that one observation was incorrectly read as 8 instead of 18 . Then, the correct value of mean and variance are
(a). 20, 9
(b). 20, 14
(c). 11, 9
(d). 11, 5
Show Answer
Answer: (b)11. In a frequency distribution, the mean and median are 21 and 22 respectively, then its mode is approximately
(a). 25.5
(b). 24.0
(c). 22.0
(d). 20.5
Show Answer
Answer: (b)12. For a symmetrical distribution $\mathrm{Q} _{1}=20$ and $\mathrm{Q} _{3}=40$, the median of the data is
(a). 20
(b). 30
(c). 40
(d). 10
Show Answer
Answer: (b)13. If the mean deviation of $1,1+\mathrm{d}, 1+2 \mathrm{~d}, \ldots \ldots, 1+100 \mathrm{~d}$ from their mean is 255 , then $\mathrm{d}=$
(a). 20.0
(b). 10.1
(c). 20.2
(d). 10.0
Show Answer
Answer: (b)14. The mean value of $\frac{{ }^{30} \mathrm{C} _{0}}{1}, \frac{{ }^{30} \mathrm{C} _{2}}{3} \ldots . ., \frac{{ }^{30} \mathrm{C} _{20}}{21}, \frac{{ }^{30} \mathrm{C} _{21}}{22}, \ldots \ldots . . \frac{{ }^{30} \mathrm{C} _{30}}{31}$ is
(a). $\frac{2^{31}}{31}$
(b). $\frac{4^{31}}{31}$
(c). $\frac{2^{13}}{31}$
(d). none of these
Show Answer
Answer: (b)15. Read the paragraph and answer the questions that follow:
If $x _{1}, x _{2}, x _{3}$ are $n$ values of the variable $x$, then mathematical averages. Arithmetic Mean (A.M.), Geometric Mean (G.M.) and Harmonic Mean (H.M.) are count by the following formula’s.
A.M. $=\frac{\mathrm{x} _{1}+\mathrm{x} _{2}+\mathrm{x} _{3}+\ldots .+\mathrm{x} _{\mathrm{n}}}{\mathrm{n}}=\frac{1}{\mathrm{n}}\left\{\sum _{\mathrm{i}=1}^{\mathrm{n}} \mathrm{x} _{\mathrm{i}}\right\} \forall \mathrm{x} _{\mathrm{i}}>0$
G.M. $=\left(\mathrm{x} _{1} \cdot \mathrm{x} _{2} \ldots . \mathrm{x} _{\mathrm{n}}\right)^{1 / \mathrm{n}}$, if each $\mathrm{x} _{\mathrm{i}}(\mathrm{i}=1,2, \ldots ., \mathrm{n})$ is positive and
H.M. $=\frac{\mathrm{n}}{\frac{1}{\mathrm{x} _{1}}+\frac{1}{\mathrm{x} _{2}}+\ldots \ldots . \frac{1}{\mathrm{x} _{\mathrm{n}}}}=\frac{1}{\frac{1}{\mathrm{n}} \sum _{\mathrm{i}=1}^{\mathrm{n}}\left(\frac{1}{\mathrm{x} _{\mathrm{i}}}\right)}$
-
In case of frequency distribution $\mathrm{x} _{\mathrm{i}} / \mathrm{f} _{\mathrm{i}}(\mathrm{i}=1,2, \ldots . \mathrm{n})$ where $\mathrm{f} _{\mathrm{i}}$ is the frequency of the variable $\mathrm{x} _{\mathrm{i}}$, then the claculation of A.M. is counted as A.M. $=\sum _{i=1}^{n} f _{i} x _{i} / N _{\text {where }} N=f _{1}+f _{2} \ldots . .+f _{n}$ If $\mathrm{w} _{1}, \mathrm{w} _{2} \ldots ., \mathrm{w} _{\mathrm{n}}$ be the weight assigned to the $\mathrm{n}$ values $\mathrm{x} _{1}, \mathrm{x} _{2}, \ldots \ldots \mathrm{x} _{\mathrm{n}}$ then weighted $\mathrm{A}$.M. is counted by $\frac{\sum _{i=1}^{n} w _{i} x _{i}}{\sum _{i=1}^{n} w _{i}}$
-
If $\mathrm{G} _{1}, \mathrm{G} _{2}$ are the G.M’s of two series of sizes $\mathrm{n} _{1} \& \mathrm{n} _{2}$ respectively, then the geometric mean (G.M.) of the combined series is counted by $\log \left(\right.$ G.M.) $=\frac{n _{1} \log G _{1}+n _{2} \log G _{2}}{n _{1}+n _{2}}$. On the basis of above information answer the following questions.
1. If the mean of a set of observations $\mathrm{x} _{1}, \mathrm{x} _{2} \ldots \ldots, \mathrm{x} _{2} \ldots \ldots, \mathrm{x} _{\mathrm{n}}$ is $\overline{\mathrm{x}}$ then the mean of observations $\mathrm{x} _{\mathrm{i}}+4 \mathrm{i}$, $\mathrm{i}=1,2,3$, $\mathrm{n}$ is
(a). $\overline{\mathrm{x}}+2(\mathrm{n}+1)$
(b). $\bar{x}+4(n+1)$
(c). $\overline{\mathrm{x}}+4 \mathrm{n}$
(d). $\overline{\mathrm{x}}+\mathrm{n}$
Show Answer
Answer: (a)2. The A.M. of $n$ numbers of series is $\bar{x}$. If the sum of first $(n-1)$ terms is $m$ then $n^{\text {th }}$ number is
(a). $\overline{\mathrm{x}}-\mathrm{m}$
(b). $n \bar{x}-m$
(c). $\overline{\mathrm{x}}-\mathrm{mn}$
(d). $n \bar{x}-n m$
Show Answer
Answer: (b)3. The mean of a set of numbers is $\bar{x}$. If each number is divided by 4 then new mean is
(a). $\overline{\mathrm{x}}$
(b). $\overline{\mathrm{x}}+4$
(c). $\frac{\bar{x}}{4}$
(d). $4 \overline{\mathrm{x}}$
Show Answer
Answer: (c)4. The weighted A.M. of first $n$ natural numbers whose weights are squares the corresponding numbers, is equal to
(a). $\frac{\mathrm{n}(\mathrm{n}+1)}{2}$
(b). $ \frac{3}{2} \frac{2 \mathrm{n}+1}{\mathrm{n}(\mathrm{n}+1)}$
(c). $ \frac{3}{2} n(n+1)$
(d). $ \frac{3}{2} \frac{\mathrm{n}(\mathrm{n}+1)}{2 \mathrm{n}+1}$
Show Answer
Answer: (d)5. Consider the series $1,4,16,64,256, \ldots \ldots .4^{\mathrm{n}}$, then which of the following is not true?
(a). A.M. $=\frac{4^{\mathrm{n}+1}-1}{3(\mathrm{n}+1)}$
(b). $G.M.=2^{\mathrm{n}}$
(c). H.M. $=\frac{3.4^{\mathrm{n}}(\mathrm{n}+1)}{4^{\mathrm{n}+1}-1}$
(d). A.M=G.M.=H.M.
Show Answer
Answer: (d)6. Let $\mathrm{G}$ be the G.M. of the product of ( $\mathrm{r}+1)$ sets of observation with, G.M., $\mathrm{G} _{1}, \mathrm{G} _{2}, \ldots . . \mathrm{G} _{\mathrm{r}}, \mathrm{G} _{\mathrm{r}+1}$ respectively, then the vaue of $G$ is
(a). $ \sum _{\mathrm{i}=1}^{\mathrm{r}+1} \log \left(\mathrm{G} _{\mathrm{i}}\right)$
(b). $ \prod _{\mathrm{i}=1}^{\mathrm{r}+1} \mathrm{G} _{\mathrm{i}}$
(c). $ \prod _{\mathrm{i}=1}^{\mathrm{r}+1} \log \left(\mathrm{G} _{\mathrm{i}}\right)$
(d). none of these
Show Answer
Answer: (b)7. The mean value of $\frac{{ }^{20} \mathrm{C} _{0}}{1}, \frac{{ }^{20} \mathrm{C} _{2}}{3}, \frac{{ }^{20} \mathrm{C} _{4}}{5}, \frac{{ }^{20} \mathrm{C} _{6}}{7}, \ldots \ldots . . . \frac{{ }^{20} \mathrm{C} _{20}}{21}$, equals
(a). $\frac{2^{20}}{3 \times 77}$
(b). $\frac{2^{19}}{3 \times 77}$
(c). $\frac{2^{20}}{21}$
(d). $\frac{2^{19}}{21}$
Show Answer
Answer: (a)8. Let $\mathrm{x}$ be the variate which assumes the values $0,1,2,3,4, \ldots \ldots \mathrm{n}$ with frequencies $\mathrm{q}^{\mathrm{n}},{ }^{\mathrm{n}} \mathrm{C} _{1} \mathrm{pq}^{\mathrm{n-1}},{ }^{\mathrm{n}} \mathrm{C} _{1} \mathrm{p}^{2} \mathrm{q}^{\mathrm{n}-1}$ is
(a). $n p q$
(b). $\mathrm{np}$
(c). $\sqrt{\mathrm{npq}}$
(d). $ n^{2} p^{2} q^{2}$
Show Answer
Answer: (b)9. The mean of the divisors of 360 which are odd is
(a). 15
(b). 11
(c). 13
(d). 9
Show Answer
Answer: (c)10. The ratio of the mean of the cubes of first $n$ natural numbers to the means of the cubes of first $(\mathrm{n}+1)$ natural nubers is given by
(a). $(\mathrm{n}+1):(\mathrm{n}+2)$
(b). $(\mathrm{n}+1)^{2}: \mathrm{n}+2$
(c). $\mathrm{n}(\mathrm{n}+1):(\mathrm{n}+2)^{2}$
(d). none of these










