Equation of a Hyperbola referred to two perpendicular lines

Let equation of hyperbola be

$\frac{{\mathrm{x}}^2}{{\mathrm{a}}^2}-\frac{{\mathrm{y}}^2}{{\mathrm{b}}^2}=1$

From diagram $\mathrm{PM}=\mathrm{y}$ and $\mathrm{PN}=\mathrm{x}$

$\frac{{\mathrm{PN}}^2}{{\mathrm{a}}^2}-\frac{{\mathrm{PM}}^2}{{\mathrm{b}}^2}=1$

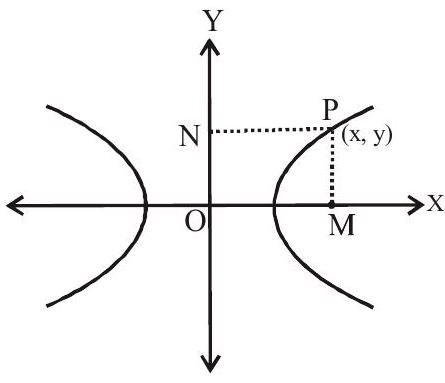
ie. if perpendicular distance of a point $P(x,y)$ from two mutually perpendicular lines say ${\ell }_1=a_{1}x+b_{1}y+c_1=0$ and ${\ell }_2={\mathrm{a}}_2\mathrm{x}+{\mathrm{b}}_2\mathrm{y}+{\mathrm{c}}_2=0$ then

$\frac{\left(\frac{a_{1}x+b_{1}y+c_1}{\sqrt{a_1^2+b_1^2}}\right)}{a^2}-\frac{\left(\frac{a_{2}x+b_{2}y+c_2}{\sqrt{a_2^2+b_2^2}}\right)}{b^2}=1$

then the locus of point $\mathrm{P}$ denotes a hyperbola

• centre of the hyperbola, we get after solving ${\ell }_1=0$ and ${\ell }_2=0$

• Transverse axis : $\ell =0$

• Conjugate axis $:{\ell }_1=0$

• Foci : The foci of the hyperbola is the point of intersection of the lines $\frac{a_{1}x+b_{1}y+c_1}{\sqrt{a_1^2+b_1^2}}=\pm ae$ and ${\ell }_2=0$

• Directrix: $\frac{a_{1}x+b_{1}y+c_1}{\sqrt{a_1^2+b_1^2}}=\pm \frac{a}{e}$

• Length of transverse axis $=2\mathrm{a}$

• Length of conjugate axis $=2b$

• Length of latus Rectum $=\frac{2b^2}{a}$

Examples

1. Find the eccentricity and centre of the hyperbola

$\frac{(3x-4y-12{)}^2}{100}-\frac{(4x+3y-12{)}^2}{225}=1$

2. Find the eccentricity of the conic $4(2y-x-3{)}^2-9(2x+y-1{)}^2=80$

3. Find the coordinates of the centre, foci and vertices, length of axes and latus rectum, equation of axes and directries, and eccentricity of the conic $9x^2-16y^2-18x+32y-151=0$

4. The equation of the transverse and conjugate axes of a hyperbola are respectively $3x+4y-7=0$, $4x-3y+8=0$ and their respective lengths are 4 and 6 . The equation of the hyperbola is

(a). $17x^2+312xy+108y^2-634x-312y-715=0$

(b). $108x^2+312xy+17y^2-312x-634y-715=0$

(c). $108x^2-312xy+17y^2-312x-634y-715=0$

(d). none of these

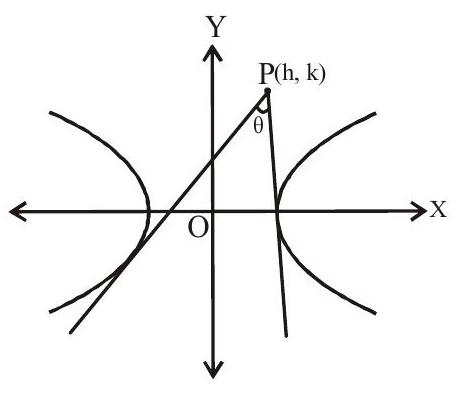
Line and Hyperbola

Let equation of line be $y=mx+c$ and equation of hyperbola be $\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$

$\begin{array}{rl}& \frac{x^2}{a^2}-\frac{(mx+c{)}^2}{b^2}=1\\ & \frac{x^2}{a^2}-\frac{m^2{x}^2}{b^2}-\frac{c^2}{b^2}-\frac{2mc}{b^2}x-1=0\\ & x^2(\frac{1}{a^2}-\frac{m^2}{b^2})-\frac{2mc}{b^2}x-\left(\frac{c^2+b^2}{b^2}\right)=0\\ & x^2(\frac{b^2}{a^2}-m^2)-2mcx-(c^2+b^2)=0\end{array}$

$\therefore \mathrm{D}=4{\mathrm{m}}^2{\mathrm{c}}^2+4\cdot \left(\frac{{\mathrm{b}}^2-{\mathrm{a}}^2{\mathrm{m}}^2}{{\mathrm{a}}^2}\right)({\mathrm{c}}^2+{\mathrm{b}}^2)=4({\mathrm{m}}^2{\varphi }^2+\frac{{\mathrm{b}}^2}{{\mathrm{a}}^2}{\mathrm{c}}^2-{\mathrm{m}}^2{\varphi }^2+\frac{{\mathrm{b}}^4}{{\mathrm{a}}^2}-{\mathrm{m}}^2{\mathrm{b}}^2)=4{\mathrm{b}}^2\left(\frac{{\mathrm{c}}^2+{\mathrm{b}}^2-{\mathrm{a}}^2{\mathrm{m}}^2}{{\mathrm{a}}^2}\right)$

i. $\mathrm{D}<0$ i.e. ${\mathrm{c}}^2-{\mathrm{a}}^2{\mathrm{m}}^2+{\mathrm{b}}^2<0$ line do not intersect hyperbola .

ii. $\mathrm{D}=0$ i.e. ${\mathrm{c}}^2-{\mathrm{a}}^2{\mathrm{m}}^2+{\mathrm{b}}^2=0$ line touches the hyperbola .

iii. $\mathrm{D}>0$ i.e. ${\mathrm{c}}^2-{\mathrm{a}}^2{\mathrm{m}}^2+{\mathrm{b}}^2>0$ line intersect hyperbola at two points.

Hence $y=mx\pm \sqrt{a^2{m}^2-b^2}$ is a tangent to hyperbola .

Let this tangent passes through a point $(\mathrm{h},\mathrm{k})$ then $\mathrm{k}=\mathrm{mh}\pm \sqrt{{\mathrm{a}}^2{\mathrm{m}}^2-{\mathrm{b}}^2}$ $(\mathrm{k}-\mathrm{mh}{)}^2={\mathrm{a}}^2{\mathrm{m}}^2-{\mathrm{b}}^2$

${\mathrm{m}}^2({\mathrm{h}}^2-{\mathrm{a}}^2)-2\mathrm{mkh}+{\mathrm{k}}^2+{\mathrm{b}}^2=0$

Hence maximum two tangents can be drawn through a point $P$.

Now ${\mathrm{m}}_1+{\mathrm{m}}_2=\frac{2\mathrm{kh}}{{\mathrm{h}}^2-{\mathrm{a}}^2}$

${\mathrm{m}}_1\cdot {\mathrm{m}}_2=\frac{{\mathrm{k}}^2+{\mathrm{b}}^2}{{\mathrm{h}}^2-{\mathrm{a}}^2}$

If $\theta$ is the angle between the two tangents, then

$\tan \theta =\left(\frac{{\mathrm{m}}_1-{\mathrm{m}}_2}{1+{\mathrm{m}}_1{\mathrm{m}}_2}\right)$

$\Rightarrow {\tan }^2\theta =\frac{{(m_1+m_2)}^2-4m_1{m}_2}{{(1+m_1{m}_2)}^2}=\frac{{\left(\frac{2kh}{h^2-a^2}\right)}^2-4\left(\frac{k^2+b^2}{h^2-a^2}\right)}{{(1+\frac{k^2+b^2}{h^2-a^2})}^2}$

$\Rightarrow {\tan }^2\theta =\frac{4{\mathrm{k}}^2{\mathrm{h}}^2-4({\mathrm{h}}^2-{\mathrm{a}}^2)({\mathrm{k}}^2+{\mathrm{b}}^2)}{{({\mathrm{h}}^2+{\mathrm{k}}^2-{\mathrm{a}}^2+{\mathrm{b}}^2)}^2}$

$\Rightarrow {\tan }^2\theta =\frac{4({\mathrm{a}}^2{\mathrm{b}}^2+{\mathrm{a}}^2{\mathrm{k}}^2-{\mathrm{h}}^2{\mathrm{b}}^2)}{{({\mathrm{h}}^2+{\mathrm{k}}^2-{\mathrm{a}}^2+{\mathrm{b}}^2)}^2}$

If $\theta ={90}^{\circ }$ then ${\mathrm{h}}^2+{\mathrm{k}}^2-{\mathrm{a}}^2+{\mathrm{b}}^2=0$

i.e. ${\mathrm{h}}^2+{\mathrm{k}}^2={\mathrm{a}}^2-{\mathrm{b}}^2$

Locus of $(h,k)$ is $x^2+y^2=a^2-b^2$

Hence, Locus of point of intersection of two perpendicular tangents is known as Director Circle. Its equation is $x^2+y^2=a^2-b^2$

If $\mathrm{a}=\mathrm{b}$, director circle is a point circle.

If $a<b$, no real director circle is possible.

For equation of hyperbola $\frac{(x-h{)}^2}{a^2}-\frac{(y-h{)}^2}{b^2}=1$, equation of tangent in slope from is $y-k=m(x-$h) $\pm \sqrt{a^2{m}^2-b^2}$.

Practice questions

1. If the foci of the ellipse $\frac{{\mathrm{x}}^2}{232}+\frac{{\mathrm{y}}^2}{{\mathrm{b}}^2}=1$ and the hyperbola $\frac{{\mathrm{x}}^2}{144}-\frac{{\mathrm{y}}^2}{81}=1$ coincide then ${\mathrm{b}}^2=$

(a). 3

(b). 5

(c). 7

(d). 9

2. If $\mathrm{PQ}$ is a double ordinate of the hyperbola $\frac{{\mathrm{x}}^2}{{\mathrm{a}}^2}-\frac{{\mathrm{y}}^2}{{\mathrm{b}}^2}=1$ such that $\mathrm{OPQ}$ is an equilateral triangle, $O$ being the centre of the hyperbola, the range of eccentricity is

(a). $(0,\frac{2}{\sqrt{3}})$

(b). $(\frac{2}{\sqrt{3}},\mathrm{\infty })$

(c). $(0,\frac{4}{3})$

(d). $(\frac{4}{3},\mathrm{\infty })$

3. An ellipse and hyperbola are confocal and the conjugate axis of the hyperbola is equal to the minor axis of the ellipse. If ${\mathrm{e}}_1$ and ${\mathrm{e}}_2$ are the eccentricities of the ellipse and hyperbola then

(a). ${\mathrm{e}}_1{}^2+{\mathrm{e}}_2{}^2=2$

(b). ${\mathrm{e}}_1+{\mathrm{e}}_2=2$

(c). $\frac{1}{{\mathrm{e}}_1}+\frac{1}{{\mathrm{e}}_2}=2$

(d). $\frac{1}{{\mathrm{e}}_1^2}+\frac{1}{{\mathrm{e}}_2^2}=2$

4. The centre of a hyperbola $\frac{(3x+4y-7{)}^2}{100}-\frac{(4x-3y+8{)}^2}{225}=1$ is

(a). $(\frac{-11}{25},\frac{52}{25})$

(b). $(\frac{11}{25},\frac{-52}{25})$

(c). $(0,0)$

(d). $(10,15)$

5. The equations of the transverse and conjugate axes of a hyperbola are $x+2y-3=0$ and $2x-y+4=0$ respectively and their respective lengths are $\sqrt{2}$ and $\frac{2}{\sqrt{3}}$, equation of hyperbola is

(a). $2(2x-y+4{)}^2-3(x+2y-3{)}^2=1$

(b). $2(x+2y-3{)}^2-3(2x-y+4{)}^2=1$

(c). $2(2x-y+4{)}^2-3(x+2y-3{)}^2=5$

(d). $2(x+2y-3{)}^2-3(2x-y+4{)}^2=5$

6. For all real values of $m$ the straight line $y=mx+\sqrt{9m^2-4}$ is a tangent to the hyperbola

(a). $4x^2-9y^2=36$

(b). $9x^2-4y^2=36$

(c). $x^2-36y^2=9$

(d). $36x^2-y^2=36$

7. The equation of tangents to the curve $4x^2-9y^2=1$ which is parallel to $4y=5x+7$ is

(a). $4y=5x-30$

(b). $4y=5x+24$

(c). $24\mathrm{y}-30\mathrm{x}=\sqrt{161}$

(d). $30\mathrm{x}-24\mathrm{y}-\sqrt{161}=0$

8. If the line $5x+12y=9$ touches the hyperbola $x^2-9y^2=9$ then point of contact is

(a). $(5,4)$

(b). $(5,\frac{-4}{3})$

(c). $(5,\frac{4}{3})$

(d). $(5,-4)$

9. If $\mathrm{y}=\mathrm{mx}+\sqrt{51}$ is a tangent to the hyperbola $\frac{{\mathrm{x}}^2}{100}-\frac{{\mathrm{y}}^2}{49}=1$, then $\mathrm{m}=$

(a). $1$

(b). $\sqrt{17}$

(c). $-1$

(d). $2$

10. The locus of the point of intersection of perpendicular tangents to $\frac{x^2}{25}-\frac{y^2}{16}=1$ is:

(a). $x^2+y^2=25$

(b). $x^2+y^2=16$

(c). $x^2+y^2=41$

(d). $x^2+y^2=9$