HYPERBOLA-2 (Conjugate Hyperbola)
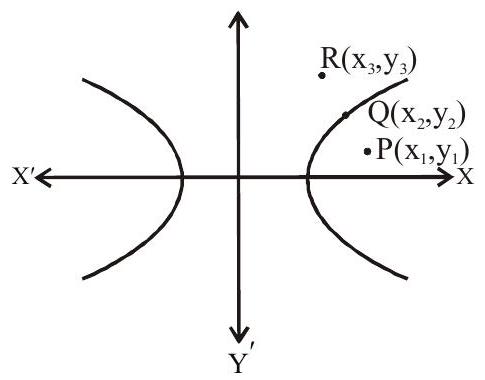
Position of a point
Let a point
If
If
If
Conjugate Hyperbola
Corresponding to every hyperbola there exists a hyperbola such that the transverse axis and conjugate axis of one is equal to the conjugate axis and transverse axis of the other. Such hyperbolas are known as conjugate to each other.
Therefore for the hyperbola
Conjugate hyperbola is
Let
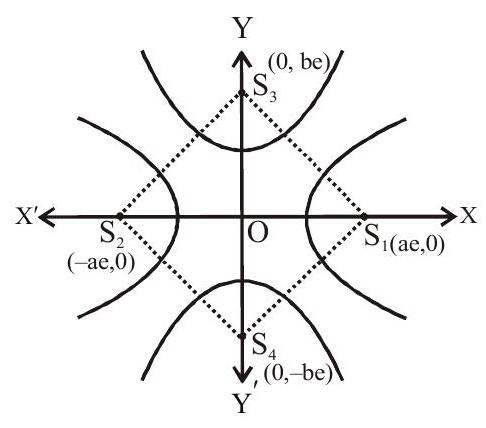
the eccentricity of
and
The foci of a hyperbola and its conjugate hyperbola are concyclic and form the vertices of a square.
Auxiliary circle and eccentric angle
A circle drawn with centre
A is any point on the circle whose coordinates are
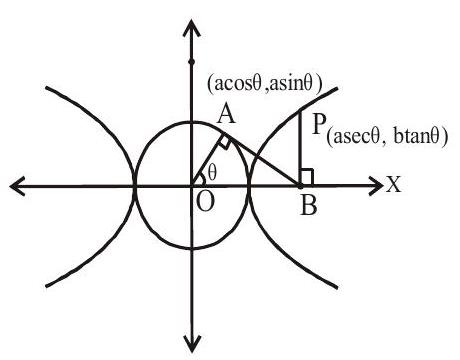
P point lies on hyperbola, so
The equations
For the hyperbola
| Hyperbola | Conjugate Hyperbola | |
|---|---|---|
| Equation | ||
| Centre | ||
| Vertice | ||
| Foci | ||
| Length of transverse axis | ||
| Length of conjugate axis | ||
| Length of latus rectum | ||
| Equation of transverse axis | ||
| Equation of conjugate axis | ||
| Equation of directrices | ||
| Eccentricity |
Examples
1. The point
(a) circle
(b) parabola
(c) ellipse
(d) hyperbola
Show Answer
Solution:
Let
Squaring and subtracting, we get
Answer: d
2. The position of the point
(a) on the hyperbola
(b) outside the hyperbola
(c) Inside the hyperbola
(d) can not say
Show Answer
Solution:
Answer: c.
3. Two circles are given such that they neither intersect nor touch. The locus of centre of variable circle which touches both the circles externally is
(a) a circle
(b) a parabola
(c) an ellipse
(d) a hyperbola
Show Answer
Solution:
Let radii of the fixed circles be
Let variable circles with centre
Then
Locus of

Answer: d
4: If the latus rectum subtends a right angle at the centre of the hyperbola
(a)
(b)
(c)
(d)
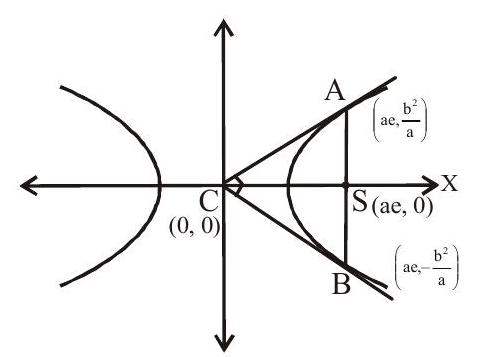
Show Answer
Solution:
[if
Answer: d
Practice questions
1. The equation of hyperbola whose foci are
(a).
(b).
(c).
(d). none of these
Show Answer
Answer: (a)2. If
(a).
(b).
(c).
(d).
Show Answer
Answer: (c)3. If e and
(a).
(b).
(c).
(d).
Show Answer
Answer: (d)4. The foci of a hyperbola coincide with the foci of the ellipse
(a).
(b).
(c).
(d).
Show Answer
Answer: (a)5. The equation
(a). an ellipse
(b). a parabola
(c). a pair of coincident line segment
(d). hyperbola
Show Answer
Answer: (c)6. For hyperbola
(a). abscissae of vertices
(b). abscissae of foci
(c). eccentricity
(d). directrix
Show Answer
Answer: (b)7. Two rods are rotating about two fixed points in opposite directions. If they start from their position of co-incidence and one rotates at the rate double that of the other, then locus of point of intersections of two rods is
(a). a parabola
(b). a circle
(c). an ellipse
(d). a hyperbola
Show Answer
Answer: (d)8. The equations
(a). an ellipse
(b). a circle
(c). a hyperbola
(d). None of these
Show Answer
Answer: (d)9. The equation
(a). no locus if
(c). a point if
(b). an ellipse if
(d). a hyperbola if
Show Answer
Answer: (c)10. A hyperbola having the transverse axis of length
(a).
(b).
(c).
(d).










