COORDINATE GEOMETRY - 5 (Straight Line)
Topics Covered
-
Image of a point in different cases.
-
Foot of the perpendicular.
-
Family of lines.
1. Image of a point in different cases
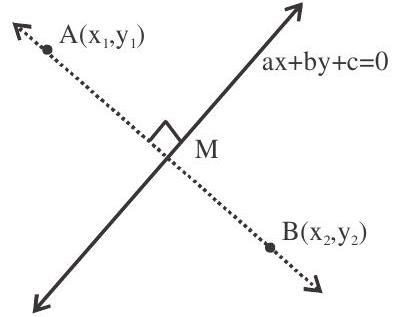
i. The image of a point with respect to the line mirror
Image of $\mathrm{A}\left(\mathrm{x} _{1}, \mathrm{y} _{1}\right)$ with respect to line mirror $\mathrm{ax}+\mathrm{by}+\mathrm{c}=0$ be $\mathrm{B}\left(\mathrm{x} _{2}, \mathrm{y} _{2}\right)$ is given by
$\frac{\mathrm{x} _{2}-\mathrm{x} _{1}}{\mathrm{a}}=\frac{\mathrm{y} _{2}-\mathrm{y} _{1}}{\mathrm{~b}}=\frac{2\left(\mathrm{ax} _{1}+\mathrm{by} _{1}+\mathrm{c}\right)}{\mathrm{a}^{2}+\mathrm{b}^{2}}$
$M$ is the foot of prependicular from $A$ on $a x+b y+c=0$
ii. The image of a point with respect to $x$-axis:-
Let $\mathrm{A}\left(\mathrm{x} _{1}, \mathrm{y} _{1}\right)$ be any point and $\mathrm{B}\left(\mathrm{x} _{2}, \mathrm{y} _{2}\right)$ its image after reflection in the $\mathrm{x}$-axis then. $x _{1}=x _{2} \& y _{2}=-y _{1}(M$ is the mid point of $A \& B)$
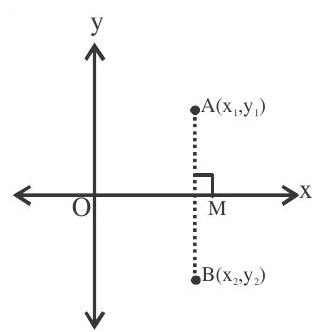
$\mathrm{M}$ is the foot of perpendicular from $\mathrm{A}$ on $\mathrm{x}$-axis
iii. The image of a point with respect to $y$-axis:-
Let $\mathrm{A}\left(\mathrm{x} _{1,} \mathrm{y} _{1}\right)$ be any point and $\mathrm{B}\left(\mathrm{x} _{2}, \mathrm{y} _{2}\right)$ its image after reflection in the mirror $\mathrm{y}$-axis then. $x _{2}=-x _{1} \& y _{1}=y _{2}(N$ is the mid point of $A \& B)$
$\mathrm{N}$ is the foot of perpendicular from $\mathrm{A}$ on $\mathrm{y}$-axis
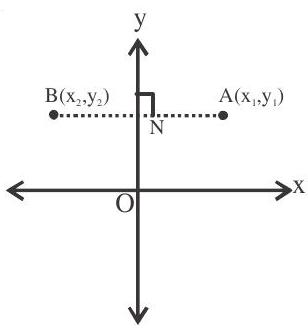
iv. The image of a point with respect to the origin:
Let $A\left(x _{1,} y _{1}\right)$ be any point $B\left(x _{2,} y _{2}\right)$ be its image after reflection through the origin then.
$x _{2}=-x _{1} \& y _{2}=-y _{1}(O$ is the mid point of $A \& B)$
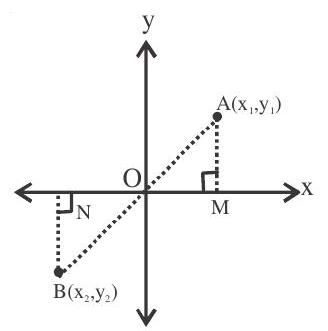
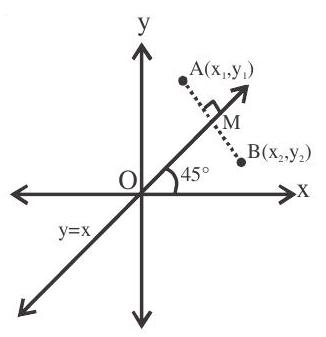
v. The image of a point with respect to the line $\mathbf{y}=\mathbf{x}$
Let $\mathrm{A}\left(\mathrm{x} _{1}, \mathrm{y} _{1}\right)$ be any point and $\mathrm{B}\left(\mathrm{x} _{2}, \mathrm{y} _{2}\right)$ be its image after reflection in the line $\mathrm{y}=\mathrm{x}$ then. $\mathrm{y} _{2}=\mathrm{x} _{1}$ and $\mathrm{x} _{2}=\mathrm{y} _{1}(\mathrm{M}$ is the mid point of $\mathrm{A} \& \mathrm{~B})$
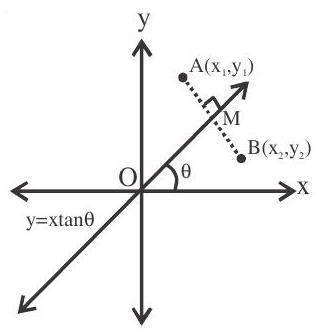
vi. The image of a point with respect to the line $y=x \tan \theta$
Let $\mathrm{A}\left(\mathrm{x} _{1,} \mathrm{y} _{1}\right)$ be any point and $\mathrm{B}\left(\mathrm{x} _{2}, \mathrm{y} _{2}\right)$ be its image after reflection in the line $\mathrm{y}=\mathrm{x} \tan \theta$ or $\mathrm{y}=\mathrm{mx}$ then.
$\mathrm{x} _{2}=\mathrm{x} _{1} \cos 2 \theta+\mathrm{y} _{1} \sin 2 \theta \quad$ (M is the mid point of $\mathrm{A} \& \mathrm{~B}$ )
$\mathrm{y} _{2}=\mathrm{x} _{1} \cos 2 \theta-\mathrm{y} _{1} \sin 2 \theta$
Line parallel and perpendicular to given line
Given equation of straight line be $a x+b y+c=0$
A line parallel to given line is $a x+b y+d=0$ only constant term changes.
A line perpendicular to given line is $b x-a y+k=0$
Here change coordinate of $\mathrm{x}$ as coordinate of $\mathrm{y} \& $ coordinate of $\mathrm{y}$ as negative of coordinate of $x$ and constant term changes
It the lines $\mathrm{a} _{1} \mathrm{x}+\mathrm{b} _{1} \mathrm{y}+\mathrm{c} _{1}=0$ and $\mathrm{a} _{2} \mathrm{x}+\mathrm{b} _{2} \mathrm{y}+\mathrm{c} _{2}=0$ are prependecular then $\mathrm{a} _{1} \mathrm{a} _{2}+\mathrm{b} _{1} \mathrm{~b} _{2}=0$
i. Coincident, if $\frac{\mathrm{a} _{1}}{\mathrm{a} _{2}}=\frac{\mathrm{b} _{1}}{\mathrm{~b} _{2}}=\frac{\mathrm{c} _{1}}{\mathrm{c} _{2}}$
ii. Parallel, if $\frac{\mathrm{a} _{1}}{\mathrm{a} _{2}}=\frac{\mathrm{b} _{1}}{\mathrm{~b} _{2}} \neq \frac{\mathrm{c} _{1}}{\mathrm{c} _{2}}$
iii. Intersecting, if $\frac{\mathrm{a} _{1}}{\mathrm{a} _{2}} \neq \frac{\mathrm{b} _{1}}{\mathrm{~b} _{2}}$
Practice questions
1. If $t _{1}$ and $t _{2}$ are roots of the equation $t^{2}+\lambda t+1=0$, where $\lambda$ is an arbitrary constant. Then the line joining the points $\left(\mathrm{at} _{1}{ }^{2}, 2 a \mathrm{at} _{1}\right) \& \left(\mathrm{at}^{2}{ } _{2}, 2 \mathrm{at} \mathrm{t} _{2}\right)$ always passes through a fixed point
(a) $(\mathrm{a}, 0)$
(b) $(-\mathrm{a}, 0)$
(c) $(0, a)$
(d) $(0,-\mathrm{a})$
Show Answer
Answer: (b)2. The equation $x^{3}+y^{3}=0$ represents
(a) three real straight lines
(b) three points
(c) combined equation of a st. line $\& $ a circle
(d) None of these.
Show Answer
Answer: (d)3. The three lines whose combined equation is $y^{3}-4 x^{2} y=0$ form a triangle which is
(a) isosceles
(b) equilateral
(c) right angled
(d) None of these
Show Answer
Answer: (d)Comprehension type
$\mathrm{A}(1,3)$ and $\mathrm{C}\left(-\frac{2}{5},-\frac{2}{5}\right)$ are the vertices of a triangle $\mathrm{ABC}$ and the equation of the angle bisector of $\angle \mathrm{ABC}$ is $\mathrm{x}+\mathrm{y}=2$
4. Equation of side $\mathrm{BC}$ is
(a) $7 x+3 y=4$
(b) $7 x+3 y+4=0$
(c) $7 x-3 y+4=0$
(d) $7 x-3 y=4$
Show Answer
Answer: (b)5. Coordinates of vertex $B$ are
(a) $\left(\frac{3}{10}, \frac{17}{10}\right)$
(b) $\left(\frac{17}{10}, \frac{3}{10}\right)$
(c) $\left(-\frac{5}{2}, \frac{9}{2}\right)$
(d) $(1,1)$
Show Answer
Answer: (c)6. Equation of side $\mathrm{AB}$ is
(a) $3 x+7 y=24$
(b) $3 x+7 y+24=0$
(c) $ 13 x+7 y+8=0$
(d) $13 x-7 y+8=0$
Show Answer
Answer: (a)Assertion and reasoning type
7. Lines $L _{1} L _{2}$ given by $y-x=0$ and $2 x+y=0$ intersect the line $L _{3}$ given by $y+2=0$ at $P$ and $Q$, respectively. The bisector of the acute angle between $\mathrm{L} _{1}$ and $\mathrm{L} _{2}$ intersects $\mathrm{L} _{3}$ at $\mathrm{R}$.
Statement 1 : The ratio PR: RQ equals $2 \sqrt{2}: \sqrt{5}$.
Statement 2 : In any triangle, bisector of an angle divides the triangle into two similar triangles.
a. Statement 1 is true, Statement 2 is True; Statement 2 is a correct explanation for Statement1.
b. Statement 1 is True, Statement 2 is True; Statement 2 is NOT a correct explanation for Statement 1.
c. Statement 1 is True, Statement 2 is False.
d. Statement 1 is False, Statement 2 is True.
Show Answer
Answer: (c)Matrix-match
8. This question contains statements given in two columns which have to be matched. Statements a, $\mathrm{b}, \mathrm{c}, \mathrm{d}$ in column I have to be matched with statements p,q, r, s in column II. If the correct match is $\mathrm{a} \rightarrow \mathrm{p}, \mathrm{a} \rightarrow \mathrm{s}, \mathrm{b} \rightarrow \mathrm{q} \mathrm{b} \rightarrow \mathrm{r}, \mathrm{c} \rightarrow \mathrm{p}, \mathrm{c} \rightarrow \mathrm{q}$ and $\mathrm{d} \rightarrow \mathrm{s}$, then the correctly dubbled $4 \times 4$ matrix should be as follows :
Consider the lines given by
$\mathrm{L} _{1}: \mathrm{x}+3 \mathrm{y}-5=0$
$\mathrm{~L} _{2}: 3 \mathrm{x}-\mathrm{ky}-1=0$
$\mathrm{~L} _{3}: 5 \mathrm{x}+2 \mathrm{y}-12=0$
| Column I | Column II |
|---|---|
| a. $ \mathrm{L} _{1}, \mathrm{~L} _{2}, \mathrm{~L} _{3}$ are concurrent, if | p. $\mathrm{k}=-9$ |
| b. One of $\mathrm{L} _{1}, \mathrm{~L} _{2}, \mathrm{~L} _{3}$ is parallel to at least one of the other two, if | q. $k=-6 / 5$ |
| c. $ \mathrm{L} _{1}, \mathrm{~L} _{2}, \mathrm{~L} _{3}$ form a triangle, if | r. $\mathrm{k}=5 / 6$ |
| d. $ \mathrm{L} _{1}, \mathrm{~L} _{2}, \mathrm{~L} _{3}$ do not form a triangle, if | s. $\mathrm{k}=5$ |










