The feasibility of any chemical reaction can be predicted thermodynamically but this study cannot give answer to

• how fast the reaction would be

• what parameters can change the rate of reaction

• what mechanism does it follow to form products

Thus, the branch of physical chemistry that deals with the study of rate of the reaction and the factors such as temperature; pressure, concentration, light that govern the rate and the mechanism by which the reaction proceeds is called Chemical Kinetics

Based on the time taken by the reaction for its completion, various reactions are divided into three categories:

The reactions can be classified as fast, slow or moderate reactions.

• Fast reaction : These reactions are so fast that they occur as soon as the reactants are brought together. Generally, such reactions involve ionic species.

$\hspace{2.2cm}$ eg: reaction between $\mathrm{H} _{3} \mathrm{O}^{+}$and $\mathrm{OH}^{-}$to form water molecule

$\hspace{2.3cm} \mathrm{H} _{3} \mathrm{O}^{+}+\mathrm{OH}^{-} \longrightarrow 2 \mathrm{H} _{2} \mathrm{O}$

• Slow reaction : These reactions may take months/years for their completion.

$\hspace{2.2cm}$eg: 1) $2 \mathrm{H} _{2}(\mathrm{~g})+\mathrm{O} _{2}(\mathrm{~g}) \xrightarrow[\text { temp }]{\text { room }} 2 \mathrm{H} _{2} \mathrm{O}$

$\hspace{2.6cm}$ 2) Rusting of iron occurs very slowly

$\hspace{2.6cm}$ 3) Reaction between carbon and oxygen.

$\hspace{3cm} \mathrm{C}+\mathrm{O} _{2} \xrightarrow{\text { room temp }} \mathrm{CO} _{2}$

Carbon and oxygen are thermodynamically less stable than $\mathrm{CO} _{2}$ at $298 \mathrm{~K}$, yet coke doesn’t catch fire spontaneously as this is a slow reaction

• Moderate reaction : Between these two extremes there are many reactions which occur at measurable speed and are commonly studied under chemical kinetics.

$\hspace{2.2cm}$ eg: 1) Decomposition of $\mathrm{H} _{2} \mathrm{O} _{2}$

$\hspace{3.1cm} 2 \mathrm{H} _{2} \mathrm{O} _{2} \longrightarrow 2 \mathrm{H} _{2} \mathrm{O}+\mathrm{O} _{2}$

$\hspace{2.7cm}$ 2) Hydrolysis of ester

$\hspace{3.1cm} \mathrm{CH} _{3} \mathrm{COOC} _{2} \mathrm{H} _{5} \xrightarrow[\mathrm{H}^{+}]{\mathrm{H} _{2} \mathrm{O}} \mathrm{CH} _{3} \mathrm{COOH}+\mathrm{C} _{2} \mathrm{H} _{5} \mathrm{OH}$

Factors Influencing the Rates of Reaction

• Nature of the Reactants: The nature of the reactants play an important role in influencing the rate of a reaction For eg: Both ferrous ions and oxalate ions can be oxidised by acidified $\mathrm{KMnO} _{4}$. It is observed that the redox reaction between $\mathrm{Fe}^{2+}$ and $\mathrm{M} _{\mathrm{n}} \mathrm{O} _{4}$ is moderate whereas heating of oxalate solution to about $50-60^{\circ} \mathrm{C}$ is required to carry out its redox reaction with $\mathrm{Mn} _{4} \mathrm{O} _{4}$ ions

• Concentration of the Reactants: As a reaction proceeds, the reactants are consumed. Greater the concentration, faster is the reaction.

• Temperature of the system: Generally, rate of reaction almost becomes double for every $10^{\circ}$ rise in temperature.

• Presence of catalyst: It increases the speed of reaction by lowering the activation energy barrier i.e., a new path is followed with lower activation energy. However, Presence of a catalyst cannot make a non-spontaneous reaction feasible.

• Surface area of the reactants: Greater the surface area, faster is the reaction. That is why pulverised wood or powdered coal burns faster than log of wood or a lump of coal (reactions involving solid reactants)

• Presence of light: It provides the necessary activation energy and the reaction starts (photochemical reactions).

Effect of change of Pressure or volume of the Vessel on the Rate of Reaction

$\hspace{3cm}$ Consider a reaction

$\hspace{4cm} 2 A+B \longrightarrow \text { Product }$

$\hspace{2.2cm}$ The rate law for above reaction is

$\hspace{2.2cm} \text { Rate }=[A]^{2}[B]$

If volume of the Vessel is doubled, concentrations are halved. Then, new rate is given as

$$ \text { Rate }=\mathrm{k}\left[\dfrac{A}{2}\right]^{2}\left[\dfrac{B}{2}\right] $$

If volume of vessel is reduced to $14^{\text {th }}$, concentrations become 4 times. Then, new rate is given as:

$$ \text { Rate }=\mathrm{k}[4 \mathrm{~A}]^{2}[4 \mathrm{~B}] $$

If at constant temperature, pressure is doubled, volume is reduced to half. Hence, concentrations are doubled. Then, new rate is given as:

$$ \text { Rate }=\mathrm{k}[2 \mathrm{~A}]^{2}[2 \mathrm{~B}] $$

Rate Law and order of a Reaction

• Rate of a reaction: It is defined as the change in concentration of the reactant or product per unit time.

$\hspace{2.2cm}$ For a general reaction

$\hspace{2.5cm} A \longrightarrow B$

The change in concentration with time is graphically represented as shows in Fig (1 and 2):

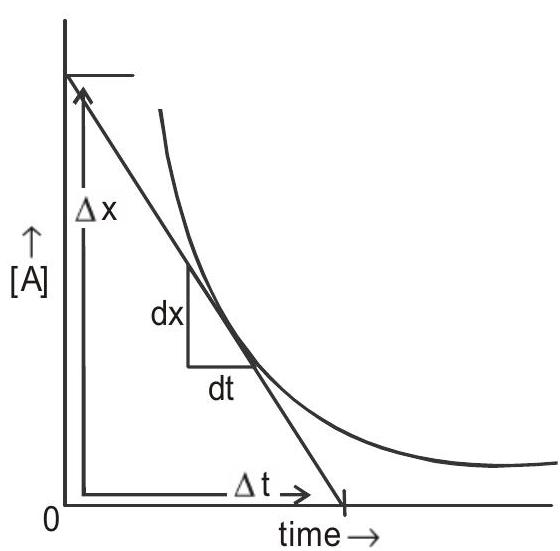
Fig 1: Plot of change in concentration of a reactant with time

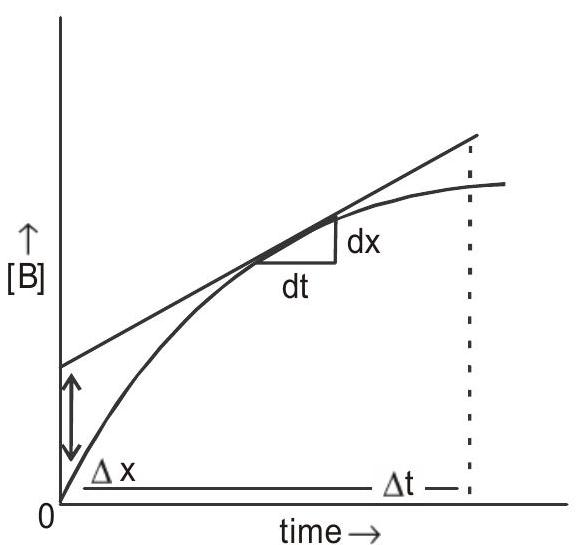
Fig 2: Plot of change in concentration of product with time

Rate of a reaction is defined in two ways

• Average rate :- Change in concentration of a reactant or a product over time interval $\Delta \mathrm{t}$,

$$ r _{\text {avg }}=\dfrac{\Delta[B]}{\Delta t}=-\dfrac{\Delta[A]}{\Delta t} $$

• Instantaneous rate : It is calculated from $\mathrm{r} _{\text {avg }}$ in the limit $\Delta \mathrm{t} \longrightarrow 0$ and is represented as

$$ r _{i n s t}=\dfrac{d[B]}{d t}=-\dfrac{d[A]}{d t} $$

Note: $r _{\text {inst }}$ is equivalent to the slope of the tangent from the plot of concentration of ’ $A$ ’ or ’ $B$ ’ at any time instant ’ $t$ ‘. Also, minus sign signifies decrease in concentration while plus sign signifies increase in concentration with time.

For the reaction, $\mathrm{N} _{2}(\mathrm{~g})+3 \mathrm{H} _{2}(\mathrm{~g}) \longrightarrow 2 \mathrm{NH} _{3}(\mathrm{~g})$

$$ \begin{aligned} & r _{(a v g)}=\dfrac{\Delta\left[N _{2}\right]}{\Delta t}=-\dfrac{1}{3} \dfrac{\Delta[H]}{\Delta t}=\dfrac{1}{2} \dfrac{\Delta\left[N H _{3}\right]}{\Delta t} \\ & r _{\text {inst }}=\dfrac{-d\left[N _{2}\right]}{d t}=-\dfrac{1}{3} \dfrac{d\left[H _{2}\right]}{d t}=+\dfrac{1}{2} \dfrac{d\left[N H _{3}\right]}{d t} \end{aligned} $$

• Rate law : Rate law is the expression in which reaction rate is given in terms of molar concentration of reactants with each term raised to some power (which is determined experimentally) which may or may not be equal to their stoichiometric coefficient.

For a reaction, $\mathrm{P}+\mathrm{Q} \longrightarrow 2 \mathrm{R}$

$$ \begin{aligned} & \text { rate }=\dfrac{-d[P]}{d t}=\dfrac{-d[Q]}{d t}=\dfrac{1}{2} \dfrac{d[R]}{d t} \\ & =r[P]^{\alpha}[Q]^{\beta} \end{aligned} $$

where $\alpha$ is called order of reaction w.r.t $P$ and $\beta$ is called order w.r.t. $Q$. The order of reaction (n) is equal to $\alpha+\beta$.

Difference between rate of reaction and rate constant:

Measurement of the Rate of reaction:

In order to measure the rate of a reaction, the progress of reaction is followed by monitoring the concentration of one of the reactant or product at different intervals of time.

There are various methods of following the same for eg. in gaseous reaction the rate of the reaction can be calculated either by collecting volumes of one of gas or by measuring change in pressure of a gas at different intervals of time.

In liquid phase, the reactions can be investigated by monitoring different physical parameters for eg. change in refractive index, change in angle of plane polarised light, change in conductance at different intervals of time. For investigating moderate reactions (neither fast nor slow) the most commonly used technique is volumetric analysis. In this method a small amount of reaction mixture $(2 \mathrm{~mL}$ or $5 \mathrm{~mL})$ is withdrawn at differnt intervals of time and immediately cooled down to $0^{\circ} \mathrm{C}$ to arrest reaction. Then, the concentration of either a reactant/product is determined by carrying out a titration with a titrant of known concentration.

Based on the dependence of the reaction on the concentration of reacting species, various reactions can be classified as:

Reaction of Zero Order: When the rate of reaction is independent of the concentration of all the reactants.

• Consider a general reaction,

$$ \mathrm{A} \longrightarrow \text { Products } $$

If it is of zero order, then

$$ \begin{aligned} & \text { Rate }=\dfrac{-d[A]}{d t}=k[A]^{0}=k \\ & \text { or } d[A]=-k d t \end{aligned} $$

On Integration, we get

$[A]=-k t+l$

At $t=0,[A]=[A] _{0}$

$\Rightarrow I=[A] _{0}$

$\therefore[\mathrm{A}]=\mathrm{kt}+[\mathrm{A}] _{0}$

or $k=\dfrac{1}{t}\left[(A) _{0}-(A)\right]$

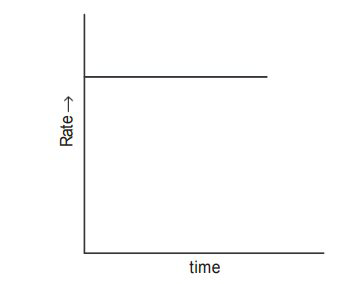
Fig 3: Variation of rate of reaction with time for zero order reaction

Zero order reaction will proceed with a constant rate and the rate will drop to zero at the end of reaction.

Examples of Zero Order Reactions

(i) Photochemical reactions: These reactions occur in the presence of light. Many such reactions are found to be of Zero order. For e.g., the combination between hydrogen and chlorine in the presence of light may be studied by enclosing these gases in a tube and inverting the tube in a trough of water. $\mathrm{HCl}$ formed during the reaction dissolves into the water and the level of water in the tube rises. Thus, though the quantities of $\mathrm{H} _{2}$ and $\mathrm{Cl} _{2}$ decrease, their amounts per unit volume remain the same i.e. the concentrations of $\mathrm{H} _{2}$ and $\mathrm{Cl} _{2}$ remain constant.

(ii) Heterogeneous reactions: A reaction in which the reactants, product and catalyst are present in different phases. The decomposition of $\mathrm{HI}$ on the surface of gold follows zero order kinetics.

Expression for Half change period

Half-change period is the time taken for half of the reaction to complete, i.e., the time in which half of the reactant is consumed in a reaction.

When $\mathrm{t}=\mathrm{t} _{1 / 2},[\mathrm{~A}]=\dfrac{[\mathrm{A}] _{0}}{2}$

$\mathrm{t}_{\dfrac{1}{2}}=\dfrac{1}{\mathrm{k}}\bigg\{[\mathrm{A}]_0-\dfrac{[\mathrm{A}]_0}{2}\bigg\}$

i.e., $t _{1 / 2}=\dfrac{[A] _{0}}{2 k}$

Thus, $\mathrm{t} _{1 / 2} \propto[\mathrm{A}] _{0}$

When reaction is complete, $[\mathrm{A}]=0, \mathrm{t}=\mathrm{t} _{100 \%}$

$$ \begin{aligned} & \mathrm{kt}=[\mathrm{A}] _{0} \\ & \therefore \quad \mathrm{t} _{100 \%}=\dfrac{[A] _{0}}{k} \end{aligned} $$

Comparision between $t _{1 / 2}$ and $t _{3 / 4}$

$\hspace{3cm} \begin{aligned} t _{3 / 4}=\dfrac{1}{k}\bigg \{[A] _{0}-\dfrac{1}{4}[A] _{0}\bigg \},=\dfrac{3}{4} \dfrac{[A] _{0}}{k} \\ & \\ t _{1 / 2}=\dfrac{1}{k}\bigg \{[A] _{0}-\dfrac{[A] _{0}}{2}\bigg \}=\dfrac{1}{2} \dfrac{[A] _{0}}{k} \end{aligned}$

$\hspace{3cm} \begin{aligned} & \dfrac{t _{3 / 4}}{t _{1 / 2}}=\dfrac{3}{4} \dfrac{[A] _{0}}{k} \times \dfrac{2 k}{[A] _{0}} \\ & \\ & \text { or } t _{3 / 4}=1.5 t _{1 / 2} \end{aligned}$

Reactions of First Order: A reaction is said to be of first order if rate of the reaction depends upon one concentration term only. For the reaction $\mathrm{A} \longrightarrow$ Products

Rate of reaction $\alpha[\mathrm{A}]$

For the reaction, $2 \mathrm{~A} \longrightarrow$ Products

Rate of reaction $\alpha[A]$

For the reaction, $\mathrm{A}+\mathrm{B} \longrightarrow$ Products

Rate of reaction $\alpha[\mathrm{A}]$ or $[\mathrm{B}]$

Consider the simplest case viz

$\mathrm{A} \longrightarrow$ Products

Rate $=\dfrac{-d[A]}{d t}=k[A]^{1}$

$ \text { or } \dfrac{-d[A]}{[A]}=\mathrm{kdt} $

Intergrating both sides

$ \begin{aligned} & -\int \dfrac{d[A]}{[A]}=k \int d t \text { we get } \\ & \ln [A]=-k t+I \end{aligned} $

when $\mathrm{t}=0,[\mathrm{~A}]=[\mathrm{A}] _{0}$

$\therefore \quad \ln [\mathrm{A}] _{0}=1$

Substituting the value of ’ $l$ ‘, the equation becomes,

$ \ln [A]=-k t+\ln [A] _{0} $

or $\quad k t=\ln [A] _{0}-\ln [A]$

$ k t=\ln \dfrac{[A] _{0}}{[A]} $

$ k=\dfrac{l}{t} \ln \dfrac{[A] _{0}}{[A]}=\dfrac{2.303}{t} \log \dfrac{[A] _{0}}{[A]} $

where $[A] _{0}$ is the initial concentration and $[A]$ is concentration of the reactant at any time ’ $t$ ‘.

If the initial concentration of reactant is ’ $a$ ’ and amount of reactant reacted is ’ $x$ ’ in time ’ $t$ ‘, then

or $\quad k=\dfrac{2.303}{t} \log \dfrac{[A] _{0}}{[A] _{t}}$

$ =\dfrac{2.303}{t} \log \dfrac{a}{a-x} $

The exponential form of the expression for first order reaction is

$ A=A _{0} \cdot e^{-k t} $

Modified expression for first order rate equation

• Reaction in gaseous phase

$ \mathrm{A}(\mathrm{g}) \longrightarrow \mathrm{B}(\mathrm{g})+\mathrm{C}(\mathrm{g}) $

Initial

$ \begin{array}{lll} P _{0} & 0 & 0 \end{array} $

After time $t, \quad P _{0}-p \quad p \quad p$

Total pressure after time $t$,

$ P _{t}=\left(P _{0}-p\right)+p+p=P _{0}+p $

or $p=P _{t}-P _{0}$

Pressure of $A$ after time $t$,

$ P _{A}=\left(P _{0}-p\right)=\left[P _{0}-\left(P _{t}-P _{0}\right)\right]=2 P _{0}-P _{t} $

Now, $[\mathrm{A}] _{0} \propto \mathrm{P} _{0}$

$ \begin{aligned} & {[A] _{t}=P _{A}} \\ & \text { or }[A] _{t} \propto 2 P _{0}-P _{t} \end{aligned} $

Hence, $k=\dfrac{2.303}{t} \log \dfrac{P _{0}}{2 P _{0}-P _{t}}$

• Some Important Characteristics of First Order Reactions

• Rate constant of a first order reaction can be also be calculated by measuring the concentration of the reactant at two different time when the initial concentration is not known.

Let $A _{1}$ and $A _{2}$ be the reactant concentration at time $t _{1}$ and $t _{2}$ respectively, then we have

$$\begin{aligned} &2.303 \log _{10} \dfrac{A_0}{A_1}=k t_1 \hspace{3cm} \cdots \cdots \text{(i)} \\ & \\ &2.303 \log _{10} \dfrac{A_0}{A_2}=k_2 \hspace{3cm} \cdots \cdots \text{(ii)} \end{aligned}$$

Subtracting equation (i) from (ii) we get:

$$ 2.303 \log _{10} \dfrac{A _{1}}{A _{2}}=k\left(t _{2}-t _{1}\right) $$

• Half change period of a first order reaction is independent of the intial concentration of the reactant

$$ \begin{aligned} & k=\dfrac{2.303}{t _{1 / 2}} \log _{10} \dfrac{a}{a-\dfrac{a}{2}} \\ & =\dfrac{2.303}{t _{1 / 2}} \log _{10} 2 \\ & k=\dfrac{0.693}{t _{1 / 2}} \end{aligned} $$

Also, for first order reaction

$$ \dfrac{A _{1}}{A _{2}}=\left(\dfrac{1}{2}\right)^{n} \quad \text { where } n=\dfrac{t}{t _{1 / 2}}=\text { no. of half change } $$

Examples of the Reaction of First Order

• Reactions taking place in the gaseous phase- e.g. decomposition of $\mathrm{N} _{2} \mathrm{O} _{5}$, thermal decomposition of Azoisopropane.

• Reactions taking place in the solution- e.g. conversion of $\mathrm{N}$-Chloroacetanilide into $\mathrm{p}$ Chloroacetanilide in acidic medium.

• The Radioactive decay follows first order kinetics

$\hspace{1cm} \lambda=\dfrac{2.303}{t} \log \dfrac{N _{0}}{N _{t}}$

$\hspace{1cm}$ where $\mathrm{N} _{0}=$ initial number of nuclei $(\mathrm{t}=0)$

$\hspace{1cm} \mathrm{N} _{\mathrm{t}}=$ final number of nuclei (at $\mathrm{t}$ )

$\hspace{1cm} \lambda=$ decay constant

Pseudo first order reaction

These are those reactions with higher order but under certain conditions become reactions of the first order

Consider a reaction

$$ \mathrm{A}+\mathrm{B} \longrightarrow \text { Products } $$

Expected rate law

$$ \text { Rate }=k[A][B] \quad \text { Expected order }=1+1=2 $$

When either of the reactant is taken in excess, say $[A] »[B]$ then observed rate law is

$$ \begin{aligned} & \text { Rate }=k^{\prime}[B] \\ & \text { where } k^{\prime}=k[A] \end{aligned} $$

The examples are :-

1) Acid catalysed hydrolysis of ester

$$ \begin{aligned} & \mathrm{CH} _{3} \mathrm{COOC} _{2} \mathrm{H} _{5}+\mathrm{H} _{2} \mathrm{O} \longrightarrow \mathrm{CH} _{3} \mathrm{COOH}+\mathrm{C} _{2} \mathrm{H} _{5} \mathrm{OH} \\ & \text { Rate }=\mathrm{k}\left[\mathrm{CH} _{3} \mathrm{COOC} _{2} \mathrm{H} _{5}\right]\left[\mathrm{H} _{2} \mathrm{O}\right] \end{aligned} $$

Here, water is taken in excess and therefore its concentration may be taken as constant. Hence,

$$ \begin{aligned} & \text { Rate }=\mathrm{k}^{\prime}\left[\mathrm{CH} _{3} \mathrm{COOC} _{2} \mathrm{H} _{5}\right] \\ & \mathrm{k}^{\prime}=\mathrm{k}\left[\mathrm{H} _{2} \mathrm{O}\right] \end{aligned} $$

The reaction is therefore pseudo first order. The rate constant for this reaction is given as:

$$ k=\dfrac{2.303}{t} \log _{10} \dfrac{V _{\infty}-V _{0}}{V _{\infty}-V _{t}} $$

where $\mathrm{V} _{0}=$ volume of $\mathrm{NaOH}$ used to neutralize the mineral acid present $\left(\mathrm{H}^{+}\right)$, which is being used as a catalyst

$V _{t}=$ volume of $\mathrm{NaOH}$ used to neutralize the acid catalyst and $\mathrm{CH} _{3} \mathrm{COOH}$ produced in the reaction at time ’ $t$ ‘.

$\mathrm{V} _{\infty}=$ volume of $\mathrm{NaOH}$ used to neutralize the catalyst and the maximum amount of acetic acid that can be produced from the hydrolysis of initial concentration of ester.

2). Inversion of cane sugar :- This is also an example of pseudo first order reaction, water being present in excess

$\underset{\text{sucrose(dextro)}}{C_{12} H_{22} O_{11}} \longrightarrow \underset{\text{Gucose(dextro)}}{C_6 H_{12} O_6} + \underset{\text{Gucose(dextro)}}{C_6 H_{12} O_6}$

The rate is measured by measuring the change in the angle of rotation by polarimeter. The change produced in the angle of rotation in time ’ $\mathrm{t}$ ’ gives the measure of ’ $x$ ’ while the total change in the angle of rotation produced at the end of the reaction is the measure of the initial concentration of sucrose

$$ k=\dfrac{2.303}{t} \log _{10} \dfrac{r _{0}-r _{\infty}}{r _{t}-r _{\infty}} $$

Reactions of $\mathrm{n}^{\text {th }}$ order

Half-change Period and Fractional Change Time

General Expression for $\mathrm{t} _{1 / 2}$

For zero order reactions, $\mathrm{t} _{1 / 2} \propto[\mathrm{A}] _{0}$

For 1 st order reactions, $\mathrm{t} _{1 / 2} \alpha[\mathrm{A}] _{0}^{0}$

For 2nd order reactions, $\mathrm{t} _{1 / 2} \alpha[\mathrm{A}] _{0}^{-1}$ and so on.

In general, for reaction of $\mathrm{n}^{\text {th }}$ order,

$$ t _{1 / 2} \alpha[A] _{0}^{n-1} \quad \text { or } \quad t _{1 / 2}, \alpha \dfrac{1}{[A] _{0}^{1-n}} $$

Time for $\mathrm{n}^{\text {th }}$ fraction of reaction to complete.

For $\mathrm{n}^{\text {th }}$ fraction to complete, put $x=\dfrac{a}{n}$

For reactions of first order,

$$ \begin{aligned} & t _{1 / n}=\dfrac{2.303}{k} \log \dfrac{a}{a-\dfrac{a}{n}} \\ & t _{1 / n}=\dfrac{2.303}{k} \log \dfrac{n}{n-1} \end{aligned} $$

Expression for the amount left after $\mathrm{n}$ half-lives

After one half-life, amount left $=\dfrac{[A] _{0}}{2}$

After two half-lives, amount left

$$ =\dfrac{1}{2} \times \dfrac{[A] _{0}}{2}=\dfrac{[A] _{0}}{2^{2}} $$

After three half-lives, amount left

$$ =\dfrac{1}{2} \times \dfrac{[A] _{0}}{2^{2}}=\dfrac{[A] _{0}}{2^{3}} $$

In general, amount left after $\mathrm{n}$ half-lives

$$ =\dfrac{[A] _{0}}{2^{n}} $$

No. of half lives $=\dfrac{\text { Total time }}{t _{1 / 2}}$

Determination of order of reaction from half life measurement: If $A _{1}, A _{2}$ are the initial concentrations of the reactants and $\left(\mathrm{t} _{1 / 2}\right) _{1}$ and $\left(\mathrm{t} _{1 / 2}\right) _{2}$ are the corresponding half lives, then order of reaction is given as

$$ n=1+\dfrac{\log _{10}\left(t _{1 / 2}\right) _{1} /\left(t _{1 / 2}\right) _{2}}{\log _{10} A _{2} / A _{1}} $$

Molecularity:

The number of atoms, ions or molecules that must collide with one another simultaneously so as to result into a chemical reaction is called the molecularity of the reaction. Reactions generally have molecularity of 1,2 or 3 . Reactions with molecularity more than 3 are very rare because chances for larger number of molecules to come simulaneously for collision are less.

The reactions are either elementary or complex in nature.

Elementary reactions- The reactions taking place in one step are called elementary reactions.

Complex reaction - The reactions which do not take place in one step but take place in a number of steps are called complex reactions. Each step of the complex reaction is an elementary reaction.

In complex reactions, the overall rate of the reaction is controlled by the slowest step in a reaction. It is also called as rate determining step.

• Mechanism of reactions- A series of steps proposed to account for the overall reaction is called the mechanism of reaction.

i) For the reaction,

$$ 2 \mathrm{~N} _{2} \mathrm{O} _{5} \longrightarrow 4 \mathrm{NO} _{2}+\mathrm{O} _{2} $$

experimentally, it is found that

$$ \text { Rate }=\mathrm{k}\left[\mathrm{N} _{2} \mathrm{O} _{5}\right] $$

This shows that the slowest step involves only one molecule of $\mathrm{N} _{2} \mathrm{O} _{5}$. Thus, the order of reactions is 1 .

hence, the probable mechanism is

$$ \begin{gathered} \mathrm{N} _{2} \mathrm{O} _{5} \xrightarrow{\text { Slow }} \mathrm{NO} _{2}+\mathrm{NO} _{3} \\ \mathrm{~N} _{2} \mathrm{O} _{5}+\mathrm{NO} _{3} \xrightarrow{\text { Fast }} 3 \mathrm{NO} _{2}+\mathrm{O} _{2} \end{gathered} $$

ii) For reaction,

$$ \begin{aligned} & 2 \mathrm{NO} _{2}+\mathrm{F} _{2} \longrightarrow 2 \mathrm{NO} _{2} \mathrm{~F} \\ & \text { Rate }=\mathrm{k}\left[\mathrm{NO} _{2}\right]\left[\mathrm{F} _{2}\right] \end{aligned} $$

The order of reaction is two, Hence, the probable mechanism is

$$ \begin{aligned} & \mathrm{NO} _{2}+\mathrm{F} _{2} \xrightarrow{\text { Slow }} \mathrm{NO} _{2} \mathrm{~F}+\mathrm{F} \\ & \mathrm{NO} _{2}+\mathrm{F} \xrightarrow{\text { Fast }} \mathrm{NO} _{2} \mathrm{~F} \end{aligned} $$

CHEMICAL KINETICS

Difference between order and molecularity of reaction

Theories of Reaction Rates: The two well known theories to explain the rates of different reactions are:

(1) Collision theory

(2) Transition state theory or Theory of Absolute reaction rates

1) Collision theory

i) It is based upon the kinetic theory of gases according to which the molecules of a gas are continuously moving and hence colliding with each other.

ii) The number of collisions that takes place per second per unit volume of the reaction mixture is known as collision frequency (Z). The value of collision frequency is very high of the order of $10^{25}$ to $10^{28}$ in case of binary collisions.

iii) Every collision does not bring a chemical change. The collisions that actually produce the product are effective collisions. The effective collisions, which bring chemical change, are few in comparison to the total number of collisions. The collisions that do not form a product are ineffective elastic collisions, i.e., molecules just collide and disperse in different directions with different velocities.

iv) For a collision to be effective, the following two barriers are to be cleared,

Fig 4: Energy of reactants

a) Energy barrier: “The minimum amount of energy which the colliding molecules must possess as to make the chemical reaction to occur, is known as threshold energy”.

• In Fig. 4 ‘E’ corresponds to fraction of molecules capable of bringing effective collision.

• There is an energy barrier for each reaction. The reacting species must be provided with sufficient energy to cross the energy barrier.

b) Orientation barrier: The colliding molecules should also have proper orientation so that the old bonds may break and new bonds are formed.

For example, $\mathrm{NO} _{2}(\mathrm{~g})+\mathrm{NO} _{2}(\mathrm{~g}) \rightarrow \mathrm{N} _{2} \mathrm{O} _{4}(\mathrm{~g})$. During this reaction, the products are formed only when the colliding molecules have proper orientation at the time of collisions. These are called effective collisions. (Fig. 5)

Fig. 5: Role of orientation of molecules during collision

v) Thus, the main points of collision theory are as follows,

a) For a reaction to occur, there must be collisions between the reacting species.

b) Only a certain fraction of the total number of collisions is effective in forming the products.

c) For effective collisions, the molecules should possess sufficient energy as well as orientation.

vi) The fraction of effective collisions, under ordinary conditions may vary from nearly zero to about one for ordinary reactions. Thus, the rate of reaction is proportional to:

a) The number of collisions per unit volume per second (Collision freqeuncy, Z) between the reacting species

b) The fraction of effective collisions (Properly oriented and possessing sufficient energy). If $Z _{A B}$ is the collision frequency $P$ is the orientation factor then.

$\mathrm{R}=\mathrm{PZ} _{\mathrm{AB}} \mathrm{e}^{-\mathrm{Ea} / R T}$

which is similar to Arrhenius equation, given as

$$ \mathrm{R}=A \mathrm{e}^{-\mathrm{Ea} / \mathrm{RT}} $$

on comparing these two equation

$$ A=P Z _{A B} $$

where the pre-exponential factor $A$ is a constant called frequency factor, which gives the total number of collisions per second per unit volume, $\mathrm{E} _{\mathrm{a}}$ is the activation energy, $R$ is gas constant, $k$ is the rate constant at temperature T. $A$ and $\mathrm{E} _{\mathrm{a}}$ are called Arrhenius parameters.

• Logarithmic form of Arrhenius equation- Taking logarithm of both sides of Arrhenius equation, we get

$$ \begin{aligned} & \ln k=\ln A-\dfrac{E _{a}}{R T} \\ & \text { or } \quad \log k=\log A-\dfrac{E _{a}}{2.303 R T} \end{aligned} $$

If $k$ is rate constant at $T _{1}$ and $k _{2}$ at $T _{2}$, then we get

$$ \log \dfrac{k _{2}}{k _{1}}=\dfrac{E _{a}}{2.303 R}\left(\dfrac{1}{T _{1}}-\dfrac{1}{T _{2}}\right)=\dfrac{E _{a}}{2.303 R}\left(\dfrac{T _{2}-T _{1}}{T _{1} T _{2}}\right) $$

Arrhenius equation can also be written in the form

$$ \dfrac{d \ln K}{d T}=\dfrac{E}{R T^{2}} $$

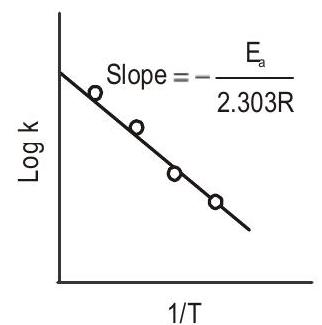
Fig 6: Plot of $\log \mathrm{k} v / \mathrm{s} 1 / \mathrm{t}$

• In the Arrhenius euqation,

$$ \mathrm{k}=\mathrm{Ae}^{-\mathrm{Ea} / \mathrm{RT}} $$

the exponential factor is dimensionless

$$ \left(\dfrac{E _{a}}{R T}=\dfrac{J m l^{-1}}{\left(J K^{1} m l^{-1}\right)(K)}\right) $$

therefore, pre-exponential factor $A$ has the same units as that of the rate constant, e.g., for a first order reaction, $\mathrm{k}$ and $\mathrm{A}$ both have the units $\mathrm{S}^{-1}$, That is why $\mathrm{A}$ is called ‘frequency factor’.

• In the Arrhenius equation, when $\mathrm{T}=\infty, \mathrm{k}=\mathrm{Ae}^{-\mathrm{E} _{\mathrm{a}} / \mathrm{RT}}=\mathrm{A}$. When $\mathrm{E} _{\mathrm{a}}=0, \mathrm{~K} _{\mathrm{a}}=\mathrm{A}$, i.e., rate of reaction becomes independent of temperature.

Activation energy

The minimum extra amount of energy absorbed by the reactant molecules so that their energy becomes equal to threshold value is called activation energy . In other words, activation energy is the difference between the threshold energy and the average kinetic energy of the reactant molecules, i.e.,

Activation energy $=$ Threshold energy-Average kinetic energy of the reactants

Evidently, lesser is the activation energy, faster is the reaction or greater is the activation energy, slower is the reaction

The physical meaning of the activation energy is that it is the minimum relative kinetic energy which the reactant molecules must possess for changing into the products molecules during their collision. This means that the fraction of successful collision is equal to $\mathrm{e}^{-\mathrm{Ea} / \mathrm{RT}}$ called Boltzmann factor.

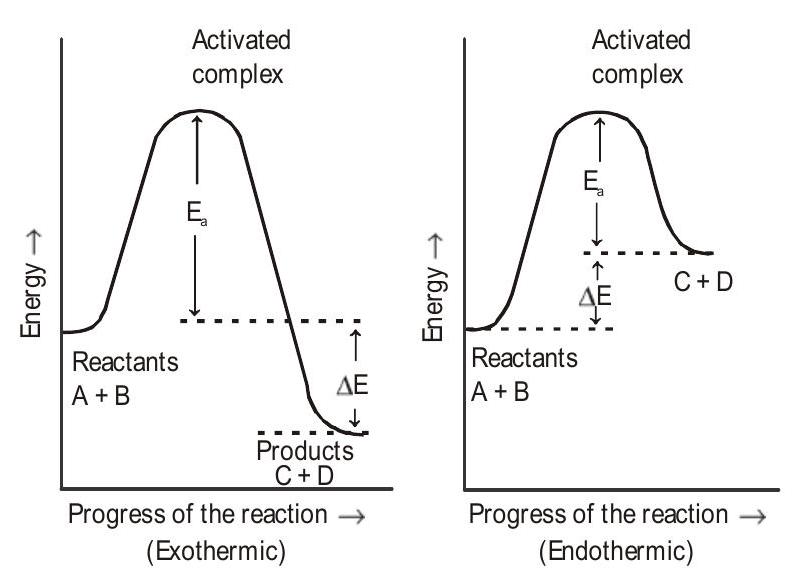
Fig. 7: Activation energy of exothermic and endothermic reactions

Limitations of Collision theory:-

(a) It is applicable to simple gaseous reactions only.

(b) It is supposed that only the kinetic energy of the colliding molecules contribute to the energy required for passing the energy barrier: There is no reason why rotational and vibrational energies of molecules should be ignored.

(c) The collision theory doesn’t talk about the manner in which old bonds are broken and new bonds are formed.

(d) There is no method for determining the number of molecules colliding with proper orientation.

Transition state theory: According to this theory, before the reacting molecules change into product, they form intermediates, called activated complex which has energy higher than both the reactants and products. The activated complex is supposed to be in equilibrium with reactant molecules i.e., it can either return to the initial reactants or proceed to form the products.

This can be represented as:

$\mathrm{A}+\mathrm{B}\rightleftharpoons \underset{\substack{\text { Activated } \\ \text { complex }}} {\mathrm{X}^\#} \xrightarrow{\mathrm{k}} \text { Product }$

By using some fundamental properties of the reacting molecules, it was shown by Eyring that the rate constant (k) for any reaction irrespective of its order or molecularity is given by

$ \mathrm{k}=\dfrac{\mathrm{kT}}{\mathrm{Nh}} \mathrm{K} $

Where $\mathrm{R}=$ Gas constant

$\mathrm{T}=$ Absolute temperature

$\mathrm{N}=$ Avogadro’s number

$\mathrm{h}=$ Planck constant

$\mathrm{K}=$ Equilibrium constant for the formation of the activated complex from the reacting molecules

$k=\dfrac{\left[X^{\#}\right]}{[A][B]}$

Also, $K=e^{-\omega^{\sharp} / k T}$

$ \begin{aligned} & k=\dfrac{R T}{N h} e^{-\Delta G^{\#} / R T} \\ & k=\dfrac{R T}{N h} e^{-\Delta H^{\#} / R T} e^{\Delta S^{\#} / R} \end{aligned} $

Greater is the value of free energy of activation for a reaction, the slower will be the reaction.

Advantage over collision theory

i) In collision theory, the factor ’ $p$ ’ was introduced arbitrarily whereas in transition state theory, it has been justified in terms of entropy of activation $\Delta \mathrm{s}^{\#}$.

ii) The concept of formation of activated complex is more appropriate than assuming that the molecules collide and form products.

Solved Examples

Question 1. For the reaction

$$ 2 \mathrm{~A}+\mathrm{B} \longrightarrow 3 \mathrm{C}+\mathrm{D} $$

The rate of reaction $\bigg \{\dfrac{-d[A]}{d t}\bigg \}$ is $4 \times 10^{-3} \mathrm{Ms}^{-1}$. What is the value of $\dfrac{-d[B]}{d t}$ and $\dfrac{d[C]}{d t}$

Question 2. $56 \mathrm{~g}$ of $\mathrm{N} _{2}$ and $17 \mathrm{~g} \mathrm{NH} _{3}$ are present in $2 \mathrm{~L}$ flask. What are their active masses?

Question 3. For a reaction

$2 A+B \longrightarrow C \text {; following data were collected }$

Calculate the overall order of the reaction and rate constant

Question 4. The decomposition of the following reaction follows first order kinetics

$$ 3 \mathrm{~A}(\mathrm{~g}) \longrightarrow 2 \mathrm{~B}(\mathrm{~g})+2 \mathrm{C}(\mathrm{g}) $$

At the begining of the reaction, only $A$ is present. The pressure developed after $10 \mathrm{~min}$. and infinte time are 3.5 and 4 atm respectively. Calculate $t _{75}$.

Question 5. The vapour pressure of two miscible liquids ’ $X$ ’ and ’ $Y$ ’ are 100 and 200 atm respectively. On addition of $Y$ to $X$, $X$ polymerises by following first order kinetics. After mixing 10 moles of $X$ with 15 moles of $Y$, the reaction is arrested by adding 0.65 moles of a non-volatile solute after $75 \mathrm{~min}$. The final vapour pressure of the solution is $220 \mathrm{~atm}$. Calculate the rate constant. Also, state approximations made during the computation.

Question 6. A reaction occurs via two paths. the value of rate constants and activation energies for two paths are $k _{1}, E _{1}$ and $k _{2}, E _{2}$ respectively. The value of rate constant $k _{1}$ is greater than $k _{2}$ at temperature $T _{1}$. At temperature $T _{2}$, the rate constants become $k _{1}^{\prime}$ and $k _{2}^{\prime}$ respectively. Show that a relation between rate constants at two temperatures is expressed as

$\dfrac{\mathrm{k} _{1}^{\prime}}{\mathrm{k} _{1}}<\dfrac{\mathrm{k} _{2}^{\prime}}{\mathrm{k} _{2}}$

Question 7. Consider a reaction

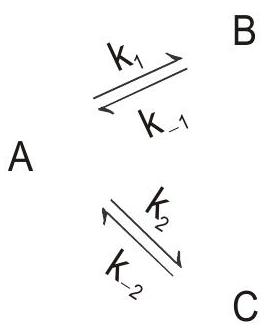
Show that concentration of ’ $A$ ’ at equitibrium is given by the expression

$$ [A] _{e q}=\dfrac{k _{-1}[B] _{e q}+k _{-2}[C] _{e q}}{k _{1}+k _{2}} $$

PRACTICE QUESTIQNS

Question 1- During the kinetic study of the reaction $2 A+B \rightarrow C+D$, following results were obtained

Based on the above data which one of the following is correct?

(a) rate $=k[A][B]^{2}$

(b) rate $=k[A]^{2}[B]$

(c) rate $=k[A][B]$

(d) rate $=k[A]^{2}[B]^{2}$

Question 2- In the reaction $2 \mathrm{NO}+\mathrm{Cl} _{2} \rightarrow 2 \mathrm{NOCl}$, it has been found that doubling the concentration of both the reactants increases the rate by a factor of eight but doubling the chlorine concentration alone only doubles that rate. Which of the following statement is correct?

(a) The reaction is second order in $\mathrm{Cl} _{2}$

(b) The reaction is first order in NO

(c) The overall order of reaction is 2

(d) The overall order of reaction is 3

Question 3- Consider the reaction:

$\mathrm{Cl} _{2}(\mathrm{aq})+\mathrm{H} _{2} \mathrm{~S}(\mathrm{aq}) \rightarrow \mathrm{S}(\mathrm{s})+2 \mathrm{H}^{+}(\mathrm{aq})+2 \mathrm{Cl}^{-}(\mathrm{aq})$

The rate equation for this reaction is rate $=\mathrm{k}\left[\mathrm{Cl} _{2}\right]\left[\mathrm{H} _{2} \mathrm{~S}\right]$

Which of these mechanisms is/are consistent with this rate equation?

A. $\mathrm{Cl} _{2}+\mathrm{H} _{2} \mathrm{~S} \rightarrow \mathrm{H}^{+}+\mathrm{Cl}^{-}+\mathrm{Cl}^{+}+\mathrm{HS}^{-}$(slow) $\mathrm{Cl}^{+}+\mathrm{HS}^{-} \rightarrow \mathrm{H}^{+}+\mathrm{Cl}^{-}+\mathrm{S}$ (fast)

B. $\mathrm{H} _{2} \mathrm{~S} \rightleftharpoons \mathrm{H}^{+}+\mathrm{HS}^{-}$(fast equilibrium)

$\quad \mathrm{Cl} _{2}+\mathrm{HS}^{-} \rightarrow 2 \mathrm{Cl}^{-}+\mathrm{H}^{+}+\mathrm{S} \text { (slow) }$

(a) Neither A nor B

(b) A only

(c) B only

(d) Both $A$ and $B$

Question 4- The rate of a gaseous reaction is halved when the volume of the vessel is doubled. The order of reaction is

(a) 0

(b) 1

(c) $3 / 2$

(d) $4 / 3$

Question 5- For the reaction, $A+B \rightarrow C+D$, if concentration of $A$ is doubled without altering the concentration of $B$, the rate gets doubled. If the concentration of $B$ is increased by nine times concentration of $\mathrm{A}$, the rate gets tripled. The order of reaction is

(a) 2

(b) 1

(c) $3 / 2$

(d) $4 / 3$

Question 6- The reaction $X \longrightarrow$ product follows first order kinetics. In 40 minutes, the concentration of $X$ changes from $0.1 \mathrm{M}$ to $0.025 \mathrm{M}$, then the rate of reaction when concentration of $\mathrm{X}$ is $0.01 \mathrm{M}$ is

(a) $1.73 \times 10^{-4} \mathrm{M} / \mathrm{min}$

(b) $3.47 \times 10^{-5} \mathrm{M} / \mathrm{min}$

(c) $3.47 \times 10^{-4} \mathrm{M} / \mathrm{min}$

(d) $1.73 \times 10^{-5} \mathrm{M} / \mathrm{min}$

Question 7- Half-life of a reaction is found to be inversely proportional to the cube of initial concentration. The order of reaction is

(a) 4

(b) 3

(c) 5

(d) 2

Question 8- Consider the reaction, $2 \mathrm{~A}+\mathrm{B} \longrightarrow$ Products: When concentration of $\mathrm{B}$ alone was doubled, the half-life did not change. When the concentration of $A$ alone was doubled, the rate increased by two times. The unit of rate constant for this reaction is

(a) $\mathrm{s}^{-1}$

(b) $\mathrm{L} \mathrm{mol}^{-1} \mathrm{~s}^{-1}$

(c) no unit

(d) $\mathrm{mol} \mathrm{L}^{-1} \mathrm{~s}^{-1}$

Question 9- For the reaction $R \longrightarrow P$, a graph of $[R]$ against time is found to be a straight line with negative slope. What is the order of reaction?

(a) Second order

(b) Third order

(c) First order

(d) Zero order

Question 10- In the reaction,

$\mathrm{BrO} _{3}^{-}(\mathrm{aq})+5 \mathrm{Br}^{-}(\mathrm{aq})+6 \mathrm{H}^{+} \longrightarrow 3 \mathrm{Br} _{2}+3 \mathrm{H} _{2} \mathrm{O}$

the rate of appearance of bromine $\left(\mathrm{Br} _{2}\right)$ is related to the rate of disappearance of bromide ions as follows:

(a) $\dfrac{d\left[B r _{2}\right]}{d t}=-\dfrac{5 d\left[B r^{-}\right]}{d t}$

(b) $\dfrac{d\left[B r _{2}\right]}{d t}=\dfrac{5}{3} \dfrac{d\left[B r^{-}\right]}{d t}$

(c) $\dfrac{d\left[\mathrm{Br} _{2}\right]}{d t}=\dfrac{3}{5} \dfrac{d\left[\mathrm{Br}^{-}\right]}{d t}$

(d) $\dfrac{d\left[B r _{2}\right]}{d t}=-\dfrac{3}{5} \dfrac{d\left[\mathrm{Br}^{-}\right]}{d t}$

Question 11- $3 \mathrm{BrO}^{-} \longrightarrow \mathrm{BrO} _{3}^{-}+2 \mathrm{Br}^{-}$

$$ \text { If }-\dfrac{\left[\mathrm{BrO}^{-}\right]}{d t}=k _{1}\left[\mathrm{BrO}^{-}\right]^{2},+\dfrac{d\left[\mathrm{BrO} _{3}^{-}\right]}{d t}=k _{2}\left[\mathrm{BrO}^{-}\right]^{2}, \dfrac{d\left[\mathrm{BrO}^{-}\right]}{d t}=k _{3}\left[\mathrm{BrO}^{-}\right]^{2} $$

the correct relation between $k _{1}, k _{2}$ and $k _{3}$ is

(a) $3 \mathrm{k} _{1}=\mathrm{k} _{2}=2 \mathrm{k} _{3}$

(b) $k _{1}=3 k _{2}=1.5 k _{3}$

(c) $k _{1}=k _{2}=k _{3}$

(d) $2 k _{1}=3 k _{2}=k _{3}$

Question 12- The rate constant of nth order has units

(a) litre $^{1-\mathrm{n}} \mathrm{mol}^{\mathrm{n}} \mathrm{s}^{-1}$

(b) $\mathrm{mol}^{1-\mathrm{n}} \operatorname{litre}^{1-\mathrm{n}} \mathrm{s}^{-1}$

(c) $\mathrm{mol}^{1-\mathrm{n}} \operatorname{litre}^{n} \mathrm{s}^{-1}$

(d) $\mathrm{mol}^{1-n} \operatorname{litre}^{n-1} s^{-1}$

Question 13- A hypothetical reaction $\mathrm{A} _{2}+\mathrm{B} _{2} \longrightarrow 2 \mathrm{AB}$ follows the mechanism as given below:

$A _{2} \rightleftharpoons A+A$ (fast)

$\mathrm{A}+\mathrm{B} _{2} \longrightarrow \mathrm{AB}+\mathrm{B}$ (slow)

$\mathrm{A}+\mathrm{B} \longrightarrow \mathrm{AB}$ (fast)

The order of the overall reaction is:

(a) 2

(b) 1

(c) $1 \dfrac{1}{2}$

(d) 0

Question 14- For a first order reaction, $\mathrm{t} _{\mathrm{av}}$ (average life time), $\mathrm{t} _{50 \%}$ and $\mathrm{t} _{75 \%}$ are in the order:

(a) $\mathrm{t} _{50}<\mathrm{t} _{\mathrm{av}}<\mathrm{t} 75$

(b) $\mathrm{t} _{50}<\mathrm{t} _{75}<\mathrm{t} _{\mathrm{av}}$

(c) $\mathrm{t} _{\mathrm{av}}<\mathrm{t} _{50}<\mathrm{t} _{75}$

(d) $\mathrm{t} _{\mathrm{av}}=\mathrm{t} _{50}<\mathrm{t} _{75}$

PRACTICE QUESTIONS

Question 1- The rate constants $k _{1}$ ad $k _{2}$ for two different reactions are $10^{16} \cdot \mathrm{e}^{-2000 / T}$ and $10^{15} . \mathrm{e}^{-1000 / T}$ respectively. The temperature at which $\mathrm{k} _{1}=\mathrm{k} _{2}$ is

(a) $1000 \mathrm{~K}$

(b) $\dfrac{2000}{2.303} K$

(c) $2000 \mathrm{~K}$

(d) $\dfrac{1000}{2.303} K$

Question 2- For a first order reaction $A \longrightarrow P$, the temperature $(T)$ dependent rate constant $(k)$ was found to follow the equation $\log k=-(2000) \dfrac{1}{T}+6.0$. The pre-exponential factor $A$ and the activation energy $E _{a}$, respectively, are

(a) $1.0 \times 10^{6} \mathrm{~s}^{-1}$ and $9.2 \mathrm{~kJ} \mathrm{~mol}^{-1}$

(b) $6.0 \mathrm{~s}^{-1}$ and $16.6 \mathrm{~kJ} \mathrm{~mol}^{-1}$

(c) $1.0 \times 10^{-1} \mathrm{~s}^{-1}$ and $38.3 \mathrm{~kJ} \mathrm{~mol}^{-1}$

(d) $1.0 \times 10^{6} \mathrm{~s}^{-1}$ and $16.6 \mathrm{~kJ} \mathrm{~mol}^{-1}$

Question 3- For the first order gas phase decomposition reaction,

$\mathrm{A}(\mathrm{g}) \longrightarrow \mathrm{B}(\mathrm{g})+\mathrm{C}(\mathrm{g})$

if $P _{0}$ is the inital pressure of $A$ and $P _{1}$ is total pressure after time $t$, then

(a) $k=\dfrac{2.303}{t} \log \dfrac{P _{0}}{P _{t}}$

(b) $k=\dfrac{2.303}{t} \log \dfrac{P _{0}}{P _{t}-P _{0}}$

(c) $k=\dfrac{2.303}{t} \log \dfrac{P _{0}}{P _{t}-2 P _{0}}$

(d) $\quad k=\dfrac{2.303}{t} \log \dfrac{P _{0}}{2 P _{0}-P _{t}}$

Question 4- $\mathrm{SO} _{2} \mathrm{Cl} _{2} \longrightarrow \mathrm{SO} _{2}+\mathrm{Cl} _{2}$ is a first order gas reaction with $\mathrm{k}=2.2 \times 10^{-5} \mathrm{~s}^{-1}$ at $320^{\circ} \mathrm{C}$. The percentage of $\mathrm{SO} _{2} \mathrm{Cl} _{2}$ decomposed on heating for 90 minutes is

(a) 1.118

(b) 0.1118

(c) 18.11

(d) 11.18

Question 5- The following data is obtained during the first order thermal decomposition of

$2 \mathrm{~A}(\mathrm{~g}) \longrightarrow \mathrm{B}(\mathrm{g})+\mathrm{C}(\mathrm{s})$

at constant volume and temperature

The rate constant in $\mathrm{min}^{-1}$ is

(a) 0.0693

(b) 6.93

(c) 0.00693

(d) 69.3

Question 6- The energies of activation for forward and reverse reactions for $\mathrm{A} _{2}+\mathrm{B} _{2} \rightleftharpoons 2 \mathrm{AB}$ are $180 \mathrm{~kJ}$ $\mathrm{mol}^{-1}$ and $200 \mathrm{~kJ} \mathrm{~mol}$ respectively. The presence of a catalyst lowers the activitation energy of both (forward and reverse) reactions by $100 \mathrm{~kJ} \mathrm{~mol}^{-1}$. The enthalphy change of the reaction $\left(\mathrm{A} _{2}+\mathrm{B} _{2} \longrightarrow 2 \mathrm{AB}\right)$ in the presence of catalyst will be (in $\mathrm{kJ} \mathrm{mol}^{-1}$ )

(a) 20

(b) 300

(c) 120

(d) 280

Question 7- The rate constant of a reaction increases by 55 when its temperature is raised from $27^{\circ}$ to $28^{\circ} \mathrm{C}$. The activation energy of the reaction is

(a) $36.6 \mathrm{~kJ} / \mathrm{mol}$

(b) $16.6 \mathrm{~kJ} / \mathrm{mol}$

(c) $46.6 \mathrm{~kJ} / \mathrm{mol}$

(d) $26.6 \mathrm{~kJ} / \mathrm{mol}$

Question 8- The activation energy of a reaction is $9 \mathrm{kcal} / \mathrm{mole}$. The increase in the rate constant when its temperature is raised from 295 to $300 \mathrm{~K}$ is approximately

(a) $10 \%$

(b) $50 \%$

(c) $100 \%$

(d) $25 \%$

Question 9- A chemical reaction was carried out at $300 \mathrm{~K}$ and $280 \mathrm{~K}$. The rate constants were found to be $k _{1}$, and $k _{2}$ respectively. Then

(a) $\mathrm{k} _{2}=4 \mathrm{k} _{1}$

(b) $k _{2}=2 k _{1}$

(c) $\mathrm{k} _{2}=0.25 \mathrm{k} _{1}$

(d) $k _{2}=0.5 k _{1}$

Question 10- What is the time required for a first order reaction to be $99 \%$ complete compared to the time taken for the reaction to be $90 \%$ complete?

(a) There is no change

(b) Time taken is double

(c) Time taken is triple

(d) Time required is half the initial value