Purification and Characterisation of organic compounds

Organic compounds, obtained from natural sources or synthesized in laboratory, are seldom pure. They are generally contaminated with impurities and therefore need purification. The principle and method of purification depend upon the nature of the substance (solid or liquid) and the type of impurity present in it.

Methods of Purification:

1.Sublimation

It involves direct conversion of a solid into the gaseous state on heating without passing through the intervening liquid state and vice versa on cooling. The process is useful to purify the compounds which sublime on heating and are associated with non volatile (solids) impurities.

Example : Camphor, naphthalene, anthracene, benzoic acid, iodine.

2.Crystallisation

This is the most commonly used technique for purification of solid compounds in laboratory. Crystals have definite geometrical shapes and are the purest form of a substance. The process by which an impure compound is converted into crystals is known as crystallisation. This method is based upon the principle that an organic compound is soluble in a suitable solvent / mixture of solvents at elevated temperature and insoluble at room temperature. For proper crytallisation choice of solvent is crucial. A suitable solvent :-

Should not react chemically with the impurities.

Should dissolve more of the substance on heating than at room temperature.

Either should not dissolve impurities or if it dissolves, it should be such an extent that impurities remain in solution (mother liquor) upon crystallisation.

Water, alcohol, chloroform, ether, benzene, acetone, ethyl acetate are commenly used solvents for crystallisation.

2.1 Fractional Crystallisation

This is the process of separation of different components of a mixture by repeated crystallisation. This method is employed to separate and purify two or more compounds having different solubilities in the some solvent.

3.Simple Distillation

It involves conversion of a liquid into vapours by heating followed by condensation of these vapours by cooling. This method is based upon the difference in boiling points of impurities and the compound to be purified. It is commonly used for the liquids, sufficiently stable at their boiling points and contain non volatile impurities.

Example: Benzene, ethanol, acetone, toluene, xylenes, ${\mathrm{CHCl}}_3,{\mathrm{CCl}}_4$ etc.

Simple distillation can also be employed for separation and purification of a mixture of two or more miscible organic liquids having sufficient difference in their boiling points. Upon distillation of such mixture, the more volatile liquid distils over first while the less volatile liquid distils over afterwards.

Example: Mixture of ether (b.p. $308\mathrm{K}$ ) and toluene (b.p. $384\mathrm{K}$ )

Mixture of hexane (b.p. $342\mathrm{K}$ ) and toluene.

Mixture of benzene (b.p. $353\mathrm{K}$ ) and aniline (b.p. $457\mathrm{K}$ )

3.1 Fractional Distillation

This method is employed when the boiling points of liquids of a mixture are very close to each other and separation can’t be done by simple distillation. Fractional distillation is carried out in fractionating column, specially designed for the purpose to increase the cooling surface area and to provide obstructions to the ascending vapours and descending liquid. This allows repeated and successive evaporations and condensations.

Example : This method is used to separate (1) petroleum into its useful fractions such as gasoline, kerosene oil, diesel oil etc. (2) acetone (b. p. $329\mathrm{K}$ ) and methyl alcohol (b.p. 338 K) from pyroligneous acid obtained by destructive distillation of wood.

3.2 Distillation under reduced pressure / Vacuum Distillation

This method is used for purification of high boiling liquids and the liquids which decompose at or below their boiling points. We know that a liquid boils when its vapour pressure becomes equal to the external pressure. Therefore, by reducing the external pressure acting on it, boiling point can be reduced and distillation can easily be achieved without decomposition. Water pumps / vacuum pumps are commonly used in laboratory to reduce the pressure above the liquid mixtures to be separated.

Example : Glycerol generally decomposes at its boiling point ( $563\mathrm{K}$ ). It can be distilled without decomposition at $453\mathrm{K}$ under $12\mathrm{mm}\mathrm{Hg}$ pressure.

3.3 Steam Distillation

This is very convenient method for separation and purification of organic compounds (solid / liquid) from nonvolatile organic / inorganic impurities. Method is applicable to the compounds that are volatile in steam, insoluble in water, boil above $373\mathrm{K}$ at $760\mathrm{mm}$, decompose at / below its boiling point and contain nonvolatile impurities. Unlike Vacuum distillation, steam distillation makes the high boiling compound to distil at low temperature and avoids its decomposition, without reducing the total pressure acting on the solution.

The impure organic compound, mixed with water is taken in a round bottom flask and steam is passed through it. When the combined vapour pressure of mixture becomes equal to the atmospheric pressure, the mixture starts boiling. At this temperature, steam mixed with the vapours of compound passes over to the condenser, gets condensed and then collected in the receiver. The distillate contains the mixture of desired compound and water which are then separated with the help of a separating funnel.

Example : Aniline, nitrobenzene, essential oils, o-nitrophenol and bromobenzene can be purified by this process.

4.Differential Extraction

This method is also known as solvent extraction. The technique is used to separate organic compounds (solid / liquid) from their aqueous solutions. In a separating funnel, the aqueous solution of organic compound is mixed with a small quantity of the suitable organic solvent. Solvent should be immiscible with water and highly miscible with the organic compound. The separating funnel is stoppered and the contents are shaken thoroughly. The organic compound, being more soluble is solvent, gets dissolved in it. The funnel is allowed to stand for some time so that the water layer separates out from the organic layer. Both the layers are separated and the process is repeated several time with the water layer in order to extract the entire / maximum amount of the organic compound. Organic compound is then recovered from the solvent through distillation / evaporation. Larger the number of extractions, greater the amount of the compound extracted. Benzene, ether, chloroform and carbon tetrachloride are some generally used solvents for extraction.

Example : Benzoic acid can be separated from its aqueous solution using benzene as a solvent.

5.Chromatography

This is the modern analytical technique, discovered by Tswett (1906) to separate the components of a mixture. The technique is based upon the principle of selective adsorption of various components of a mixture between the two phases; stationary phase and mobile phase. It is widely used, not only for separation but for purification, identification and characterization of the components present in a mixture.

Depending upon the nature of the stationary and the mobile phases, the different types of chromatographic techniques, commonly used are given in the table.

Some common types of chromatographic techniques

Some common terms used in chromatography

(i) Mobile phase

Phase that moves through the stationary phase. It may be liquid or a gas.

(ii) Stationary phase

It is the substance fixed in place for the chromatography. It may be solid or liquid supported on solid. For example silica layer in TLC.

(iii) Eluent

The solvent used in column chromatography is know as eluent. During elution (running) of eluent (solvent) from the column, the most weakly adsorbed component is eluted first by least polar solvent while more strongly adsorbed component is eluted later by highly polar solvents.

(iv) $\mathbf{R}\mathbf{f}$ Value

It is the ratio of the distance travelled by a component to the distance travelled by the solvent front. It is a constant for a given component under a given set of conditions. Therefore, it is possible to identify the various components by determining their $\mathrm{Rf}$ values.

Qualitative analysis of organic compounds

It is detection of various elements present in the compound.

1.Detection of carbon and hydrogen

Organic compound is heated with dry cupric oxide and produced vapours are passed through lime water or anhydrous ${\mathrm{CuSO}}_4$. If lime water turns milky, it shows presence of ${\mathrm{CO}}_2$ or carbon. If anhydrous ${\mathrm{CuSO}}_4$, turns blue it shows presence of ${\mathrm{H}}_2\mathrm{O}$ or hydrogen

Compound $+\mathrm{CuO}\stackrel{\mathrm{\Delta }}{\to }{\mathrm{CO}}_2+{\mathrm{H}}_2\mathrm{O}+\mathrm{Cu}$

${\mathrm{CO}}_2+\mathrm{Ca}(\mathrm{OH}{)}_2⟶{\mathrm{CaCO}}_3\downarrow +{\mathrm{H}}_2\mathrm{O}$

lime water $$ milkiness

${\mathrm{H}}_2\mathrm{O}+{\mathrm{CuSO}}_4⟶{\mathrm{CuSO}}_4\cdot 5{\mathrm{H}}_2\mathrm{O}$

colourless $$ blue

2.Detection of other elements

Nitrogen, sulphur & halogen are detected by Lassaigne’s test. Compound is heated with sodium metal and poured in cold & distilled water. This, after heating for sometime, gives Lassaigne’s solution or sodium extract. In Lassaigne solution, elements present in compound, get converted from their covalent to easily identifiable ionic forms

e.g. $\mathrm{Na}+\mathrm{C}+\mathrm{N}⟶\mathrm{NaCN}$

$\mathrm{Na}+\mathrm{S}⟶{\mathrm{Na}}_2\mathrm{S}$

$\mathrm{Na}+\mathrm{X}⟶\mathrm{NaX}$ = halogen

(i) Test for nitrogen

Lassaigne extract is boiled with ${\mathrm{FeSO}}_4$ and acidified with dil. ${\mathrm{H}}_2{\mathrm{SO}}_4$. Formation of prussian blue colour confirms the presence of $\mathrm{N}$.

$6\mathrm{NaCN}+{\mathrm{FeSO}}_4⟶{\mathrm{Na}}_4[\mathrm{Fe}(\mathrm{CN}{)}_6]+{\mathrm{Na}}_2{\mathrm{SO}}_4$

$$ sodium hexacyanoferrate(II)

$3{\mathrm{Na}}_4[\mathrm{Fe}(\mathrm{CN}{)}_6]+4{\mathrm{Fe}}^{+3}⟶{\mathrm{Fe}}_4{[\mathrm{Fe}(\mathrm{CN}{)}_6]}_3+12{\mathrm{Na}}^{+}$

$$ ferri ferrocyanide (prussian blue)

Note : On heating with ${\mathrm{FeSO}}_4$, sodium ferrocyanide is formed and at the same time some ferrous $({\mathrm{Fe}}^{+2}$ ) ions are oxidized to ferric ( ${\mathrm{Fe}}^{+3}$ ) ion which react with sodium ferrocyanide to give ferri ferrocyanide.

(ii) Test for sulphur

(a) Sodium extract is acidified with acetic acid and lead acetate is added to it which gives black ppt of PbS and shows the presence of ’ $S$ ‘.

${\mathrm{Na}}_2\mathrm{S}+{\left({\mathrm{CH}}_3\mathrm{COO}\right)}_2\mathrm{Pb}⟶\mathrm{PbS}+2{\mathrm{CH}}_3\mathrm{COONa}$ black (b) Lassaigne extract reacts with sodium nitroprusside and gives violet colour.

$${\mathrm{Na}}_2\mathrm{S}+{\mathrm{Na}}_2(\mathrm{Fe}(\mathrm{CN}{)}_5\mathrm{NO})⟶\underset{\text{ violet }}{⟶}{\mathrm{Na}}_4[\mathrm{Fe}(\mathrm{CN}{)}_5\mathrm{NOS}]$$

Note: If ’ $\mathrm{N}$ ’ and ’ $\mathrm{S}$ ’ both are present in compound they form sodium thiocyanate in Lassaigne extract, due to insufficient sodium.

$$\mathrm{Na}+\mathrm{C}+\mathrm{N}+\mathrm{S}\stackrel{\mathrm{\Delta }}{\to }\mathrm{NaSCN}$$

Therefore, during test of ’ $N$ ‘, instead of prussian blue, blood red colouration appears.

$$3\mathrm{NaSCN}+{\mathrm{Fe}}^{+3}⟶\begin{array}{rlr}& \text{ ferric thiocyanate (blood red) }& \mathrm{Fe}(\mathrm{SCN}{)}_3+3{\mathrm{Na}}^{+}\end{array}$$

Note: In excess of sodium metal, sodium thiocyanate decomposes to sodium sulphide & sodium cyanide. Therefore, $L$ E is prepared in excess of sodium.

$$\mathrm{NaSCN}+\mathrm{Na}\text{ (excess) }⟶{\mathrm{Na}}_2\mathrm{S}+\mathrm{NaCN}$$

(iii) Detection of halogens

If ’ $N$ ’ or ’ $S$ ’ present in the compound they may interfere in the ${\mathrm{AgNO}}_3$ test for halogen. Therefore, before the test for halogens, sodium extract is boiled with conc. ${\mathrm{HNO}}_3$ to decompose ${\mathrm{Na}}_2\mathrm{S}$ and $\mathrm{NaCN}$ in the form of ${\mathrm{H}}_2\mathrm{S}$ and $\mathrm{HCN}$.

${\mathrm{Na}}_2\mathrm{S}+\mathrm{Conc}.{\mathrm{HNO}}_3⟶{\mathrm{H}}_2\mathrm{S}\uparrow$

$\mathrm{NaCN}+\mathrm{Conc}.{\mathrm{HNO}}_3⟶\mathrm{HCN}$

Now, sodium extract is treated with ${\mathrm{AgNO}}_3$, which gives precipitate of silver halide

$\mathrm{NaX}+{\mathrm{AgNO}}_3⟶\mathrm{AgX}+{\mathrm{NaNO}}_3$

Sod. extract $$ ppt. $\mathrm{X}=\mathrm{Cl},\mathrm{Br},\mathrm{I}$

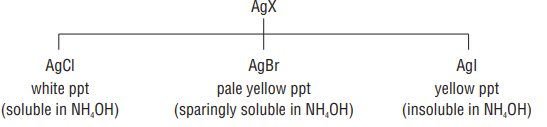
(iv) Detection of phosphorus

Organic compound is fused with sodium peroxide to convert phosphorus into sodium phosphate, which on further reaction with ammonium molybdate & conc. ${\mathrm{HNO}}_3$ gives yellow ppt/colouration of ammonium phosphomolybdate. $2\mathrm{P}$ (From organic compound) $+{\mathrm{Na}}_2{\mathrm{O}}_2⟶\mathrm{\Delta }{\mathrm{Na}}_3{\mathrm{PO}}_4+2{\mathrm{Na}}_2\mathrm{O}$

$$\begin{array}{rl}{\mathrm{Na}}_3{\mathrm{PO}}_4+3{\mathrm{HNO}}_3\stackrel{\mathrm{\Delta }}{\to }& {\mathrm{H}}_3{\mathrm{PO}}_4+3{\mathrm{NaNO}}_3\\ 21{\mathrm{HNO}}_3+{\mathrm{H}}_3{\mathrm{PO}}_4+12{\left({\mathrm{NH}}_4\right)}_2{\mathrm{MoO}}_4⟶& {\left({\mathrm{NH}}_4\right)}_3{\mathrm{PO}}_4\cdot 12{\mathrm{MoO}}_3+21{\mathrm{NH}}_4{\mathrm{NO}}_3+12{\mathrm{H}}_2\mathrm{O}\\ \text{ Amm. molybdate }& \text{ qquad Amm. phospho molybdate (yellow) }\end{array}$$

Quantitative analysis of organic compounds

This is done to calculate the percentage of each element in the compound.

1.Estimation of ’ $\mathrm{C}$ ’ and ’ $\mathrm{H}$ ’ [Liebig’s Method]

A known mass of organic compound in burnt in presence of excess of $\mathrm{CuO}$. ’ $\mathrm{C}$ ’ and ’ $\mathrm{H}$ ’ of the compound oxidize to ${\mathrm{CO}}_2$ and ${\mathrm{H}}_2\mathrm{O}$, respectively. These vapours are passed through weighed ’ $\mathrm{U}$ ’ tubes having anhydrous ${\mathrm{CaCl}}_2$ followed by $\mathrm{KOH}$ solution. ${\mathrm{CaCl}}_2$ absorbs ${\mathrm{H}}_2\mathrm{O}$ while $\mathrm{KOH}$ absorbs ${\mathrm{CO}}_2$. Increase in the mass of ${\mathrm{CaCl}}_2$ and $\mathrm{KOH}$ gives the amount of ${\mathrm{H}}_2\mathrm{O}$ and ${\mathrm{CO}}_2$ produced in combustion which inturn gives the $$ of $\mathrm{H}$ and $\mathrm{C}$ present in the compound.

Calculation of $\mathrm{\%}$ of ’ $\mathrm{C}$ ’ and ’ $\mathrm{H}$ ’ : Suppose mass of compound $=\mathrm{Wg}$

$$\text{ mass of }{\mathrm{CO}}_2/{\mathrm{H}}_2\mathrm{O}\text{ produced }=\mathrm{wg}$$

For ${\mathrm{CO}}_2\equiv \mathrm{C}$

$44\mathrm{g}12\mathrm{g}$

$44\mathrm{g}{\mathrm{CO}}_2$ Contains $12\mathrm{g}$ ’ $\mathrm{C}$ '

w g CO $2_2$ contains $\frac{12}{44}\times \mathrm{w}g$ ’ $C$ '

$$ of ’ $\mathrm{C}$ ’ in $\mathrm{Wg}$ organic compound

For $\mathrm{H}{\mathrm{H}}_2\mathrm{O}\equiv 2\mathrm{H}$

$\begin{array}{ll}18g& 2g\end{array}$

$18\mathrm{g}$ of ${\mathrm{H}}_2\mathrm{O}$ contains $2\mathrm{g}$ of ’ $\mathrm{H}$ '

w g of ${\mathrm{H}}_2\mathrm{O}$ contains $\frac{2}{18}\times \mathrm{wg}$ ’ $\mathrm{H}$ '

$$ of ’ $\mathrm{H}$ ’ in Wg organic compound

2.Estimation of ’ $\mathrm{N}$ '

There are two most commenly used methods (i) Duma’s method (ii) Kjeldahl’s method.

(i) Duma’s Method: This method is applicable to all organic compounds containing nitrogen. organic compound is heated with excess of $\mathrm{CuO}$ to give free nitrogen, ${\mathrm{CO}}_2$ and ${\mathrm{H}}_2\mathrm{O}$

$${\mathrm{C}}_x{\mathrm{H}}_y{\mathrm{N}}_2+\mathrm{CuO}\stackrel{\mathrm{\Delta }}{\to }{\mathrm{XCO}}_2+\frac{\mathrm{Y}}{2}{\mathrm{H}}_2\mathrm{O}+\frac{\mathrm{Z}}{2}{\mathrm{N}}_2+\mathrm{Cu}$$

Now, the above mixture is collected over conc. $\mathrm{KOH}$ solution which absorbs all the gases except ${\mathrm{N}}_2$. The volume of ${\mathrm{N}}_2$ collected is calculated at STP.

$$\begin{array}{ll}\frac{P_1{V}_1}{T_1}=\frac{P_2{V}_2}{T_2}& V_1=\text{ Volume of }{N}_2\text{ gas }\\ & P_1=\text{ Pressure of }{N}_2\text{-Aqueous tension }\\ T_1=\text{ Room temperature }\\ V_2=\frac{P_1{V}_1}{T_1}\times \frac{273}{760}& \text{ Letit be ‘V’ }ml\end{array}$$

Calculation of $\mathrm{\%}$ of ’ $\mathrm{N}$ '

$\therefore 22400\mathrm{mL}$ of ${\mathrm{N}}_2$ at STP weighs $=28\mathrm{g}$

$\mathrm{VmL}$ of ${\mathrm{N}}_2$ at STP weighs $=\frac{28}{22400}\times \mathrm{V}\mathrm{g}$

$\mathrm{\%}$ of N in W g organic compound $=\frac{28}{22400}\times \frac{\mathrm{V}}{\mathrm{W}}\times 100$

(ii) Kjeldahl’s Method

Compound is heated with conc.${\mathrm{H}}_2{\mathrm{SO}}_4$. ’ $\mathrm{N}$ ’ in the compound gets converted into ${\left({\mathrm{NH}}_4\right)}_2{\mathrm{SO}}_4$. Resulting acidic mixture is heated with excess of $\mathrm{NaOH}$ to liberate ${\mathrm{NH}}_3$ gas. ${\mathrm{NH}}_3$ is absorbed in excess of standard ${\mathrm{H}}_2{\mathrm{SO}}_4$ solution. The amount of ${\mathrm{H}}_2{\mathrm{SO}}_4$ consumed tells about the amount of ${\mathrm{NH}}_3$ produced. The volume of acid left, after absorption of ${\mathrm{NH}}_3$, is found by titration against standard alkali solution.

Organic compound $+{\mathrm{H}}_2{\mathrm{SO}}_4⟶{\left({\mathrm{NH}}_4\right)}_2{\mathrm{SO}}_4$

${\left({\mathrm{NH}}_4\right)}_2{\mathrm{SO}}_4+\mathrm{NaOH}⟶{\mathrm{Na}}_2{\mathrm{SO}}_4+2{\mathrm{NH}}_3\uparrow +{\mathrm{H}}_2\mathrm{O}$

${\mathrm{NH}}_3+{\mathrm{H}}_2{\mathrm{SO}}_4⟶{\left({\mathrm{NH}}_4\right)}_2{\mathrm{SO}}_4$

Let the mass of organic compound taken $=\mathrm{Wg}$

Volume of ${\mathrm{H}}_2{\mathrm{SO}}_4$ of normality ${\mathrm{N}}_1={\mathrm{V}}_1\mathrm{mL}$

Volume of $\mathrm{NaOH}$ of normality ${\mathrm{N}}_2$, used $={\mathrm{V}}_2\mathrm{mL}$

Now ${\mathrm{V}}_2\mathrm{mL}$ of ${\mathrm{N}}_1\mathrm{NaOH}={\mathrm{V}}_2\mathrm{mL}$ of ${\mathrm{N}}_1{\mathrm{H}}_2{\mathrm{SO}}_4$ left unused

$\therefore$ Volume of ${\mathrm{H}}_2{\mathrm{SO}}_4$ used up by ${\mathrm{NH}}_3=({\mathrm{V}}_1-{\mathrm{V}}_2)\mathrm{mL}$ of ${\mathrm{N}}_1$ normality $=$ Volume of ${\mathrm{NH}}_3$ evolved

$1000\mathrm{mL}$ of $1\mathrm{N}{\mathrm{NH}}_3$ contains $14\mathrm{g}$ ’ $\mathrm{N}$ '

$({\mathrm{V}}_1-{\mathrm{V}}_2)\mathrm{mL}$ of $1\mathrm{N}{\mathrm{NH}}_3$ will contain $\frac{14}{1000}\times ({\mathrm{V}}_1-{\mathrm{V}}_2)\times {\mathrm{N}}_1\mathrm{g}$ ’ $\mathrm{N}$ '

This is mass of ’ $\mathrm{N}$ ’ in $\mathrm{Wg}$ of compound.

$$\begin{array}{rl}& \mathrm{\%}\text{ of }\mathrm{N}=\frac{14}{1000}\times \frac{({\mathrm{V}}_1-{\mathrm{V}}_2)\times {\mathrm{N}}_1\times 100}{\mathrm{W}}\\ & \text{ or }\mathrm{\%}\text{ of }\mathrm{N}=\frac{1.4\times \text{ normality of acid }\times \text{ volume of acid used }}{\text{ mass of compound }}\end{array}$$

3.Estimation of Halogens : (Carius Method)

A known mass of organic compound is heated with fuming ${\mathrm{HNO}}_3$ in presence of ${\mathrm{AgNO}}_3$. The halogen of compound gets precipitated in the form of $\mathrm{Ag}X$ which can be filtered, washed, dried & weighed.

Let the mass of organic compound $=\mathrm{Wg}$

& mass of AgX formed = wg

$\mathrm{Ag}X\equiv \mathrm{Ag}$

$(108+$ atomic mass of halogen $)=($ atomic mass of halogen $)$

Now 1 mole of $\mathrm{AgX}$ contains $1\mathrm{g}$ atom of $\mathrm{X}(\mathrm{X}=\mathrm{Cl},\mathrm{Br},\mathrm{I})$

wg of AgX contains $=\frac{\text{ Atomic mass of }X}{(108+\text{ at. mass of }X)}\times wg$

This is mass of halogen $(X)$ present in $\mathrm{Wg}$ of compound

$$ of halogen $=\frac{(\text{ at. mass of }X)}{(108+\text{ at. mass of }X)}\times \frac{W}{W}\times 100$

4.Estimation of Sulphur: (Carius method)

A known mass of compound is heated with fuming ${\mathrm{HNO}}_3$. Sulphur present in the compound gets converted into ${\mathrm{H}}_2{\mathrm{SO}}_4$ which is then precipitated as ${\mathrm{BaSO}}_4$.

$\mathrm{S}+{\mathrm{H}}_2\mathrm{O}+30($ from ${\mathrm{HNO}}_3)\stackrel{\mathrm{\Delta }}{\to }{\mathrm{H}}_2{\mathrm{SO}}_4$

${\mathrm{H}}_2{\mathrm{SO}}_4+{\mathrm{BaCl}}_2⟶{\mathrm{BaSO}}_4$

${\mathrm{BaSO}}_4\equiv \mathrm{S}$

$233\mathrm{g}32\mathrm{g}$

mass of ’ $S$ ’ in $w\mathrm{g}$ of ${\mathrm{BaSO}}_4=\frac{32}{233}\times \mathrm{wg}$

$\mathrm{\%}$ of ‘S’ in Wg compound

$\mathrm{\%}$ of $S=\frac{32}{233}\times \frac{W}{W}\times 100$

5.Estimation of Phosphorus (Carius method)

When known mass of compound is heated with fuming ${\mathrm{HNO}}_3$, phosphorus of compound is oxidised to ${\mathrm{H}}_3{\mathrm{PO}}_4$ which is precipitated as magnesium ammonium phosphate and then ignited to give magnesium pyrophosphate.

${\mathrm{Mg}}_2{\mathrm{P}}_2{\mathrm{O}}_7\equiv {\mathrm{P}}_2$

$222\mathrm{g}31\times 2\mathrm{g}$

mass of phosphorus in wg of ${\mathrm{Mg}}_2{\mathrm{P}}_2{\mathrm{O}}_7=\frac{62}{222}\times \mathrm{wg}$

$$ of ‘P’ in W g organic compound

$$ of $P=\frac{62}{222}\times \frac{W}{W}\times 100$

6.Estimation of Oxygen

Oxygen, in an organic compound, can be calculated by the difference method.

Percentage of oxygen $=(100-$ Sum of $$ of all other elements $)$

Calculations of Empirical and Molecular Formula

Empirical Formula

It is the simplest whole number ratio between the atoms of various elements present in one molecule of the substance. eg. ratio of $\mathrm{H}$ & $\mathrm{O}$ in ${\mathrm{H}}_2{\mathrm{O}}_2$ is 1 : 1, therefore, Empirical formula is $\mathrm{HO}$.

Molecular Formula

It gives the actual number of atoms of various elements present in one molecule of the compound eg. actual no. of atoms of $\mathrm{H}$ and $\mathrm{O}$ in ${\mathrm{H}}_2{\mathrm{O}}_2$ is 2 & 2, Therefore, molecular formula is ${\mathrm{H}}_2{\mathrm{O}}_2$.

Relationship between Empirical and Molecular formula

Molecular formula $=\mathrm{n}\times$ (Empirical formula)

Where $\mathrm{n}$ is a simple integer $1,2,3$, etc. given by the equation

$$\mathrm{n}=\frac{\text{ Molecular mass }}{\text{ Empirical formula mass }}$$

For example empirical formula of glucose is ${\mathrm{CH}}_2\mathrm{O}$

Its empirical formula mass $=12+(2\times 1)+16=30$

Molecular Mass of glucose $=180$

$\mathrm{n}=\frac{\text{ Molecular mass }}{\text{ Empirical formula mass }}=\frac{180}{30}=6$

Molecular formula of glucose $=\mathrm{n}\times$ (Empirical formula)

$$=6\times \left({\mathrm{CH}}_2\mathrm{O}\right)={\mathrm{C}}_6{\mathrm{H}}_{12}{\mathrm{O}}_6$$

Steps to calculate Empirical formula

1. Devide the percentage of each element by its atomic mass to get the relative number of atoms in the molecule.

2. Devide the result obtained in the above step by the smallest value among them to get the simplest ratio of atoms of various elements present in the molecule.

3. If the ratio obtained in step 2 is not a whole no., then multiply the figures with a suitable integer to make it simplest whole number ratio.

4. Now write the symbols of the various elements present along with the whole numbers obtained in step 3. This gives the empirical or simplest formula.

Steps to determine Molecular formula

1. Determine empirical formula from percentage composition of atoms of various elements present.

2. Calculate empirical formula mass.

3. Calculate molecular mass by suitable method.

4. Determine value of $n;n=\frac{\text{ Molecular mass }}{\text{ Empirical formula mass }}$

5. Multiply empirical formula by ’ $n$ ’ to get the molecular formula

Molecular formula $=\mathrm{nx}$ (Empirical formula)

Empirical and Molecular formulae of some compounds

Solved problems

Question 1. Calculate empirical and molecular formula of an organic compound containing $58.53$ carbon, $4.06$ hydrogen, $11.38\mathrm{\%}$ nitrogen and rest is oxygen. Molecular mass of compound is 123 .

Question 2. Calculate molecular formula of compound (Molecular mass $=88$ ), having $54.2$ carbon, $9.2$ hydrogen and $36.6$ oxygen.

Question 3. $0.246\mathrm{g}$ of an organic compound gave $0.198\mathrm{g}$ of ${\mathrm{CO}}_2$ and $0.1014\mathrm{g}$ of water on complete combustion. $0.37\mathrm{g}$ of compound gave $0.638\mathrm{g}$ of silver bromide. What is the molecular formula of the compound if its vapour density is 54.4 .

Question 4. $0.25\mathrm{g}$ of an organic compound upon Kjeldahl’s analysis evolved ammonia which was absorbed in $25\mathrm{mL}$ of $\mathrm{IN}{\mathrm{H}}_2{\mathrm{SO}}_4$. The residual acid required $30\mathrm{mL}$ of $\frac{\mathrm{N}}{2}\mathrm{NaOH}$ solution. Find the $$ of ’ $\mathrm{N}$ ’ in the compound.

Question 5. $0.5\mathrm{g}$ of an organic compound, when treated by Carius method, gave $0.65\mathrm{g}{\mathrm{BaSO}}_4$. Calculate the $$ of ’ $S$ ’ in the compound.

Question 6. $0.2\mathrm{g}$ of an organic compound containing phosphorus gave $0.32\mathrm{g}$ of ${\mathrm{Mg}}_2{\mathrm{P}}_2{\mathrm{O}}_7$ by the usual analysis. Calculate the % of ’ $P$ ’ in the compound.

Question 7. $0.35\mathrm{g}$ of a substance gave $0.25\mathrm{g}$ of silver bromide in Carius method of halogen estimation. Find the $$ of bromine in the compound.

Question 8. $0.15\mathrm{g}$ of an organic compound gave $25\mathrm{mL}$ of nitrogen, collected at $300\mathrm{K}$ and $715\mathrm{mm}$ pressure in Duma’s method. Calculate the percentage of nitrogen in the compound (Aqueous tension of water at $300\mathrm{K}$ is $15\mathrm{mm}$ ).

Question 9. $0.35\mathrm{g}$ of a substance on combustion gave $0.52\mathrm{g}$ of ${\mathrm{CO}}_2$ and $0.3\mathrm{g}$ of ${\mathrm{H}}_2\mathrm{O}$. Calculate the percentage of ‘C’ & ’ $H$ ’ in it.

Question 10. $0.25\mathrm{g}$ of an organic compound at NTP gave $31\mathrm{mL}$ of ${\mathrm{N}}_2$ gas by Duma’s estimation method. Find out percentage of ’ $N$ '

Question 11. Suggesta suitable method to separate :-

(a) Mixture of $o$-nitrophenol and $p$-nitrophenol

(b) Mixture of benzene (b.p.353K) and toluene (b.p.384K)

(c) Sugar containing impurity of common salt.

(d) Acetone (b.p. 329K) and methanol (b.p.338K)

(e) Mixture of benzoic acid and naphthalene

Question 12. Give method for the purification of

(a) Camphor

(b) Nitrobenzene

(c) Kerosene having water impurity

Practice Problems

Question 1. In the Lassaigne’s test for ’ $N$ ’ the prussian blue colour is obtained due to the formation of

(a) ${\mathrm{Na}}_4[\mathrm{Fe}(\mathrm{CN}{)}_6]$

(b) ${\mathrm{Fe}}_4[\mathrm{Fe}(\mathrm{CN}{)}_6]$

(c) ${\mathrm{Fe}}_2[\mathrm{Fe}(\mathrm{CN}{)}_6]$

(d) ${\mathrm{Fe}}_3[\mathrm{Fe}(\mathrm{CN}{)}_6]$

Question 2. A mixture of liquid $\mathrm{A}$ and $\mathrm{B}$ having boiling points $365\mathrm{K}$ and $356\mathrm{K}$, respectively, can be separated by

(a) Simple distillation

(b) Steam distillation

(c) Fractional distillation

(d) Distillation under reduced pressure

Question 3. The best and latest technique for isolation, purification and separation of organic compounds is

(a) Crystallization

(b) Distillation

(c) Sublimation

(d) Chromatography

Question 4. In which out of the following compounds, ’ $\mathrm{N}$ ’ can not be analysed by Lassaigne’s test

(a) Nitrobenzene

(b) Aniline

(c) Urea

(d) Hydrazine

Question 5. Which of the following compounds is / are formed when an organic compound containing both $N$ & $S$ is fused with sodium

(a) Thiocyanate

(b) Nitrate and sulphide

(c) Cyanide and sulphite

(d) Sulphide & cyanide

Question 6. In Duma’s method, ’ $\mathrm{N}$ ’ is determined in the form of

(a) $\mathrm{NaCN}$

(b) Gaseous nitrogen

(c) Ammonium sulphate

(d) Gaseous ammonia

Question 7. The concentration of $\mathrm{C}=85.45$ is not obeyed by the Empirical formula

(a) ${\mathrm{C}}_2{\mathrm{H}}_4$

(b) ${\mathrm{C}}_4{\mathrm{H}}_8$

(c) ${\mathrm{C}}_2{\mathrm{H}}_6$

(d) ${\mathrm{CH}}_2$

Question 8. An organic compound decomposes below its boiling point, can be purified by

(a) Simple distillation

(b) Sublimation

(c) Distillation under reduced pressure

(d) Fractional distillation

Question 9. Camphor can be purified by

(a) Crystallization

(b) Sublimation

(c) Distillation

(d) Extraction

Question 10. The appearance of blood red colouration during Lassaigne’s test indicates

(a) Presence of $S$

(b) Presence of $\mathrm{N}$

(c) Presence of $\mathrm{N}\mathrm{\&}\mathrm{S}$

(d) Presence of halogen

Question 11. Identify the compound that con not be Kjeldahlised

(a) Urea

(b) Pyridine

(c) Aniline

(d) Benzamide

Question 12. Which of the following fertilizers has the highest percentage of ’ $N$ '

(a) Ammonium sulphate

(b) Urea

(c) Ammonium nitrate

(d) Calcium cyanamide

Question 13. The Lassaigne’s extract is boiled with dil. ${\mathrm{HNO}}_3$ before testing for halogens because

(a) Silver halides are soluble in ${\mathrm{HNO}}_3$

(b) ${\mathrm{Ag}}_2\mathrm{S}$ is soluble in ${\mathrm{HNO}}_3$

(c) $\mathrm{AgCN}$ is soluble in ${\mathrm{HNO}}_3$

(d) ${\mathrm{Na}}_2\mathrm{S}$ and $\mathrm{NaCN}$ are decomposed by ${\mathrm{HNO}}_3$

Question 14. In the Kjeldahl’s method of estimation of ’ $N$ ’ present in a soil sample, ammonia evolved from $0.75\mathrm{g}$ of sample, neutralized $10\mathrm{mL}$ of $1{\mathrm{MH}}_2{\mathrm{SO}}_4$. The % of ’ $\mathrm{N}$ ’ in the soil is

(a) 37.33

(b) 45.33

(c) 35.33

(d) 43.33

Question 15. $31.7\mathrm{mL}$ of moist ${\mathrm{N}}_2$ was obtained from $0.2033\mathrm{g}$ of an organic compound in Duma’s method at ${14}^{\circ }\mathrm{C}$ and $758\mathrm{mm}$ pressure. If aq. tension at ${14}^{\circ }\mathrm{C}=14\mathrm{mm}$, the $$ of ’ $N$ ’ in the sample is

(a) 19.25

(b) 18.15

(c) 19.01

(d) 20.2

Question 16. $0.189\mathrm{g}$ of substance, during Carius determination gave $0.287\mathrm{g}$ of $\mathrm{AgCl}$. What is the $$ of chlorine in the sample

(a) 40.20

(b) 38.36

(c) 37.57

(d) 39.04

Question 17. $0.40\mathrm{g}$ of an organic compound containing phosphorus gave $0.555\mathrm{g}$ of ${\mathrm{Mg}}_2{\mathrm{P}}_2{\mathrm{O}}_7$ in Carius analysis. % of phosphorus in the organic compound is

(a) 38.75

(b) 50.23

(c) 40.57

(d) 37.85

Question 18. A compound has $\mathrm{C},\mathrm{H},\mathrm{N}$ in the following percentage : $\mathrm{C}=40$. What is its Empirical formula

(a) ${\mathrm{CH}}_2\mathrm{N}$

(b) ${\mathrm{C}}_2{\mathrm{H}}_5\mathrm{N}$

(c) ${\mathrm{CH}}_5\mathrm{N}$

(d) ${\mathrm{CH}}_4\mathrm{N}$

Question 19. 1.89g of an organic compound containg $\mathrm{C},\mathrm{H}\mathrm{\&}\mathrm{O}$ gave $2.64\mathrm{g}{\mathrm{CO}}_2$ and $1.08{\mathrm{g}}_2\mathrm{O}$ upon combustion. Its Empirical formula is

(a) ${\mathrm{C}}_2{\mathrm{H}}_2\mathrm{O}$

(b) ${\mathrm{CH}}_2\mathrm{O}$

(c) ${\mathrm{CH}}_3\mathrm{O}$

(d) ${\mathrm{C}}_2{\mathrm{H}}_2{\mathrm{O}}_2$

Question 20. When acetic acid and lead acetate are added to sodium extract during Lassaigne’s test, appearance of black precipitate shows the presence of

(a) Phosphorus

(b) Nitrogen

(c) Sulphur

(d) Halogen

Question 21. An organic substance from its aqueous solution can be separated by

(a) Simple Distillation

(b) Steam distillation

(c) Solvent extraction

(d) Fractional distillation

Question 22. In steam distillation, the vapour pressure of the volatile compound is

(a) Equal to atmospheric pressure

(b) Less than atmospheric pressure

(c) More than atmospheric pressure

(d) Exactly half of the atomospheric pressure

Question 23. Refining of petroleum involves the process of

(a) Simple distillation

(b) Steam distillation

(c) Distillation under reduced pressure

(d) Fractional distillation

Question 24. In Kjeldahl’s method, the nitrogen present in the compound is converted into

(a) Gaseous ammonia

(b) Gaseous nitrogen

(c) Ammonium sulphate

(d) Ammonium nitrate

Question 25. The property which serves as a criterion of purity of an organic compound its

(a) Solubility in water

(b) Conductivity

(c) Density

(d) Crystalline nature

Question 26. Separation of two substances by fractional crystallization depends upon their difference in

(a) Densities

(b) Solubilities

(c) Melting points

(d) Boiling points

Question 27. The separation of the constituents of a mixture by column chromatography depends upon their

(a) Different solubilities

(b) Different boiling points

(c) Different refractive index

(d) Differential adsorption

Question 28. Ammonia evolved from $0.4\mathrm{g}$ of an organic substance by Kjeldahl’s method was absorbed in $30\mathrm{mL}$ of semi-normal (N/2) ${\mathrm{H}}_2{\mathrm{SO}}_4$. The excess of the acid was neutralised by the addition of $30\mathrm{mL}$ of $\frac{\mathrm{N}}{5}\mathrm{NaOH}$. % of ’ $\mathrm{N}$ ’ in the substance is

(a) 30.5

(b) 31.5

(c) 33.5

(d) 32.5

Question 29. An organic compound contains carbon $34.6$ and hydrogen $3.84$, the rest being oxygen. Its vapour density is 52 . The molecular formula of the compound is

(a) ${\mathrm{C}}_3{\mathrm{H}}_5\mathrm{O}$

(b) ${\mathrm{C}}_3{\mathrm{H}}_4{\mathrm{O}}_2$

(c) ${\mathrm{C}}_3{\mathrm{H}}_3\mathrm{O}$

(d) ${\mathrm{C}}_3{\mathrm{H}}_4{\mathrm{O}}_4$

Question 30. If two compounds have same Empirical formula but different Molecular formula, they must have

(a) Different percentage composition

(b) Same viscosity

(c) Different molecular weight

(d) Same vapour density

Question 31. An oxide of nitrogen contains $30.43$ of nitrogen. The molecular weight of the compound is equal to 92 a.m.u. What is the molecular formula of the compound

(a) ${\mathrm{N}}_2{\mathrm{O}}_3$

(b) ${\mathrm{N}}_2{\mathrm{O}}_4$

(c) ${\mathrm{N}}_2{\mathrm{O}}_5$

(d) ${\mathrm{N}}_2\mathrm{O}$

Question 32. An acid contains $\mathrm{C},\mathrm{H}\mathrm{\&}0$. Its $4.24\mathrm{mg}$, upon burning gives $8.45\mathrm{mg}{\mathrm{CO}}_2$ and $3.46\mathrm{mg}{\mathrm{H}}_2\mathrm{O}$. Molecular mass of acid is 88 amu. What is its molecular formula

(a) ${\mathrm{C}}_3{\mathrm{H}}_8{\mathrm{O}}_2$

(b) ${\mathrm{C}}_4{\mathrm{H}}_8{\mathrm{O}}_2$

(c) ${\mathrm{C}}_2{\mathrm{H}}_4{\mathrm{O}}_2$

(d) ${\mathrm{C}}_3{\mathrm{H}}_6{\mathrm{O}}_2$

Question 33. A compound contains $4.07$ and $71.65$ chlorine. Its molecular mass is 98.96 . What is its molecular formula

(a) ${\mathrm{C}}_2{\mathrm{H}}_4{\mathrm{Cl}}_2$

(b) ${\mathrm{CH}}_3\mathrm{Cl}$

(c) ${\mathrm{C}}_2{\mathrm{H}}_6\mathrm{Cl}$

(d) ${\mathrm{C}}_3{\mathrm{H}}_7{\mathrm{Cl}}_2$

Question 34. The most satisfactory method to separate sugars is

(a) Fractional crystallization

(b) Sublimation

(c) Chromatography

(d) Benedict’s reagent

Question 35. $0.36\mathrm{g}$ of the compound during Duma’s estimation gave $48.88{\mathrm{cm}}^3$ of ${\mathrm{N}}_2$ at $300\mathrm{K}$ and $740\mathrm{mm}$ pressure. What is the percentage of nitrogen (Aqueous tension at $300\mathrm{K}$ is $15\mathrm{mm}$ )

(a) 14.73

(b) 15.34

(c) 16.43

(d) 13.37