Unit 07 Equilibrium Part-A
Chemical reactions can be classified as reversible and irreversible reactions. The reactions which occur in one direction i.e. forward direction such that reactants are completely converted into products are called irreversible reactions.
e.g. (i) reaction between equimolar amounts of barium chloride and sodium sulphate resulting in the formation of barium sulphate.
(ii) neutralization reaction
The reactions which can occur in both the directions i.e. forward and backward directions, under similar conditions are called reversible reactions.
e.g. (i) when hydrogen gas is passed over heated iron oxide, iron and water are produced.
On the other hand if steam is passed over powdered iron at the same temperature, oxide of iron and hydrogen are formed.
When the reaction is carried out in a closed vessel, both reactions can take place in the vessel.
Thus, reactions which proceed in both the directions and donot reach to completion stage are called reversible reactions.
The characteristic property of a reversible reaction is that it always attains a state of chemical equilibrium. It is defined as “The state in which the measurable properties of the system such as pressure, density, colour, concentration do not undergo any change with time under given set of conditions”.
At equilibrium, the rate of the forward reaction is exactly equal to the rate of the backward reaction. In this state, the concentrations of reactants and products attain a constant value. This state will continue indefinitely if the conditions such as temperature and concentration are not changed.
The study of chemical equilibrium helps in the elucidation of the optimum conditions for greater yields of the product.
Characteristics of Equilibrium State
(i) It is achieved in a closed system only.
(ii) It is dynamic, can be attained from either side i.e. from the side of reactants or products. (Fig. 1)
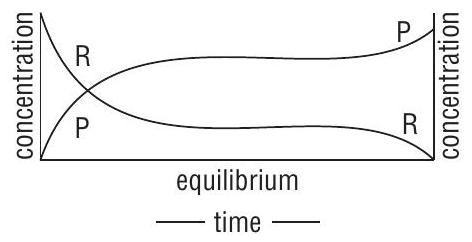
(iii) A catalyst cannot alter the state of equilibrium. It simply helps to attain equilibrium quickly.
Types of chemical equilibria
1. Homogeneous equilibria, i.e., when all the reactants and products are present in the same phase (all gaseous or all liquids). Gaseous reactions are of two types :
2. Heterogeneous equilibria, i.e., when all the reactants and products are not in the same phase, e.g.,
The Law of Chemical equilibrium
Application of Law of Mass Action
Consider a reversible homogeneous reaction at equilibrium state.
Let the active masses of
Active Mass
(i) For solution and gases active mass is the molar concentration.
(ii) For solids and pure liquids, active mass is unity.
Equilibrium constant can also be expressed in terms of partial pressure
For gas phase reactions
Relation between
Equilibrium constant can also be expressed in terms of mole fraction,
Relation between
When concentration are expressed in terms of mole fractions
We know that partial pressure
For the gas phase reaction,
Characteristics of equilibrium constant.
Units of equilibrium constant
Effect of temperature
where
For
Reaction Quotient-Predicting the direction of the Reaction
Reaction Quotient may be defined as :
The ratio of molar concentrations or the partial pressures of the product species to that of the reacting species at any stage in the reaction, each term being raised to the power equal to its stoichiometric coefficient.
If molar concentration of the species are considered, the reaction quotient is called concentration quotient
For a reversible reaction,
Normally reaction quotient is indicated as
It may be noted that if
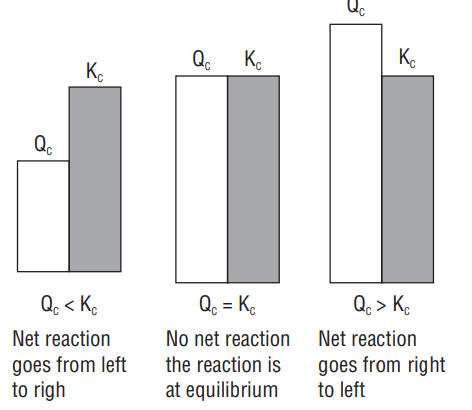
(i) If
(ii) If
(iii) If
Question:1 Consider a reaction
At what pressure,
B. Given that the temperature of the system is
Show Answer
Answer. Since pressure is to be calculated, the given value of
Here
For reaction
Moles
Initial
at equilibrium
Total moles at equilibrium
Let ’
Given
Question:2 Consider the equilibrium
At a certain temperature, the equilibrium concentrations of
Show Answer
Answer. Given,
Let at first equilibrium, the concentration of
When volume is doubled, concentrations are halved, a new equilibrium is established but the value of
Since
| Concentration | A | B | C |
|---|---|---|---|
| Initial | 1 | 1.5 | |
| At new equilibrium |
Given
Comparing equation (i) and (ii)
Activation Energies for forward and Backward reactions
In a reversible reaction, both reactions follow the same path and form the same activated complex but their activation energies are different.

For exothermic reactions,
For endothermic reaction,
Standard Gibbs energy change of a reaction and its equilibrium constant
Let
This equation represents one of the most important results of thermodynamics and relates the equilibrium constant of a reaction to a thermochemical property. It is sometimes easier to calculate the Gibbs energy in a reaction rather than to measure the equilibrium constant.
Standard Gibbs energy change can be thermodynamically calculated as :
Here
(i) When
(ii) When
(iii) When
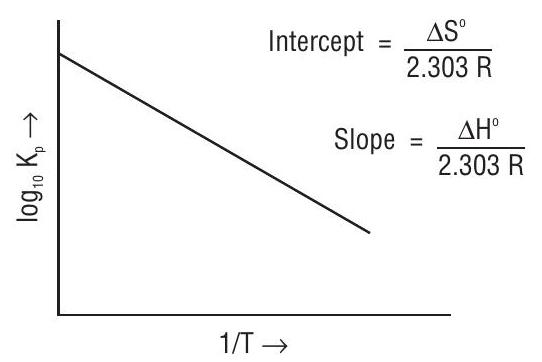
Fig : Variation of Equilibrium constant with inverse of temperature
Question:3 Calculate
When hydrogen gas is introduced in a
Show Answer
Answer. Let the mole of hydrogen gas introduced be ’
The value of ’
The number of moles of various species in the system at equilibrium can be represented as
here
Question:4 At
At what pressure, the equilibrium mixture has the amounts of
Show Answer
Answer.
Given
At pressure ’
Given that
At new pressure
LE CHATELIER’SPRINCIPLE
If a system, at equilibrium, is subjected to a change of concentration, pressure or temperature, the equilibrium shifts in the direction that tends to undo the effect of the change.
This leads to the following important results:
(i) An increase in concentration of reactants always favours forward reaction so as to reduce the amount of reactants.
(ii) An increase in concentration of products always favours backward reaction so as to reduce the amount of products.
(iii) An increase in pressure always favours the reaction where the number of gaseous molecules is reduced.
(iv) A rise in temperature favours the endothermic reaction
Effect of addition of inert gas
| Condition | effect | |
|---|---|---|
| No effect | ||
| No effect | ||
| Forward shift | ||
| backward shift |
Applications of Le Chatelier’s Principle
1. Physical equilibrium
(a) Ice-water system (Melting of ice):
The change can be represented as
Ice + Heat
In this change, heat is absorbed (endothermic) and there is decrease in volume. According to Le Chatelier’s principle, the favourable conditions for the melting of ice are :
(i) High pressure and
(ii) Hightemperature
(b) Water-water vapour system (Vaporisation of water)
The change can be represented as:
Water + Heat
This change is endothermic (heat is absorbed) and there is increase in volume. Thus, favourable conditions for the conversion of water into water vapour are:
(i) High temperature and
(ii) Low pressure
(c) Effect of pressure on solubility of gases
Gas + Solvent
where
(d) Effect of temperature on solubility of solids
Solute + Solvent
An increase in temperature always favours endothermic process and thus, solute having endothermic dissolution (e.g., urea, glucose) show an increase in their solubility with temperature
Solute + Solvent
Accordingly, solute having exothermic dissolution (e.g., lime, acids) show a decrease in their solubility with temperature.
Note: (i) Hydrated salts like
2. For chemical equilibrium
(a) Formation of ammonia
The increase in pressure favours forward reaction,
The increase in temperature favours backward reaction.
The increase in concentration of reactant(s) favours forward reaction.
The increase in concentration of product favours backward reaction. Thus, to get better yield of
(a) High pressure
(b) Low temperature
(c) High concentration of reactants
(d)
(b) Formation of
Effect of concentration
When concentration of
Effect of pressure
As there is no change in the number of moles in the reaction, the equilibrium state remains unaffected by change of pressure.
Effect of temperature
By increasing temperature the equilibrium state shift towards the reaction which moves with absorption of heat. The formation of
In short, we can say that the favourable conditions for greater yield of
(i) High concentrations of
(ii) Lowtemperature
Relation between vapour density and degree of dissociation
In case of reversible reactions, degree of dissociation is determined by measuring density of reaction mixture at equilibrium. Consider a general reaction
Initial no of moles
No. of moles at equilibrium
Total no. of moles
Let the initial volume be
where
and
Question. 5 What is the percentage dissociation of
Show Answer
Answer. Let the degree of dissociation be ’
Percentage dissociation is
Question. 6
Show Answer
Answer. Let ’
moles
initial
at equilibrium
Total gaseous moles in the container
Assuming ideal gas behaviour
PRACTICE QUESTIONS
1. For the reaction:
(a)
(b)
(c)
(d)
Show Answer
Answer: (c)2. For the equilibrium reaction:
the concentration of
(a)
(b)
(c)
(d)
Show Answer
Answer: (b)3. What is the equilibrium expression for the following reaction?
(a)
(b)
(c)
(d)
Show Answer
Answer: (d)4. The equilibrium constant
The value of
(a)
(b)
(c)
(d)
Show Answer
Answer: (a)5. The equilibrium constant
(a)
(b)
(c)
(d)
Show Answer
Answer: (b)6. For which of the following reaction,
(a)
(b)
(c)
(d)
Show Answer
Answer: (d)7. An equilibrium mixture for the reaction
had 1 mole of
(a)
(b)
(c)
(d)
Show Answer
Answer: (c)8. For the reversible reaction
at
(a)
(b)
(c)
(d)
Show Answer
Answer: (d)9. At constant temperature, the equilibrium constant
(a)
(b)
(c)
(d)
Show Answer
Answer: (d)10. A mixture of
(a)
(b)
(c)
(d)
Show Answer
Answer: (b)11. For the reaction:
(a)
(b)
(c)
(d)
Show Answer
Answer: (a)12. Consider the following gaseous equilibrium with equilibrium constants
The equilibrium constants are related as
(a)
(b)
(c)
(d)
Show Answer
Answer: (a)13. Consider the following equilibrium in a closed container:
(a)
(b)
(c)
(d)
Show Answer
Answer: (d)14. Chemical equilibrium is attained in a reversible reaction carried in a close container and is of dynamic nature. The value of equilibrium constant may be expressed either as
Free energy change
1. A reaction attains equilibrium when the free energy change accompanying the reaction is:
(a) Positive and large
(b) Zero
(c) Negative
(d) Negative and small
Show Answer
Answer: (b)2. The equilibrium constant for the reaction:
and for the reaction
(a)
(b)
(c)
(d)
Show Answer
Answer: (b)3. For the reaction
the value of
(a)
(b)
(c)
(d)
Show Answer
Answer: (a)4. When the two reactants
(a) zero
(b) decreases with time
(c) is independent of time
(d) increases with time
Show Answer
Answer: (d)PRACTICE QUESTIONS
1.
(a) Adding
(b) Adding D
(c) Raising the temperature
(d) Increasing the pressure
Show Answer
Answer: (d)2. The value of equilibrium constant for an endothermic reaction
(a) increases with increase of temperature
(b) decreases with increase of temperature
(c) is independent of temperature
(d) sufficient information is not available to answer the question
Show Answer
Answer: (a)3. A reaction reaches a state of chemical equilibrium only when
(a) the reactants and the products are reacting
(b) the concentrations of the reactants and products become equal
(c) the products react together at the same rate at which they are formed
(d) all the reactants and the products are in the same state of matter.
Show Answer
Answer: (c)4. For the gas phase reaction
which one of the statements below is true for
(a)
(b) Kincreases as
(c) Kdecreases as
(d) Kvaries with the addition of
Show Answer
Answer: (c)5. According to Le Chatelier’s principle, adding heat to a solid and liquid in equilibrium will cause the
(a) temperature to increase
(b) temperature to decrease
(c) amount of liquid to decrease
(d) amount of solid to decrease
Show Answer
Answer: (d)6. For the reaction
(a) High pressure, high temperature
(b) Only low temperature
(c) High pressure, low temperature
(d) Only low pressure
Show Answer
Answer: (a)7. For the reaction
(a) Introducing an inert gas at constant volume
(b) Introducing
(c) Introducing
(d) Introducing
Show Answer
Answer: (c)8. On the basis of Le Chatelier’s principle, predict which of the following conditions would be un-favourable for the formation of
(a) Low pressure
(b) High pressure
(c) Low temperature
(d) High concentration of
Show Answer
Answer: (c)9. For the chemical reaction:
the amount of
(a) temperature and pressure
(b) pressure only
(c) temperature only
(d) temperature, pressure and catalyst
Show Answer
Answer: (a)10. 2.2 moles of phosphorus pentachloride were taken in a closed vessel and dissociated into phosphorus trichloride and chlorine. At equilibrium, the total number of moles of the reactant and the products was 2.53. The degree of dissociation is :
(a)
(b)
(c)
(d)
Show Answer
Answer: (c)11. Starting with 1 mole of
then at equilibrium the total number of moles of
(a) 2
(b)
(c)
(d)
Show Answer
Answer: (d)12. Vapour density of
(a)
(b)
(c)
(d)
Show Answer
Answer: (b)CHEMICAL EQUILIBRIUM ASSIGNMENT - I
OBJECTIVE QUESTIONS
1. An aqueous solutions of hydrogen sulphide shows the equilibrium:
If dilute
a. The equilibrium constant will change
b. The concentration of
c. The concentration of un-dissociated hydrogen sulphide will decrease
d. The concentration of
Show Answer
Answer: (d)2. On applying pressure to the equilibrium:
Which phenomenon will happen?
a. More ice will be formed
b. More water will be formed
c. Equilibrium will not be disturbed
d. Water will evaporate
Show Answer
Answer: (b)3. The vapour density of completely dissociated
a. Slightly less than half of that of ammonium chloride
b. Half of that of ammonium chloride
c. Double that of ammonium chloride
d. Determined by the amount of solid ammonium chloride used in the experiment
Show Answer
Answer: (b)4. Which of the following statements is (are) correct regarding the solubility of a non-reacting gas into a fixed amount of water?
a. The dissolution of gas results in the decrease of temperature
b. The solubility is favoured by high pressure and low temperature
c. The volume of gas dissolved at a fixed temperature and pressure remains constant
d. The amount dissolved increases exponentially with the pressure of the gas
Show Answer
Answer: (b and c)5. If the reaction
a. 3
b. 1
c.
d.
Show Answer
Answer: (d)6. Eight mole of a gas
a.
b.
c.
d.
Show Answer
Answer: (d)7. For the reaction,
a.
b. RT
c. RT
d.
Show Answer
Answer: (d)8. A definite amount of solid
a.
b.
c.
d.
Show Answer
Answer: (d)9. For a polyprotic acid say
a.
b.
c.
d.
Show Answer
Answer: (b)10. When a sample of
a. The reaction is exothermic and
b. The reaction is exothermic and
c. The reaction is endothermic and
d. The reaction is endothermic and
Show Answer
Answer: (b)11. For the reaction
a. the mass of
b. the mass of
c. the masses of both
d. temperature of the system
Show Answer
Answer: (d)12. For the reaction
a.
b.
c.
d.
Show Answer
Answer: (c)13. The concentration of a pure solid or liquid phase is not included in the expression of equilibrium constant because
a. solid and liquid concentrations are independent of their quantities
b. solids and liquids react slowly
c. solids and liquids at equilibrium do not interact with gaseous phase
d. the molecules of solids and liquids cannot migrate to the gaseous phase
Show Answer
Answer: (a)14. When a bottle of cold drink is opened, the gas come out with a fizz due to
a. decrease in pressure suddenly which results in a decrease in solubility of
b. decrease in temperature
c. increase in pressure
d. none of the above
Show Answer
Answer: (a)15. Densities of diamond and graphite are 3.5 and
a. favours backward reaction
b. favours forward reaction
c. has no effect
d. increases the reaction rate
Show Answer
Answer: (a)16. Solubility of a gas in a liquid increases on:
a. addition of catalyst
b. increasing the pressure
c. decreasing the pressure
d. increases the reaction rate
Show Answer
Answer: (b)17. For the reaction
a.
b.
c.
d.
Show Answer
Answer: (b)SUBJECTIVE QUESTIONS
1.
Show Answer
Answer:
2. At temperature
Show Answer
Answer:
3. A sample of air consisting of
was established with an equilibrium constant
Show Answer
Answer:4.
Show Answer
Answer:5. At
Show Answer
Answer: 5.02 litre6. One mole of
Show Answer
Answer:7. A vessel at
Show Answer
Answer: 1.88. A two litre vessel contains 0.48 mole of
Show Answer
Answer:
9. The standard Gibbs function at
Show Answer
Answer:10. The dissociation pressure of solid ammonium hydro-sulphide at










