Unit 9 Coordination Compounds (Exercises)
Exercises
9.1 Explain the bonding in coordination compounds in terms of Werner’s postulates.
Show Answer
Answer
Werner’s postulates explain the bonding in coordination compounds as follows:
(i) A metal exhibits two types of valencies namely, primary and secondary valencies. Primary valencies are satisfied by negative ions while secondary valencies are satisfied by both negative and neutral ions.
In modern terminology, the primary valency corresponds to the oxidation number of the metal ion, whereas the secondary valency refers to the coordination number of the metal ion.
(ii) A metal ion has a definite number of secondary valencies around the central atom. Also, these valencies project in a specific direction in the space assigned to the definite geometry of the coordination compound.
(iii) Primary valencies are usually ionizable, while secondary valencies are non-ionizable.
9.2 $\mathrm{FeSO_4}$ solution mixed with $\left(\mathrm{NH_4}\right)_{2} \mathrm{SO_4}$ solution in $1: 1$ molar ratio gives the test of $\mathrm{Fe}^{2+}$ ion but $\mathrm{CuSO_4}$ solution mixed with aqueous ammonia in 1:4 molar ratio does not give the test of $\mathrm{Cu}^{2+}$ ion. Explain why?
Show Answer
Answer
$\mathrm{(NH_4)_2} \mathrm{SO_4}+\mathrm{FeSO_4}+6 \mathrm{H_2} \mathrm{O} \longrightarrow \underset{\text{Mohr’s Salt}}{\mathrm{FeSO_4} \cdot\mathrm{(NH_4)_2} \mathrm{SO_4} \cdot 6 \mathrm{H_2} \mathrm{O}}$
$\mathrm{CuSO_4}+4 \mathrm{NH_3}+5 \mathrm{H_2} \mathrm{O} \longrightarrow \underset{\text{tetraamminocopper(ii)sulphate}}{\left[\mathrm{Cu}\left(\mathrm{NH_3}\right)_{4}\right] \mathrm{SO_4} \cdot 5 \mathrm{H_2} \mathrm{O}}$
Both the compounds i.e., $\mathrm{FeSO_4} \cdot \mathrm{(NH_4)_2} \mathrm{SO_4} \cdot 6 \mathrm{H_2} \mathrm{O}$ and $[\mathrm{Cu}\mathrm{(NH_3)_4}] \mathrm{SO_4} \cdot 5 \mathrm{H_2} \mathrm{O}$ fall under the category of addition compounds with only one major difference i.e., the former is an example of a double salt, while the latter is a coordination compound.
A double salt is an addition compound that is stable in the solid state but that which breaks up into its constituent ions in the dissolved state. These compounds exhibit individual properties of their constituents. For
e.g. $\mathrm{FeSO_4} \cdot\left(\mathrm{NH_4}\right)_{2} \mathrm{SO_4} \cdot 6 \mathrm{H_2} \mathrm{O}$ breaks into $\mathrm{Fe}^{2+}, \mathrm{NH}^{4+}$, and $\mathrm{SO_4}{ }^{2}$ - ions. Hence, it gives a positive test for $\mathrm{Fe}^{2+}$ ions.
A coordination compound is an addition compound which retains its identity in the solid as well as in the dissolved state. However, the individual properties of the constituents are lost. This happens
because $\left[\mathrm{Cu}\left(\mathrm{NH_3}\right)_{4}\right] \mathrm{SO_4} \cdot 5 \mathrm{H_2} \mathrm{O}$ does not show the test for $\mathrm{Cu}^{2+}$. The ions present in the solution
of $[\mathrm{Cu}\mathrm{(NH_3)_4}] \mathrm{SO_4} \cdot 5 \mathrm{H_2} \mathrm{O}$ are $\left[\mathrm{Cu}\mathrm{(NH_3)_4}\right]^{2+}$ and $\mathrm{SO_4}{ }^{2-}$
9.3 Explain with two examples each of the following: coordination entity, ligand, coordination number, coordination polyhedron, homoleptic and heteroleptic.
Show Answer
Answer
(i) Coordination entity:
A coordination entity is an electrically charged radical or species carrying a positive or negative charge. In a coordination entity, the central atom or ion is surrounded by a suitable number of neutral molecules or negative ions ( called ligands). For example:
$$ \begin{aligned} & {\left[\mathrm{Ni}\mathrm{(NH_3)_6}\right]^{2+},\left[\mathrm{Fe}\mathrm{(CN)_6}\right]^{4+}=\text { cationic complex }} \\ & {\left[\mathrm{PtCl_4}\right]^{2-}, \quad\left[\mathrm{Ag}\mathrm{(CN)_2}\right]^{-}=\text {anionic complex }} \\ & {\left[\mathrm{Ni}\mathrm{(CO)_4}\right], \quad\left[\mathrm{Co}\mathrm{(NH_3)_4} \mathrm{Cl_2}\right] {=\text {neutral complex }}} \end{aligned} $$
(ii) Ligands
The neutral molecules or negatively charged ions that surround the metal atom in a coordination entity or a coordinal complex are known as ligands. For example, $\stackrel{\large{\cdot \cdot} }{\mathrm{N}}\mathrm{H_3}, \mathrm{H_2} \stackrel{\large{\cdot \cdot}}{\mathrm{O}}, \mathrm{Cl^-} , \mathrm{OH}$. Ligands are usually polar in nature and possess at least one unshared pair of valence electrons.
(iii) Coordination number:
The total number of ligands (either neutral molecules or negative ions) that get attached to the central metal atom in the coordination sphere is called the coordination number of the central metal atom. It is also referred to as its ligancy.
For example:
(a) In the complex, $\mathrm{K_2}\left[\mathrm{PtCl_6}\right]$, there as six chloride ions attached to $\mathrm{Pt}$ in the coordinate sphere. Therefore, the coordination number of $\mathrm{Pt}$ is 6 .
(b) Similarly, in the complex $\left[\mathrm{Ni}\left(\mathrm{NH_3}\right)_{4}\right] \mathrm{Cl_2}$, the coordination number of the central atom (Ni) is 4.
(vi) Coordination polyhedron:
Coordination polyhedrons about the central atom can be defined as the spatial arrangement of the ligands that are directly attached to the central metal ion in the coordination sphere. For example:
(a)
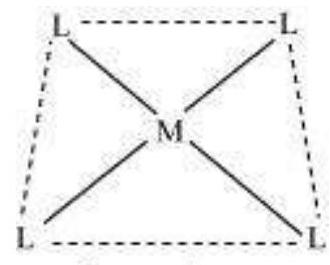
Square planar
(b) Tetrahedral

Tetrahedral
(v) Homoleptic complexes:
These are those complexes in which the metal ion is bound to only one kind of a donor group. For
eg: $\left[\mathrm{Co}\mathrm{(NH_3)_6}\right]^{3+},\left[\mathrm{PtCl_4}\right]^{2-}$ etc.
(vi) Heteroleptic complexes:
Heteroleptic complexes are those complexes where the central metal ion is bound to more than one type of a donor group.
For e.g.: $\left[\mathrm{Co}\mathrm{(NH_3)_4} \mathrm{Cl_2}\right]^{+},\left[\mathrm{Co}\mathrm{(NH_3)_5} \mathrm{Cl}\right]^{2+}$
9.4 What is meant by unidentate, didentate and ambidentate ligands? Give two examples for each.
Show Answer
Answer
A ligand may contain one or more unshared pairs of electrons which are called the donor sites of ligands. Now, depending on the number of these donor sites, ligands can be classified as follows:
(a) Unidentate ligands: Ligands with only one donor sites are called unidentate ligands. For e.g., $\stackrel{\sim}{\mathrm{N}} \mathrm{H_3}, \mathrm{Cl}$ etc.
(b) Didentate ligands: Ligands that have two donor sites are called didentate ligands. For e.g.,
(a) Ethane-1,2-diamine
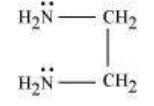
(b) Oxalate ion
$\left(\mathrm{C_2} \mathrm{O_4}^{2-}\right)$ or $\begin{array}{l} \mathrm{COO}^{-} \\ | \\ \mathrm{COO}^{-} \end{array}$
(c) Ambidentate ligands:
Ligands that can attach themselves to the central metal atom through two different atoms are called ambidentate ligands. For example: (a)
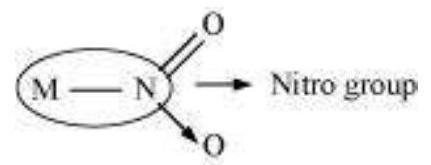
(The donor atom is $\mathrm{N}$ )
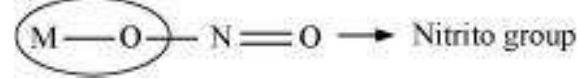
(The donor atom is oxygen)
(b)
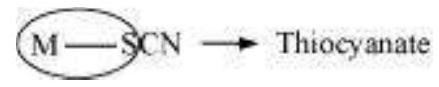
(The donor atom is $\mathrm{S}$ )

(The donor atom is $\mathrm{N}$ )
9.5 Specify the oxidation numbers of the metals in the following coordination entities:
(i) $\left[\mathrm{Co}\left(\mathrm{H_2} \mathrm{O}\right)(\mathrm{CN})(\mathrm{en})_2\right]^{2+}$
(ii) $\left[\mathrm{CoBr_2}(\mathrm{en})_2\right]^{+}$
(iii) $\left[\mathrm{PtCl_4}\right]^{2-}$
(iv) $\mathrm{K_3}\left[\mathrm{Fe}(\mathrm{CN})_6\right]$
(v) $\left[\mathrm{Cr}\left(\mathrm{NH_3}\right)_3 \mathrm{Cl_3}\right]$
Show Answer
Answer
(i) $\left[\mathrm{Co}\left(\mathrm{H_2} \mathrm{O}\right)(\mathrm{CN})(\mathrm{en})_{2}\right]^{2+}$
Let the oxidation number of $\mathrm{Co}$ be $x$.
The charge on the complex is +2 .
$\begin{array}{cccccccr} [\mathrm{Co} & & \left(\mathrm{H_2} \mathrm{O}\right) & &(\mathrm{CN}) && \left.(\mathrm{en})_{2}\right]^{2+} &\\ \downarrow & &\downarrow && \downarrow & &\downarrow & \\ x &+ & 0 &+ & (-1)& + &2(0)& =+2 \\ &&& x & -1 & = & +2 & \\ &&&& x & = & +3& \end{array}$
(ii) $\left[\mathrm{Pt}(\mathrm{Cl})_{4}\right]^{2-}$
Let the oxidation number of Pt be $x$.
The charge on the complex is -2 .
$\begin{array}{cccc} [\mathrm{Pt} & & \left(\mathrm{Cl_4}\right) \left. \right]^{2+} &\\ \downarrow & &\downarrow & & \\ x &+ & 4(-1) &= & -2 \\ x & = & +2 \\ \end{array}$
(iii)
$\begin{array}{cccccc} [\mathrm{Co} & & \mathrm{(Br)_2} & & \left.\mathrm{(en)_2}\right]^{2+} &\\ \downarrow & &\downarrow && \downarrow & \\ x &+ & 2(-1) &+ & 2(0)& = &+1 \\ x&-&2 & = & +1& \\ x & = & +3& \end{array}$
(iv) $\mathrm{K_3}\left[\mathrm{Fe}(\mathrm{CN})_{6}\right]$
i.e.,
$\begin{array}{cccc} [\mathrm{Fe} & & \mathrm{(CN)_6} \left. \right]^{3-} &\\ \downarrow & &\downarrow & & \\ x &+ & 6(-1) &= & -3 \\ x & = & +3 \\ \end{array}$
(v)
$\begin{array}{cccccc} [\mathrm{Cr} & & \mathrm{(NH_3)_2} & & \left.\mathrm{(Cl)_3}\right] &\\ \downarrow & &\downarrow && \downarrow & \\ x &+ & 3(0) &+ & 3(-1)& = &0 \\ x&-&2 & = & +1& \\ x & = & +3& \end{array}$
9.6 Using IUPAC norms write the formulas for the following:
(i) Tetrahydroxidozincate(II)
(ii) Potassium tetrachloridopalladate(II)
(iii) Diamminedichloridoplatinum(II)
(iv) Potassium tetracyanidonickelate(II)
(v) Pentaamminenitrito-O-cobalt(III)
(vi) Hexaamminecobalt(III) sulphate
(vii) Potassium tri(oxalato)chromate(III)
(viii) Hexaammineplatinum(IV)
(ix) Tetrabromidocuprate(II)
(x) Pentaamminenitrito-N-cobalt(III)
Show Answer
Answer
(i) $\left[\mathrm{Zn}(\mathrm{OH})_{4}\right]^{2}$
(ii) $\mathrm{K_2}\left[\mathrm{PdCl_4}\right]$
(iii) $\left[\mathrm{Pt}\left(\mathrm{NH_3}\right)_{2} \mathrm{Cl_2}\right]$
(iv) $\mathrm{K_2}\left[\mathrm{Ni}(\mathrm{CN})_{4}\right]$
(v) $\left[\mathrm{Co}(\mathrm{ONO})\left(\mathrm{NH_3}\right)_{5}\right]^{2+}$
(vi) $\left[\mathrm{Co}\mathrm{(NH_3t)_6}\right]_2\mathrm{(SO_4)_3}$
(vii) $\mathrm{K_3}\left[\mathrm{Cr}\left(\mathrm{C_2} \mathrm{O_4}\right)_{3}\right]$
(viii) $\left[\mathrm{Pt}\left(\mathrm{NH_3}\right)_{6}\right]^{4+}$
(ix) $\left[\mathrm{Cu}(\mathrm{Br})_{4}\right]^{2}$
(x) $\left[\mathrm{Co}\left[\mathrm{NO_2}\right)\left(\mathrm{NH_3}\right)_{5}\right]^{2+}$
9.7 Using IUPAC norms write the systematic names of the following:
(i) $\left[\mathrm{Co}\left(\mathrm{NH_3}\right)_{6}\right] \mathrm{Cl_3}$
(ii) $\left[\mathrm{Pt}\left(\mathrm{NH_3}\right)_{2} \mathrm{Cl}\left(\mathrm{NH_2} \mathrm{CH_3}\right)\right] \mathrm{Cl}$
(iii) $\left[\mathrm{Ti}\left(\mathrm{H_2} \mathrm{O}\right)_{6}\right]^{3+}$
(iv) $\left[\mathrm{Co}\left(\mathrm{NH_3}\right)_{4} \mathrm{Cl}\left(\mathrm{NO_2}\right)\right] \mathrm{Cl}$
(v) $\left[\mathrm{Mn}\left(\mathrm{H_2} \mathrm{O}\right)_{6}\right]^{2+}$
(vi) $\left[\mathrm{NiCl_4}\right]^{2-}$
(vii) $\left[\mathrm{Ni}\left(\mathrm{NH_3}\right)_{6}\right] \mathrm{Cl_2}$
(viii) $\left[\mathrm{Co}(\mathrm{en})_{3}\right]^{3+}$
(ix) $\left[\mathrm{Ni}(\mathrm{CO})_{4}\right]$
Show Answer
Answer
(i) Hexaamminecobalt(III) chloride
(ii) Diamminechlorido(methylamine) platinum(II) chloride
(iii) Hexaquatitanium(III) ion
(iv) Tetraamminichloridonitrito-N-Cobalt(III) chloride
(v) Hexaquamanganese(II) ion
(vi) Tetrachloridonickelate(II) ion
(vii) Hexaamminenickel(II) chloride
(viii) Tris(ethane-1, 2-diammine) cobalt(III) ion
(ix) Tetracarbonylnickel(0)
9.8 List various types of isomerism possible for coordination compounds, giving an example of each.
Show Answer
Answer
(a) Geometric isomerism:
This type of isomerism is common in heteroleptic complexes. It arises due to the different possible geometric arrangements of the ligands. For example:
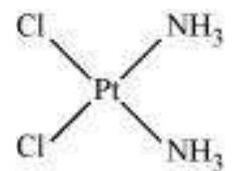
Cis-isomer
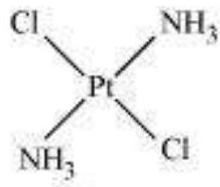
Trans-isomer
(b) Optical isomerism:
This type of isomerism arises in chiral molecules. Isomers are mirror images of each other and are nonsuperimposable.

$\left[\mathrm{Co}(\mathrm{en})_{3}\right]^{3+}$ (dextro)
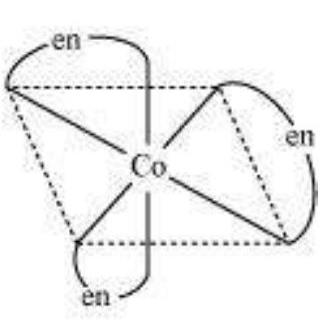
$\left[\mathrm{Co}(\mathrm{en})_{3}\right]^{3+}$
(Laevo)
(c) Linkage isomerism: This type of isomerism is found in complexes that contain ambidentate ligands. For example:
$\left[\mathrm{Co}\mathrm{(NH_3)_5}\mathrm{(NO_2)}\right] \mathrm{Cl_2}$ and $\left[\mathrm{Co}\mathrm{(NH_3)_5}(\mathrm{ONO}) \mathrm{Cl_2}\right]$
Yellow form Red form
(d) Coordination isomerism:
This type of isomerism arises when the ligands are interchanged between cationic and anionic entities of differnet metal ions present in the complex.
$\left[\mathrm{Co}\mathrm{(NH_3)_6}\right]\left[\mathrm{Cr}\mathrm{(CN)_6}\right]$ and $\left[\mathrm{Cr}\mathrm{(NH_3)_6}\right]\left[\mathrm{Co}\mathrm{(CN)_6}\right]$
(e) lonization isomerism:
This type of isomerism arises when a counter ion replaces a ligand within the coordination sphere. Thus, complexes that have the same composition, but furnish different ions when dissolved in water are called ionization isomers. For e.g., $\left(\mathrm{Co}\mathrm{(NH_3)_5} \mathrm{SO_4}\right) \mathrm{Br}$ and $[\mathrm{Co}\mathrm{(NH_3)_5} \mathrm{Br} ]\mathrm{SO_4}$
(f) Solvate isomerism:
Solvate isomers differ by whether or not the solvent molecule is directly bonded to the metal ion or merely present as a free solvent molecule in the crystal lattice.
$\underset{\text{Violet}}{\left[\mathrm{Cr}\mathrm{(H_2O)_6}\right] \mathrm{Cl_3}\cdot \mathrm{H_2O}}$
$\underset{\text{Blue-green}}{\left[\mathrm{Cr}\mathrm{(H_2O)_5}\right] \mathrm{Cl_2}\cdot \mathrm{H_2O}}$
$\underset{\text{Dark green}}{\left[\mathrm{Cr}\mathrm{(H_2O)_6}\right] \mathrm{Cl_3}\cdot \mathrm{H_2O}}$
9.9 How many geometrical isomers are possible in the following coordination entities?
(i) $\left[\mathrm{Cr}\left(\mathrm{C_2} \mathrm{O_4}\right)_3\right]^{3-}$
(ii) $\left[\mathrm{Co}\left(\mathrm{NH_3}\right)_3 \mathrm{Cl_3}\right]$
Show Answer
Answer
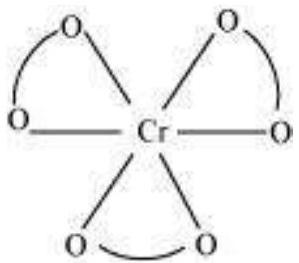
(i) For $\left[\mathrm{Cr}\left(\mathrm{C_2} \mathrm{O_4}\right)_{3}\right]^{3-}$, no geometric isomer is possible as it is a bidentate ligand.
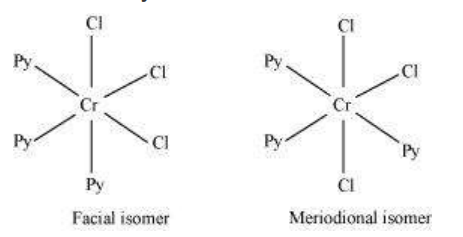
(ii) $\left[\mathrm{Co}\left(\mathrm{NH_3}\right)_{3} \mathrm{Cl_3}\right]$
Two geometrical isomers are possible.
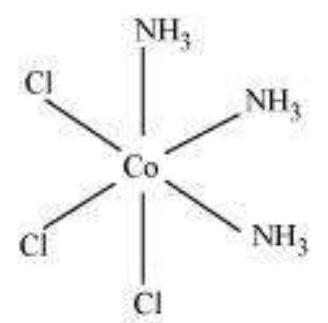
Facial
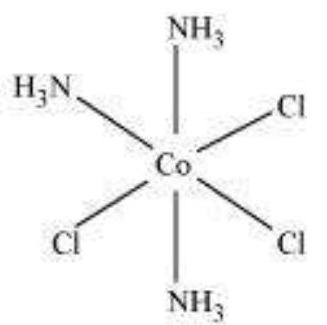
Meridional
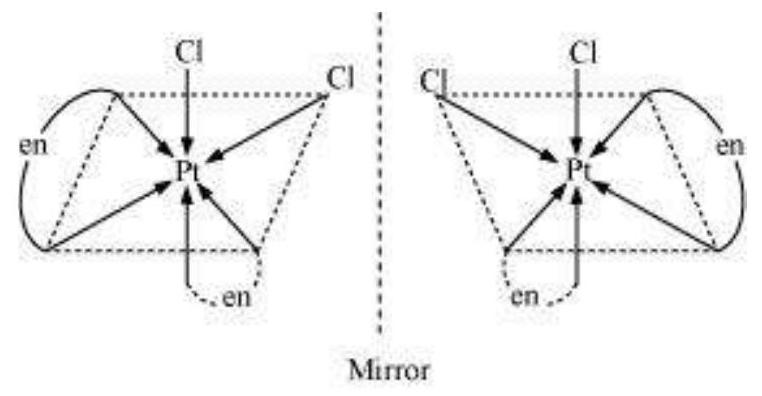
9.10 Draw the structures of optical isomers of:
(i) $\left[\mathrm{Cr}\left(\mathrm{C_2} \mathrm{O_4}\right)_3\right]^{3-}$
(ii) $\left[\mathrm{PtCl_2}(\mathrm{en})_2\right]^{2+}$
(iii) $\left[\mathrm{Cr}\left(\mathrm{NH_3}\right)_2 \mathrm{Cl_2}(\mathrm{en})\right]^{+}$
Show Answer
Answer
(i) $\left[\mathrm{Cr}\left(\mathrm{C_2} \mathrm{O_4}\right)_{3}\right]^{3-}$
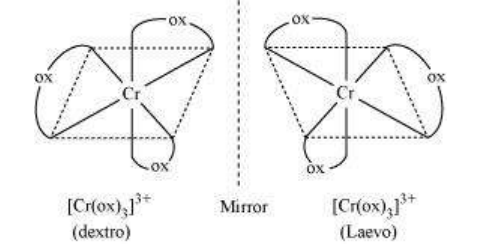
(ii) $\left[\mathrm{PtCl_2}(\mathrm{en})_{2}\right]^{2+}$
(iii) $\left[\mathrm{Cr}\left(\mathrm{NH_3}\right)_{2} \mathrm{Cl_2}(\mathrm{en})\right]^{+}$
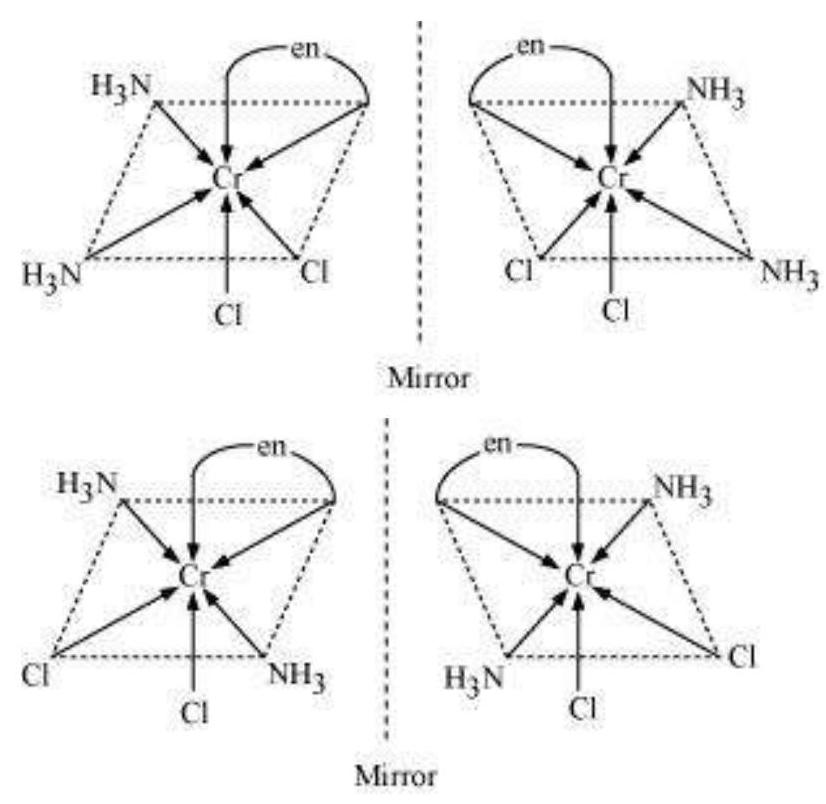
9.11 Draw all the isomers (geometrical and optical) of:
(i) $\left[\mathrm{CoCl_2}(\mathrm{en})_2\right]^{+}$
(ii) $\left[\mathrm{Co}\left(\mathrm{NH_3}\right) \mathrm{Cl}(\mathrm{en})_2\right]^{2+}$
(iii) $\left[\mathrm{Co}\left(\mathrm{NH_3}\right)_2 \mathrm{Cl_2}(\mathrm{en})\right]^{+}$
Show Answer
Answer
(i) $\left[\mathrm{CoCl_2}(\mathrm{en})_{2}\right]^{+}$
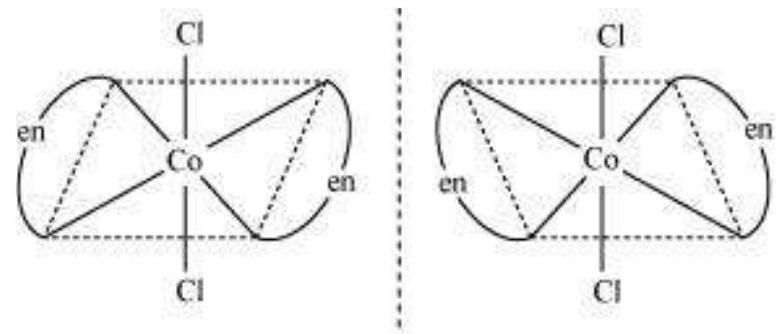
Trans $\left[\mathrm{CoCl_2}(\mathrm{en})_{2}\right]^{+}$isomer-optically inactive
(Superimposable mirror images)

Cis $\left[\mathrm{CoCl_2}(\mathrm{en})_{2}\right]^{+}$isomer-optically active
(Non-superimposable mirror images)
In total, three isomers are possible.
(ii) $\left[\mathrm{Co}\left(\mathrm{NH_3}\right) \mathrm{Cl}(\text { en })_{2}\right]^{2+}$
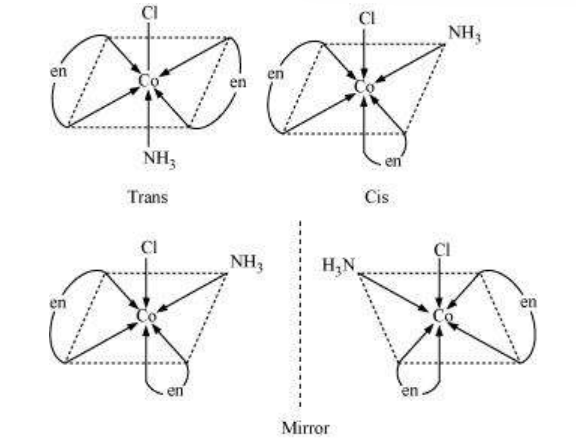
Trans-isomers are optically inactive.
Cis-isomers are optically active.
(iii) $\left[\mathrm{Co}\left(\mathrm{NH_3}\right)_{2} \mathrm{Cl_2}(\mathrm{en})\right]^{+}$

9.12 Write all the geometrical isomers of $\left[\mathrm{Pt}\left(\mathrm{NH_3}\right)(\mathrm{Br})(\mathrm{Cl})(\mathrm{py})\right]$ and how many of these will exhibit optical isomers?
Show Answer
Answer
$\left[\mathrm{Pt}\left(\mathrm{NH_3}\right)(\mathrm{Br})(\mathrm{Cl})(\mathrm{py})\right.$

From the above isomers, none will exhibit optical isomers. Tetrahedral complexes rarely show optical isomerization. They do so only in the presence of unsymmetrical chelating agents.
9.13 Aqueous copper sulphate solution (blue in colour) gives:
(i) a green precipitate with aqueous potassium fluoride and
(ii) a bright green solution with aqueous potassium chloride. Explain these experimental results.
Show Answer
Answer
Aqueous $\mathrm{CuSO_4}$ exists as $\left[\mathrm{Cu}\mathrm{(H_2O)_4}\right] \mathrm{SO_4}$. It is blue in colour due to the presence of $\left[\mathrm{Cu}\mathrm{(H_2O)_4}\right]^{2+}$ ions.
(i) When KF is added:
$$ \left[\mathrm{Cu}\left(\mathrm{H_2} \mathrm{O}\right)_{4}\right]^{2+}+4 \mathrm{~F}^{-} \longrightarrow \underset{\text { (green) }}{[\mathrm{Cu(F)_4 } ]^{2-}} + 4 \mathrm {H_2O} $$
(ii) When $\mathrm{KCl}$ is added:
$$ \left[\mathrm{Cu}\left(\mathrm{H_2} \mathrm{O}\right)_{4}\right]^{2+}+4 \mathrm{Cl}^{-} \longrightarrow \underset{\text{(bright green)}}{\left[\mathrm{CuCl_4}\right]^{2-}}+4 \mathrm{H_2} \mathrm{O} $$
In both these cases, the weak field ligand water is replaced by the $\mathrm{F} \cdot$ and $\mathrm{Cl}$ - ions.
9.14 What is the coordination entity formed when excess of aqueous $\mathrm{KCN}$ is added to an aqueous solution of copper sulphate? Why is it that no precipitate of copper sulphide is obtained when $\mathrm{H_2} \mathrm{~S}(\mathrm{~g})$ is passed through this solution?
Show Answer
Answer
$\mathrm{CuSO_{4(a q)}}+4 \mathrm{KCN_{(a q)}} \longrightarrow \mathrm{K_2[Cu(CN)_4]} {(aq)} + \mathrm{K_2SO_4 {(aq)}} $
i.e., $\left[\mathrm{Cu}\mathrm{(H_O)_4}\right]^{2+}+4 \mathrm{CN}^{-} \longrightarrow\left[\mathrm{Cu}\mathrm{(CN)_4}\right]^{2-}+4 \mathrm{H_2} \mathrm{O}$
Thus, the coordination entity formed in the process is $\mathrm{K_2[Cu(CN)_4]} . \mathrm{K_2}[\mathrm{Cu}\mathrm{(CN)_4}]$ is a very stable complex, which does not ionize to give $\mathrm{Cu^{2+}}$ ions when added to water. Hence, $\mathrm{Cu}^{2+}$ ions are not precipitated when $\mathrm{H_2S {(g)}}$ is passed through the solution.
9.15 Discuss the nature of bonding in the following coordination entities on the basis of valence bond theory:
(i) $\left[\mathrm{Fe}(\mathrm{CN})_6\right]^{4-}$
(ii) $\left[\mathrm{FeF_6}\right]^{3-}$
(iii) $\left[\mathrm{Co}\left(\mathrm{C_2} \mathrm{O_4}\right)_{3}\right]^{3-}$
(iv) $\left[\mathrm{CoF_6}\right]^{3-}$
Show Answer
Answer
(i) $\left[\mathrm{Fe}(\mathrm{CN})_{6}\right]^{4}$
In the above coordination complex, iron exists in the +II oxidation state.
$\mathrm{Fe}^{2+}$ : Electronic configuration is $3 d^{6}$
Orbitals of $\mathrm{Fe}^{2+}$ ion:

As $\mathrm{CN}^{-}$is a strong field ligand, it causes the pairing of the unpaired $3 d$ electrons.

Since there are six ligands around the central metal ion, the most feasible hybridization is $d^{2} s p^{3}$.
$d^{2} s p^{3}$ hybridized orbitals of $\mathrm{Fe}^{2+}$ are:
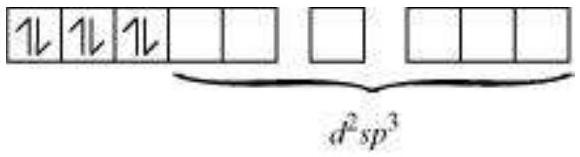
6 electron pairs from $\mathrm{CN}^{-}$ions occupy the six hybrid $d^{2} s p^{3}$ orbitals.
Then,
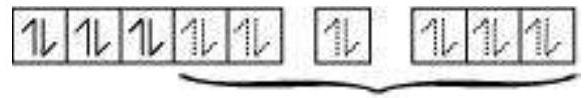
6 pairs of electrons
from $6 \mathrm{CN}^{-}$ions
Hence, the geometry of the complex is octahedral and the complex is diamagnetic (as there are no unpaired electrons).
(ii) $\left[\mathrm{FeF_6}\right]^{3}$
In this complex, the oxidation state of $\mathrm{Fe}$ is +3 .
Orbitals of $\mathrm{Fe}^{+3}$ ion:
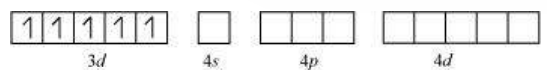
There are $6 \mathrm{~F}^{-}$ions. Thus, it will undergo $d^{2} s p^{3}$ or $s p^{3} d^{2}$ hybridization. As $\mathrm{F}^{-}$is a weak field ligand, it does not cause the pairing of the electrons in the $3 d$ orbital. Hence, the most feasible hybridization is $s p^{3} d^{2}$.
$s p^{3} d^{2}$ hybridized orbitals of $\mathrm{Fe}$ are:
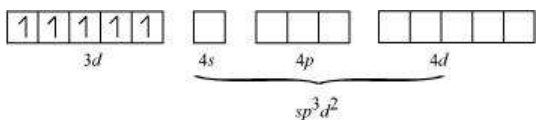
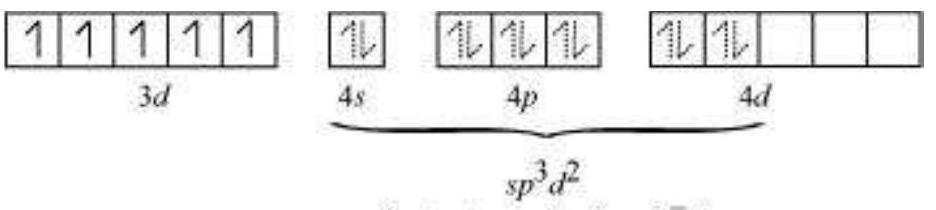
6 electron pairs from $\mathrm{F}^{-}$ions
Hence, the geometry of the complex is found to be octahedral.
(iii) $\left[\mathrm{Co}\left(\mathrm{C_2} \mathrm{O_4}\right)_{3}\right]^{3-}$
Cobalt exists in the +3 oxidation state in the given complex.
Orbitals of $\mathrm{Co}^{3+}$ ion:
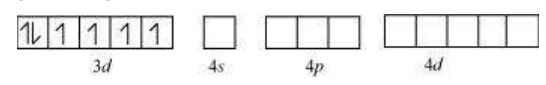
Oxalate is a weak field ligand. Therefore, it cannot cause the pairing of the $3 d$ orbital electrons. As there are 6 ligands, hybridization has to be either $s p^{3} d^{2}$ or $d^{2} s p^{3}$ hybridization.
$s p^{3} d^{2}$ hybridization of $\mathrm{Co}^{3+}$

The 6 electron pairs from the 3 oxalate ions (oxalate anion is a bidentate ligand) occupy these $s p^{3} d^{2}$ orbitals.

Hence, the geometry of the complex is found to be octahedral.
(iv) $\left[\mathrm{CoF_6}\right]^{3-}$
Cobalt exists in the +3 oxidation state.
Orbitals of $\mathrm{Co}^{3+}$ ion:

Again, fluoride ion is a weak field ligand. It cannot cause the pairing of the $3 d$ electrons. As a result, the $\mathrm{Co}^{3+}$ ion will undergo $s p^{3} d^{2}$ hybridization.
$s p^{3} d^{2}$ hybridized orbitals of $\mathrm{Co}^{3+}$ ion are:
9.16 Draw figure to show the splitting of $d$ orbitals in an octahedral crystal field.
Show Answer
Answer
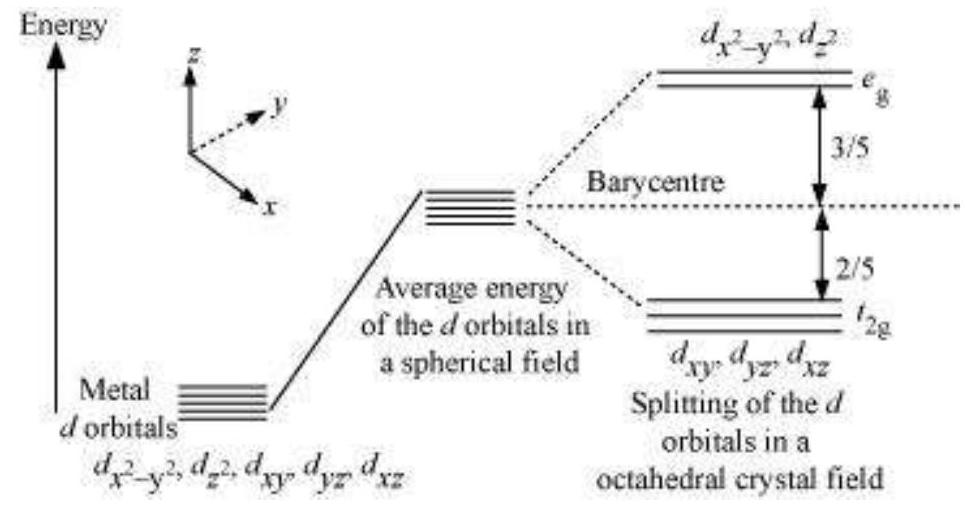
The splitting of the $d$ orbitals in an octahedral field takes palce in such a way that $d_{x^{2}-y^{2}}, d_{z^{2}}$ experience a rise in energy and form the $e_{g}$ level, while $d_{x y}, d_{y z}$ and $d_{z x}$ experience a fall in energy and form the $t_{2 g}$ level.
9.17 What is spectrochemical series? Explain the difference between a weak field ligand and a strong field ligand.
Show Answer
Answer
A spectrochemical series is the arrangement of common ligands in the increasing order of their crystal-field splitting energy (CFSE) values. The ligands present on the R.H.S of the series are strong field ligands while that on the L.H.S are weak field ligands. Also, strong field ligands cause higher splitting in the $d$ orbitals than weak field ligands.
$ \mathrm{I}^{-} < \mathrm{Br}^{-} < \mathrm{S}^{2-}<\mathrm{SCN}^{-}<\mathrm{Cl}^{-}<\mathrm{N_3}<\mathrm{F}^{-}<\mathrm{OH}^{-}<\mathrm{C_2} \mathrm{O_4}{ }^{2-}< \mathrm{H_2} \mathrm{O}<\mathrm{NCS}^{-} < \mathrm{H}^{-}<\mathrm{CN}^{-}<\mathrm{NH_3}<$ en $< \mathrm{SO_3}{ }^{2-}<\mathrm{NO_2}<$ phen $<\mathrm{CO}$
9.18 What is crystal field splitting energy? How does the magnitude of $\Delta_{\mathrm{o}}$ decide the actual configuration of $d$ orbitals in a coordination entity?
Show Answer
Answer
The degenerate $d$-orbitals (in a spherical field environment) split into two levels i.e., $e_{g}$ and $t_{2 g}$ in the presence of ligands. The splitting of the degenerate levels due to the presence of ligands is called the crystal-field splitting while the energy difference between the two levels $\left(e_{g}\right.$ and $t_{2 \mathrm{~g}}$ ) is called the crystal-field splitting energy. It is denoted by $\Delta_{0}$.
After the orbitals have split, the filling of the electrons takes place. After 1 electron (each) has been filled in the three $t_{2 g}$ orbitals, the filling of the fourth electron takes place in two ways. It can enter the $e_{g}$ orbital (giving rise to $t_{2 g}{ }^{3} e_{\mathrm{g}}{ }^{1}$ like electronic configuration) or the pairing of the electrons can take place in the $t_{2 \mathrm{~g}}$ orbitals (giving rise to $t_{2 g}{ }^{4} e_{g}{ }^{0}$ like electronic configuration). If the $\Delta_{0}$ value of a ligand is less than the pairing energy (P), then the electrons enter the $e_{g}$ orbital. On the other hand, if the $\Delta_{0}$ value of a ligand is more than the pairing energy $(P)$, then the electrons enter the $t_{2 g}$ orbital.
9.19 $\left[\mathrm{Cr}\left(\mathrm{NH_3}\right)_6\right]^{3+}$ is paramagnetic while $\left[\mathrm{Ni}(\mathrm{CN})_4\right]^{2-}$ is diamagnetic. Explain why?
Show Answer
Answer
$\mathrm{Cr}$ is in the +3 oxidation state i.e., $d^{\beta}$ configuration. Also, $\mathrm{NH_3}$ is a weak field ligand that does not cause the pairing of the electrons in the $3 d$ orbital.
$\mathrm{Cr}^{3+}$

Therefore, it undergoes $d^{2} s p^{3}$ hybridization and the electrons in the $3 d$ orbitals remain unpaired. Hence, it is paramagnetic in nature.
In $\left[\mathrm{Ni}(\mathrm{CN})_{4}\right]^{2}$, Ni exists in the +2 oxidation state i.e., $d^{8}$ configuration.
$\mathrm{Ni}^{2+}$

$\mathrm{CN}^{-}$is a strong field ligand. It causes the pairing of the $3 d$ orbital electrons. Then, $\mathrm{Ni}^{2+}$ undergoes $d s p^{2}$ hybridization.
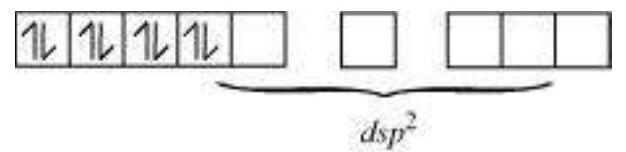
As there are no unpaired electrons, it is diamagnetic.
9.20 A solution of $\left[\mathrm{Ni}\left(\mathrm{H_2} \mathrm{O}\right)_6\right]^{2+}$ is green but a solution of $\left[\mathrm{Ni}(\mathrm{CN})_4\right]^{2-}$ is colourless. Explain.
Show Answer
Answer
In $[\mathrm{Ni(H_2O)_6}]^{2+}$ the delectrons from the lower energy level can be excited to the higher energy level i.e., the possibility of $d$ $d$ transition is present. Hence, $\mathrm{Ni(H_2O)_6}]^{2+}$ is coloured.
In $\left[\mathrm{Ni}(\mathrm{CN}) _{4}\right]^{2 \cdot}$, the electrons are all paired as $\mathrm{CN}^{\prime}$ is a strong field ligand. Therefore, $d-d$ transition is not possible in $\left[\mathrm{Ni}(\mathrm{CN}) _{4}\right]^{2}$. Hence, it is colourless.
9.21 $\left[\mathrm{Fe}(\mathrm{CN})_6\right]^{4-}$ and $\left[\mathrm{Fe}\left(\mathrm{H_2} \mathrm{O}\right)_6\right]^{2+}$ are of different colours in dilute solutions. Why?
Show Answer
Answer
The colour of a particular coordination compound depends on the magnitude of the crystal-field splitting energy, $\Delta$. This CFSE in turn depends on the nature of the ligand. In case of $\left[\mathrm{Fe}(\mathrm{CN}) _{6}\right]^{4}$ and $\left[\mathrm{Fe}\left(\mathrm{H_2} \mathrm{O}\right) _{6}\right]^{2+}$, the colour differs because there is a difference in the CFSE. Now, CN is a strong field ligand having a higher CFSE value as compared to the CFSE value of water. This means that the absorption of energy for the intra $d-d$ transition also differs. Hence, the transmitted colour also differs.
9.22 Discuss the nature of bonding in metal carbonyls.
Show Answer
Answer
The metal-carbon bonds in metal carbonyls have both $\tilde{A} E^{\prime}$ and $\left.\tilde{A} a ̂,\right\urcorner$ characters. A $\tilde{A} E^{\prime}$ bond is formed when the carbonyl carbon donates a lone pair of electrons to the vacant orbital of the metal. A Ãâ, $\neg$ bond is formed by the donation of a pair of electrons from the filled metal $d$ orbital into the vacant anti-bonding Ãâ, $\urcorner^{*}$ orbital (also known as back bonding of the carbonyl group). The $\tilde{A} \vDash{ }^{\prime}$ ’ bond strengthens the Ãâ, $\urcorner$ bond and vice-versa. Thus, a synergic effect is created due to this metal-ligand bonding. This synergic effect strengthens the bond between $\mathrm{CO}$ and the metal.
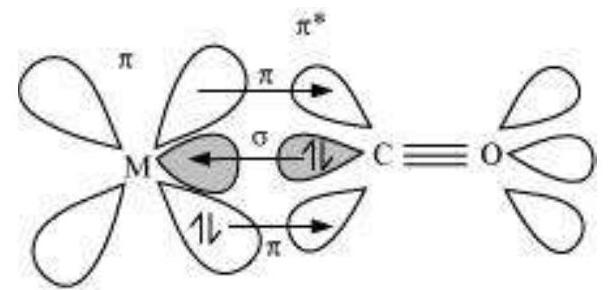
Synergic bonding in metal carbonyls
9.23 Give the oxidation state, $d$ orbital occupation and coordination number of the central metal ion in the following complexes:
(i) $\mathrm{K_3}\left[\mathrm{Co}\left(\mathrm{C_2} \mathrm{O_4}\right)_3\right]$
(ii) cis- $\left[\mathrm{CrCl_2}(\mathrm{en})_{2}\right] \mathrm{Cl}$
(iii) $\left(\mathrm{NH_4}\right)_{2}\left[\mathrm{CoF_4}\right]$
(iv) $\left[\mathrm{Mn}\left(\mathrm{H_2} \mathrm{O}\right)_{6}\right] \mathrm{SO_4}$
Show Answer
Answer
(i) $\mathrm{K_3}\left[\mathrm{Co}\left(\mathrm{C_2} \mathrm{O_4}\right)_{3}\right]$
The central metal ion is Co.
Its coordination number is 6 .
The oxidation state can be given as:
$x-6=-3$ $x=+3$
The $d$ orbital occupation for $\mathrm{Co}^{3+}$ is $t_{2 g}{ }^{6} e_{g}{ }^{0}$.
(ii) cis- $\left[\mathrm{Cr}(\mathrm{en})_{2} \mathrm{Cl_2}\right] \mathrm{Cl}$
The central metal ion is $\mathrm{Cr}$.
The coordination number is 6 .
The oxidation state can be given as:
$x+2(0)+2(-1)=+1$
$x-2=+1$
$x=+3$
The $d$ orbital occupation for $\mathrm{Cr}^{3+}$ is $t_{2 g}{ }^{3}$.
(iii) $\left(\mathrm{NH_4}\right)_{2}\left[\mathrm{CoF_4}\right]$
The central metal ion is Co.
The coordination number is 4 .
The oxidation state can be given as:
$x-4=-2$
$x=+2$
The $d$ orbital occupation for $\mathrm{Co}^{2+}$ is $\mathrm{e_g}{ }^{4} t_{2 g}{ }^{3}$.
(iv) $\left[\mathrm{Mn}\left(\mathrm{H_2} \mathrm{O}\right)_{6}\right] \mathrm{SO_4}$
The central metal ion is Mn.
The coordination number is 6 .
The oxidation state can be given as:
$x+0=+2$
$x=+2$
The $d$ orbital occupation for $\mathrm{Mn}$ is $t_{29}{ }^{3} e_{g}{ }^{2}$.
9.24 Write down the IUPAC name for each of the following complexes and indicate the oxidation state, electronic configuration and coordination number. Also give stereochemistry and magnetic moment of the complex:
(i) $\mathrm{K}\left[\mathrm{Cr}\left(\mathrm{H_2} \mathrm{O}\right)_{2}\left(\mathrm{C_2} \mathrm{O_4}\right)_2\right] \cdot 3 \mathrm{H_2} \mathrm{O}$
(ii) $\left[\mathrm{Co}\left(\mathrm{NH_3}\right)_{5} \mathrm{Cl}\right] \mathrm{Cl_2}$
(iii) $\left[\mathrm{CrCl_3}(\mathrm{py})_{3}\right]$
(iv) $\mathrm{Cs}\left[\mathrm{FeCl_4}\right]$
(v) $\mathrm{K_4}\left[\mathrm{Mn}(\mathrm{CN})_{6}\right]$
Show Answer
Answer
(i) Potassium diaquadioxalatochromate (III) trihydrate.
Oxidation state of chromium $=3$
Electronic configuration: $3 d^{\beta}: t_{2 g}{ }^{3}$
Coordination number $=6$
Shape: octahedral
Stereochemistry:

Magnetic moment, $= \sqrt{n(n+2)}$
$=\sqrt{3(3+2)}$
$=\sqrt{15}$
(ii) $\left[\mathrm{Co}\left(\mathrm{NH_3}\right)_{5} \mathrm{Cl}\right] \mathrm{Cl_2}$
IUPAC name: Pentaamminechloridocobalt(III) chloride
Oxidation state of $\mathrm{Co}=+3$
Coordination number $=6$
Shape: octahedral.
Electronic configuration: $d^{6}: t_{29}{ }^{6}$.
Stereochemistry:

Magnetic Moment $=0$
(iii) $\mathrm{CrCl_3}(\mathrm{py})_{3}$
IUPAC name: Trichloridotripyridinechromium (III)
Oxidation state of chromium $=+3$
Electronic configuration for $d^{3}=t_{29}{ }^{3}$
Coordination number $=6$
Shape: octahedral.
Stereochemistry:
Both isomers are optically active. Therefore, a total of 4 isomers exist.
Magnetic moment, $=\sqrt{n(n+2)}$
$=\sqrt{3(3+2)}$
$=\sqrt{15}$
4 BM
(iv) $\mathrm{Cs}\left[\mathrm{FeCl_4}\right]$
IUPAC name: Caesium tetrachloroferrate (III)
Oxidation state of $\mathrm{Fe}=+3$
Electronic configuration of $d^{6}=e_{g}{ }^{2} t_{2 g}{ }^{3}$
Coordination number $=4$
Shape: tetrahedral
Stereochemistry: optically inactive
Magnetic moment:
$=\sqrt{n(n+2)}$
$=\sqrt{5(5+2)}$
$=\sqrt{35} \sim 6 \mathrm{BM}$
(v) $\mathrm{K_4}\left[\mathrm{Mn}(\mathrm{CN})_{6}\right]$
Potassium hexacyanomanganate(II)
Oxidation state of manganese $=+2$
Electronic configuration: $d^{5+}: t_{2 g}{ }^{5}$
Coordination number $=6$
Shape: octahedral.
Streochemistry: optically inactive
Magnetic moment, $\tilde{\mathrm{A}} \tilde{\mathrm{A}} \mathrm{A_1 / 4}=\sqrt{n(n+2)}$
$=\sqrt{1(1+2)}$
$=\sqrt{3}$
$=1.732 \mathrm{BM}$
9.25 Explain the violet colour of the complex $\left[\mathrm{Ti}\left(\mathrm{H_2} \mathrm{O}\right)_{6}\right]^{3+}$ on the basis of crystal field theory.
Show Answer
#missing9.26 What is meant by the chelate effect? Give an example.
Show Answer
Answer
When a ligand attaches to the metal ion in a manner that forms a ring, then the metal- ligand association is found to be more stable. In other words, we can say that complexes containing chelate rings are more stable than complexes without rings. This is known as the chelate effect.
For example:
$ \begin{aligned} \mathrm{Ni _{(a q)}}^{2+}+6 \mathrm{NH _{3(a q)}} \longleftrightarrow & {\left[\mathrm{Ni}\left(\mathrm{NH _3}\right) _{6}\right] _{(a q)}^{2+} } \\ & \log \beta=7.99 \\ \mathrm{Ni _{(a q)}}^{2+}+3 \mathrm{en _{(a q)}} \longleftrightarrow & {\left[\mathrm{Ni}(\mathrm{en}) _{3}\right] _{(a q)}^{2+} } \\ & \log \beta=18.1 \\ & \text { (more stable) } \end{aligned} $
9.27 Discuss briefly giving an example in each case the role of coordination compounds in:
(i) biological systems
(ii) medicinal chemistry and
(iii) analytical chemistry
(iv) extraction/metallurgy of metals.
Show Answer
Answer
(i) Role of coordination compounds in biological systems:
We know that photosynthesis is made possible by the presence of the chlorophyll pigment. This pigment is a coordination compound of magnesium. In the human biological system, several coordination compounds play important roles. For example, the oxygen-carrier of blood, i.e., haemoglobin, is a coordination compound of iron.
(ii) Role of coordination compounds in medicinal chemistry:
Certain coordination compounds of platinum (for example, cis-platin) are used for inhibiting the growth of tumours.
(iii) Role of coordination compounds in analytical chemistry:
During salt analysis, a number of basic radicals are detected with the help of the colour changes they exhibit with different reagents. These colour changes are a result of the coordination compounds or complexes that the basic radicals form with different ligands.
(iii) Role of coordination compounds in extraction or metallurgy of metals:
The process of extraction of some of the metals from their ores involves the formation of complexes. For example, in aqueous solution, gold combines with cyanide ions to form $\left[\mathrm{Au}(\mathrm{CN})_{2}\right]$. From this solution, gold is later extracted by the addition of zinc metal.
9.28 How many ions are produced from the complex $\mathrm{Co}\left(\mathrm{NH_3}\right)_6 \mathrm{Cl_2}$ in solution?
(i) 6
(ii) 4
(iii) 3
(iv) 2
Show Answer
Answer
(iii) The given complex can be written as $\left[\mathrm{Co}\left(\mathrm{NH_3}\right)_{6}\right] \mathrm{Cl_2}$
Thus, $\left[\mathrm{Co}\left(\mathrm{NH_3}\right)_{6}\right]^{+}$along with two $\mathrm{Cl}^{-}$ions are produced.
9.29 Amongst the following ions which one has the highest magnetic moment value?
(i) $\left[\mathrm{Cr}\left(\mathrm{H_2} \mathrm{O}\right)6\right]^{3+}$
(ii) $\left[\mathrm{Fe}\left(\mathrm{H_2} \mathrm{O}\right)_6\right]^{2+}$
(iii) $\left[\mathrm{Zn}\left(\mathrm{H_2} \mathrm{O}\right)_6\right]^{2+}$
Show Answer
Answer
(i) No. of unpaired electrons in $\left[\mathrm{Cr}\left(\mathrm{H_2} \mathrm{O}\right)_{6}\right]^{3+}=3$
Then, $=\sqrt{n(n+2)}$
$=\sqrt{3(3+2)}$
$=\sqrt{15}$
$\sim 4 \mathrm{BM}$
(ii) No. of unpaired electrons in $\left[\mathrm{Fe}\left(\mathrm{H_2} \mathrm{O}\right)_{6}\right]^{2+}=4$
Then, $=\sqrt{4(4+2)}$
$=\sqrt{24}$
$\sim 5 \mathrm{BM}$
(iii) No. of unpaired electrons in $\left[\mathrm{Zn}\left(\mathrm{H_2} \mathrm{O}\right)_{6}\right]^{2+}=0$
Hence, $\left[\mathrm{Fe}\left(\mathrm{H_2} \mathrm{O}\right)_{6}\right]^{2+}$ has the highest magnetic moment value.
9.30 Amongst the following, the most stable complex is
(i) $\left[\mathrm{Fe}\left(\mathrm{H_2} \mathrm{O}\right)_6\right]^{3+}$
(ii) $\left[\mathrm{Fe}\left(\mathrm{NH_3}\right)_6\right]^{3+}$
(iii) $\left[\mathrm{Fe}\left(\mathrm{C_2} \mathrm{O_4}\right)_3\right]^{3-}$
(iv) $\left[\mathrm{FeCl_6}\right]^{3-}$
Show Answer
#missing9.31 Amongst the following, the most stable complex is
(i) $\left[\mathrm{Fe}\left(\mathrm{H}_2 \mathrm{O}\right)_6\right]^{3+}$
(ii) $\left[\mathrm{Fe}\left(\mathrm{NH}_3\right)_6\right]^{3+}$
(iii) $\left[\mathrm{Fe}\left(\mathrm{C}_2 \mathrm{O}_4\right)_3\right]^{3-}$
(iv) $\left[\mathrm{FeCl}_6\right]^{3-}$
Show Answer
Answer
We know that the stability of a complex increases by chelation. Therefore, the most stable complex is $\left[\mathrm{Fe}\left(\mathrm{C_2} \mathrm{O_4}\right)_{3}\right]^{3 .}$.

9.32 What will be the correct order for the wavelengths of absorption in the visible region for the following:
$$ \left[\mathrm{Ni}\left(\mathrm{NO_2}\right)_6\right]^{4-},\left[\mathrm{Ni}\left(\mathrm{NH_3}\right)_6\right]^{2+},\left[\mathrm{Ni}\left(\mathrm{H_2} \mathrm{O}\right)_6\right]^{2+} ? $$
Show Answer
Answer
The central metal ion in all the three complexes is the same. Therefore, absorption in the visible region depends on the ligands. The order in which the CFSE values of the ligands increases in the spectrochemical series is as follows:
$\mathrm{H_2} \mathrm{O}<\mathrm{NH_3}<\mathrm{NO_2}$
Thus, the amount of crystal-field splitting observed will be in the following order: $\Delta _{\left(\mathrm{H _2} \mathrm{O}\right)}<\Delta _{\left(\mathrm{NH _3}\right)}<\Delta _{\left(\mathrm{NO _2}^{-}\right)}$
Hence, the wavelengths of absorption in the visible region will be in the order:
$\left[\mathrm{Ni}\left(\mathrm{H _2} \mathrm{O}\right) _{6}\right]^{2+}>\left[\mathrm{Ni}\left(\mathrm{NH_3}\right) _{6}\right]^{2+}>\left[\mathrm{Ni}\left(\mathrm{NO _2}\right) _{6}\right]^{4}$










