Nuclei
Multiple Choice Questions (MCQs)
1. Suppose we consider a large number of containers each containing initially 10000 atoms of a radioactive material with a half life of 1 yr. After 1 yr,
(a) all the containers will have 5000 atoms of the material
(b) all the containers will contain the same number of atoms of the material but that number will only be approximately 5000
(c) the containers will in general have different numbers of the atoms of the material but their average will be close to 5000
(d) none of the containers can have more than 5000 atoms
Show Answer
Thinking Process
$T_{\sqrt{ } 2}=\frac{\ln 2}{\lambda}, \lambda \rightarrow$ decay constant
Answer
(c) Radioactivity is a process due to which a radioactive material spontaneously decays. In half-life $(t=1 yr)$ of the material on the average half the number of atoms will decay.
Therefore, the containers will in general have different number of atoms of the material, but their average will be approx 5000 .
-
(a) This option is incorrect because radioactive decay is a random process, and not all containers will have exactly 5000 atoms after 1 year. The number of atoms remaining will vary due to the probabilistic nature of decay.
-
(b) This option is incorrect because, while the average number of atoms remaining will be approximately 5000, the actual number in each container will vary. The decay process is random, so the exact number of atoms will differ between containers.
-
(d) This option is incorrect because it is possible for some containers to have more than 5000 atoms remaining after 1 year. The half-life indicates an average decay rate, but individual containers can have more or fewer atoms due to the randomness of the decay process.
2. The gravitational force between a $H$-atom and another particle of mass $m$ will be given by Newton’s law
$$ F=G \frac{M \cdot m}{r^{2}} \text {, where } r \text { is in } km \text { and } $$
(a) $M=m_{\text {proton }}+m_{\text {electron }}$
(b) $M=m_{\text {proton }}+m_{\text {electron }}-\frac{B}{c^{2}}(B=13.6 eV)$.
(c) $M$ is not relate to the mass of the hydrogen atom.
(d) $M=m_{\text {proton }}+m_{\text {electron }}-\frac{|V|}{c^{2}}(|V|=$ magnitude of the potential energy of electron in the $H$-atom.
Show Answer
Answer
(b) Given, $F=\frac{G M m}{r^{2}}$
$$ \begin{aligned} M & =\text { effective mass of hydrogen atom } \\ & =\text { mass of electron }+ \text { mass of proton }-\frac{B^{2}}{C} \end{aligned} $$
where $B$ is $B E$ of hydrogen atom $=13.6 eV$.
-
(a) Incorrect because it does not account for the binding energy of the hydrogen atom. The mass of the hydrogen atom is slightly less than the sum of the masses of the proton and electron due to the binding energy.
-
(c) Incorrect because the gravitational force calculation requires the mass of the hydrogen atom, which is related to the masses of the proton and electron and the binding energy.
-
(d) Incorrect because the term (\frac{|V|}{c^{2}}) represents the potential energy of the electron in the hydrogen atom, not the binding energy. The correct term should be (\frac{B}{c^{2}}), where (B) is the binding energy.
3. When a nucleus in an atom undergoes a radioactive decay, the electronic energy levels of the atom
(a) do not change for any type of radioactivity
(b) change for $\alpha$ and $\beta$-radioactivity but not for $\gamma$-radioactivity
(c) change for $\alpha$-radioactivity but not for others
(d) change for $\beta$-radioactivity but not for others
Show Answer
Answer
(b) $\alpha-\beta$ particle carries one unit of negative charge, an $\alpha$-particle carries 2 units of positive charge and $\gamma$ (particle) carries no charge, therefore electronic energy levels of the atom charges for $\alpha$ and $\beta$ decay, but not for $\gamma$-decay.
- (a) This option is incorrect because the electronic energy levels do change for $\alpha$ and $\beta$-radioactivity due to the change in the nuclear charge and the number of electrons in the atom.
- (c) This option is incorrect because $\beta$-radioactivity also changes the electronic energy levels, not just $\alpha$-radioactivity.
- (d) This option is incorrect because $\alpha$-radioactivity also changes the electronic energy levels, not just $\beta$-radioactivity.
4. $M_{x}$ and $M_{y}$ denote the atomic masses of the parent and the daughter nuclei respectively in radioactive decay. The $Q$-value for a $\beta^{-}$decay is $Q_1$ and that for a $\beta^{+}$decay is $Q_2$. If $m_{e}$ denotes the mass of an electron, then which of the following statements is correct?
(a) $Q_1=(M_{x}-M_{y}) c^{2}$ and $Q_2=[M_{x}-M_{y}-2 m_{e}] c^{2}$
(b) $Q_1=(M_{x}-M_{y}) c^{2}$ and $Q_2=(M_{x}-M_{y}) c^{2}$
(c) $Q_1=(M_{x}-M_{y}-2 m_{e}) c^{2}$ and $Q_2=(M_{x}-M_{y}+2 c_{e}) c^{2}$
(d) $Q_1=(M_{x}-M_{y}+2 m_{e}) c^{2}$ and $Q_2=(M_{x}-m_{y}+2 m_{e}) c^{2}$
Show Answer
Answer
(a) Let the nucleus is $ _{z} X^{A} \cdot \beta^{+}$decay is represented as
$${}_z X^A \rightarrow _{z-1} Y^A+ _ {+1} e^0 +v+Q_2 \newline $$
$$\therefore \quad Q_2 =[m( {} _z X^A) - m_n ({} _{z-1} Y^A) - m _{e}] c^{2} $$
$$ =[m( {} _z X^A) + zm _e - m_n ({} _{z-1} Y^A) -(z-1) m_e - 2m_e]c^{2} $$
$$ =[m( {} _z X^A) -m({} _{z-1} Y^A)-2 m _{e}] c^{2} $$
$$ =(M _{x}- M _{y} -2 m _{e}) c^{2} $$
$\beta^{-}$decay is represented as
$$ \therefore \quad {}_z X^A \rightarrow _{z-1} y^A+ _ {+1} e^0 +v+Q_2 \newline $$
$$ \alpha_1 =[m( {} _z X^A) - m_n ({} _{z-1} Y^A) - m _{e}] c^{2} $$
$$ =[m( {} _z X^A) + zm _e - m_n ({} _{z+1} Y^A) -(z-1) m_e ] c^2 $$
$$ =[m( {} _z X^A) -m({} _{z-1} Y^A)] c^{2} $$
$$ =(M _{x}- M _{y} ) c^{2} $$
-
Option (b): This option states that the Q-value for both $\beta^{-}$ decay and $\beta^{+}$ decay is $(M_{x} - M_{y}) c^{2}$. This is incorrect because, for $\beta^{+}$ decay, the mass of two electrons (one positron and one electron) must be accounted for, leading to the term $-2m_{e}$ in the Q-value expression. Therefore, the correct Q-value for $\beta^{+}$ decay is $(M_{x} - M_{y} - 2m_{e}) c^{2}$, not $(M_{x} - M_{y}) c^{2}$.
-
Option (c): This option states that the Q-value for $\beta^{-}$ decay is $(M_{x} - M_{y} - 2m_{e}) c^{2}$ and for $\beta^{+}$ decay is $(M_{x} - M_{y} + 2c_{e}) c^{2}$. This is incorrect because, for $\beta^{-}$ decay, the correct Q-value is $(M_{x} - M_{y}) c^{2}$, as there is no need to account for the mass of electrons. Additionally, the term $2c_{e}$ in the Q-value for $\beta^{+}$ decay is incorrect; it should be $-2m_{e}$, not $+2c_{e}$.
-
Option (d): This option states that the Q-value for $\beta^{-}$ decay is $(M_{x} - M_{y} + 2m_{e}) c^{2}$ and for $\beta^{+}$ decay is $(M_{x} - m_{y} + 2m_{e}) c^{2}$. This is incorrect because, for $\beta^{-}$ decay, the correct Q-value is $(M_{x} - M_{y}) c^{2}$, and there is no addition of $2m_{e}$. For $\beta^{+}$ decay, the correct Q-value is $(M_{x} - M_{y} - 2m_{e}) c^{2}$, not $(M_{x} - m_{y} + 2m_{e}) c^{2}$.
5. Tritium is an isotope of hydrogen whose nucleus triton contains 2 neutrons and 1 proton. Free neutrons decay into $p+\bar{e}+\bar{n}$. If one of the neutrons in Triton decays, it would transform into $He^{3}$ nucleus. This does not happen. This is because
(a) Triton energy is than that of $aHe^{3}$ nucleus
(b) The electron created in the beta decay process cannot remain in the nucleus
(c) both the neutons in triton have to decay simultaneously resulting in a nucleus with 3 protons, which is not a $He^{3}$ nucleus.
(d) free neutrons decay due to external perturbations which is absent in triton nucleus
Show Answer
Thinking Process
Isotopes of an element are having same atomic numbers and different mass numbers.
Answer
(a) Tritium $( _1 H^{3})$ contains 1 proton and 2 neutrons. A neutron decays as $n \rightarrow P+\bar{e}+\bar{v}$, the nucleus may have 2 protons and one neutron, i.e., tritium will transform into $2 He^{3}$ (2 protons and 1 neutron).
Triton energy is less than that of $2 He^{3}$ nucleus, i.e., transformation is not allowed energetically.
-
(b) The electron created in the beta decay process cannot remain in the nucleus: This is incorrect because the electron produced in beta decay is not required to remain in the nucleus. It is emitted and leaves the nucleus, so this does not prevent the transformation.
-
(c) both the neutrons in triton have to decay simultaneously resulting in a nucleus with 3 protons, which is not a $He^{3}$ nucleus: This is incorrect because the decay of a single neutron in triton would result in a $He^{3}$ nucleus (2 protons and 1 neutron). There is no requirement for both neutrons to decay simultaneously.
-
(d) free neutrons decay due to external perturbations which is absent in triton nucleus: This is incorrect because free neutrons decay spontaneously due to the weak nuclear force, not due to external perturbations. The absence of external perturbations in the triton nucleus does not prevent the decay of a neutron within it.
6. Heavy stable nuclei have more neutrons than protons. This is because of the fact that
(a) neutrons are heavier than protons
(b) electrostatic force between protons are repulsive
(c) neutrons decay into protons through beta decay
(d) nuclear forces between neutrons are weaker than that between protons
Show Answer
Answer
(b) Stable heavy nuclei have more neutrons than protons. This is because electrostatic force between protons is repulsive, which may reduce stability.
-
(a) Neutrons are heavier than protons: The mass difference between neutrons and protons is not the reason for the neutron-to-proton ratio in stable heavy nuclei. The stability is more influenced by the balance between nuclear forces and electrostatic repulsion.
-
(c) Neutrons decay into protons through beta decay: While neutrons can decay into protons, this process does not explain why stable heavy nuclei have more neutrons. Beta decay is more relevant to the stability of individual neutrons rather than the overall neutron-to-proton ratio in heavy nuclei.
-
(d) Nuclear forces between neutrons are weaker than that between protons: This is incorrect because nuclear forces (strong interaction) are actually similar in strength between any nucleons (protons or neutrons). The difference in stability is due to the repulsive electrostatic forces between protons, not the strength of nuclear forces.
7. In a nuclear reactor, moderators slow down the neutrons which come out in a fission process. The moderator used have light nuclei. Heavy nuclei will not serve the purpose, because
(a) they will break up
(b) elastic collision of neutrons with heavy nuclei will not slow them down
(c) the net weight of the reactor would be unbearably high
(d) substances with heavy nuclei do not occur in liquid or gaseous state at room temperature
Show Answer
Thinking Process
When there is an elastic collision between two bodies of same mass their velocities are exchanged.
Answer
(b) According to the question, the moderator used have light nuclei (like proton). When protons undergo perfectly elastic collision with the neutron emitted their velocities are exchanged, i.e., neutrons come to rest and protons move with the velocity of neutrons. Heavy nuclei will not serve the purpose because elastic collisions of neutrons with heavy nuclei will not slow them down.
- (a) Heavy nuclei will not break up easily under neutron collisions; they are generally stable and can withstand such interactions.
- (c) The net weight of the reactor is not a primary concern in the choice of moderator; the effectiveness in slowing down neutrons is the critical factor.
- (d) Substances with heavy nuclei can exist in solid state at room temperature, and the state of matter is not a determining factor for their use as moderators.
Multiple Choice Questions (More Than One Options)
8. Fusion processes, like combining two deuterons to form a He nucleus are impossible at ordinary temperatures and pressure.The reasons for this can be traced to the fact
(a) nuclear forces have short range
(b) nuclei are positively charged
(c) the original nuclei must be completely ionized before fusion can take place
(d) the original nuclei must first break up before combining with each other
Show Answer
Answer
$(a, b)$
Fusion processes are impossible at ordinary temperatures and pressures. The reason is nuclei are positively charged and nuclear forces are short range strongest forces.
-
Option (c) is incorrect because the original nuclei do not need to be completely ionized before fusion can take place. Fusion can occur with nuclei that are already ionized or partially ionized, as long as they have enough kinetic energy to overcome the electrostatic repulsion between them.
-
Option (d) is incorrect because the original nuclei do not need to break up before combining with each other. Fusion involves the direct combination of nuclei, not their prior disintegration.
9. Samples of two radioactive nuclides $A$ and $B$ are taken $\lambda_{A}$ and $\lambda_{B}$ are the disintegration constants of $A$ and $B$ respectively. In which of the following cases, the two samples can simultaneously have the same decay rate at any time?
(a) Initial rate of decay of $A$ is twice the initial rate of decay of $B$ and $\lambda_{A}=\lambda_{B}$
(b) Initial rate of decay of $A$ is twice the initial rate of decay of $B$ and $\lambda_{A}>\lambda_{B}$
(c) Initial rate of decay of $B$ is twice the initial rate of decay of $A$ and $\lambda_{A}>\lambda_{B}$
(d) Initial rate of decay of $B$ is same as the rate of decay of $A$ at $t=2 h$ and $\lambda_{B}<\lambda_{A}$
Show Answer
Answer
$(b, d)$
The two samples of the two radioactive nuclides $A$ and $B$ can simultaneously have the same decay rate at any time if initial rate of decay of $A$ is twice the initial rate of decay of $B$ and $\lambda_{A}>\lambda_{B}$. Also, when initial rate of decay of $B$ is same as rate of decay of $A$ at $t=2$ hand $\lambda_{B}<\lambda_{A}$.
-
Option (a): This option states that the initial rate of decay of $A$ is twice the initial rate of decay of $B$ and $\lambda_{A}=\lambda_{B}$. If the decay constants are equal, the decay rates will always maintain the same ratio as their initial rates. Therefore, they cannot have the same decay rate at any time because the initial rate of decay of $A$ is twice that of $B$.
-
Option (c): This option states that the initial rate of decay of $B$ is twice the initial rate of decay of $A$ and $\lambda_{A}>\lambda_{B}$. Given that $\lambda_{A}>\lambda_{B}$, $A$ will decay faster than $B$. Since $B$ starts with a higher initial rate of decay, the decay rates of $A$ and $B$ will diverge over time, and they will not be able to have the same decay rate simultaneously.
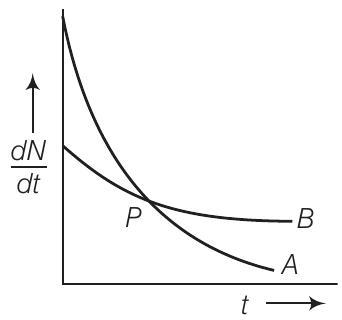
10. The variation of decay rate of two radioactive samples $A$ and $B$ with time is shown in figure.
Which of the following statements are true?
(a) Decay constant of $A$ is greater than that of $B$, hence $A$ always decays faster than $B$
(b) Decay constant of $B$ is greater than that of $A$ but its decay rate is always smaller than that of $A$
(c) Decay constant of $A$ is greater than that of $B$ but it does not always decay faster than $B$
(d) Decay constant of $B$ is smaller than that of $A$ but still its decay rate becomes equal to that of $A$ at a later instant
Show Answer
Answer
$(c, d)$
From the given figure, it is clear that slope of curve $A$ is greater than that of curve $B$. So rate of decay is faster for $A$ than that of $B$.
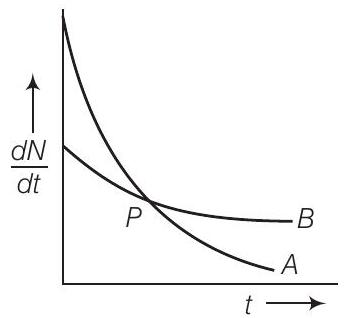
We know that $(\frac{d N}{d t}) \propto, \lambda$, at any instant of time hence we can say that $\lambda_{A}>\lambda_{B}$. At point $P$ shown in the diagram the two curve intersect.
Hence at point $P$, rate of decay for both $A$ and $B$ is the same.
-
(a) Decay constant of ( A ) is greater than that of ( B ), hence ( A ) always decays faster than ( B ): This statement is incorrect because although the decay constant of ( A ) is greater than that of ( B ), ( A ) does not always decay faster than ( B ). The decay rate depends on both the decay constant and the number of undecayed nuclei present. At point ( P ), the decay rates of ( A ) and ( B ) are equal, indicating that ( A ) does not always decay faster than ( B ).
-
(b) Decay constant of ( B ) is greater than that of ( A ) but its decay rate is always smaller than that of ( A ): This statement is incorrect because the decay constant of ( B ) is not greater than that of ( A ). The given figure shows that the slope of curve ( A ) is greater than that of curve ( B ), indicating that the decay constant of ( A ) is greater than that of ( B ). Additionally, the decay rate of ( B ) is not always smaller than that of ( A ); at point ( P ), the decay rates are equal.
Very Short Answer Type Questions
11. $He_2^{3}$ and $He_1^{3}$ nuclei have the same mass number. Do they have the same binding energy?
Show Answer
Answer
Nuclei $He_2^{3}$ and $He_1^{3}$ have the same mass number. $He_2^{3}$ has two proton and one neutron. $He_1^{3}$ has one proton and two neutron. The repulsive force between protons is missing in $ _1 He^{3}$ so the binding energy of $ _1 He^{3}$ is greater than that of $ _2 He^{3}$.
12. Draw a graph showing the variation of decay rate with number of active nuclei.
Show Answer
Answer
We know that, rate of decay $=\frac{-d N}{d t}=\lambda N$
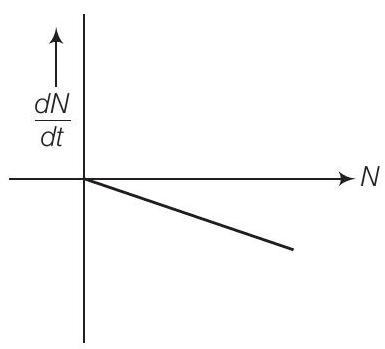
where decay constant $(\lambda)$ is constant for a given radioactive material. Therefore, graph between $N$ and $\frac{d N}{d t}$ is a straight line as shown in the diagram.
13. Which sample $A$ or $B$ shown in figure has shorter mean-life?

Show Answer
Answer
From the given figure, we can say that
$$ \text { at } t=0, (\frac{d N}{d t})_{A}=(\frac{d N}{d t}) _{B}\newline \Rightarrow \qquad(N_0)_A=(N_0)_B $$
Considering any instant $t$ by drawing a line perpendicular to time axis, we find that $(\frac{d N}{d t})_A>(\frac{d N}{d t})_B$
$$ \begin{matrix} \Rightarrow & \lambda_{A} N_{A}>\lambda_{B} N_{B} & \\ \because & N_{A}>N_{B} & \text { (rate of decay of } B \text { is slower) } \\ \therefore & \lambda_{B}>\lambda_{A} & \\ \Rightarrow & \tau_{A}>\tau_{B} & [\because \text { Average life } \tau=\frac{1}{\lambda}] \end{matrix} $$
14. Which one of the following cannot emit radiation and why? Excited nucleus, excited electron.
Show Answer
Answer
Excited electron cannot emit radiation because energy of electronic energy levels is in the range of $eV$ and not $MeV$ ( mega electron volt ). $\gamma$-radiations have energy of the order of $MeV$.
15. In pair annihilation, an electron and a positron destroy each other to produce gamma radiations. How is the momentum conserved?
Show Answer
Answer
In pair annihilation, an electron and a positron destroy each other to produce $2 \gamma$ photons which move in opposite directions to conserve linear momentum. The annihilation is shown below $ _0 e^{-1}+ _0 e^{+1}\rightarrow 2 \gamma$ ray photons.
Short Answer Type Questions
16. Why do stable nuclei never have more protons than neutrons?
Show Answer
Answer
Because protons are positively charged and repel one another electrically. This repulsion becomes so great in nuclei with more than 10 protons or so, that an excess of neutrons which produce only attractive forces, is required for stability.
17. Consider a radioactive nucleus $A$ which decays to a stable nucleus $C$ through the following sequence
$$ A \rightarrow B \rightarrow C $$
Here $B$ is an intermediate nuclei which is also radioactive. Considering that there are $N_0$ atoms of $A$ initially, plot the graph showing the variation of number of atoms of $A$ and $B$ versus time.
Show Answer
Thinking Process
Based or decay law of unstable radioactive nuclei.
Answer
Consider the situation shown in the graph.
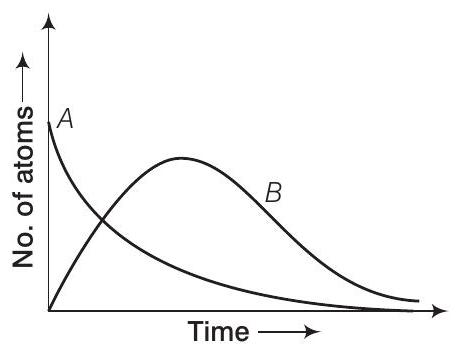
At $t=0, N_{A}=N_0$ (maximum) while $N_{B}=0$. As time increases, $N_{A}$ decreases exponentially and the number of atoms of $B$ increases. They becomes $(N_{B})$ maximum and finally drop to zero exponentially by radioactive decay law.
18. A piece of wood from the ruins of an ancient building was found to have a ${ }^{14} C$ activity of 12 disintegrations per minute per gram of its carbon content. The ${ }^{14} C$ activity of the living wood is 16 disintegrations per minute per gram. How long ago did the tree, from which the wooden sample came, die? Given half-life of ${ }^{14} C$ is $5760 yr$.
Show Answer
Thinking Process
Carbon dating is a technique that uses the decay of carbon $-14({ }^{14} C)$ to estimate the age of organic materials, such as wood and leather.
Answer
Given, $R=12 dis / min$ per $g, R_0=16 dis / min$ per $g, T_{1 / 2}=5760 yr$ Let $t$ be the span of the tree
According to radioactive decay law,
$$ R=R_0 e^{-\lambda t} \text { or } \frac{R}{R_0}=e^{-\lambda t} \text { or } e^{\lambda t}=\frac{R_0}{R} $$
Taking log on both the sides
$$ \lambda t \log _e e =\log _e \frac{R_0}{R} \Rightarrow \lambda t=\log _{10} \frac{16}{12} \quad \times 2.303 \newline $$
$$ t =\frac{2.303(\log 4-\log 3)}{\lambda} \newline =\frac{2.303(0.6020-4.771) \times 5760}{0.6931} \quad [{\because \lambda=\frac{0.6931}{T_{1 / 2}}}] \newline =2391.20 \text{yr} $$
19. Are the nucleons fundamental particles, or do they consist of still smaller parts? One way to find out is to probe a nucleon just as Rutherford probed an atom. What should be the kinetic energy of an electron for it to be able to probe a nucleon? Assume the diameter of a nucleon to be approximately $10^{-15} m$.
Show Answer
Thinking Process
We have to use de-Broglie formula $(\boldsymbol{\lambda}=h / p)$ to find momentum of the particle.
Answer
Each particle (neutron and proton) present inside the nucleus is called a nucleon.
Let $\boldsymbol{\lambda}$ be the wavelength $\boldsymbol{\lambda}=10^{-15} m$
To detect separate parts inside a nucleon, the electron must have wavelength less than $10^{-15} m$.
We know that
Energy
$$ \begin{aligned} \lambda & =\frac{h}{p} \text { and } KE=PE \\ & =\frac{h c}{\lambda} \end{aligned} $$
From Eq. (i) and Eq. (ii),
$$ \begin{aligned} \text { kinetic energy of electron } & =P E=\frac{h c}{\lambda}-\frac{6.6 \times 10^{-34} \times 3 \times 10^{8}}{10^{-15} \times 1.6 \times 10^{-19}} eV \\ KE & =10^{9} eV \end{aligned} $$
20. A nuclide 1 is said to be the mirror isobar of nuclide 2 if $Z_1=N_2$ and $Z_2=N_1$.(a) What nuclide is a mirror isobar of $ _11^{23} Na$ ? (b) Which nuclide out of the two mirror isobars have greater binding energy and why?
Show Answer
Thinking Process
Based on the mirror isobar concept and binding energy concept.
Answer
(a) According to question, a nuclide 1 is said to be mirror isobar of nuclide 2 , if $Z_1=N_2$ and $Z_2=N_1$.
Now in $ _{11} Na^{23}, Z_1=11, N_1=23-11=12$
$\therefore$ Mirror isobar of $ _{11} Na^{23}$ is $ _{12}, Mg^{23}$, for which $Z_2=12=N_1$ and $N_2=23-12=11=Z_1$
(b) As $ _12^{23} Mg$ contains even number of protons (12) against $ _11^{23} Na$ which has odd number of protons (11), therefore $ _11^{23} Mg$ has greater binding energy than $ _{11} Na^{23}$.
Long Answer Type Questions
21. Sometimes a radioactive nucleus decays into a nucleus which itself is radioactive. An example is
$^{38}\text{Sulphur} \quad \quad \substack{\text{half-life} \\ \\ =2.48h}{\longrightarrow} {\quad} ^{38}Cl \quad \substack{\text{half-life} \\ \\ =0.62h} {\longrightarrow} {\quad} ^{38}Ar(\text{stable})$
Assume that we start with $1000{ }^{38} S$ nuclei at time $t=0$. The number of ${ }^{38} Cl$ is of count zero at $t=0$ and will again be zero at $t=\infty$. At what value of $t$, would the number of counts be a maximum?
Show Answer
Thinking Process
To solve this problem concept of chain of two decays will be used. For the process,
$A \rightarrow B \rightarrow C$, the law of decay is $\frac{d N_{B}}{d t}=\lambda_{B} N_{B}+\lambda_{A} N_{A}$.
Answer
Consider the chain of two decays
$$ { }^{38} \mathrm{~S} \xrightarrow[2.48 \mathrm{~h}]{ }{ }^{38} \mathrm{Cl} \xrightarrow[0.62 \mathrm{~h}]{ }^{38} \mathrm{Ar} $$
At time $t$, Let ${ }^{38} S$ have $N_1(t)$ active nuclei and ${ }^{38} Cl$ have $N_2(t)$ active nuclei.
Also,
$$ \begin{aligned} \frac{d N_1}{d t} & =-\lambda_1 N_1=\text { rate of formation of } Cl^{38} . \\ \frac{d N_2}{d t} & =-\lambda_1 N_2+\lambda_1 N_1 \\ N_1 & =N_0 e^{-\lambda_1 t} \\ \frac{d N_2}{d t} & =\lambda_1 N_0 e^{-\lambda_1 t}-\lambda_2 N_2 \end{aligned} $$
$$ \text { But } \quad N_1=N_0 e^{-\lambda_1 t} $$
Multiplying by $e^{\lambda z^{\star}} d t$ and rearranging
$$ e^{\lambda_2 t} d N_2+\lambda_2 N_2 e^{\lambda_2 t} d t=\lambda_1 N_0 e^{(\lambda_2-\lambda_1) t} d t $$
Integrating both sides
$$ \begin{aligned} & N_2 e^{\lambda_2 t}=\frac{N_0 \lambda_1}{\lambda_2-\lambda_1} e^{(\lambda_2-\lambda_1) t}+C \\ & \text { Since, } \\ & \text { at } t=0, N_2=0, C=-\frac{N_0 \lambda_1}{\lambda_2-\lambda_1} \\ & N_2 e^{\lambda_2 t}=\frac{N_0 \lambda_1}{\lambda_2-\lambda_1}(e^{(\lambda_2-\lambda_1) t}-1) \\ & N_2=\frac{N_0 \lambda_1}{\lambda_2-\lambda_1}(e^{-\lambda_1 t}-e^{-\lambda_2 t}) \\ & \frac{d N_2}{d t}=0 \\ & \text { For maximum count, } \quad \text {.. (ii) } \\ & \lambda_1 N_0 e^{-\lambda_1 t}-\lambda_2 N_2=0 \\ & e^{\lambda_2 t}-\frac{\lambda_2}{\lambda_1} \cdot \frac{\lambda_1}{N_2}=\frac{\lambda_2}{\lambda_1} e^{\lambda_1 t} \\ & e^{\lambda_2 t}-\frac{.\lambda_2)}{\lambda_2} e^{\lambda_1 t}[e^{(\lambda_2-\lambda_1) t}-1]=0 \text { [From Eq. (i)] } \\ & \text { or } e^{\lambda_2 t}+\frac{\lambda_2}{(\lambda_2-\lambda_1)} e^{\lambda_1 t}=0 \end{aligned} $$
$$ 1-\frac{\lambda_2}{(\lambda_2-\lambda_1)}+\frac{\lambda_2}{(\lambda_2-\lambda_1)} e^{(\lambda_1-\lambda_2) t}=0 \newline $$ $$\frac{\lambda_2}{(\lambda_2-\lambda_1)} e^{(\lambda_1-\lambda_2) t}=\frac{\lambda_2}{(\lambda_2-\lambda_1)}-1 \newline$$
$$e^{(\lambda_1-\lambda_2) t}=\frac{\lambda_1}{\lambda_2} \newline t=\log _{e} \frac{\lambda_1}{\lambda_2} /(\lambda_1-\lambda_2) \newline$$ $$=\frac{\log _e \frac{2.48}{0.62}}{2.48-0.62} \newline $$ $$=\frac{\log _e 4}{1.86}=\frac{2.303 \times 2 \times 0.3010}{1.86} \newline $$
$$=0.745 s \qquad [\because \lambda=\frac{0.693}{T_{1 / 2}}] $$
Note Do not apply directly the formula of radioactive. Apply formulae related to chain decay.
22. Deuteron is a bound state of a neutron and a proton with a binding energy $B=2.2 MeV$. A $\gamma$-ray of energy $E$ is aimed at a deuteron nucleus to try to break it into a (neutron + proton) such that the $n$ and $p$ move in the direction of the incident $\gamma$-ray. If $E=B$, show that this cannot happen. Hence, calculate how much bigger than $B$ must be $E$ be for such a process to happen.
Show Answer
Thinking Process
Apply conservation of energy as well as conservation of momentum.
Answer
Given binding energy
$$ B=2.2 MeV $$
From the energy conservation law,
$$ E-B=K_{n}+K_{p}=\frac{p_n^{2}}{2 m}+\frac{p_p^{2}}{2 m} \qquad …(i) $$
From conservation of momentum,
$$ p_{n}+p_{p}=\frac{E}{C} \qquad …(ii) $$
As
$$ E=B \text {, Eq. (i) } p_n^{2}+p_p^{2}=0 $$
It only happen if $p_{n}=p_{p}=0$
So, the Eq. (ii) cannot satisfied and the process cannot take place.
Let $E=B+X$, where $X«B$ for the process to take place.
Put value of $p_{n}$ from Eq. (ii) in Eq. (i), we get
$$ X=\frac{(\frac{E}{c}-p_{p})}{2 m}+\frac{p_p^{2}}{2 m} $$
$$ or \qquad 2 p_p^{2}-\frac{2 E p_{p}}{c}+\frac{E^{2}}{c^{2}}-2 m X=0 $$
Using the formula of quadratic equation, we get
$$ p_{p}=\frac{\frac{2 E}{c} \pm \sqrt{\frac{4 E^{2}}{c^{2}}-8 (\frac{E^{2}}{c^{2}}-2 m X})}{4} $$
For the real value $p_{p}$, the discriminant is positive
$$ \begin{aligned} \frac{4 E^{2}}{c^{2}} & =8 (\frac{E^{2}}{c^{2}}-2 m X) \\ 16 m X & =\frac{4 E^{2}}{c^{2}} \\ X & =\frac{E^{2}}{4 m c^{2}} \approx \frac{B^{2}}{4 m c^{2}} \qquad[\because X < < B \Rightarrow E \cong B] \end{aligned} $$
23. The deuteron is bound by nuclear forces just as $H$-atom is made up of $p$ and $e$ bound by electrostatic forces. If we consider the force between neutron and proton in deuteron as given in the form a coulomb potential but with an effective charge $e^{\prime}$
$$ F=\frac{1}{4 \pi \varepsilon_0} \cdot \frac{e^{2}}{r} $$
estimate the value of $(e^{\prime} / e)$ given that the binding energy of a deuteron is $2.2 MeV$.
Show Answer
Answer
The binding energy is $H$-atom
$$ E=\frac{m e^{4}}{\pi \varepsilon_0^{2} h^{2}}=13.6 eV \quad …(i) $$
If proton and neutron had charge $e^{\prime}$ each and were governed by the same electrostatic force, then in the above equation we would need to replace electronic mass $m$ by the reduced mass $m^{\prime}$ of proton-neutron and the electronic charge $e$ by $e^{\prime}$.
$$ \begin{aligned} m^{\prime} & =\frac{M \times N}{M+N}=\frac{M}{2} \\ & =\frac{1836 m}{2}=918 m \end{aligned} $$
Here, $M$ represents mass of a neutron/proton
$$ \therefore \quad \text { Binding energy }=\frac{918 m(e^{\prime})^{4}}{8 \varepsilon_0^{2} h^{2}}=2.2 MeV \quad …(ii) $$
Dividing Eqs. (ii) and (i), we get
$$ \begin{aligned} 918 (\frac{e^{\prime}}{e})^4 & =\frac{2.2 MeV}{13.6 eV}=\frac{2.2 \times 10^{6}}{13.6} \\ (\frac{e^{\prime}}{e})^4 & =\frac{2.2 \times 10^{6}}{13.6 \times 918}=176.21 \\ \frac{e^{\prime}}{e} & =(176.21)^{1 / 4}=3.64 \end{aligned} $$
24. Before the neutrino hypothesis, the beta decay process was throught to be the transition.
$$ n\rightarrow p+e $$
If this was true, show that if the neutron was at rest, the proton and electron would emerge with fixed energies and calculate them. Experimentally, the electron energy was found to have a large range.
Show Answer
Answer
Before $\beta$-decay, neutron is at rest. Hence, $E_{n}=m_{n} c^{2}, p_{n}=0$
$$p_{n}=p_{p}+p_{e}$$
$$Or \qquad p_{p} + p_{e} = 0 \Rightarrow |p_p|=|p_{e}|=p $$
Also,
$E_{p} =(m_p^{2} c^{4}+p_p^{2} c^{2})^{\frac{1}{2}}, \\ E_{e} =(m_e^{2} c^{4}+p_p^{2} c^{2})^{\frac{1}{2}} \\ =(m_e^{2} c^{4}+p_e^{2} c^{2})^{\frac{1}{2}} $
From conservation of energy,
$$ \begin{aligned} (m_p^{2} c^{4}+p^{2} c^{2})^{\frac{1}{2}}+ & =(m_e^{2} c^{4}+p^{2} c^{2})^{\frac{1}{2}}=m_{n} c^{2} \\ m_{p} c^{2} \approx 936 MeV, m_{n} c^{2} & \approx 938 MeV, m_{e} c^{2}=0.51 MeV \end{aligned} $$
Since, the energy difference between $n$ and $p$ is small, $p c$ will be small, $p c«<m_{p} c^{2}$, while $p c$ may be greater than $m_{e} c^{2}$
$$ \Rightarrow \quad m_{p} c^{2}+\frac{p^{2} c^{2}}{2 m_p^{2} c^{4}}=m_{n} c^{2}-p c $$
To first order $\quad p c \simeq m_{n} c^{2}-m_{p} c^{2}=938 MeV-936 MeV=2 MeV$
This gives the momentum of proton or neutron. Then,
$$ \begin{aligned} E_{p} & =(m_p^{2} c^{4}+p^{2} c^{2})^{\frac{1}{2}}=\sqrt{936^{2}+2^{2}} \\ & \simeq 936 MeV \\ E_{e} & =(m_e^{2} c^{4}+p^{2} c^{2})^{\frac{1}{2}}=\sqrt{(0.51)^{2}+2^{2}} \\ & \simeq 2.06 MeV \end{aligned} $$
Long Answer Type Questions
25. The activity $R$ of an unknown radioactive nuclide is measured at hourly intervals. The result found are tabulated as follows
| $\boldsymbol{t}(h)$ | 0 | 1 | 2 | 3 | 4 |
|---|---|---|---|---|---|
| $\boldsymbol{R}(MB_{q})$ | 100 | 35.36 | 12.51 | 4.42 | 1.56 |
(i) Plot the graph of $R$ versus $t$ and calculate half-life from the graph.
(ii) Plot the graph of In $\frac{R}{R_0}$ versus $t$ and obtain the value of half-life from the graph.
Show Answer
Thinking Process
Based on Decay law and half-life.
Answer
In the table given below, we have listed values of $R(MB_{q})$ and $\ln \frac{R}{R_0}$.
| $\boldsymbol{t}(h)$ | 0 | 1 | 2 | 3 | 4 |
|---|---|---|---|---|---|
| $\boldsymbol{R}(MB_q)$ | 100 | 35.36 | 12.51 | 4.42 | 1.56 |
| $\frac{R}{R_0}$ | - | -1.04 | -2.08 | -3.11 | -4.16 |
(i) When we plot the graph of $R$ versust, we obtain an exponential curve as shown.
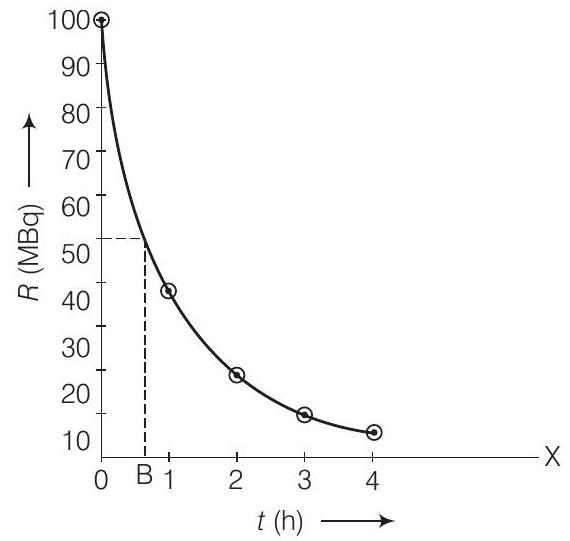
From the graph we can say that activity $R$ reduces to $50 %$ in $t=O B \approx 40 min$ So, $t_{1 / 2} \approx 40 min$.
(ii) The adjacent figure shows the graph of $\ln (R / R_0)$ versus $t$.
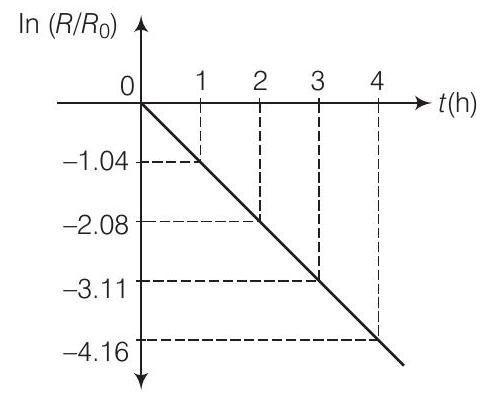
Slope of this graph $=-\lambda$
from the graph,
Half-life
$$ \begin{aligned} \lambda & =-\frac{-4.16-3.11}{1} \Rightarrow=1.05 h^{-1} \\ T_{1 / 2} & =\frac{0.693}{\lambda}=\frac{0.693}{1.05}=0.66 h \\ & =39.6 min \approx 40 min \end{aligned} $$
26. Nuclei with magic number of proton $Z=2,8,20,28,50,52$ and magic number of neutrons $N=2,8,20,28,50,82$ and 126 are found to be very stable.
(i) Verify this by caculating the proton. separation energy $S_{p}$ for ${ }^{120} Sn(Z=50)$ and ${ }^{121} Sb(Z=51)$.
The proton separation energy for a nuclide is the minimum energy required to separate the least tightly bound proton from a nucleus of that nuclide. It is given by
Given,
$$ \begin{aligned} S_{p} & =(M_{Z-1, N}+M_{H}-M_{Z, N}) c^{2} . \\ { }^{119} \ln & =118.9058 u,{ }^{120} S n=199.902199 u, \\ { }^{121} S b & =120.903824 u,{ }^{1} H=1.0078252 u . \end{aligned} $$
(ii) What does the existence of magic number indicate?
Show Answer
Answer
(i) The proton separation energy is given by
$$ \begin{aligned} S_{p S n} & =(M_{119.70}+M_{H}-M_{120,70}) c^{2} \\ & =(118.9058+1.0078252-119.902199) c^{2} \\ & =0.0114362 c^{2} \\ S_{p S p} & =(M_{120,70}+M_{H}-M_{121,70}) c^{2} \\ & =(119.902199+1.0078252-120.903822) c^{2} \\ & =0.0059912 c^{2} \end{aligned} $$
Similarly
Since, $S_{pSn}>S_{pSb}$, Sn nucleus is more stable than Sb nucleus.
(ii) The existence of magic numbers indicates that the shell structure of nucleus similar to the shell structure of an atom. This also explains the peaks in binding energy/nucleon curve.










