Moving Charges and Magnetism
Multiple Choice Questions (MCQs)
1. Two charged particles traverse identical helical paths in a completely opposite sense in a uniform magnetic field $\mathbf{B}=\mathrm{B}_{0} \hat{\mathbf{k}}$.
(a) They have equal z-components of momenta
(b) They must have equal charges
(c) They necessarily represent a particle, anti-particle pair
(d) The charge to mass ratio satisfy
$$ \frac{e}{m_{1}}+\frac{e}{m_{2}}=0 $$
Show Answer
Thinking Process
The uniqueness of helical path is determined by its pitch which is given by
$$ \text { Pitch }=\frac{2 \pi m v \cos \theta}{q B} $$
Answer
(d) For given pitch d correspond to charge particle, we have
$$ \frac{q}{m}=\frac{2 \pi v \cos \theta}{q B}=\text { constant } $$
Since, charged particles traverse identical helical paths in a completely opposite sense in a uniform magnetic field B, LHS for two particles should be same and of opposite sign. Therefore,
$$ \frac{e}{m_{1}}+\frac{e}{m_{2}}=0 $$
Note Consider e in place of $q$ in solution.
-
(a) They have equal z-components of momenta: This is incorrect because the z-component of momentum depends on both the charge and the velocity of the particles. Since the particles traverse identical helical paths in opposite senses, their velocities in the z-direction could be different, leading to different z-components of momenta.
-
(b) They must have equal charges: This is incorrect because the particles can have different charges as long as their charge-to-mass ratios are such that they produce identical helical paths in opposite senses. The condition for identical helical paths in opposite senses is that the charge-to-mass ratios are equal in magnitude but opposite in sign, not that the charges themselves are equal.
-
(c) They necessarily represent a particle, anti-particle pair: This is incorrect because having opposite helical paths in a magnetic field does not necessarily imply that the particles are a particle and its anti-particle. They could simply be two different particles with charge-to-mass ratios that are equal in magnitude but opposite in sign.
2. Biot-Savart law indicates that the moving electrons (velocity $v$ ) produce a magnetic field $\mathbf{B}$ such that
(a) $B$ is perpendicular of
(b) $B$ is parallel to $v$
(c) it obeys inverse cube law
(d) it is along the line joining the electron and point of observation
Show Answer
Thinking Process
Here use of Biot-Savart law play vital role.
Answer
(a) In Biot-Savart’s law, magnetic field $\mathbf{B} | \mathrm{idll} \times \mathbf{r}$ and idl due to flow of electron is in opposite direction of $\mathbf{v}$ and by direction of cross product of two vectors
$$ B \perp v $$
-
(b) $B$ is parallel to $v$: This is incorrect because, according to the Biot-Savart law, the magnetic field $\mathbf{B}$ is given by the cross product of the current element $\mathbf{idll}$ and the position vector $\mathbf{r}$. The cross product of two vectors is always perpendicular to the plane containing the vectors, hence $\mathbf{B}$ cannot be parallel to $\mathbf{v}$.
-
(c) it obeys inverse cube law: This is incorrect because the Biot-Savart law states that the magnetic field $\mathbf{B}$ produced by a current element is inversely proportional to the square of the distance from the element, not the cube. Mathematically, $\mathbf{B} \propto \frac{1}{r^2}$, where $r$ is the distance from the current element to the point of observation.
-
(d) it is along the line joining the electron and point of observation: This is incorrect because the magnetic field $\mathbf{B}$ produced by a moving charge or current element is not along the line joining the electron and the point of observation. Instead, it is perpendicular to both the direction of the current (or velocity of the electron) and the line joining the electron to the point of observation, as determined by the right-hand rule for the cross product.
3. A current carrying circular loop of radius $\mathrm{R}$ is placed in the $x$-y plane with centre at the origin. Half of the loop with $x>0$ is now bent so that it now lies in the $y-z$ plane.
(a) The magnitude of magnetic moment now diminishes
(b) The magnetic moment does not change
(c) The magnitude of $B$ at $(0,0, z), z>R$ increases
(d) The magnitude of $B$ at $(0,0, z), z \gg R$ is unchanged
Show Answer
Thinking Process
The magnetic moment of circular loop and the net magnitudes of magnetic moment of each semicircular loop of radius R lie in the $x-y$ plane and the $y-z$ plane are compared.
Answer
(a) The direction of magnetic moment of circular loop of radius $R$ is placed in the $x-y$ plane is along $z$-direction and given by $M=I\left(\pi^{2}\right)$, when half of the loop with $x>0$ is now bent so that it now lies in the $y-z$ plane, the magnitudes of magnetic moment of each semicircular loop of radius $R$ lie in the $x-y$ plane and the $y-z$ plane is $M^{\prime}=I\left(\pi^{2}\right) / 4$ and the direction of magnetic moments are along $z$-direction and $x$-direction respectively.
Their resultant
$$ M_{\text {net }}=\sqrt{M^{\prime 2}+M^{\prime 2}}=\sqrt{2} M^{\prime}=\sqrt{2} I\left(\pi^{2}\right) / 4 $$
So, $M_{\text {net }}<M$ or $M$ diminishes.
-
(b) The magnetic moment does not change: This option is incorrect because the magnetic moment does change when the loop is bent. Initially, the magnetic moment is along the z-direction. After bending, the magnetic moments of the two semicircular loops are in different planes (x-y and y-z), resulting in a net magnetic moment that is less than the original magnetic moment.
-
(c) The magnitude of $B$ at $(0,0, z), z>R$ increases: This option is incorrect because the magnetic field at a point on the z-axis (for $z > R$) is primarily determined by the net magnetic moment of the loop. Since the net magnetic moment diminishes after bending, the magnetic field at $(0,0,z)$ for $z > R$ would also decrease, not increase.
-
(d) The magnitude of $B$ at $(0,0, z), z \gg R$ is unchanged: This option is incorrect because the magnetic field at a point far along the z-axis (for $z \gg R$) is proportional to the net magnetic moment of the loop. Since the net magnetic moment diminishes after bending, the magnetic field at $(0,0,z)$ for $z \gg R$ would also decrease, not remain unchanged.
4. An electron is projected with uniform velocity along the axis of a current carrying long solenoid. Which of the following is true?
(a) The electron will be accelerated along the axis
(b) The electron path will be circular about the axis
(c) The electron will experience a force at $45^{\circ}$ to the axis and hence execute a helical path
(d) The electron will continue to move with uniform velocity along the axis of the solenoid
Show Answer
Thinking Process
Here, magnetic lorentz force comes into existence when a charge moves in uniform magnetic field produces by current carrying long solenoid.
Answer
(d) Magnetic Lorentz force electron is projected with uniform velocity along the axis of a current carrying long solenoid $F=-\operatorname{ev} B \sin 180^{\circ}=0\left(\theta=0^{\circ}\right)$ as magnetic field and velocity are parallel. The electron will continue to move with uniform velocity along the axis of the solenoid.
-
(a) The electron will not be accelerated along the axis because the magnetic Lorentz force acts perpendicular to both the velocity of the electron and the magnetic field. Since the electron’s velocity is parallel to the magnetic field inside the solenoid, the force is zero, and there is no acceleration along the axis.
-
(b) The electron path will not be circular about the axis because a circular path requires a perpendicular component of velocity relative to the magnetic field. In this case, the electron’s velocity is parallel to the magnetic field, resulting in no perpendicular force to cause circular motion.
-
(c) The electron will not experience a force at $45^{\circ}$ to the axis and hence execute a helical path because the force on a charged particle in a magnetic field is given by the Lorentz force, which depends on the sine of the angle between the velocity and the magnetic field. Here, the angle is $0^{\circ}$, making the force zero, so the electron will not follow a helical path.
5. In a cyclotron, a charged particle
(a) undergoes acceleration all the time
(b) speeds up between the dees because of the magnetic field
(c) speeds up in a dee
(d) slows down within a dee and speeds up between dees
Show Answer
Thinking Process
Here, understanding of working of cyclotron is needed.
Answer
(a) The charged particle undergoes acceleration as
(i) speeds up between the dees because of the oscillating electric field and
(ii) speed remain the same inside the dees because of the magnetic field but direction undergoes change continuously.
-
(b) The charged particle does not speed up between the dees because of the magnetic field; it speeds up due to the oscillating electric field present in the gap between the dees. The magnetic field only changes the direction of the particle within the dees.
-
(c) The charged particle does not speed up in a dee; it maintains a constant speed within the dees because the magnetic field only changes its direction, not its speed.
-
(d) The charged particle does not slow down within a dee; it maintains a constant speed within the dees due to the magnetic field. It speeds up only in the gap between the dees due to the oscillating electric field.
6. A circular current loop of magnetic moment $M$ is in an arbitrary orientation in an external magnetic field $\mathbf{B}$. The work done to rotate the loop by $30^{\circ}$ about an axis perpendicular to its plane is
(a) $M B$ $ \newline $
(b) $\sqrt{3} \frac{M B}{2}$ $ \newline $
(c) $\frac{M B}{2}$ $ \newline $
(d) zero $ \newline $
Show Answer
Thinking Process
The rotation of the loop by $30^{\circ}$ about an axis perpendicular to its plane imply that the axis of the loop still continues to inclined with the same angle with the direction of magnetic field.
Answer
(a) The rotation of the loop by $30^{\circ}$ about an axis perpendicular to its plane make no change in the angle made by axis of the loop with the direction of magnetic field, therefore, the work done to rotate the loop is zero.
Note The work done to rotate the loop in magnetic field $W=M B\left(\cos \theta_{1}-\cos \theta_{2}\right)$. where signs are as usual.
-
Option (b) $\sqrt{3} \frac{M B}{2}$: This option is incorrect because it suggests that there is a non-zero work done to rotate the loop by $30^{\circ}$. However, since the rotation is about an axis perpendicular to the plane of the loop, the angle between the magnetic moment and the magnetic field does not change, resulting in zero work done.
-
Option (c) $\frac{M B}{2}$: This option is incorrect for the same reason as option (b). It implies that there is a non-zero work done, but the angle between the magnetic moment and the magnetic field remains unchanged during the rotation, leading to zero work done.
-
Option (d) zero: This option is actually correct, as explained in the provided answer. The work done to rotate the loop by $30^{\circ}$ about an axis perpendicular to its plane is indeed zero because the angle between the magnetic moment and the magnetic field does not change.
7. The gyro-magnetic ratio of an electron in an $\mathrm{H}$-atom, according to Bohr model, is
(a) independent of which orbit it is in
(b) negative
(c) positive
(d) increases with the quantum number $n$.
Show Answer
Thinking Process
The gyro-magnetic ratio of an electron in an $\mathrm{H}$-atom is equal to the ratio of the magnetic moment and the angular momentum of the electron.
Answer
(a) If $I$ is the magnitude of the angular momentum of the electron about the central nucleus (orbital angular momentum). Vectorially,
$$ \mu_{l}=-\frac{e}{2 m_{e}} l $$
The negative sign indicates that the angular momentum of the electron is opposite in direction to the magnetic moment.
-
(b) negative: The gyro-magnetic ratio is defined as the ratio of the magnetic moment to the angular momentum. While the magnetic moment itself is negative (due to the negative charge of the electron), the gyro-magnetic ratio is a scalar quantity and is positive because it is the magnitude of the ratio of two vectors (magnetic moment and angular momentum).
-
(c) positive: This option is not incorrect. The gyro-magnetic ratio is indeed positive as it is the magnitude of the ratio of the magnetic moment to the angular momentum.
-
(d) increases with the quantum number ( n ): According to the Bohr model, the gyro-magnetic ratio is given by (\frac{e}{2m_e}), which is a constant and does not depend on the quantum number ( n ). Therefore, it does not increase with ( n ).
Multiple Choice Questions (More Than One Options)
8. Consider a wire carrying a steady current, $I$ placed in a uniform magnetic field $\mathbf{B}$ perpendicular to its length. Consider the charges inside the wire. It is known that magnetic forces do no work. This implies that,
(a) motion of charges inside the conductor is unaffected by $\mathbf{B}$, since they do not absorb energy
(b) some charges inside the wire move to the surface as a result of $\mathbf{B}$
(c) If the wire moves under the influence of $\mathbf{B}$, no work is done by the force
(d) if the wire moves under the influence of $\mathbf{B}$, no work is done by the magnetic force on the ions, assumed fixed within the wire.
Show Answer
Answer
$(b, d)$
Magnetic forces on a wire carrying a steady current, $I$ placed in a uniform magnetic field $B$, a wire carrying a steady current, $I$ placed in a uniform magnetic field $\mathbf{B}$ perpendicular to its length is given by
$$ F=I l B $$
The direction of force is given by Fleming’s left hand rule and $F$ is perpendicular to the direction of magnetic field $\mathbf{B}$. Therefore, work done by the magnetic force on the ions is zero.
-
(a) Motion of charges inside the conductor is affected by $\mathbf{B}$ because the magnetic field exerts a force on the moving charges, causing them to experience a Lorentz force perpendicular to both their velocity and the magnetic field. This can lead to a redistribution of charges within the conductor, even though the magnetic force does no work.
-
(c) If the wire moves under the influence of $\mathbf{B}$, work can be done by the force. The magnetic force can cause the wire to move, and if the wire moves in the direction of the force, mechanical work is done. However, this work is not done by the magnetic force directly on the charges but rather through the interaction of the magnetic field with the current in the wire.
9. Two identical current carrying coaxial loops, carry current $I$ in an opposite sense. A simple amperian loop passes through both of them once. Calling the loop as $C$,
(a) $\oint \mathbf{B} \cdot \mathbf{d I}=m \mu_{0} I$
(b) the value of $\oint \mathbf{B} . \mathbf{d I}=\bar{\mp} 2 \mu_{0} I$ is independent of sense of $C$
(c) there may be a point on $C$ where, $\mathbf{B}$ and $\mathbf{d l}$ are perpendicular
(d) B vanishes everywhere on C
Show Answer
Thinking Process
The Ampere’s circuital law is to be applied on given situation.
Answer
( $b, c$ )
Applying the Ampere’s circuital law, we have
$$ \int_{C} \mathrm{~B} \cdot \mathrm{dl}=\mu_{0}(I-I)=0 \quad \text { (because current is in opposite sense.) } $$
Also, there may be a point on $\mathbf{C}$ where $\mathbf{B}$ and $\mathbf{d l}$ are perpendicular and hence,
$$ \int_{c} \mathrm{~B} \cdot \mathrm{dl}=0 $$
-
Option (a) is incorrect: The expression $\oint \mathbf{B} \cdot \mathbf{dI} = m \mu_{0} I$ is incorrect because the correct application of Ampere’s circuital law in this scenario, where the currents are in opposite directions, results in the net current being zero. Therefore, $\oint \mathbf{B} \cdot \mathbf{dI} = \mu_{0}(I - I) = 0$, not $m \mu_{0} I$.
-
Option (d) is incorrect: The statement that $\mathbf{B}$ vanishes everywhere on $C$ is incorrect. While the net integral $\oint \mathbf{B} \cdot \mathbf{dI}$ is zero due to the opposite currents canceling each other out, this does not imply that the magnetic field $\mathbf{B}$ is zero at every point on $C$. The magnetic field can still exist at various points on $C$, but the contributions from the two loops will cancel out when integrated over the entire loop.
10. A cubical region of space is filled with some uniform electric and magnetic fields. An electron enters the cube across one of its faces with velocity $v$ and a positron enters via opposite face with velocity $-v$. At this instant,
(a) the electric forces on both the particles cause identical accelerations
(b) the magnetic forces on both the particles cause equal accelerations
(c) both particles gain or loose energy at the same rate
(d) the motion of the Centre of Mass (CM) is determined by B alone
Show Answer
Thinking Process
The Lorentz force is experienced by the single moving charge in space is filled with some uniform electric and magnetic fields is given by $\mathbf{F}=\mathbf{q} \mathbf{E}+q(\mathbf{v} \times \mathbf{B})$.
Answer
$(b, c, d)$
The magnetic forces $\mathbf{F}=q(\mathbf{v} \times \mathbf{B})$, on charge particle is either zero or $\mathbf{F}$ is perpendicular to $\mathbf{v}$ (or component of $\mathbf{v}$ ) which in turn revolves particles on circular path with uniform speed. In both the cases particles have equal accelerations.
Both the particles gain or loss energy at the same rate as both are subjected to the same electric force $(\mathbf{F}=q \mathbf{E})$ in opposite direction.
Since, there is no change of the Centre of Mass (CM) of the particles, therefore the motion of the Centre of Mass (CM) is determined by $\mathbf{B}$ alone.
-
(a) the electric forces on both the particles cause identical accelerations
This option is incorrect because the electric force on a charged particle is given by (\mathbf{F} = q \mathbf{E}). Since the electron and positron have opposite charges ((q) and (-q)), the electric forces on them will be in opposite directions. Therefore, the accelerations will also be in opposite directions, not identical.
-
(b) the magnetic forces on both the particles cause equal accelerations
This option is correct, so no reason is needed here.
-
(c) both particles gain or lose energy at the same rate
This option is correct, so no reason is needed here.
-
(d) the motion of the Centre of Mass (CM) is determined by B alone
This option is correct, so no reason is needed here.
11. A charged particle would continue to move with a constant velocity in a region wherein,
(a) $\mathbf{E}=0, \mathbf{B} \neq 0$
(b) $\mathbf{E} \neq 0, \mathbf{B} \neq 0$
(c) $\mathbf{E} \neq 0, \mathbf{B}=0$
(d) $\mathbf{E}=0, \mathbf{B}=0$
Show Answer
Thinking Process
The Lorentz force is experienced by the single moving charge in space is filled with some uniform electric and magnetic fields is given by $\mathbf{F}=q \mathbf{E}+q(\mathbf{v} \times \mathbf{B})$.
Answer
$(a, b, d)$
Here, force on charged particle due to electric field $\mathrm{F}_{\mathrm{E}}=q \mathrm{E}$.
Force on charged particle due to magnetic field, $\mathbf{F}_{m}=q(\mathbf{v} \times \mathbf{B})$
Now, $F_{E}=0$ if $E=0$ and $F_{m}=0$ if $\sin \theta=0$ or $\theta^{\circ}=0^{\circ}$ or $180^{\circ}$
Hence, $B \neq 0$.
Also, $E=0$ and $B=0$ and the resultant force $q \mathbf{E}+q(\mathbf{v} \times \mathbf{B})=0$. In this case $E \neq 0$ and $B \neq 0$
- For option (c) $\mathbf{E} \neq 0, \mathbf{B}=0$: The presence of a non-zero electric field $\mathbf{E}$ would exert a force $\mathbf{F}_{\mathbf{E}} = q\mathbf{E}$ on the charged particle, causing it to accelerate rather than move with a constant velocity.
Very Short Answer Type Questions
12. Verify that the cyclotron frequency $\omega=e B / m$ has the correct dimensions of $[\mathrm{T}]^{-1}$.
Show Answer
Answer
For a charge particle moving perpendicular to the magnetic field, the magnetic Lorentz forces provides necessary centripetal force for revolution.
$$ \frac{m v^{2}}{R}=q v B $$
On simplifying the terms, we have
$$ \therefore \quad \frac{q B}{m}=\frac{v}{R}=\omega $$
Finding the dimensional formula of angular frequency
$$ \begin{array}{ll} \therefore & {[\omega]=\frac{q B}{m}=\frac{v}{R}=[T]^{-1}} \end{array} $$
13. Show that a force that does no work must be a velocity dependent force.
Show Answer
Answer
Let no work is done by a force, so we have
$$ \begin{array}{rlrl} \mathrm{d} \mathbf{W}=\mathbf{F} \cdot \mathbf{d l}=0 \ \Rightarrow & \text { F.v } d t & =0 \ \Rightarrow & \text { F.v } & =0 & \end{array} $$
Thus, $F$ must be velocity dependent which implies that angle between $F$ and $v$ is $90^{\circ}$. If $v$ changes (direction), then (directions) $F$ should also change so that above condition is satisfied,
14. The magnetic force depends on $v$ which depends on the inertial frame of reference. Does then the magnetic force differ from inertial frame to frame? Is it reasonable that the net acceleration has a different value in different frames of reference?
Show Answer
Answer
Yes, the magnetic force differ from inertial frame to frame. The magnetic force is frame dependent.
The net acceleration which comes into existing out of this is however, frame independent (non -relativistic physics) for inertial frames.
15. Describe the motion of a charged particle in a cyclotron if the frequency of the radio frequency ( $\mathrm{rf}$ ) field were doubled.
Show Answer
Thinking Process
The relationship of radio frequency and charge particle frequency must be equal in order to accelerate the charge particle between the dees in cyclotron.
Answer
Here, the condition of magnetic resonance is violated.
When the frequency of the radio frequency (rf) field were doubled, the time period of the radio frequency ( $\mathrm{rf}$ ) field were halved. Therefore, the duration in which particle completes half revolution inside the dees, radio frequency completes the cycle.
Hence, particle will accelerate and decelerate alternatively. So, the radius of path in the dees will remain same.
16. Two long wires carrying current $I_{1}$ and $I_{2}$ are arranged as shown in figure. The one carrying current $I_{1}$ is along is the $x$-axis. The other carrying current $I_{2}$ is along a line parallel to the $y$-axis given by $x=0$ and $z=d$. Find the force exerted at $\mathrm{O}_{2}$ because of the wire along the $x$-axis.
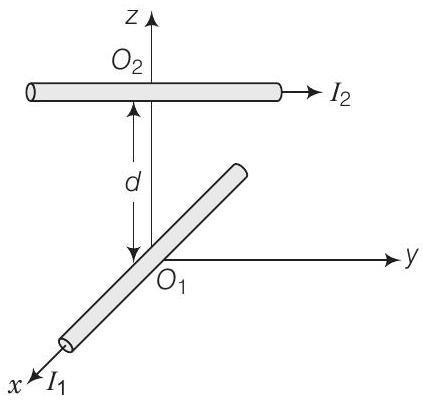
Show Answer
Thinking Process
Here, the understanding of application of the rule of finding directions of magnetic field and magnetic force on current carrying wire placed in magnetic field is beautifully tested.
Answer
In Biot- Savart law, magnetic field $\mathbf{B}$ is parallel to $i \mathrm{dl} \times \mathbf{r}$ and $i \mathbf{d l}$ have its direction along the direction of flow of current.
$\text {Here, for the direction of magnetic field, At due to wire carrying $I_{1}$ current is}$
$$ \text { B } | \text { parallel } i \text { dl } \times r \text { or } \hat{\mathbf{i}} \times \hat{\mathbf{k}} \text {, but } \hat{\mathbf{i}} \times \hat{\mathbf{k}}=-\hat{\mathbf{j}} $$
So, the direction at $\mathrm{O}_{2}$ is along $Y$ - direction.
The direction of magnetic force exerted at $\mathrm{O}_{2}$ because of the wire along the, $x$-axis.
$$ \mathrm{F}=I l \times \mathrm{B} \approx \hat{\mathbf{j}} \times(-\hat{\mathbf{j}})=0 $$
So, the magnetic field due to $l_{1}$ is along the $y$-axis. The second wire is along the $y$-axis and hence, the force is zero.
Short Answer Type Questions
17. A current carrying loop consists of 3 identical quarter circles of radius $R$, lying in the positive quadrants of the $x-y, y-z$ and $z-x$ planes with their centres at the origin, joined together. Find the direction and magnitude of $\mathbf{B}$ at the origin.
Show Answer
Thinking Process
The magnetic field due to arc of current carrying coil which subtends an angle $\theta$ at centre is given by $\mathbf{B}=\frac{\mu_{0}}{4 \pi} \frac{I \theta}{R}$.
Answer
For the current carrying loop quarter circles of radius $R$, lying in the positive quadrants of the $x$-y plane
$$ B_1=\frac{\mu_{0}}{4 \pi} \frac{I(\pi / 2)}{R} \hat{k}=\frac{\mu_{0}}{4} \frac{I}{2 R} \hat{{k}} $$
For the current carrying loop quarter circles of radius $R$, lying in the positive quadrants of the $y-z$ plane
$$ B_2=\frac{0}{4} \frac{I}{2R} \hat{i} $$
For the current carrying loop quarter circles of radius $R$, lying in the positive quadrants of the $z-x$ plane
$$ B_{3}=\frac{\mu_{0}}{4} \frac{I}{2 R} \hat{i} $$
Current carrying loop consists of 3 identical quarter circles of radius $R$, lying in the positive quadrants of the $x-y, y-y$ and $z-z$ planes with their centres at the origin, joined together is equal to the vector sum of magnetic field due to each quarter and given by
$$ \mathbf{B}=\frac{1}{4 \pi}(\hat{\mathbf{i}}+\hat{\mathbf{j}}+\hat{\mathbf{k}}) \frac{\mu_{0} I}{2 R} . $$
18. A charged particle of charge $e$ and mass $m$ is moving in an electric field $\mathbf{E}$ and magnetic field $\mathbf{B}$. Construct dimensionless quantities and quantities of dimension $[T]^{-1}$.
Show Answer
Answer
No dimensionless quantity can be constructed using given quantities.
For a charge particle moving perpendicular to the magnetic field, the magnetic Lorentz forces provides necessary centripetal force for revolution.
$$ \frac{m v^{2}}{R}=q v B $$
On simplifying the terms, we have
$$ \therefore \quad \frac{q B}{m}=\frac{v}{R}=\omega $$
Finding the dimensional formula of angular frequency
$$ \begin{array}{ll} \therefore & {[\omega]=\frac{q B}{m}=\frac{v}{R}=[T]^{-1}} \end{array} $$
This is the required expression.
19. An electron enters with a velocity $\mathbf{v}=v_{0} \hat{\mathbf{i}}$ into a cubical region (faces parallel to coordinate planes) in which there are uniform electric and magnetic fields. The orbit of the electron is found to spiral down inside the cube in plane parallel to the $x-y$ plane. Suggest a configuration of fields $\mathbf{E}$ and $\mathbf{B}$ that can lead to it.
Show Answer
Thinking Process
The magnetic field revolves the charge particle in uniform circular motion in $x-y$ plane and electric field along $x$-direction increases the speed, which in turn increases the radius of circular path and hence, particle traversed on spiral path.
Answer
Considering magnetic field $\mathbf{B}=B_{0} \hat{\mathbf{k}}$, and an electron enters with a velocity $\mathbf{v}=v_{0} \hat{\mathbf{i}}$ into a cubical region (faces parallel to coordinate planes).
The force on electron, using magnetic Lorentz force, is given by
$$ F=-e\left(v_{0} \hat{i} \times B_{0} \hat{k}\right)=e v_{0} B_{0} \hat{i} $$
which revolves the electron in $x$ - $y$ plane.
The electric force $\mathbf{F}=-\mathbf{e} \mathrm{E}_{0} \hat{\mathbf{k}}$ accelerates $e$ along $z$-axis which in turn increases the radius of circular path and hence particle traversed on spiral path.
20. Do magnetic forces obey Newton’s third law. Verify for two current elements $dl_1=dl \hat{i}$ located at the origin and $dl_2=dl \hat{j}$ located at $(0, R, 0)$. Both carry current $I$.
Show Answer
Thinking Process
Here, the understanding of application of the rules of finding directions of magnetic field and magnetic force on current carrying wire placed in magnetic field is needed.
Answer
In Biot-Savart’s law, magnetic field $\mathbf{B}$ is parallel (II) to $i \mathbf{d l} \times \mathbf{r}$ and $i d$ have its direction along the direction of flow of current.
Here, for the direction of magnetic field, At $dl_2$, located at $(0, R, 0)$ due to wire $d_1$ is given by $B | i dl \times r$ or $ \hat {i} \times \hat{j} $ (because point $(0, R, 0)$ lies on $y$-axis), but $\hat{i} \times \hat{j}=\hat{k}$
So, the direction of magnetic field at $d_{2}$ is along $z$-direction.
The direction of magnetic force exerted at $d_{2}$ because of the first wire along the $x$-axis.
$F=i(I \times B)$ i.e., $F |(i \times k)$ or along $-\hat{\mathbf{j}}$ direction.
Therefore, force due to $dl_1$ on $dl_2$ is non-zero.
Now, for the direction of magnetic field, At $d_{1}$, located at $(0,0,0)$ due to wire $d_{2}$ is given by $B | i \mathbf{d l} \times \mathbf{r}$ or $\hat{\mathbf{j}} \times \hat{\mathbf{j}}$ (because origin lies on $y$-direction w.r.t. point $(0, R, 0)$.), but $\mathbf{j} \times \mathbf{j}=0$.
So, the magnetic field at $d_{1}$ does not exist.
Force due to $dl_2$ on $dl_1$ is zero.
So, magnetic forces do not obey Newton’s third law.
21. A multirange voltmeter can be constructed by using a galvanometer circuit as shown in figure. We want to construct a voltmeter that can measure $2 \mathrm{~V}, 20 \mathrm{~V}$ and $200 \mathrm{~V}$ using a galvanometer of resistance $10 \Omega$ and that produces maximum deflection for current of $1 \mathrm{~mA}$. Find $R_{1}, R_{2}$ and $R_{3}$ that have to be used.
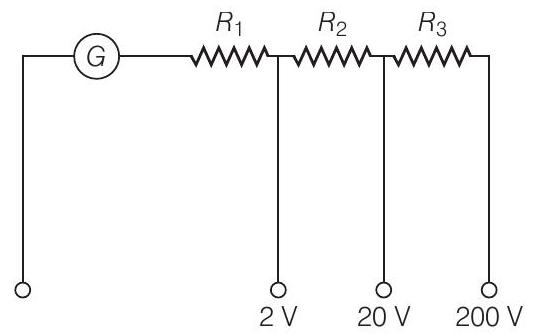
Show Answer
Thinking Process
A galvanometer can be converted into voltmeter by connecting a very high resistance wire connected in series with galvanometer. The relationship is given by $I_{g}(G+R)=V$ where $I_{g}$ is range of galvanometer, $G$ is resistance of galvanometer and $R$ is resistance of wire connected in series with galvanometer.
Answer
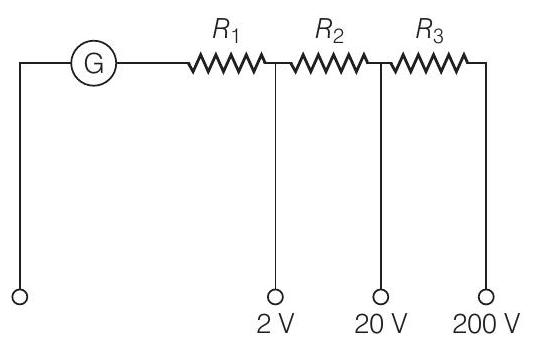
Applying expression in different situations
$$ \begin{array}{lll} \text { For } & i_{G}\left(G+R_{1}\right)=2 & \text { for 2V range } \ \text { For } & i_{G}\left(G+R_{1}+R_{2}\right)=20 & \text { for 20V range } \ \text { and For } & i_{G}\left(G+R_{1}+R_{2}+R_{3}\right)=200 & \text { for 200V range } \end{array} $$
On solving, we get $R_{1}=1990 \Omega, R_{2}=18 \mathrm{k} \Omega$ and $R_{3}=180 \mathrm{k} \Omega$.
22. A long straight wire carrying current of $25 \mathrm{~A}$ rests on a table as shown in figure. Another wire $P Q$ of length $1 \mathrm{~m}$, mass $2.5 \mathrm{~g}$ carries the same current but in the opposite direction. The wire $P Q$ is free to slide up and down. To what height will $P Q$ rise?
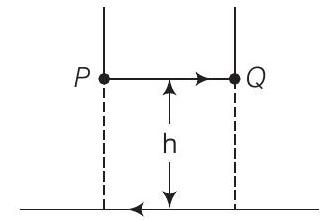
Show Answer
Thinking Process
The force applied on PQ by long straight wire carrying current of 25A rests on a table must balance the weight of small current carrying wire.
Answer
The magnetic field produced by long straight wire carrying current of $25 \mathrm{~A}$ rests on a table on small wire
$$ B=\frac{\mu_{0} I}{2 \pi h} $$
The magnetic force on small conductor is
$$ F=B I l \sin \theta=B I l $$
Force applied on $P Q$ balance the weight of small current carrying wire.
$$ \begin{aligned} F & =m g=\frac{\mu_{0} I^{2} l}{2 \pi h} \ h & =\frac{\mu_{0} I^{2} l}{2 \pi m g}=\frac{4 \pi \times 10^{-7} \times 25 \times 25 \times 1}{2 \pi \times 2.5 \times 10^{-3} \times 9.8}=51 \times 10^{-4} \ h & =0.51 \mathrm{~cm} \end{aligned} $$
Long Answer Type Questions
23. A 100 turn rectangular coil $A B C D$ (in $X-Y$ plane) is hung from one arm of a balance figure. A mass $500 \mathrm{~g}$ is added to the other arm to balance the weight of the coil. A current $4.9 \mathrm{~A}$ passes through the coil and a constant magnetic field of $0.2 \mathrm{~T}$ acting inward (in $x-z$ plane) is switched on such that only arm $C D$ of length $1 \mathrm{~cm}$ lies in the field. How much additional mass $m$ must be added to regain the balance?
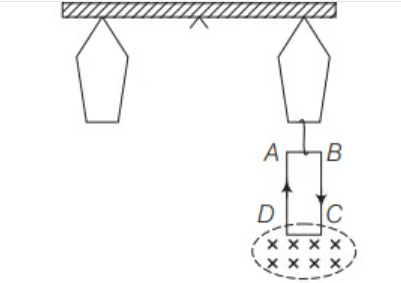
Show Answer
Thinking Process
The magnetic force applied on CD by magnetic field must balance the weight.
Answer
For equilibrium/ balance, net torque should also be equal to zero.
When the field is off $\Sigma t=0$ considering the separation of each hung from mid-point be $I$.
$$ \begin{aligned} M g l & =W_{\text {coil }} l \newline \ 500 g l & =W_{\text {coil }} l \newline \ W_{\text {coil }} & =500 \times 9.8 \mathrm{~N} \end{aligned} $$
Taking moment of force about mid-point, we have the weight of coil When the magnetic field is switched on
$$ \begin{aligned} M g l+m g l & =W_{\text {coil }} l+I B L \sin 90^{\circ} I \newline \ m g l & =B I L l \newline \ m & =\frac{B I L}{g}=\frac{0.2 \times 4.9 \times 1 \times 10^{-2}}{9.8}=10^{-3} \mathrm{~kg}=1 \mathrm{~g} \end{aligned} $$
Thus, $1 \mathrm{~g}$ of additional mass must be added to regain the balance.
24. A rectangular conducting loop consists of two wires on two opposite sides of length $l$ joined together by rods of length $d$. The wires are eachof the same material but with cross-sections differing by a factor of 2. The thicker wire has a resistance $R$ and the rods are of low resistance, which in turn are connected to a constant voltage source $V_{0}$. The loop is placed in uniform a magnetic field $\mathbf{B}$ at $45^{\circ}$ to its plane. Find $\tau$, the torque exerted by the magnetic field on the loop about an axis through the centres of rods.
Show Answer
Thinking Process
After finding current in both wires, magnetic forces and torques need to be calculated for finding the net torque.
Answer

Front view
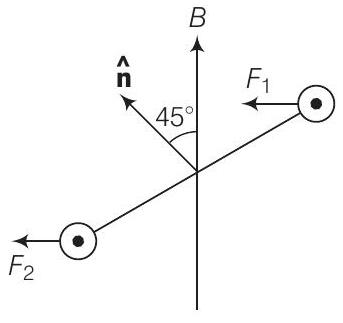
Side view
The thicker wire has a resistance $R$, then the other wire has a resistance $2 R$ as the wires are of the same material but with cross-sections differing by a factor 2 .
Now, the force and hence, torque on first wire is given by
$$ F_{1}=i_{1} l B=\frac{V_{0}}{2 R} l B, \tau_{1}=\frac{d}{2 \sqrt{2}} F_{1}=\frac{V_{0} l d B}{2 \sqrt{2} R} $$
Similarly, the force hence torque on other wire is given by
$$ F_{2}=i_{2} l B=\frac{V_{0}}{2 R} l B, \tau_{2}=\frac{d}{2 \sqrt{2}} F_{2}=\frac{V_{0} l d B}{4 \sqrt{2} R} $$
So, net torque, $\quad \tau=\tau_{1}-\tau_{2}$
$$ \tau=\frac{1}{4 \sqrt{2}} \frac{V_{0} l d B}{R} $$
25. An electron and a positron are released from $(0,0,0)$ and $(0,0,1.5 R)$ respectively, in a uniform magnetic field $\mathbf{B}=B_{0} \hat{\mathbf{i}}$, each with an equal momentum of magnitude $p=e B R$. Under what conditions on the direction of momentum will the orbits be non-intersecting circles?
Show Answer
Thinking Process
The circles of the electron and a positron shall not overlap if the distance between the two centers are greater than $2 R$
Answer
Since, $B$ is along the $x$-axis, for a circular orbit the momenta of the two particles are in the $y-z$ plane. Let $p_{1}$ and $p_{2}$ be the momentum of the electron and positron, respectively. Both traverse a circle of radius $R$ of opposite sense. Let $p_{1}$ make an angle $\theta$ with the $y$-axis $p_{2}$ must make the same angle.
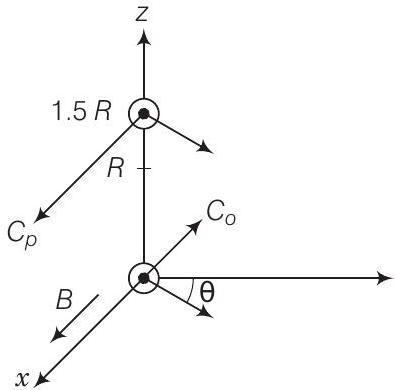
The centres of the respective circles must be perpendicular to the momenta and at a distance $R$. Let the centre of the electron be at $C_{e}$ and of the positron at $C_{p}$. The coordinates of $C_{e}$ is
$$ C_{e} \equiv(0,-R \sin \theta, R \cos \theta) $$
The coordinates of $C_{p}$ is
$$ C_{p} \equiv\left(0,-R \sin \theta, \frac{3}{2} R-R \cos \theta\right) $$
The circles of the two shall not overlap if the distance between the two centers are greater than $2 R$.
Let $d$ be the distance between $C_{p}$ and $C_{e}$.
Let $d$ be the distance between $C_{p}$ and $C_{e}$
Then,
$$ \begin{aligned} d^{2} & =(2 R \sin \theta)^{2}+\frac{3}{2} R-2 R \cos \theta \newline \ & =4 R^{2} \sin ^{2} \theta+\frac{9^{2}}{4} R-6 R^{2} \cos \theta+4 R^{2} \cos ^{2} \theta \newline \ & =4 R^{2}+\frac{9}{4} R^{2}-6 R^{2} \cos \theta \end{aligned} $$
Since, $d$ has to be greater than $2 R$
$$ \begin{array}{rlrl} d^{2} >4 R^{2} \newline \ \Rightarrow 4 R^{2}+\frac{9}{4} R^{2}-6 R^{2} \cos \theta >4 R^{2} \newline \ \Rightarrow \frac{9}{4} >6 \cos \theta \newline \ \text { or, } \cos \theta <\frac{3}{8} \end{array} $$
26. A uniform conducting wire of length $12 a$ and resistance $R$ is wound up as a current carrying coil in the shape of (i) an equilateral triangle of side $a$, (ii) a square of sides $a$ and, (iii) a regular hexagon of sides $a$. The coil is connected to a voltage source $V_{0}$. Find the magnetic moment of the coils in each case.
Show Answer
Thinking Process
The different shapes forms figures of different area and hence, there magnetic moments varies.
Answer
We know that magnetic moment of the coils $m=n / A$.
Since, the same wire is used in three cases with same potentials, therefore, same current flows in three cases.
(i) for an equilateral triangle of side $a$,
$n=4$ as the total wire of length $=12 a$

Magnetic moment of the coils $m=n I A=4 I \quad \frac{\sqrt{3}}{4} a^{2}$
$\therefore \quad m=I a^{2} \sqrt{3}$
(ii) For a square of sides a,

$$ A=a^{2} $$
$n=3$ as the total wire of length $=12 a$
Magnetic moment of the coils $m=n I A=3 I\left(a^{2}\right)=3 I a^{2}$
(iii) For a regular hexagon of sides a,
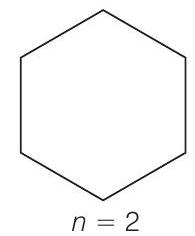
$n=2$ as the total wire of length $=12 a$
Magnetic moment of the coils $m=n \left\lvert, A=21 \frac{6 \sqrt{3}}{4} a^{2}\right.$
$$ m=3 \sqrt{3} a^{2} I $$
$m$ is in a geometric series.
27. Consider a circular current-carrying loop of radius $R$ in the $x$-yplane with centre at origin. Consider the line integral
$$ J(L)=\left|\int_{\mathcal{L}}^{t} B \cdot d l\right| $$
taken along $z$-axis.
(a) Show that $\mathfrak{I}(L)$ monotonically increases with $L$
(b) Use an appropriate amperian loop to show that $\mathfrak{I}(\infty)=\mu_{0} I$. where $I$ is the current in the wire
(c) Verify directly the above result
(d) Suppose we replace the circular coil by a square coil of sides $R$ carrying the same current $I$.
What can you say about $\mathfrak{I}(L)$ and $\mathfrak{I}(\infty)$ ?
Show Answer
Thinking Process
This question revolves around the application of Ampere circuital law.
Answer
(a) B (z) points in the same direction on $z$-axis and hence, $J(L)$ is a monotonically function of $L$.
Since, $B$ and $\mathbf{d l}$ along the same direction, therefore $\mathbf{B} \cdot \mathbf{d l}=\mathbf{B} \cdot \mathbf{d l}$ as $\cos 0=1$
(b) $J(L)+$ contribution from large distance on contour $C=\mu_{0} I$
$\therefore \quad$ as $L \rightarrow \infty$
Contribution from large distance $\rightarrow 0\left(\right.$ as $\left.B \propto 1 / r^{3}\right)$
$$ J(\infty)-\mu_{0} I $$
(c) The magnetic field due to circular current-carrying loop of radius $R$ in the $x$-y plane with centre at origin at any point lying at a distance of from origin.
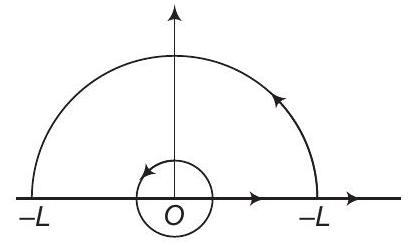
$$ \begin{aligned} B_{z} & =\frac{\mu_{0} I R^{2}}{2\left(z^{2}+R^{2}\right)^{3 / 2}} \ \int_{-\infty}^{\infty} B_{z} d z & =\int_{-\infty}^{\infty} \frac{\mu_{0} / R^{2}}{2\left(z^{2}+R^{2}\right)^{3 / 2}} d z \end{aligned} $$
Put $\quad z=R \tan \theta_{1}$
$\Rightarrow \quad d z=R \sec ^{2} \theta d \theta$
$\therefore \quad \int_{-\infty}^{\infty} B_{z} d z=\frac{\mu_{0} I}{2} \int_{-\pi / 2}^{\pi / 2} \cos \theta d \theta=\mu_{0} I$
(d)$Bz_{\text {square}}<Bz_{\text {circular coil }}$
$\therefore \quad IL_{\text {square }}< IL_{\text {circular coil }}$
But by using arguments as in (b)
$$ I\infty_{\text {square }}=I\infty_{\text {circular }} $$
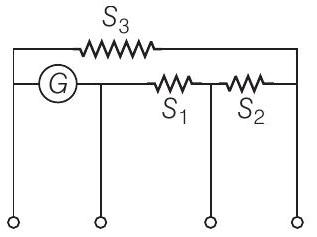
28. A multirange current meter can be constructed by using a galvanometer circuit as shown in figure. We want a current meter that can measure $10 \mathrm{~mA}, 100 \mathrm{~mA}$ and $1 \mathrm{~mA}$ using a galvanometer of resistance $10 \Omega$ and that produces maximum deflection for current of $1 \mathrm{~mA}$. Find $S_{1}, S_{2}$ and $S_{3}$ that have to be used.
Show Answer
Thinking Process
A galvanometer can be converted into ammeter by connecting a very low resistance wire (shunt S) connected in parallel with galvanometer. The relationship is given by $I_{g} G=\left(I-I_{g}\right)$ S, where $I_{g}$ is range of galvanometer, $G$ is resistance of galvanometer.
Answer
$$ \begin{aligned} I_{G} \cdot G & =\left(I_{1}-I_{G}\right)\left(S_{1}+S_{2}+S_{3}\right) \text { for } I_{1}=10 \mathrm{~mA} \newline \ I_{G}\left(G+S_{1}\right) & =\left(I_{2}-I_{G}\right)\left(S_{2}+S_{3}\right) \text { for } I_{2}=100 \mathrm{~mA} \newline \ I_{G}\left(G+S_{1}+S_{2}\right) & =\left(I_{3}-I_{G}\right)\left(S_{3}\right) \text { for } I_{3}=1 \mathrm{~A} \newline \ S_{1} & =1 \mathrm{~W}, S_{2}=0.1 \mathrm{~W} \newline \ S_{3} & =0.01 \mathrm{~W} \end{aligned} $$
29. Five long wires $A, B, C, D$ and $E$, each carrying current $I$ are arranged to form edges of a pentagonal prism as shown in figure. Each carries current out of the plane of paper.
(a) What will be magnetic induction at a point on the axis 0 ? Axis is at a distance $R$ from each wire.
(b) What will be the field if current in one of the wires (say $A$ ) is switched off?
(c) What if current in one of the wire (say $A$ ) is reversed?

Show Answer
Thinking Process
The vector sum of magnetic field produced by each wire at $O$ is equal to 0.
Answer
(a) Suppose the five wires $A, B, C, D$ and $E$ be perpendicular to the plane of paper at locations as shown in figure.
Thus, magnetic field induction due to five wires will be represented by various sides of a closed pentagon in one order, lying in the plane of paper. So, its value is zero.
(b) Since, the vector sum of magnetic field produced by each wire at $O$ is equal to 0 . Therefore, magnetic induction produced by one current carrying wire is equal in magnitude of resultant of four wires and opposite in direction.
Therefore, the field if current in one of the wires (say $A$ ) is switched off is $\frac{\mu_{0}}{2 \pi} \frac{i}{R}$ perpendicular to $A O$ towards left.
(c) If current in wire $A$ is reversed, then
total magnetic field induction at $O$
$=$ Magnetic field induction due to wire $A+$ magnetic field induction due to wires $B, C, D$ and $E$
$$ =\frac{\mu_{0}}{4 \pi R} \frac{2 I}{R} $$
(acting perpendicular to $A O$ towards left) $+\frac{\mu_{0}}{\pi} \frac{2 I}{R}$ (acting perpendicular $A O$ towards left) $=\frac{\mu_{0} I}{\pi R}$ acting perpendicular $A O$ towards left.










