Communication System
Multiple Choice Questions (MCQs)
1. Three waves $A, B$ and $C$ of frequencies $1600 kHz, 5 MHz$ and $60 MHz$, respectively are to be transmitted from one place to another. Which of the following is the most appropriate mode of communication?
(a) $A$ is transmitted via space wave while $B$ and $C$ are transmitted via sky wave
(b) $A$ is transmitted via ground wave, $B$ via sky wave and $C$ via space wave
(c) $B$ and $C$ are transmitted via ground wave while $A$ is transmitted via sky wave
(d) $B$ is transmitted via ground wave while $A$ and $C$ are transmitted via space wave
Show Answer
Thinking Process
Mode of communication depend on the frequencies of a wave.
Answer
(b) Mode of communication frequency range
Ground wave propagation $-530 kHz$ to $1710 kHz$
Sky wave propagation $-1710 kHz$ to $40 MHz$
Space wave propagation $-54 MHz$ to $4.2 GHz$
-
Option (a) is incorrect:
- Wave $A$ (1600 kHz) falls within the ground wave propagation range (530 kHz to 1710 kHz), not the space wave range.
- Wave $C$ (60 MHz) falls within the space wave propagation range (54 MHz to 4.2 GHz), not the sky wave range.
-
Option (c) is incorrect:
- Wave $B$ (5 MHz) falls within the sky wave propagation range (1710 kHz to 40 MHz), not the ground wave range.
- Wave $C$ (60 MHz) falls within the space wave propagation range (54 MHz to 4.2 GHz), not the ground wave range.
- Wave $A$ (1600 kHz) falls within the ground wave propagation range (530 kHz to 1710 kHz), not the sky wave range.
-
Option (d) is incorrect:
- Wave $B$ (5 MHz) falls within the sky wave propagation range (1710 kHz to 40 MHz), not the ground wave range.
- Wave $A$ (1600 kHz) falls within the ground wave propagation range (530 kHz to 1710 kHz), not the space wave range.
2. A $100 m$ long antenna is mounted on a $500 m$ tall building. The complex can become a transmission tower for waves with $\lambda$ $\newline$
(a) $\sim 400 m$ $\newline$
(b) $\sim 25 m$ $\newline$
(c) $\sim 150 m$ $\newline$
(d) $\sim 2400 m$ $\newline$
Show Answer
Answer
(a) Given, length of the building $(l)$ is given by
$$ l=500 m $$
we know that, wavelength of the wave which can be transmitted by
$$ \lambda \sim 4 l=4 \times 100=400 m $$
-
Option (b) $\sim 25 m$: The wavelength $\lambda \sim 25 m$ is much shorter than the length of the antenna (100 m). For efficient transmission, the wavelength should be comparable to or longer than the length of the antenna.
-
Option (c) $\sim 150 m$: The wavelength $\lambda \sim 150 m$ is not an integer multiple of the length of the antenna (100 m). Efficient transmission typically requires the wavelength to be an integer multiple of the antenna length.
-
Option (d) $\sim 2400 m$: The wavelength $\lambda \sim 2400 m$ is much longer than the length of the antenna (100 m). While longer wavelengths can sometimes be transmitted, they are not optimal for an antenna of this length.
3. A $1 kW$ signal is transmitted using a communication channel which provides attenuation at the rate of $-2 dB$ per $km$. If the communication channel has a total length of $5 km$, the power of the signal received is
[gain in $dB=10 \log \frac{P_0}{P_{j}}$ ] $\newline$
(a) $900 W$ $\newline$
(b) $100 W$ $\newline$
(c) $990 W$ $\newline$
(d) $1010 W$ $\newline$
Show Answer
Answer
(b) Given, power of signal transmitted is given $P_{i}=1 kW=1000 W$
Rate of attenuation of signal $=-2 dB / km$
Length of total path $=5 km$
Thus,
$$ \text { gain in } d B=5 \times(-2)=-10 dB $$
Also,
$$ \text { gain in } d B=10 \log \frac{P_0}{P_{i}} $$
Here $P_0$ is the power of the received signal.
Putting the given values in Eq. (i),
$$ -10=10 \log \frac{P_0}{P_{i}}=-10 \log \frac{P_{i}}{P_0} \newline \Rightarrow \log \frac{P_{i}}{P_0}=1 \Rightarrow \log \frac{P_{i}}{P_0}=\log 10 \newline \Rightarrow \frac{P_{i}}{P_0}=10 \Rightarrow 1000 W=10 P_0 \newline \Rightarrow P_0=100 W $$
-
Option (a) $900 W$: This option is incorrect because the attenuation of the signal over the 5 km distance results in a significant reduction in power, not just a small reduction to 900 W. The calculation shows that the power received is much lower, specifically 100 W.
-
Option (c) $990 W$: This option is incorrect because it implies an almost negligible attenuation over the 5 km distance. Given the attenuation rate of -2 dB per km, the power loss is substantial, leading to a received power of 100 W, not 990 W.
-
Option (d) $1010 W$: This option is incorrect because it suggests that the received power is greater than the transmitted power, which is not possible given the attenuation in the channel. The correct calculation shows that the received power is 100 W, not 1010 W.
4. A speech signal of $3 kHz$ is used to modulate a carrier signal of frequency $1 MHz$, using amplitude modulation. The frequencies of the side bands will be $\newline$
(a) $1.003 MHz$ and $0.997 MHz$ $\newline$
(b) $3001 kHz$ and $2997 kHz$ $\newline$
(c) $1003 kHz$ and $1000 kHz$ $\newline$
(d) $1 MHz$ and $0.997 MHz$ $\newline$
Show Answer
Thinking Process
The amplitude modulated signal consists of the carrier wave of frequency $\omega_{c}$ with two additional sinusoidal waves, one of frequency $(\omega_{c}-\omega_{m})$ and other of frequency $(\omega_{c}+\omega_{m})$. These two waves are called side bands and their frequencies are called side band frequency.
Answer
(a) Given, frequency of carrier signal is $\omega_{c}=1 MHz$
and $\quad$ frequency of speech signal $=3 kHz$
$$ \begin{aligned} & =3 \times 10^{-3} MHz \\ & =0.003 MHz \end{aligned} $$
Now, we know that,
Frequencies of side bands $=(\omega_{c} \pm \omega_{m})$
$$ =(1 \pm 0.003) $$
$$ =1.003 MHz \text { and } 0.997 MHz $$
-
Option (b): The frequencies $3001 kHz$ and $2997 kHz$ are incorrect because they are not in the correct units. The correct sideband frequencies should be in MHz, not kHz. Additionally, the values should be $1.003 MHz$ and $0.997 MHz$, not $3001 kHz$ and $2997 kHz$.
-
Option (c): The frequencies $1003 kHz$ and $1000 kHz$ are incorrect because they do not represent the correct sideband frequencies. The correct sideband frequencies are $1.003 MHz$ and $0.997 MHz$. The given values in this option do not match the calculated sideband frequencies.
-
Option (d): The frequencies $1 MHz$ and $0.997 MHz$ are incorrect because one of the sideband frequencies is missing. The correct sideband frequencies are $1.003 MHz$ and $0.997 MHz$. This option only includes one correct sideband frequency and omits the other.
5. A mesage signal of frequency $\omega_{m}$ is superposed on a carrier wave of frequency $\omega_{c}$ to get an Amplitude Modulated Wave (AM). The frequency of the AM wave will be $\newline$
(a) $\omega_{m}$ $\newline$
(b) $\omega_{c}$ $\newline$
(c) $\frac{\omega_{c}+\omega_{m}}{2}$ $\newline$
(d) $\frac{\omega_{c}-\omega_{m}}{2}$ $\newline$
Show Answer
Thinking Process
In amplitude modulation, the frequency of modulated wave is equal to the frequency of carrier wave.
Answer
(b) Here, according to the question, frequency of carrier wave is $\omega_{c}$.
Thus the amplitude modulated wave also has frequency $\omega_{c}$.
-
Option (a) $\omega_{m}$: This option is incorrect because $\omega_{m}$ represents the frequency of the message signal, not the frequency of the amplitude modulated (AM) wave. The AM wave’s frequency is primarily determined by the carrier wave’s frequency, not the message signal’s frequency.
-
Option (c) $\frac{\omega_{c}+\omega_{m}}{2}$: This option is incorrect because the frequency of the AM wave is not the average of the carrier and message signal frequencies. The AM wave consists of the carrier frequency $\omega_{c}$ and sidebands at frequencies $\omega_{c} \pm \omega_{m}$, but the central frequency remains $\omega_{c}$.
-
Option (d) $\frac{\omega_{c}-\omega_{m}}{2}$: This option is incorrect because the frequency of the AM wave is not determined by the difference between the carrier and message signal frequencies divided by 2. The AM wave’s frequency is centered around the carrier frequency $\omega_{c}$, with sidebands at $\omega_{c} \pm \omega_{m}$.
6. I-V characteristics of four devices are shown in figure.
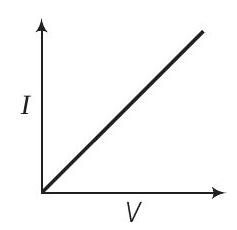
(i)
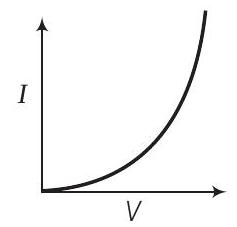
(ii)
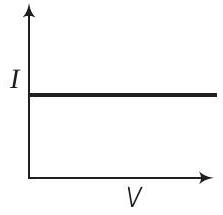
(iii)
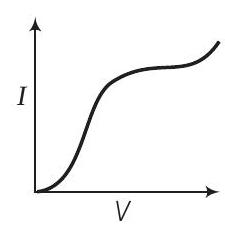
(iv)
Identify devices that can be used for modulation
(a) (i) and (iii)
(b) only (iii)
(c) (ii) and some regions of (iv)
(d) All the devices can be used
Show Answer
Thinking Process
A square law device is something where either current or voltage depends on the square of the other.
Answer
(c) The device which follows square law is used for modulation purpose. Characteristics shown by (i) and (iii) corresponds to linear devices.
Characteristics shown by (ii) corresponds to square law device. Some part of (i) also follow square law.
Hence, (ii) and (iv) can be used for modulation.
-
Option (a) (i) and (iii): This option is incorrect because devices (i) and (iii) correspond to linear devices, which do not follow the square law. Modulation requires devices that follow the square law.
-
Option (b) only (iii): This option is incorrect because device (iii) is a linear device and does not follow the square law. Modulation requires devices that follow the square law.
-
Option (d) All the devices can be used: This option is incorrect because not all the devices follow the square law. Devices (i) and (iii) are linear devices and cannot be used for modulation. Only devices (ii) and some regions of (iv) follow the square law and can be used for modulation.
7. A mae voice after modulation-transmission sounds like that of a female to the receiver. The problem is due to
(a) poor selection of modulation index (selected $0<m<1$ )
(b) poor bandwidth selection of amplifiers
(c) poor selection of carrier frequency
(d) loss of energy in transmission.
Show Answer
Thinking Process
The frequency of male voice less than that of a female voice.
Answer
(b) Here, in this question, the frequency of modulated signal received becomes more, which is possible with the poor bandwidth selection of amplifiers.
This happens because bandwidth in amplitude modulation is equal to twice the frequency of modulating signal.
But, the frequency of male voice is less than that of a female.
-
(a) poor selection of modulation index (selected $0<m<1$): The modulation index primarily affects the amplitude of the modulated signal rather than its frequency. A modulation index in the range $0<m<1$ indicates under-modulation, which would result in a weaker signal but not necessarily a change in the perceived frequency of the voice.
-
(c) poor selection of carrier frequency: The carrier frequency determines the frequency at which the signal is transmitted, but it does not affect the frequency characteristics of the modulating signal (the voice). Therefore, a poor selection of carrier frequency would not cause a male voice to sound like a female voice.
-
(d) loss of energy in transmission: Loss of energy in transmission would result in a weaker signal at the receiver, potentially causing a reduction in signal quality or intelligibility. However, it would not alter the frequency characteristics of the received signal, so it would not cause a male voice to sound like a female voice.
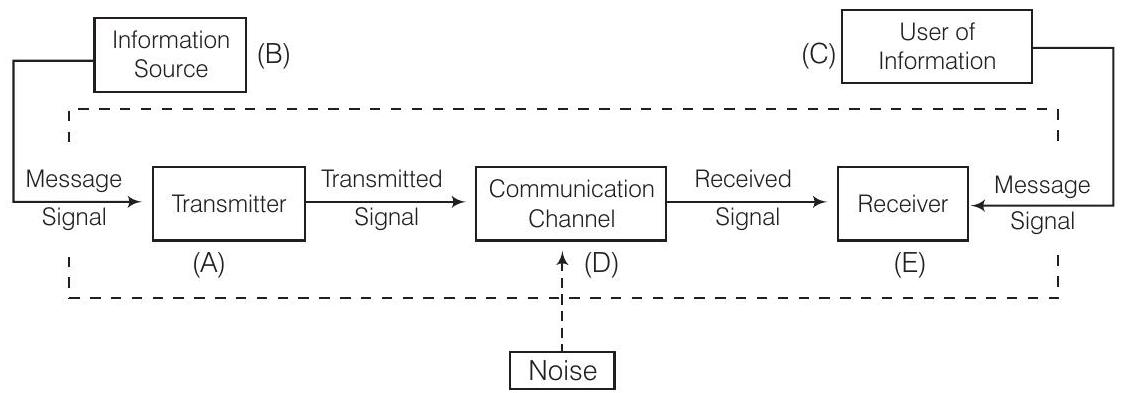
8. A basic communication system consists of
A. transmitter.
B. information source.
C. user of information.
D. channel.
E. receiver.
Choose the correct sequence in which these are arranged in a basic communication system. $\newline$
(a) ABCDE $\newline$
(b) BADEC $\newline$
(c) BDACE $\newline$
(d) BEADC $\newline$
Show Answer
Answer
(b) A communication system is the set-up used in the transmission and reception of information from one place to another.
The whole system consist of several elements in a sequence. It can be represented as the diagram given below
-
Option (a) ABCDE: This sequence is incorrect because it starts with the transmitter (A) instead of the information source (B). In a basic communication system, the information source is the starting point, not the transmitter.
-
Option (c) BDACE: This sequence is incorrect because it places the transmitter (A) after the channel (D). The correct sequence should have the transmitter before the channel, as the transmitter sends the information through the channel.
-
Option (d) BEADC: This sequence is incorrect because it places the receiver (E) before the channel (D). In a basic communication system, the receiver should come after the channel, as it receives the information transmitted through the channel.
9. Identify the mathematical expression for amplitude modulated wave $\newline$
(a) $A_{c} \sin [{\omega_{c}+k_1 V_{m}(t)} t+\phi]$ $\newline$
(b) $A_{c} \sin {\omega_{c} t+\phi+k_2 V_{m}(t)}$ $\newline$
(c) ${A_{c}+k_2 V_{m}(t)} \sin (\omega_{c} t+\phi)$ $\newline$
(d) $A_{c} V_{m}(t) \sin (\omega_{c} t+\phi)$ $\newline$
Show Answer
Thinking Process
An arbitrary change in phase angle of the modulating signal is given by $\phi$.
Answer
(c) Consider a sinusoidal modulating signal represented by
$$ m(t)=A_{m} \sin \omega_{m} t $$
where, $A_{m}=$ Amplitude of modulating signal $\omega_{m}=$ Angular frequency $=2 \pi V_{m}=\phi V_{m}$
Also consider a sinusoidal carrier wave represented by $C(t)=A_{c} \sin \omega_{c} t$
Thus, modulated wave is given by
Here,
$$ \begin{aligned} C_{m}(t) & =(A_{c}+A_{m} \sin \omega_{m} t) \sin \omega_{c} t \\ & =A_{c}[1+\frac{A_{m}}{A_{c}} \sin \omega_{m} t) \sin \omega_{c} t \end{aligned} $$
$\Rightarrow$
$$ \frac{A_{m}}{A_{c}}=M $$
$\Rightarrow \quad C_{m}(t)=(A_{c}+A_{c} \times \mu \sin \omega_{m} t) \sin \omega_{c} t$
Now, we know that $A_{c} \times \mu=K$ [wave constant] and $\sin \omega_{m} t=V_{m}$ [wave velocity]
Thus, Eq. (iii) becomes
$$ C_{m}(t)=(A_{c}+K \times V_{m}) \sin \omega_{c} t $$
Now, consider a change in phase angle by $\phi$ then $\sin \omega_{c} t arrow \sin (\omega_{c} t+\phi)$
Thus,
$$ C_{m}(t)=(A_{c}+K V_{m})(\sin \omega_{c}+\phi) $$
-
Option (a): The expression ( A_{c} \sin [{\omega_{c}+k_1 V_{m}(t)} t+\phi] ) represents a frequency-modulated wave rather than an amplitude-modulated wave. In amplitude modulation, the carrier frequency (\omega_{c}) remains constant, and it is the amplitude that varies with the modulating signal (V_{m}(t)).
-
Option (b): The expression ( A_{c} \sin {\omega_{c} t+\phi+k_2 V_{m}(t)} ) represents a phase-modulated wave rather than an amplitude-modulated wave. In amplitude modulation, the phase (\phi) remains constant, and it is the amplitude that varies with the modulating signal (V_{m}(t)).
-
Option (d): The expression ( A_{c} V_{m}(t) \sin (\omega_{c} t+\phi) ) incorrectly suggests that the amplitude of the carrier wave is directly multiplied by the modulating signal (V_{m}(t)). In amplitude modulation, the correct form involves adding the modulating signal to the carrier amplitude, not multiplying it.
Multiple Choice Questions (More Than One Options)
10. An audio signal of $15 kHz$ frequency cannot be transmitted over long distances without modulation, because
(a) the size of the required antenna would be at least $5 km$ which is not convenient
(b) the audio signal can not be transmitted through sky waves
(c) the size of the required antenna would be at least $20 km$, which is not convenient
(d) effective power transmitted would be very low, if the size of the antenna is less than $5 km$
Show Answer
Thinking Process
Transmission of a signal depends on three factors. These are size of antenna, medium of transmission and power of transmitted wave.
Answer
$(a, b, d)$
Given, frequency of the wave to be transmitted is
$$ \begin{aligned} v_{m} & =15 kHz=15 \times 10^{3} Hz \\ \text { Wavelength } \lambda_{m} & =\frac{c}{v_{m}}=\frac{3 \times 10^{8}}{15 \times 10^{3}}=\frac{1}{5} \times 10^{5} m \end{aligned} $$
Size of the antenna required, $\quad l=\frac{\lambda}{4}=\frac{1}{4} \times \frac{1}{5} \times 10^{5}$
$$ =5 \times 10^{3} m=5 km $$
The audio signals are of low frequency waves. Thus, they cannot be transmitted through sky waves as they are absorbed by atmosphere.
If the size of the antenna is less than $5 km$, the effective power transmission would be very low because of deviation from resonance wavelength of wave and antenna length.
- Option (c) is incorrect because the size of the required antenna for a 15 kHz frequency signal is calculated to be 5 km, not 20 km.
11. Audio sine waves of $3 kHz$ frequency are used to amplitude modulate a carrier signal of $1.5 MHz$. Which of the following statements are true?
(a) The side band frequencies are $1506 kHz$ and $1494 kHz$
(b) The bandwidth required for amplitude modulation is $6 kHz$
(c) The bandwidth required for amplitude modulation is $3 MHz$
(d) The side band frequencies are $1503 kHz$ and $1497 kHz$
Show Answer
Thinking Process
Here, in this question, options are giving the value of side band frequencies and band width of amplitude modulation. So, first of all find this quantities.
Answer
$(b, d)$
Given,
$$ \begin{aligned} \omega_{m} & =3 kHz \\ \omega_{c} & =1.5 MHz=1500 kHz \end{aligned} $$
Now, side band frequencies
$$ \begin{aligned} \omega_{c} \pm \omega_{m} & =(1500 \pm 3) \\ & =1503 kHz \text { and } 1497 kHz \end{aligned} $$
Also, bandwidth $=2 \omega_{m}=2 \times 3=6 kHz$
-
Option (a) is incorrect because the side band frequencies are calculated as $\omega_{c} \pm \omega_{m} = 1500 \pm 3 = 1503 \text{kHz}$ and $1497 \text{kHz}$, not $1506 \text{kHz}$ and $1494 \text{kHz}$.
-
Option (c) is incorrect because the bandwidth required for amplitude modulation is $2 \omega_{m} = 2 \times 3 \text{kHz} = 6 \text{kHz}$, not $3 \text{MHz}$.
12. A TV transmission tower has a height of $240 m$. Signals broadcast from this tower will be received by LOS communication at a distance of (assume the radius of earth to be $(6.4 \times 10^{6} m)$
(a) $100 km$
(b) $24 km$
(c) $55 km$
(d) $50 km$
Show Answer
Thinking Process
$$ \text { Range } d_{T}=\sqrt{2 R h_{T}} $$
Answer
$(b, c, d)$
Given, $\quad$ height of tower $h=240 m$
For LOS (line of sight) communication.
The maximum distance on earth from the transmitter upto which a signal can be received is given by
$$ d=\sqrt{2 R h} $$
Here $R$ is the radius of the earth i.e., $R=6.4 \times 10^{6} m$
Putting all these values in Eq. (i), we get
$$ \begin{aligned} d & =\sqrt{2 R h}=\sqrt{2 \times 6.4 \times 10^{6} \times 240} \\ & =55.4 \times 10^{3} m=55.4 km \end{aligned} $$
Thus, the range of $55.4 km$ covers the distance $24 km, 55 km$ and $50 km$.
-
Option (a) $100 km$: This option is incorrect because the calculated maximum distance for LOS communication from the tower is $55.4 km$. A distance of $100 km$ exceeds this maximum range, making it impossible for the signal to be received at this distance.
-
Option (b) $24 km$: This option is correct because $24 km$ is within the calculated maximum distance of $55.4 km$.
-
Option (c) $55 km$: This option is correct because $55 km$ is within the calculated maximum distance of $55.4 km$.
-
Option (d) $50 km$: This option is correct because $50 km$ is within the calculated maximum distance of $55.4 km$.
13. The frequency response curve (figure) for the filter circuit used for production of AM wave should be
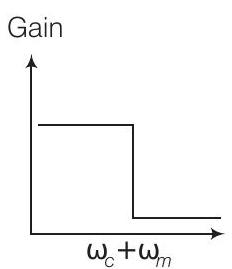
(i)
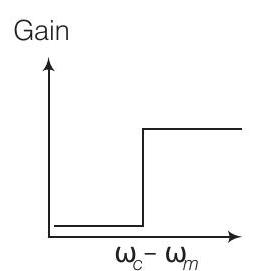
(ii)
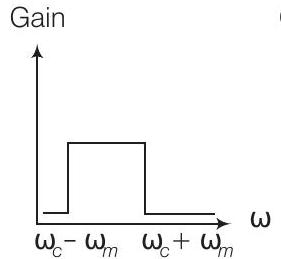
(iii)

(iv)
(a) (i) followed by (ii)
(b) (ii) followed by (i)
(c) (iii)
(d) (iv)
Show Answer
Answer
$(a, b, c)$
Here, for the production of amplitude modulated wave, bandwidth is given by =frequency of upper side band - frequency of lower side band
$$ =\omega_{USB}-\omega_{LSB}=(\omega_{c}+\omega_{m})-(\omega_{c}-\omega_{m}) $$
-
Option (b) (ii) followed by (i):
- This option suggests that the frequency response curve should first follow the pattern of (ii) and then (i). However, for the production of an AM wave, the filter needs to pass the frequencies corresponding to the upper and lower sidebands while rejecting others. The sequence of (ii) followed by (i) does not align with this requirement as it would not correctly filter the necessary frequencies.
-
Option (c) (iii):
- This option suggests that the frequency response curve should follow the pattern of (iii). The curve in (iii) does not represent the correct bandpass filter required for AM wave production. It does not appropriately pass the upper and lower sidebands while rejecting other frequencies.
-
Option (d) (iv):
- This option suggests that the frequency response curve should follow the pattern of (iv). The curve in (iv) does not match the required bandpass filter characteristics for AM wave production. It does not correctly filter the necessary frequencies for the upper and lower sidebands.
14. In amplitude modulation, the modulation index $m$, is kept less than or equal to 1 because
(a) $m>1$, will result in interference between carrier frequency and message frequency, resulting into distortion
(b) $m>1$, will result in overlapping of both side bands resulting into loss of information
(c) $m>1$, will result in change in phase between carrier signal and message signal
(d) $m>1$, indicates amplitude of message signal greater than amplitude of carrier signal resulting into distortion
Show Answer
Answer
$(b, d)$
The modulation index $(m)$ of amplitude modulated wave is
$$ m=\frac{\text { amplitude of message signal }(A_{m})}{\text { amplitude of carrier signal }(A_{c})} $$
If $m>1$, then $A_{m}>A_{c}$.
In this situation, there will be distortion of the resulting signal of amplitude modulated wave. Maximum modulation frequency $(m_{f})$ of $A_{m}$ wave is
$$ \begin{aligned} m_{f} & =\frac{\Delta v_{\max }}{v_{m}(\max )} \\ & =\frac{\text { frequency deviation }}{\text { maximum frequency value of modulating wave }} \end{aligned} $$
If $m_{t}>1$, then $\Delta v_{\max }>v_{m}$. It means, there will be overlapping of both side bands of modulated wave resulting into loss of information.
-
Option (a) is incorrect because interference between carrier frequency and message frequency is not the primary reason for keeping the modulation index $m \leq 1$. The main issue with $m > 1$ is the distortion and overlapping of sidebands, not interference between the carrier and message frequencies.
-
Option (c) is incorrect because a change in phase between the carrier signal and the message signal is not a direct consequence of having a modulation index $m > 1$. The primary concern with $m > 1$ is the distortion and overlapping of sidebands, not phase changes.
Very Short Answer Type Questions
15. Which of the following would produce analog signals and which would produce digital signals?
(a) A vibrating tuning fork
(b) Musical sound due to a vibrating sitar string
(c) Light pulse
(d) Output of NAND gate
Show Answer
Answer
Analog and digital signals are used to transmit information, usually through electric signals. In both these technologies, the information such as any audio or video is transformed into electric signals.
The difference between analog and digital technologies is that in analog technology, information is translated into electric pulses of varying amplitude. In digital technology, translation of information is into binary formal (zero or one) where each bit is representative of two distinct amplitudes.
Thus, (a) and
(b) would produce analog signal and
(c) and
(d) would produce digital signals.
16. Would sky waves be suitable for transmission of TV signals of $60 MHz$ frequency?
Show Answer
Answer
A signal to be transmitted through sky waves must have a frequency range of $1710 kHz$ to $40 MHz$.
But, here the frequency of TV signals are $60 MHz$ which is beyond the required range.
So, sky waves will not be suitable for transmission of TV signals of $60 MHz$ frequency.
17. Two waves $A$ and $B$ of frequencies $2 MHz$ and $3 MHz$, respectively are beamed in the same direction for communication via sky wave. Which one of these is likely to travel longer distance in the ionosphere before suffering total internal reflection?
Show Answer
Answer
As the frequency of wave $B$ is more than wave $A$, it means the refractive index of wave $B$ is more than refractive index of wave $A$ (as refractive index increases with frequency increases).
For higher frequency wave (i.e., higher refractive index) the angle of refraction is less i.e., bending is less. So, wave $B$ travel longer distance in the ionosphere before suffering total internal reflection.
18. The maximum amplitude of an $A M$ wave is found to be $15 V$ while its minimum amplitude is found to be $3 V$. What is the modulation index?
Show Answer
Answer
Let $A_{c}$ and $A_{m}$ be the amplitudes of carrier wave and modulating wave respectively. So,
$$ \text { Maximum amplitude } arrow A_{\max }=A_{c}+A_{m}=15 V $$
$$ \text { Minimum amplitude } arrow A_{\min }=A_{c}-A_{m}=3 V $$
Adding Eqs. (i) and (ii), we get
$$ \begin{aligned} 2 A_{c} & =18 \\ A_{c} & =9 V \end{aligned} $$
$$ \text { and } \quad \begin{aligned} A_{m} & =15-9=6 V \\ \text { Modulating index of wave } \mu & =\frac{A_{m}}{A_{c}}=\frac{6}{9}=\frac{2}{3} \end{aligned} $$
19. Compute the $L C$ product of a tuned amplifier circuit required to generate a carrier wave of $1 MHz$ for amplitude modulation.
Show Answer
Thinking Process
$$ \text { For tuned amplifier } f=\frac{1}{2 \pi \sqrt{L C}} $$
Answer
Given, the frequency of carrier wave is $1 MHz$.
Formula for the frequency of tuned amplifier,
$$ \begin{aligned} \frac{1}{2 \pi \sqrt{L C}} & =1 MHz \\ \sqrt{L C} & =\frac{1}{2 \pi \times 10^{6}} \\ L C & =\frac{1}{(2 \pi \times 10^{6})^{2}}=2.54 \times 10^{-14} S \end{aligned} $$
Thus, the product of $L C$ is $2.54 \times 10^{-14} s$.
20. Why is a AM signal likely to be more noisy than a FM signal upon transmission through a channel?
Show Answer
Answer
In case of AM, the instantaneous voltage of carrier waves is varied by the modulating wave voltage. So, during the transmission, nosie signals can also be added and receiver assumes noise a part of the modulating signal.
In case of FM, the frequency of carrier waves is changed as the change in the instantaneous voltage of modulating waves. This can be done by mixing and not while the signal is transmitting in channel. So, noise does not affect FM signal.
Short Answer Type Questions
21. Figure shows a communication system. What is the output power when input signal is of $1.01 mW$ ? [gain in $dB=10 \log _{10}(P _0 / P _{i})$ ]
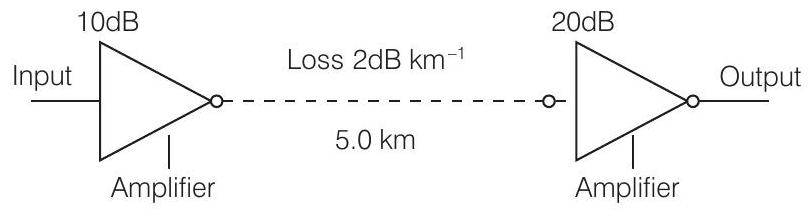
Show Answer
Answer
The distance travelled by the signal is $5 km$ Loss suffered in path of transmission $=2 dB / km$
So, total loss suffered in $5 km=-2 \times 5=-10 dB$
Total amplifier gain $=10 dB+20 dB=30 dB$
Overall gain in signal $=30-10=20 dB$
According to the question, gain in $dB=10 \log _{10} \frac{P_0}{P_j}$
$$ \therefore 20 =10 \log_{10} \frac{P_0}{P_i} $$ $$ \text {or} \log _{10} \frac{P_0}{P_i}=2 $$
Here, $P_{i}=1.01 mW$ and $P_0$ is the output power.
$$ \begin{matrix} \therefore & \frac{P_0}{P_{i}}=10^{2}=100 \\ \Rightarrow & P_0=P_{i} \times 100=1.01 \times 100 \\ \text { or } & P_0=101 mW \\ \text { Thus, the output power is } 101 mW . \end{matrix} $$
22. A TV transmission tower antenna is at a height of $20 m$. How much service area can it cover if the receiving antenna is (i) at ground level, (ii) at a height of $25 m$ ? Calculate the percentage increase in area covered in case (ii) relative to case (i).
Show Answer
Answer
Given, height of antenna $h=20 m$
Radius of earth $=6.4 \times 10^{6} m$
At the ground level,
(i) Range $=\sqrt{2 h R}=\sqrt{2 \times 20 \times 6.4 \times 10^{6}}$
$$ \begin{aligned} & =16000 m=16 km \\ \text { Area covered } A & =\pi(\text { range })^{2} \\ & =3.14 \times 16 \times 16=803.84 km^{2} \end{aligned} $$
(ii) At a height of $H=25 m$ from ground level
$$ \begin{aligned} \text { Range } & =\sqrt{2 h R}+\sqrt{2 H R} \\ & =\sqrt{2 \times 20 \times 6.4 \times 10^{6}}+\sqrt{2 \times 25 \times 6.4 \times 10^{6}} \\ & =16 \times 10^{3}+17.9 \times 10^{3} \\ & =33.9 \times 10^{3} m \\ & =33.9 km \end{aligned} $$
$$ \begin{aligned} \text { Area covered } & =\pi(\text { Range })^{2} \\ & =3.14 \times 33.9 \times 33.9 \\ & =3608.52 km^{2} \\ \text { Percentage increase in area } & =\frac{\text { Difference in area }}{\text { Initial area }} \times 100 \\ & =\frac{(3608.52-803.84)}{803.84} \times 100 \\ & =348.9 % \end{aligned} $$
Thus, the percentage increase in area covered is $348.9 %$
23. If the whole earth is to be connected by LOS communication using space waves (no restriction of antenna size or tower height), what is the minimum number of antennas required? Calculate the tower height of these antennas in terms of earth’s radius.
$$ \begin{gathered} K \text { Thinking Process } \\ \text { Range } d_{T}=\sqrt{2 R h t} \end{gathered} $$
Show Answer
Answer
Consider the figure given below to solve this question
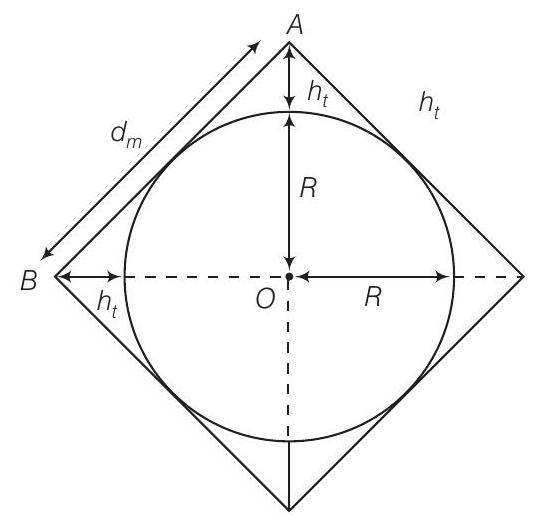
Suppose the height of transmitting antenna or receiving antenna in order to cover the entire surface of earth through communication is $h_{t}$ and radius of earth is $R$
Then, maximum distance
$$ \begin{matrix} d_m^{2} =(R+h_{t})^{2}+(R+h_{t})^{2} \newline =2(R+h_{t})^{2} \newline \therefore d_{m} =\sqrt{2 h_{t} R}+\sqrt{2 h_{t} R}=2 \sqrt{2 h_{t} R} \newline \Rightarrow 8 h_{t} R =2(R+h_{t})^{2} \newline \Rightarrow 4 h_{t} R =R^{2}+2 R h_{t}+h_t^{2} \newline \Rightarrow R^{2}-2 h_{t} R+h_t^{2} =0 \newline \Rightarrow (R-h_{t})^{2} =0 \newline \Rightarrow R =h_{t} \end{matrix} $$
Since, space wave frequency is used so $\lambda«h_{t}$, hence only tower height is to be taken into consideration. In three dimensions of earth, 6 antenna towers of each of height $h_{t}=R$ would be used to cover the entire surface of earth with communication programme.
24. The maximum frequency for reflection of sky waves from a certain layer of the ionosphere is found to be $f_{\max }=9(N_{\max })^{1 / 2}$, where $N_{\max }$ is the maximum electron density at that layer of the ionosphere.
On a certain day it is observed that signals of frequencies higher than $5 MHz$ are not received by reflection from the $F_1$ layer of the ionosphere while signals of frequencies higher than $8 MHz$ are not received by reflection from the $F_2$ layer of the ionosphere. Estimate the maximum electron densities of the $F_1$ and $F_2$ layers on that day.
Show Answer
Answer
The maximum frequency for reflection of sky waves
$$ f_{\max }=9(N_{\max })^{1 / 2} $$
where, $N_{\text {max }}$ is a maximum electron density.
$$ \begin{aligned} & \text { For } F_1 \text { layer, } \\ & \begin{aligned} f_{\max } & =5 MHz \\ 5 \times 10^{6} & =9(N_{\max })^{1 / 2} \end{aligned} \end{aligned} $$
Maximum electron density
$$ \begin{aligned} & N_{\max }=\frac{5}{9} \times 10^{6}{ }^{2}=3.086 \times 10^{11} / m^{3} \\ & f_{\max }=8 MHz \\ & 8 \times 10^{6}=9(N_{\max })^{1 / 2} \end{aligned} $$
Maximum electron density
$$ N_{\max }=\frac{8 \times 10^{6}}{9}=7.9 \times 10^{11} / m^{3} $$
25. On radiating (sending out) and $A M$ modulated signal, the total radiated power is due to energy carried by $\omega_{c}, \omega_{c}-\omega_{m}$ and $\omega_{c}+\omega_{m}$. Suggest ways to minimise cost of radiation without compromising on information.
Show Answer
Answer
In amplitude modulated signal, only side band frequencies contain information. Thus only $(\omega_{c}+\omega_{m})$ and $(\omega_{c}-\omega_{m})$ contain information.
Now, according to question, the total radiated power is due to energy carried by
$$ \omega_{c},(\omega_{c}-\omega_{m}) \text { and }(\omega_{c}+\omega_{m}) \text {. } $$
Thus to minimise the cost of radiation without compromising on information $\omega_{c}$ can be left and transmitting. $(\omega_{c}+\omega_{m}),(\omega_{c}-\omega_{m})$ or both $(\omega_{c}+\omega_{m})$ and $(\omega_{c}-\omega_{m})$.
Long Answer Type Questions
26. The intensity of a light pulse travelling along a communication channel decreases exponentially with distance $x$ according to the relation $I=I_0 e^{-\alpha x}$, where $I_0$ is the intensity at $x=0$ and $\alpha$ is the attenuation constant.
(a) Show that the intensity reduces by $75 %$ after a distance of $\frac{\ln 4}{\alpha}$.
(b) Attenuation of a signal can be expressed in decibel ( $dB$ ) according to the relation $dB=10 \log _{10} \frac{I}{I_0}$. What is the attenuation in $dB / km$ for an optical fibre in which the intensity falls by $50 %$ over a distance of $50 km$ ?
Show Answer
Answer
(a) Given, the intensity of a light pulse $I=I_0 e^{-\alpha x}$
where, $I_0$ is the intensity at $x=0$ and $\alpha$ is constant.
According to the question, $I=25 %$ of $I_0=\frac{25}{100} \cdot I_0=\frac{I_0}{4}$
Using the formula mentioned in the question,
or
$$ \begin{aligned} I & =I_0 e^{-\alpha x} \\ \frac{I_0}{4} & =I_0 e^{-\alpha x} \\ \frac{1}{4} & =e^{-\alpha x} \end{aligned} $$
Taking log on both sides, we get
$$ \begin{aligned} \ln 1-\ln 4 & =-\alpha x \ln e \quad(\because \ln e=1) \\ -\ln 4 & =-\alpha x \\ x & =\frac{\ln 4}{\alpha} \end{aligned} $$
Therefore, at distance $x=\frac{\ln 4}{\alpha}$, the intensity is reduced to $75 %$ of initial intensity.
(b) Let $\alpha$ be the attenuation in $dB / km$. If $x$ is the distance travelled by signal, then
$$ 10 \log _{10} \frac{I}{I_0}=-\alpha x $$
where, $I_0$ is the intensity initially.
According to the question, $I=50 %$ of $I_0=\frac{I_0}{2}$ and $x=50 km$
Putting the value of $x$ in Eq. (i), we get
$$ \begin{aligned} 10 \log _{10} \frac{I_0}{2 I_0} & =-\alpha \times 50 \\ 10[\log 1-\log 2] & =-50 \alpha \\ \frac{10 \times 0.3010}{50} & =\alpha \end{aligned} $$
$\therefore$ The attenuation for an optical fibre
$$ \alpha=0.0602 dB / km $$
27. A $50 MHz$ sky wave takes $4.04 ms$ to reach a receiver via re-transmission from a satellite $600 km$ above Earth’s surface. Assuming re-transmission time by satellite negligible, find the distance between source and receiver. If communication between the two was to be done by Line of Sight (LOS) method, what should size and placement of receiving and transmitting antenna be?
Show Answer
Answer
Let the receiver is at point $A$ and source is at $B$.

Velocity of waves $=3 \times 10^{8} m / s$
Time to reach a receiver $=4.04 ms=4.04 \times 10^{-3} s$
Let the height of satellite is
$h_{s}=600 km$
Radius of earth $=6400 km$
Size of transmitting antenna $=h_{T}$
We know that $\frac{\text { Distance travelled by wave }}{\text { Time }}=$ Velocity of waves
$$ \begin{aligned} \frac{2 x}{4.04 \times 10^{-3}} & =3 \times 10^{8} \\ x & =\frac{3 \times 10^{8} \times 4.04 \times 10^{-3}}{2} \\ & =6.06 \times 10^{5}=606 km \end{aligned} $$
or
Using Phythagoras theorem,
or
$$ d^{2}=x^{2}-h_s^{2}=(606)^{2}-(600)^{2}=7236 $$
So, the distance between source and receiver $=2 d$
$$ =2 \times 85.06=170 km $$
The maximum distance covered on ground from the transmitter by emitted EM waves
$$ \text { or } $$
$$ d=\sqrt{2 R h_{T}} $$
$$ \frac{d^{2}}{2 R}=h_{T} $$
or
$$ \text { size of antenna } h_{T}=\frac{7236}{2 \times 6400} $$
$$ =0.565 km=565 m $$
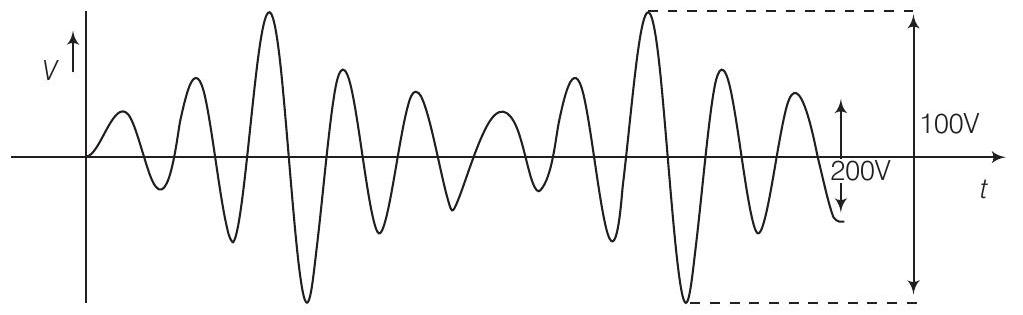
28. An amplitude modulated wave is as shown in figure. Calculate
(i) the percentage modulation,
(ii) peak carrier voltage and
(iii) peak value of information voltage
Show Answer
Answer
From the diagram,
$$ \begin{aligned} & \text { Maximum voltage } V_{\max }=\frac{100}{2}=50 V \\ & \text { Minimum voltage } V_{\min }=\frac{20}{2}=10 V \end{aligned} $$
(i) Percentage modulation, $\mu=\frac{V_{\max }-V_{\min }}{V_{\max }+V_{\min }} \times 100=\frac{50-10}{50+10} \times 100$
$$ =\frac{40}{60} \times 100=66.67 % $$
(ii) Peak carrier voltage, $\quad V_{c}=\frac{V_{\max }+V_{\min }}{2}=\frac{50+10}{2}=30 V$
(iii) Peak value of information voltage,
$$ V_{m}=\mu V_{c}=\frac{66.67}{100} \times 30=20 V $$
29. (i) Draw the plot of amplitude versus $\omega$ for an amplitude modulated were whose carrier wave $(\omega_{c})$ is carrying two modulating signals, $\omega_1$ and $\omega_2$ $(\omega_2>\omega_1)$.
(ii) Is the plot symmetrical about $\omega_{c}$ ? Comment especially about plot in region $\omega<\omega_{c}$.
(iii) Extrapolate and predict the problems one can expect if more waves are to be modulated.
(iv) Suggest solutions to the above problem. In the process can one understand another advantage of modulation in terms of bandwidth?
Show Answer
Answer
(i) The plot of amplitude versus $\omega$ can be shown in the figure below

(ii) From figure, we note that frequency spectrum is not symmetrical about $\omega_{c}$. Crowding of spectrum is present for $\omega<\omega_{t}$.
(iii) If more waves are to be modulated then there will be more crowding in the modulating signal in the region $\omega<\omega_{c}$. That will result more chances of mixing of signals.
(iv) To accommodate more signals, we should increase bandwidth and frequency carrier waves $\omega_{t}$. This shows that large carrier frequency enables to carry more information (i.e., more $\omega_{m}$ ) and the same will in turn increase bandwidth.
30. An audio signal is modulated by a carrier wave of $20 MHz$ such that the bandwidth required for modulation is $3 kHz$. Could this wave be demodulated by a diode detector which has the values of $R$ and $C$ as
(i) $R=1 k \Omega, C=0.01 \mu F$.
(ii) $R=10 k \Omega, C=0.01 \mu F$.
(iii) $R=10 k \Omega, C=0.1 \mu F$.
Show Answer
Answer
Given, carrier wave frequency $f_{c}=20 MHz$
$$ =20 \times 10^{6} Hz $$
Bandwidth required for modulation is
$$ \begin{aligned} & 2 f_{m}=3 kHz=3 \times 10^{3} Hz \\ \Rightarrow & f_{m}=\frac{3 \times 10^{3}}{2}=1.5 \times 10^{3} Hz \end{aligned} $$
Demodulation by a diode is possible if the condition $\frac{1}{f_{c}} \ll R C<\frac{1}{f_{m}}$ is satisfied
Thus,
$$ \begin{aligned} \frac{1}{f_{c}} & =\frac{1}{20 \times 10^{6}}=0.5 \times 10^{-7} \\ \frac{1}{f_{m}} & =\frac{1}{1.5 \times 10^{3}} Hz=0.7 \times 10^{-3} s \end{aligned} $$
$$ \text { and } $$
Now, gain through all the options of $R$ and $C$ one by one, we get
(i) $R C=1 k \Omega \times 0.01 \mu F=10^{3} \Omega \times(0.01 \times 10^{-6} F)=10^{-5} S$
Here, condition $\frac{1}{f_{c}} \ll R C<\frac{1}{f_{m}}$ is satisfied.
Hence it can be demodulated.
(ii) $R C=10 K \Omega \times 0.01 \mu F=10^{4} \Omega \times 10^{-8} F=10^{-4} S$
Here condition $\frac{1}{f_{c}} \ll R C<\frac{1}{f_{m}}$ is satisfied.
Hence, it can be demodulated.
(iii) $R C=10 k \Omega \times 1 \mu \mu F=10^{4} \Omega \times 10^{-12} F=10^{-8} S$
Here, condition $\frac{1}{f_{c}}>R C$, so this cannot be demodulated.
The emf induced across $P Q$ due to its motion or change in magnetic flux linked with the loop change due to change of enclosed area.
For nth minima to be formed on the screen path difference between the rays coming from $S_1$ and $S_2$ must be $(2 n-1) \frac{\lambda}{2}$.










