Mechanical properties of solids
Multiple Choice Questions (MCQs)
1. Modulus of rigidity of ideal liquids is
(a) infinity
(b) zero
(c) unity
(d) some finite small non-zero constant value
Show Answer
Answer (b) No frictional (viscous) force exists in case of ideal fluid, hence, tangential forces are zero so there is no stress developed.
-
(a) Infinity: If the modulus of rigidity were infinity, it would imply that the liquid is perfectly rigid and cannot be deformed by any amount of shear stress, which contradicts the nature of liquids that can flow and deform under shear stress.
-
(c) Unity: A modulus of rigidity of unity would imply that the liquid has a specific, non-zero resistance to shear stress, which is not the case for ideal liquids that have no resistance to shear stress.
-
(d) Some finite small non-zero constant value: This would imply that the liquid has some measurable resistance to shear stress, which contradicts the definition of an ideal liquid that has no viscosity and thus no resistance to shear stress.
2. The maximum load a wire can withstand without breaking, when its length is reduced to half of its original length, will
(a) be double
(b) be half
(c) be four times
(d) remain same
Show Answer
Answer
(d) We know that, Breaking stress
When length of the wire changes, area of cross-section remains same.
Hence, breaking force will be same when length changes.
-
Option (a) is incorrect because the breaking force is determined by the breaking stress and the area of cross-section, which remains unchanged when the length is halved. Therefore, the breaking force does not double.
-
Option (b) is incorrect because halving the length of the wire does not affect the breaking stress or the area of cross-section. Thus, the breaking force does not become half.
-
Option (c) is incorrect because the breaking force is not dependent on the length of the wire but on the breaking stress and the area of cross-section. Therefore, the breaking force does not become four times.
3. The temperature of a wire is doubled. The Young’s modulus of elasticity
(a) will also double
(b) will become four times
(c) will remain same
(d) will decrease
Show Answer
Answer (d) We know that with increase in temperature length of a wire changes as
where
Now we can write
where
Young’s modulus
As,
When temperature increases
-
(a) will also double: This option is incorrect because Young’s modulus of elasticity is inversely proportional to the change in temperature. As temperature increases, Young’s modulus decreases, not increases.
-
(b) will become four times: This option is incorrect because Young’s modulus does not increase with temperature. Instead, it decreases as temperature increases. The relationship is inverse, not direct or exponential.
-
(c) will remain same: This option is incorrect because Young’s modulus is affected by temperature changes. As temperature increases, the modulus decreases, so it does not remain the same.
4. A spring is stretched by applying a load to its free end. The strain produced in the spring is
(a) volumetric
(b) shear
(c) longitudinal and shear
(d) longitudinal
Show Answer
Answer (c) Consider the diagram where a spring is stretched by applying a load to its free end. Clearly the length and shape of the spring changes.
The change in length corresponds to longitudinal strain and change in shape corresponds to shearing strain.
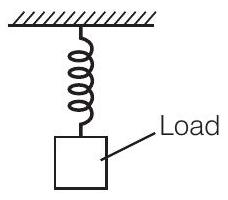
-
(a) Volumetric: Volumetric strain refers to a change in the volume of a material. When a spring is stretched, its volume does not change significantly; rather, it is the length and shape that are primarily affected.
-
(b) Shear: While shear strain is indeed produced in the spring, it is not the only type of strain. The spring also experiences longitudinal strain due to the change in length. Therefore, this option is incomplete.
-
(d) Longitudinal: Longitudinal strain is produced in the spring due to the change in length. However, this option is incomplete because it does not account for the shear strain that also occurs due to the change in shape of the spring.
5. A rigid bar of mass
(a)
(b)
(c)
(d)
Show Answer
Answer (b) We know that Young’s modulus
Hence,
Now, we can find ratio as
-
Option (a)
-
Option (c)
-
Option (d)
6. A mild steel wire of length
(a)
(b)
(c)
(d)
Show Answer
Thinking Process
We will assume the vertical displacement
Answer (a) Consider the diagram below
Hence, change in length
-
Option (b)
-
Option (c)
-
Option (d)
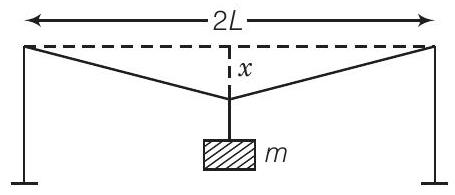
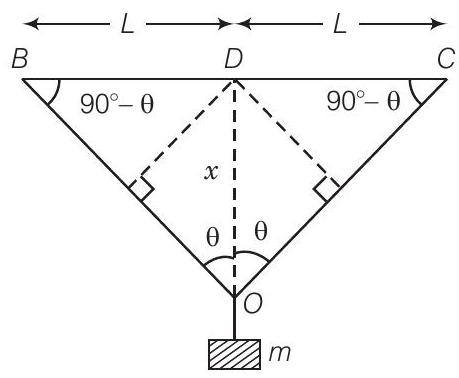
7. A rectangular frame is to be suspended symmetrically by two strings of equal length on two supports (figure). It can be done in one of the following three ways;
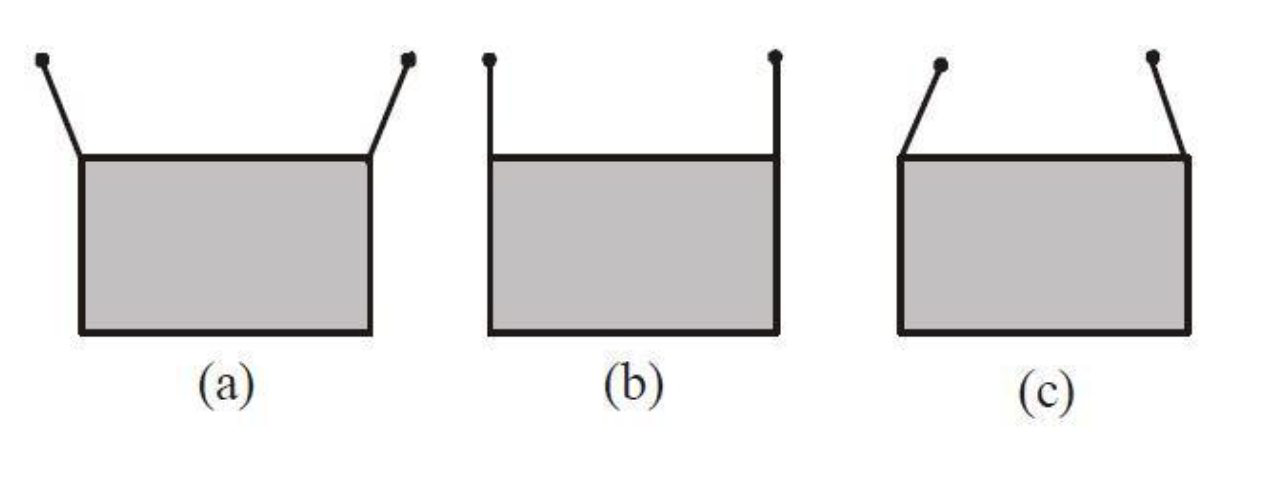
The tension in the strings will be
(a) the same in all cases
(b) least in (a)
(c) least in (b)
(d) least in (c)
Show Answer
Answer (c) Consider the FBD diagram of the rectangular frame
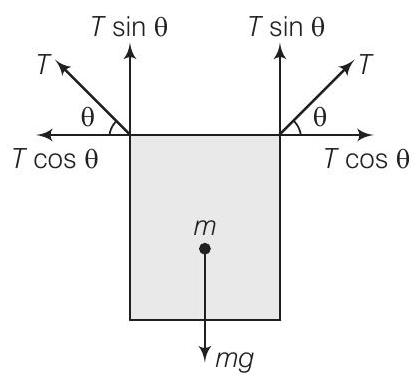
Balancing vertical forces
Total horizontal force
Now from Eq. (i),
As
No option matches with
Matches with option (b)
Hence, tension is least for the case (b).
Note We should be careful when measuring the angle, it must be in the direction as given in the diagram.
-
Option (a): This option is incorrect because the tension in the strings depends on the angle (\theta) between the string and the vertical. As (\theta) changes, the value of (\sin \theta) changes, which in turn affects the tension (T). Therefore, the tension cannot be the same in all cases.
-
Option (b): This option is incorrect because, as derived from the equation (T = \frac{mg}{2 \sin \theta}), the tension is inversely proportional to (\sin \theta). The tension is least when (\sin \theta) is maximum, which occurs at (\theta = 90^\circ). In case (b), (\theta) is not (90^\circ), so the tension is not the least in this case.
-
Option (d): This option is incorrect because, similar to option (b), the tension is least when (\sin \theta) is maximum. In case (c), (\theta) is (90^\circ), which makes (\sin \theta = 1), resulting in the least tension. Therefore, the tension is not least in case (d).
8. Consider two cylindrical rods of identical dimensions, one of rubber and the other of steel. Both the rods are fixed rigidly at one end to the roof. A mass
(a) Both the rods will elongate but there shall be no perceptible change in shape
(b) The steel rod will elongate and change shape but the rubber rod will only elongate
(c) The steel rod will elongate without any perceptible change in shape, but the rubber rod will elongate and the shape of the bottom edge will change to an ellipse
(d) The steel rod will elongate, without any perceptible change in shape, but the rubber rod will elongate with the shape of the bottom edge tapered to a tip at the centre
Show Answer
Answer (d) Consider the diagram.
A mass

-
Option (a) is incorrect: While both rods will indeed elongate, the rubber rod will also change shape due to its higher elasticity compared to steel. The rubber rod will not maintain its original cylindrical shape as it stretches.
-
Option (b) is incorrect: The steel rod will elongate without a significant change in shape due to its high rigidity. The rubber rod, however, will not only elongate but also change shape, contradicting the statement that it will only elongate.
-
Option (c) is incorrect: The rubber rod will elongate and change shape, but the shape of the bottom edge will not change to an ellipse. Instead, it will taper to a tip at the center due to the distribution of the mass and the elastic properties of rubber.
Multiple Choice Questions (More Than One Options)
9. The stress-strain graphs for two materials are shown in figure. (assume same scale)
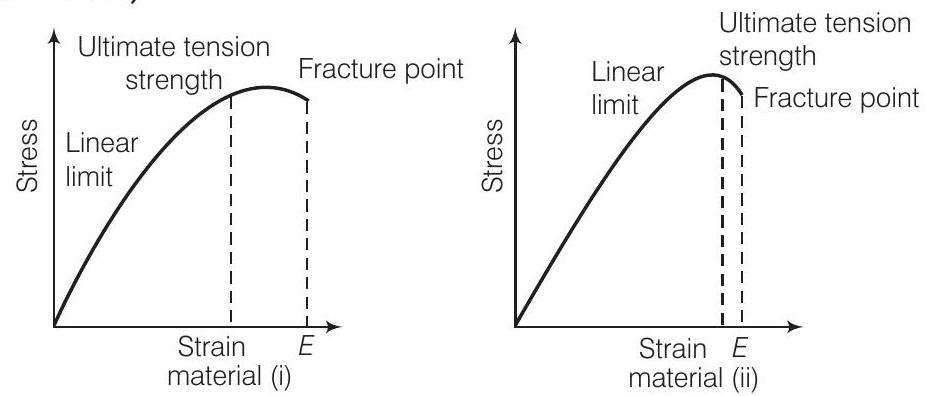
(a) Material (ii) is more elastic than material (i) and hence material (ii) is more brittle
(b) Material (i) and (ii) have the same elasticity and the same brittleness
(c) Material (ii) is elastic over a larger region of strain as compared to (i)
(d) Material (ii) is more brittle than material (i)
Show Answer
Thinking Process
We have to compare ultimate tensile strength for each material. Material having more ultimate tensile strength will be elastic over larger region.
Answer
(c,
It is clear from the two graphs, the ultimate tensile strength for material (ii) is greater, hence material(ii) is elastic over larger region as compared to material (i). For material(ii) fracture point is nearer, hence it is more brittle.
-
(a) Material (ii) is more elastic than material (i) and hence material (ii) is more brittle:
- Incorrect because elasticity is not directly related to brittleness. Elasticity refers to the ability of a material to return to its original shape after deformation, while brittleness refers to the tendency of a material to fracture without significant deformation. The graph does not provide information that directly correlates elasticity with brittleness.
-
(b) Material (i) and (ii) have the same elasticity and the same brittleness:
- Incorrect because the graphs show different stress-strain behaviors for the two materials. Material (ii) has a higher ultimate tensile strength and a different fracture point compared to material (i), indicating that they do not have the same elasticity or brittleness.
10. A wire is suspended from the ceiling and stretched under the action of a weight
(a) Tensile stress at any cross-section
(b) Tensile stress at any cross-section is zero
(c) Tensile stress at any cross-section
(d) Tension at any cross-section
Show Answer
Answer
As shown in the diagram
Clearly, forces at each cross-section is
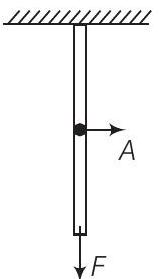
Now applying formula,
-
(b) Tensile stress at any cross-section is zero: This option is incorrect because tensile stress is defined as the force per unit area. Since there is a force ( F ) acting on the wire and the wire has a cross-sectional area ( A ), the tensile stress cannot be zero. It is given by ( \frac{F}{A} ).
-
(c) Tensile stress at any cross-section ( A ) of the wire is ( 2F / A ): This option is incorrect because the tensile stress is calculated as the force divided by the cross-sectional area. The force acting on the wire is ( F ), not ( 2F ). Therefore, the correct tensile stress is ( \frac{F}{A} ), not ( \frac{2F}{A} ).
11. A rod of length

(a) Mass
(b) Mass
(c) Mass
(d) Mass
Show Answer
Answer
Let the mass is placed at
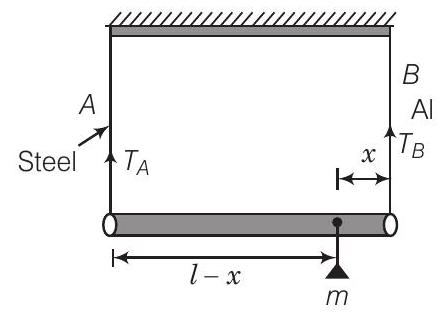
Let
For the rotational equilibrium of the system,
where
By question
Now, for equal stress
Hence, mass
For equal strain,
Hence, mass
-
Option (a) is incorrect: The mass should not be suspended close to wire A to have equal stresses in both wires. The correct placement for equal stress is closer to wire B, as derived in the solution. This is because the cross-sectional area of wire B is larger, and to balance the stress, the tension in wire B must be higher, which is achieved by placing the mass closer to wire B.
-
Option (c) is incorrect: Suspending the mass at the middle of the wires will not result in equal stresses in both wires. The stress in each wire depends on the tension, which is influenced by the position of the mass. Placing the mass in the middle would not account for the differences in cross-sectional areas and Young’s moduli of the two wires, leading to unequal stresses.
-
Option (d) is correct: This option is actually correct, as derived in the solution. The mass should be suspended closer to wire A to have equal strain in both wires. This is because the Young’s modulus and cross-sectional area differences require the mass to be positioned such that the strains (which are stress divided by Young’s modulus) are equal.
12. For an ideal liquid,
(a) the bulk modulus is infinite
(b) the bulk modulus is zero
(c) the shear modulus is infinite
(d) the shear modulus is zero
Show Answer
Answer
As an ideal liquid is not compressible.
Hence, change in volume,
As there is no tangential (viscous) force exists in case of an ideal fluid, hence, shear modulus
-
Option (b) the bulk modulus is zero: This is incorrect because for an ideal liquid, the bulk modulus is actually infinite. An ideal liquid is incompressible, meaning any applied pressure does not change its volume. Therefore, the bulk modulus, which measures resistance to compression, is infinitely large.
-
Option (c) the shear modulus is infinite: This is incorrect because for an ideal liquid, the shear modulus is zero. An ideal liquid cannot resist shear stress; it flows under any applied tangential force, indicating that it has no rigidity against shear deformation.
13. A copper and a steel wire of the same diameter are connected end to end. A deforming force
(a) the same stress
(b) different stress
(c) the same strain
(d) different strain
Show Answer
Answer
Consider the diagram where a deforming force
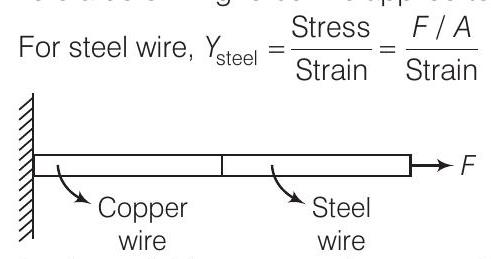
where
As
As,
Hence, the two wires will have differents strain.
-
Option (b) different stress: This option is incorrect because the stress in both wires is the same. Stress is defined as the force per unit area, and since the force
-
Option (c) the same strain: This option is incorrect because the strain in the two wires is different. Strain is defined as the ratio of the change in length to the original length, and it depends on the material’s Young’s modulus (
Very Short Answer Type Questions
14. The Young’s modulus for steel is much more than that for rubber. For the same longitudinal strain, which one will have greater tensile stress?
Show Answer
Answer
Young’s modulus
For same longitudinal strain,
But
Therefore, from Eq. (i),
15. Is stress a vector quantity?
Show Answer
Answer
Stress
Therefore, stress is a scalar quantity not a vector quantity.
16. Identical springs of steel and copper are equally stretched. On which, more work will have to be done?
Show Answer
Answer
Work done in stretching a wire is given by
[where
As both springs are identical,
From Eqs. (i) and (ii), we get
Therefore, more work will be done for stretching copper spring.
17. What is the Young’s modulus for a perfect rigid body?
Show Answer
Answer
Young’s modulus
For a perfectly rigid body, change in length
Therefore, Young’s modulus for a perfectly rigid body is infinite(
18. What is the Bulk modulus for a perfect rigid body?
Show Answer
Answer
Bulk modulus
For perfectly rigid body, change in volume
Therefore, bulk modulus for a perfectly rigid body is infinity
19. A wire of length
Show Answer
Thinking Process
In this problem, we have to apply Hooke’s law and then elongation in each wire will be compared.
Answer The situation is shown in the diagram.
Now,
For first wire,
For second wire,
From Eqs. (i) and (ii),

(
20. A steel
Show Answer
Thinking Process
Due to increase in temperature of the rod, length, increases. The equation of thermal expansion for linear expansion will be applied.
Answer
Given, Young’s modulus of steel
Coefficient of thermal expansion
Length
Area of cross-section
Increase in temperature
Tension produced in steel rod
21. To what depth must a rubber ball be taken in deep sea so that its volume is decreased by
Show Answer
Answer
Given, Bulk modulus of rubber
Density of sea water
Percentage decrease in volume,
Let the rubber ball be taken upto depth
22. A truck is pulling a car out of a ditch by means of a steel cable that is
Show Answer
Answer
23. Two identical solid balls, one of ivory and the other of wet-clay, are dropped from the same height on the floor. Which one will rise to a greater height after striking the floor and why?
Show Answer
Answer Since, ivory ball is more elastic than wet-clay ball, therefore, ivory ball tries to regain its original shape quickly. Hence, more energy and momentum is transferred to the ivory ball in comparison to the wet clay ball and therefore, ivory ball will rise higher after striking the floor.24. Consider a long steel bar under a tensile stress due to forces
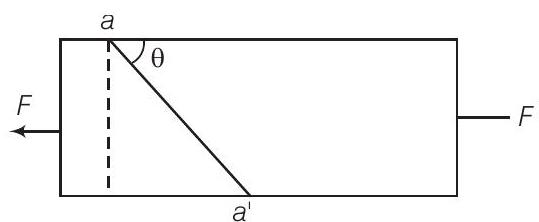
(a) For what angle is the tensile stress a maximum?
(b) For what angle is the shearing stress a maximum?
Show Answer
Thinking Process
To solve this question, we have to resolve the force along and perpendicular to the inclined plane. Now, we can easily calculate the tensile and shearing stress.
Answer Consider the adjacent diagram.
Let the cross-sectional area of the bar be
Let the area of the face aa’ be
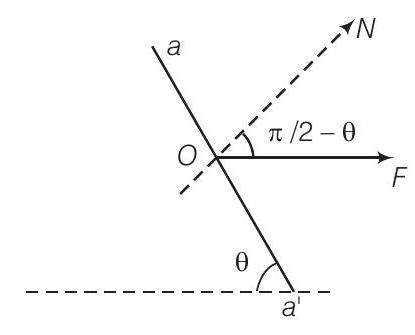
(a) For tensile stress to be maximum,
(b) For shearing stress to be maximum,
Note We must not apply the formula for stress directly, forces must be resolved.
25. (a) A steel wire of mass
(b) If the yield strength of steel is
Show Answer
Thinking Process
When the wire is hanging different part of the wire will be acted upon by different forces. To find the tension due to whole wire, consider a small element and find the tension there and then integrate for the whole wire.
Answer
Consider the diagram when a small element of length
(a) Let
Let the length
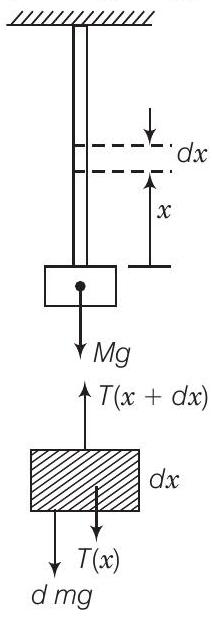
(
(b) Clearly tension will be maximum at
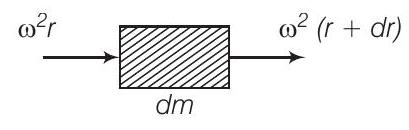
26. A steel rod of length
Show Answer
Thinking Process
To solve this question, we have to consider a small element, find tension in the rod at this element and then calculate for the whole rod.
Answer
Consider an element of width
Net centrifugal force on the element
(where

Let the increase in length of the element
27. An equilateral triangle
Show Answer
Thinking Process
Due to increase in temperature length of each side will change, hence, the angle corresponding to any vertex also changes.
Answer Consider the diagram shown
Let
Differentiating
(assume
Now,
and

Putting
(for equilateral triangle)
28. In nature, the failure of structural members usually result from large torque because of twisting or bending rather than due to tensile or compressive strains. This process of structural breakdown is called buckling and in cases of tall cylindrical structures like trees, the torque is caused by its own weight bending the structure. Thus, the vertical through the centre of gravity does not fall within the base. The elastic torque caused because of this bending about the central axis of the tree is given by
Show Answer
Thinking Process
In this question, the elastic torque is given we have to find the torque caused by the weight due to bending and equate with the given value.
Answer
Consider the diagram according to the question, the bending torque on the trunk of radius
where
When the tree is about to buckle
If
From
if
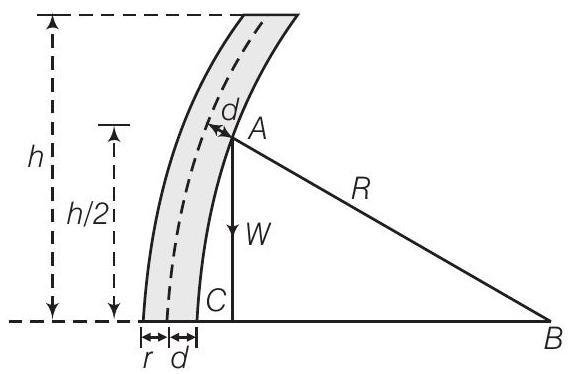
If
29. A stone of mass
(a) Find the distance
(b) What is the maximum velocity attained by the stone in this drop?
(c) What shall be the nature of the motion after the stone has reached its lowest point?
Show Answer
Thinking Process
In this problem ,the given string is elastic and there is no dissipation of energy we can apply conservation of mechanical energy.
Answer
Consider the diagram the stone is dropped from point
(a) Till the stone drops through a length
The loss in PE of the stone is the PE stored in the stretched string.
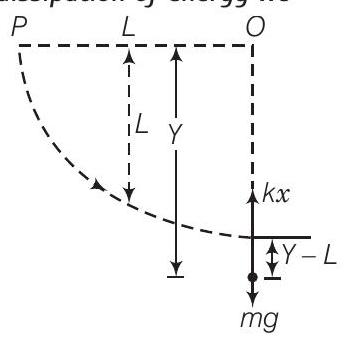
Retain the positive sign.
(b) In SHM, the maximum velocity is attained when the body passes, through the “equilibrium, position” i.e., when the instantaneous acceleration is zero. That is
Let the velocity be
Now,
(c) When stone is at the lowest position i.e., at instantaneous distance
Make a transformation of variables,
It is a differential equation of second order which represents SHM.
Comparing with equation
Angular frequency of harmonic motion
The solution of above equation will be of the type
Thus, the stone will perform SHM with angular frequency










