Oscillations
Multiple Choice Questions (MCQs)
1. The displacement of a particle is represented by the equation
(a) simple harmonic with period
(b) simple harmonic with period
(c) periodic but not simple harmonic
(d) non- periodic
Show Answer
Answer
(b) Given,
Velocity of the particle
Hence, due to negative sign motion is SHM.
Clearly, from the equation
So, motion is SHM with period
-
Option (a): The period given in option (a) is ( \frac{2\pi}{\omega} ). However, from the given equation ( y = 3 \cos \left( \frac{\pi}{4} - 2\omega t \right) ), the angular frequency is ( 2\omega ). Therefore, the period ( T ) is calculated as ( T = \frac{2\pi}{2\omega} = \frac{\pi}{\omega} ), not ( \frac{2\pi}{\omega} ).
-
Option (c): The motion described by the equation ( y = 3 \cos \left( \frac{\pi}{4} - 2\omega t \right) ) is simple harmonic motion (SHM) because it can be expressed in the form ( y = A \cos (\omega’ t + \phi) ), where ( A ) is the amplitude, ( \omega’ ) is the angular frequency, and ( \phi ) is the phase constant. Therefore, it is not just periodic but specifically SHM.
-
Option (d): The given equation ( y = 3 \cos \left( \frac{\pi}{4} - 2\omega t \right) ) represents a periodic function, as it repeats at regular intervals. Since it is periodic and follows the form of SHM, it cannot be classified as non-periodic.
2. The displacement of a particle is represented by the equation
(a) non-periodic
(b) periodic but not simple harmonic
(c) simple harmonic with period
(d) simple harmonic with period
Show Answer
Answer (b) Given equation of motion is
As the expression is involving sine function, hence it will be periodic.
-
(a) The motion is not non-periodic because the given equation ( y = \sin^3(\omega t) ) involves trigonometric functions, which are inherently periodic. Therefore, the motion must be periodic.
-
(c) The motion is not simple harmonic with period ( 2\pi / \omega ) because simple harmonic motion (SHM) requires that the acceleration (second derivative of displacement) be directly proportional to the displacement and opposite in direction. The given equation does not satisfy this condition.
-
(d) The motion is not simple harmonic with period ( \pi / \omega ) for the same reason as option (c). The acceleration is not directly proportional to the displacement, which is a requirement for SHM.
3. The relation between acceleration and displacement of four particles are given below
(a)
(b)
(c)
(d)
Which, one of the particle is exempting simple harmonic motion?
Show Answer
Answer (d) For motion to be SHM acceleration of the particle must be proportional to negative of displacement.
i.e.,
We should be clear that
-
(a)
-
(b)
-
(c)
4. Motion of an oscillating liquid column in a U-tube is
(a) periodic but not simple harmonic
(b) non-periodic
(c) simple harmonic and time period is independent of the density of the liquid
(d) simple harmonic and time period is directly proportional to the density of the liquid
Show Answer
Answer (c) Consider the diagram in which a liquid column oscillates. In this case, restoring force acts on the liquid due to gravity. Acceleration of the liquid column, can be calculated in terms of restoring force.

Restoring force
Which is independent of the density of the liquid.
-
(a) periodic but not simple harmonic: This option is incorrect because the motion of the liquid column in a U-tube is governed by a restoring force that is directly proportional to the displacement, which is a characteristic of simple harmonic motion (SHM). Therefore, the motion is not just periodic but also simple harmonic.
-
(b) non-periodic: This option is incorrect because the motion of the liquid column in a U-tube is periodic. The liquid oscillates back and forth in a regular, repeating pattern, which is the definition of periodic motion.
-
(d) simple harmonic and time period is directly proportional to the density of the liquid: This option is incorrect because the time period of the oscillation is independent of the density of the liquid. The time period ( T ) is given by ( T = 2\pi \sqrt{\frac{l}{g}} ), where ( l ) is the length of the liquid column and ( g ) is the acceleration due to gravity. The density of the liquid does not appear in this expression, indicating that it does not affect the time period.
5. A particle is acted simultaneously by mutually perpendicular simple harmonic motion
(a) an ellipse
(b) a parabola
(c) a circle
(d) a straight line
Show Answer
Thinking Process
We have to find resultant-displacement by adding
Answer (c) Given,
Squaring and adding Eqs. (i) and (ii),
This is the equation. of a circle
Clearly, the locus is a circle of constant radius a.
-
Option (a) an ellipse: The equation (x = a \cos \omega t) and (y = a \sin \omega t) describe a circle, not an ellipse. For an ellipse, the coefficients of (\cos \omega t) and (\sin \omega t) would need to be different, i.e., (x = a \cos \omega t) and (y = b \sin \omega t) where (a \neq b).
-
Option (b) a parabola: The given equations do not form a parabola. A parabolic trajectory typically involves a quadratic relationship between (x) and (y), such as (y = kx^2) or (x = ky^2). The given equations describe a circular motion, not a parabolic one.
-
Option (d) a straight line: The equations (x = a \cos \omega t) and (y = a \sin \omega t) describe a circular motion, not a linear one. For a straight line, there would need to be a linear relationship between (x) and (y), such as (y = mx + c).
6. The displacement of a particle varies with time according to the relation
(a) The motion is oscillatory but not SHM
(b) The motion is SHM with amplitude
(c) The motion is SHM with amplitude
(d) The motion is SHM with amplitude
Show Answer
Answer (d) According to the question, the displacement
Hence, it is an equation of SHM with amplitude
-
(a) The motion is oscillatory but not SHM: The given displacement equation ( y = a \sin \omega t + b \cos \omega t ) can be rewritten in the form ( y = A \sin (\omega t + \phi) ), which is the standard form of simple harmonic motion (SHM). Therefore, the motion is indeed SHM, not just oscillatory.
-
(b) The motion is SHM with amplitude ( a + b ): The amplitude of SHM is determined by the resultant of the coefficients of the sine and cosine terms, which is given by ( \sqrt{a^2 + b^2} ). The sum ( a + b ) does not correctly represent the amplitude of the motion.
-
(c) The motion is SHM with amplitude ( a^2 + b^2 ): The amplitude of SHM is the square root of the sum of the squares of the coefficients of the sine and cosine terms, which is ( \sqrt{a^2 + b^2} ). The expression ( a^2 + b^2 ) represents the square of the amplitude, not the amplitude itself.
7. Four pendulums
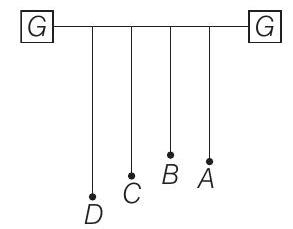
elastic support as shown in figure.
(a)
(b)
(c)
(d) All the four will oscillate with equal amplitude
Show Answer
Answer
(b) According to the question,
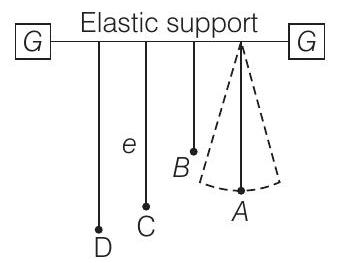
Through the elastic support the disturbance is transferred to all the pendulums.
So, amplitude of
Note In this problem, we have assumed that the support is perfectly elastic and there is no damping. Hence, oscillation is considered as undamped.
-
Option (a) is incorrect: Pendulum
-
Option (c) is incorrect: Pendulum
-
Option (d) is incorrect: The pendulums have different lengths, which means they have different time periods of oscillation. Only pendulums with the same length (and thus the same time period) will resonate and have maximum amplitude. Therefore, not all four pendulums will oscillate with equal amplitude.
8. Figure shows the circular motion of a particle. The radius of the circle, the period, sense of revolution and the initial position are indicated on the figure. The simple harmonic motion of the
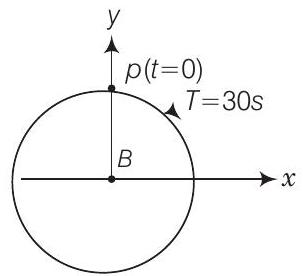
(a)
(b)
(c)
(d)
Show Answer
Answer
(a) Let angular velocity of the particle executing circular motion is
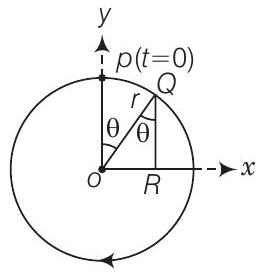
Clearly,
Now, we can write
Clearly, this equation represents SHM.
-
Option (b):
- This option is incorrect because it uses the cosine function instead of the sine function. The initial position of the particle at
- This option is incorrect because it uses the cosine function instead of the sine function. The initial position of the particle at
-
Option (c):
- This option is incorrect because it includes a phase shift of
- This option is incorrect because it includes a phase shift of
-
Option (d):
- This option is incorrect for two reasons: it uses the cosine function instead of the sine function, and it includes a phase shift of
- This option is incorrect for two reasons: it uses the cosine function instead of the sine function, and it includes a phase shift of
9. The equation of motion of a particle is
(a) periodic but not oscillatory
(b) periodic and oscillatory
(c) oscillatory but not periodic
(d) neither periodic nor oscillatory
Show Answer
Answer (c) As the given equation is
is a cosine function. Hence, it is an oscillatory motion.
Now, putting
where,
Hence, it is not periodic.
-
(a) periodic but not oscillatory: The given equation ( x = a \cos (\alpha t)^2 ) describes an oscillatory motion because it involves a cosine function, which inherently represents oscillations. Therefore, the motion cannot be periodic but not oscillatory.
-
(b) periodic and oscillatory: Although the motion is oscillatory due to the cosine function, it is not periodic. This is because substituting ( t + T ) into the equation does not yield the same function as ( x(t) ), indicating that there is no fixed period ( T ) for which the motion repeats itself.
-
(d) neither periodic nor oscillatory: The motion is oscillatory as it involves a cosine function, which represents oscillations. Therefore, the motion cannot be classified as neither periodic nor oscillatory.
10. A particle executing
(a)
(b)
(c)
(d)
Show Answer
Answer
(a) Let equation of an SHM is represented by
v =
-
Option (b)
-
Option (c)
-
Option (d)
Multiple Choice Questions (More Than One Options)
11. When a mass
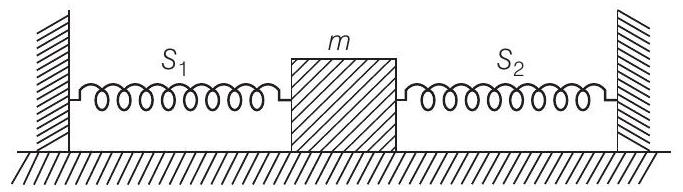
(a)
(b)
(c)
(d)
Show Answer
Thinking Process
To solve this question, we have to find equivalent spring constant of the system when mass is connected in between.
Answer (b)
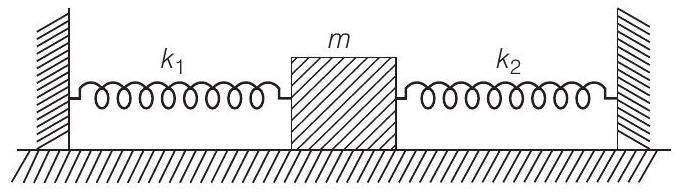
Consider the diagram, two springs can be considered as parallel.
Hence,
Time period of oscillation of the spring block- system
When the mass is connected to the two springs individually
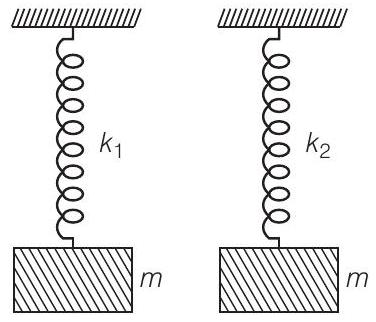
From Eqs. (i), (ii) and (iii),
Note Do not confuse with parallel and series combinations of springs.
-
Option (a) (v_1 + v_2): This option is incorrect because the frequencies of oscillation do not simply add up when two springs are combined in parallel. The correct approach involves considering the combined spring constant and the resulting frequency, which is derived from the square root of the sum of the squares of the individual frequencies.
-
Option (c) ((\frac{1}{v_1} + \frac{1}{v_2})^{-1}): This option is incorrect because it represents the formula for combining frequencies in series, not in parallel. When springs are in series, the effective spring constant is different, and the resulting frequency would be calculated differently.
-
Option (d) (\sqrt{v_1^2 - v_2^2}): This option is incorrect because the square root of the difference of the squares of the frequencies does not represent the correct physical relationship for the combined system. The correct formula involves the sum of the squares of the individual frequencies, not their difference.
12. The rotation of earth about its axis is
(a) periodic motion
(b) simple harmonic motion
(c) periodic but not simple harmonic motion
(d) non-periodic motion
Show Answer
Answer
The rotation of earth about its axis is periodic because it repeats after a regular interval of time.
The rotation of earth is obviously not a to and fro type of motion about a fixed point, hence its motion is not SHM.
-
Option (b) simple harmonic motion: The rotation of the Earth about its axis is not a simple harmonic motion because simple harmonic motion involves oscillatory motion where an object moves back and forth about an equilibrium position. The Earth’s rotation is a continuous circular motion, not an oscillatory one.
-
Option (d) non-periodic motion: The rotation of the Earth about its axis is not non-periodic because it occurs at regular intervals. Non-periodic motion does not repeat at regular intervals, whereas the Earth’s rotation repeats every 24 hours.
13. Motion of a ball bearing inside a smooth curved bowl, when released from a point slightly above the lower point is
(a) simple harmonic motion
(b) non-periodic motion
(c) periodic motion
(d) periodic but not
Show Answer
Answer
Consider the motion of the ball inside a smooth curved bowl.
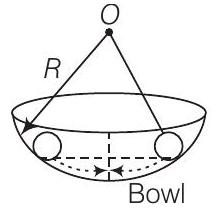
For small angular displacement or slightly released motion, it can be considered as angular SHM.
When the ball is at an angle of
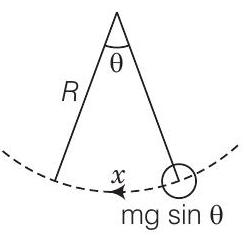
Hence ,motion is SHM
As motion is SHM, hence it must be periodic.
-
(b) non-periodic motion: The motion of the ball bearing inside a smooth curved bowl is periodic because it repeats itself after a fixed interval of time. Non-periodic motion does not repeat at regular intervals, which is not the case here.
-
(d) periodic but not
14. Displacement versus time curve for a particle executing SHM is shown in figure. Choose the correct statements.
(a) Phase of the oscillator is same at
(b) Phase of the oscillator is same at
(c) Phase of the oscillator is same at
(d) Phase of the oscillator is same at
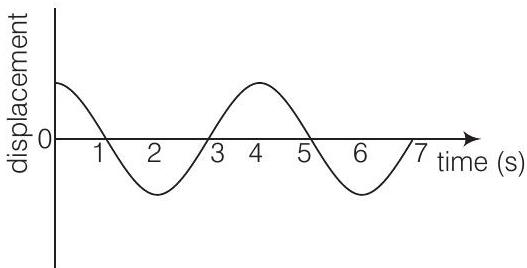
Show Answer
Answer
It is clear from the curve that points corresponding to
Similarly, points belong to
-
At ( t = 0 , \text{s} ) and ( t = 2 , \text{s} ), the particle is at different positions in its cycle. ( t = 0 , \text{s} ) corresponds to the maximum displacement, while ( t = 2 , \text{s} ) corresponds to the equilibrium position. Therefore, the phase of the oscillator is not the same at these times.
-
At ( t = 1 , \text{s} ) and ( t = 7 , \text{s} ), the particle is at different positions in its cycle. ( t = 1 , \text{s} ) corresponds to a point where the particle is moving towards the equilibrium position, while ( t = 7 , \text{s} ) corresponds to a point where the particle is moving away from the equilibrium position. Therefore, the phase of the oscillator is not the same at these times.
15. Which of the following statements is/are true for a simple harmonic oscillator?
(a) Force acting is directly proportional to displacement from the mean position and opposite to it
(b) Motion is periodic
(c) Acceleration of the oscillator is constant
(d) The velocity is periodic
Show Answer
Thinking Process
We have to write equation for the SHM and then find out velocity as well as acceleration corresponding to the displacement.
Answer
Let the equation for the SHM is
Clearly, it is a periodic motion because it involves sine function.
Velocity is also periodic because of cosine function.
Acceleration,
[
Force,
Hence, force acting is directly proportional to displacement from the mean position and opposite to it.
- Option (c): The acceleration of the oscillator is not constant. The acceleration in simple harmonic motion is given by ( A = -a \omega^2 \sin \omega t ), which is a sine function. Since the sine function varies with time, the acceleration is not constant.
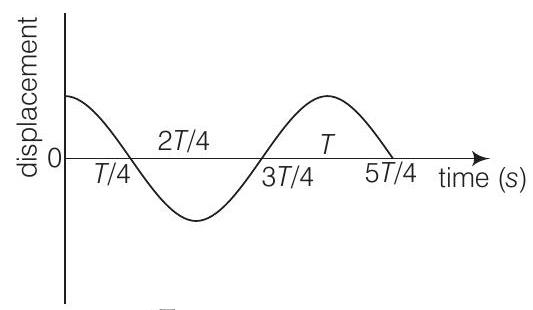
16. The displacement-time graph of a particle executing SHM is shown in figure. Which of the following statement is/are true?
Show Answer
Answer
Consider the diagram
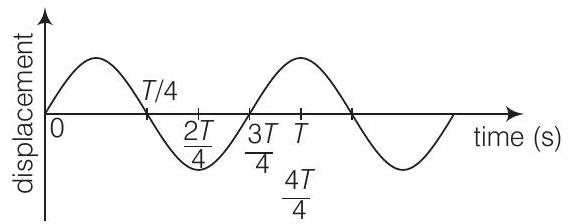
From the given diagram; it is clear that
(a) A
(b) At
(c) At
(d) At
- Reason for (d) being incorrect: At ( t = \frac{T}{2} ), the particle is at the extreme position. At this position, the kinetic energy (KE) is zero and the potential energy (PE) is maximum. Therefore, the statement that the potential energy (PE) is equal to the kinetic energy (KE) of oscillation at ( t = \frac{T}{2} ) is incorrect.
17. A body is performing SHM, then its
(a) average total energy per cycle is equal to its maximum kinetic energy
(b) average kinetic energy per cycle is equal to half of its maximum kinetic energy
(c) mean velocity over a complete cycle is equal to
(d) root mean square velocity is
Show Answer
Answer
Let the equation of a SHM is represented as
Assume mass of the body is
(a) Total mechanical energy of the body at any time
Kinetic energy at any instant
(b)
K =
[from Eq. (ii)]
(c) Velocity
[For a complete cycle]
(d)
-
Option (c) is incorrect because:
The mean velocity over a complete cycle of SHM is zero, not (\frac{2}{\pi}) times its maximum velocity. This is because the velocity is a sinusoidal function that averages out to zero over one complete cycle.
18. A particle is in linear simple harmonic motion between two points.
(a) The sign of velocity, acceleration and force on the particle when it is
(b) The sign of velocity of the particle at
(c) The sign of velocity, acceleration and force on the particle when it is
(d) The sign of acceleration and force on the particle when it is at points
Show Answer
Answer
Consider the diagram.
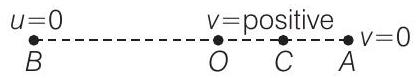
(a) When the particle is
In SHM, acceleration is always towards mean position
(b) When the particle is at
(c) When the particle is
(d) Acceleration is always towards mean position
-
(b) The sign of velocity of the particle at
Reason: When the particle is at
Very Short Answer Type Questions
19. Displacement versus time curve for a particle executing SHM is shown in figure. Identify the points marked at which (i) velocity of the oscillator is zero, (ii) speed of the oscillator is maximum.
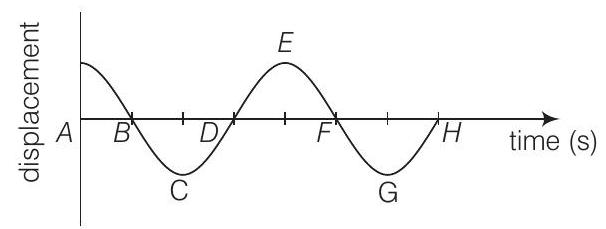
Show Answer
Answer In SHM y-t graph, zero displacement values correspond to mean position; where velocity of the oscillator is maximum.
Whereas the crest and troughs represent extreme positions, where displacement is maximum and velocity of the oscillator is minimum and is zero. Hence,
(a)
(b) speed is maximum at mean positions represented by
20. Two identical springs of spring constant
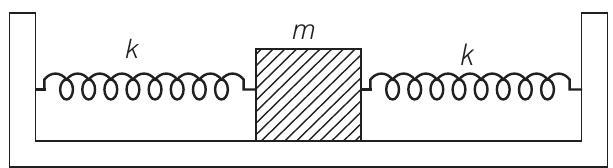
Show Answer
Answer
Consider the diagram in which the block is displaced right through
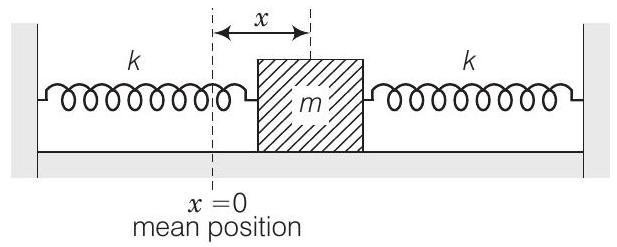
The right spring gets compressed by
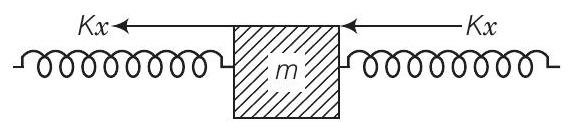
Hence, total force (restoring)
21. What are the two basic characteristics of a simple harmonic motion?
Show Answer
Answer The two basic characteristics of a simple harmonic motion
(i) Acceleration is directly proportional to displacement.
(ii) The direction of acceleration is always towards the mean position, that is opposite to displacement.
22. When will the motion of a simple pendulum be simple harmonic?
Show Answer
Answer Consider the diagram of a simple pendulum.
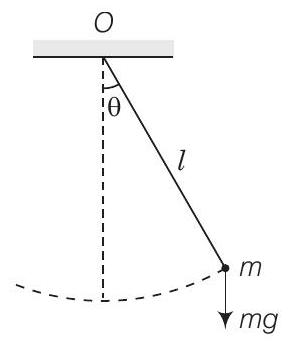
The bob is displaced through an angle
The restoring torque about the fixed point
If
Hence, motion of a simple pendulum is SHM for small angle of oscillations.
23. What is the ratio of maximum acceleration to the maximum velocity of a simple harmonic oscillator?
Show Answer
Thinking Process
We have to find velocity by differentiating the equation representing displacement and acceleration by differentiating the equation relating velocity and time.
Answer
Let
Velocity,
Acceleration,
From Eqs. (i) and (ii), we get
24. What is the ratio between the distance travelled by the oscillator in one time period and amplitude?
Show Answer
Answer The diagram represents
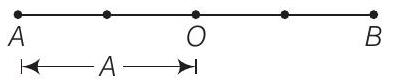
the motion of a particle executing SHM between
Total distance travelled while it goes from
Amplitude
Hence, ratio of distance and amplitude
25. In figure, what will be the sign of the velocity of the point
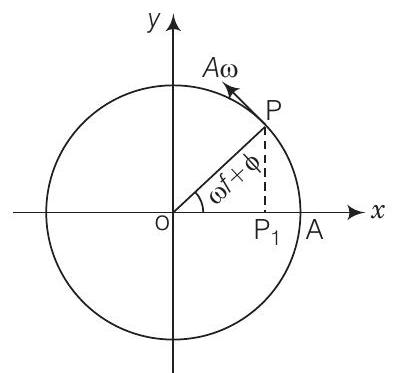
Show Answer
Answer
As the particle on reference circle moves in anti-iclockwise direction. The projection will move from
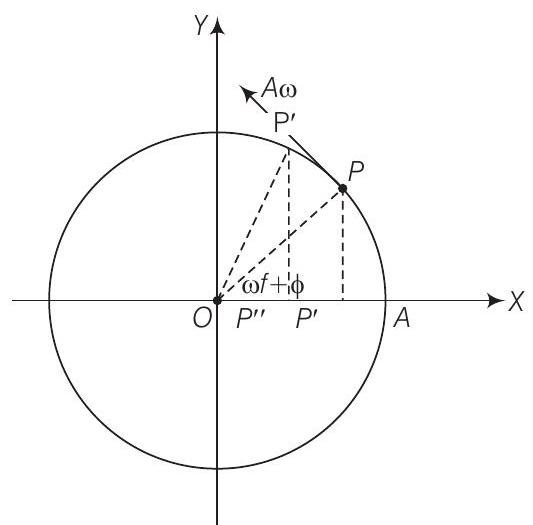
Hence, in the position shown the velocity is directed from
26. Show that for a particle executing SHM, velocity and displacement have a phase difference of
Show Answer
Answer Let us assume the displacement function of SHM
where,
or
Now, phase of displacement
Phase of velocity
27. Draw a graph to show the variation of
Show Answer
Answer Potential energy (PE) of a simple harmonic oscillator is
where,
When, PE is plotted against displacement
When
When
This is also parabola, if plot KE against displacement
Which is constant and does not depend on
Plotting under the above guidelines KE, PE and TE versus displacement

28. The length of a second’s pendulum on the surface of earth is
Show Answer
Answer
A second’s pendulum means a simple pendulum having time period
For a simple pendulum,
where,
On the surface of the moon,
But the acceleration due to gravity at moon is
Squaring Eq. (iii) and putting this value,
1 =
Short Answer Type Questions
29. Find the time period of mass
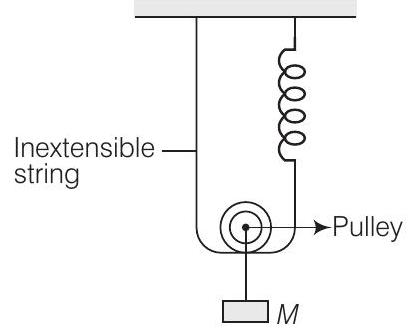
Show Answer
Thinking Process
To predict the nature of the motion, we have to displace the mass slightly and find the acceleration due to the restoring force.
Answer For the calculation purpose, in this situation we will neglect gravity because it is constant throughout and will not effect the net restoring force.
Let in the equilibrium position, the spring has extended by an amount
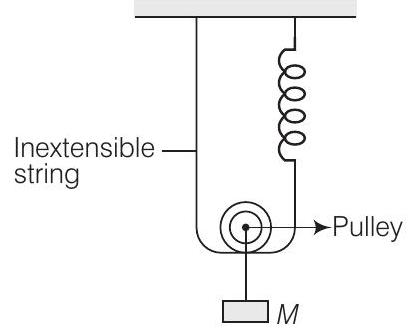
Now, if the mass is given a further displacement downwards by an amount
But, string is inextensible, hence the spring alone will contribute the total extension

Now force on the mass before bulling (in the
But
[where
When the mass is lowered down further by
But new spring length
Restoring force on the system.
Using Eqs. (i) and (ii), we get
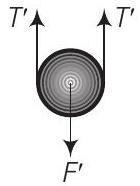
or
where,
(As,
k,
Hence, motion is SHM.
Comparing the above acceleration expression with standard SHM equation
30. Show that the motion of a particle represented by
Show Answer
Answer We have to convert the given combination of two harmonic functions to a single harmonic (sine or cosine) function.
Given, displacement function
Comparing with standard equation
Clearly, the function represents SHM with a period
31. Find the displacement of a simple harmonic oscillator at which its PE is half of the maximum energy of the oscillator.
Show Answer
Answer
Let us assume that the required displacement be
where,
Maximum energy of the oscillator
where,
Given,
or
Sign
32. A body of mass
Show Answer
Answer Given potential energy associated with the field
Now,
[We have assumed the field to be conservative]
Note The motion is SHM only for small oscillations and hence, the time period is valid only in case of small oscillations.
33. A mass of
Show Answer
Thinking Process
The spring-block system will perform SHM about the mean position with an amplitude of
Answer
Consider the diagram of the spring block system. It is a SHM with amplitude of
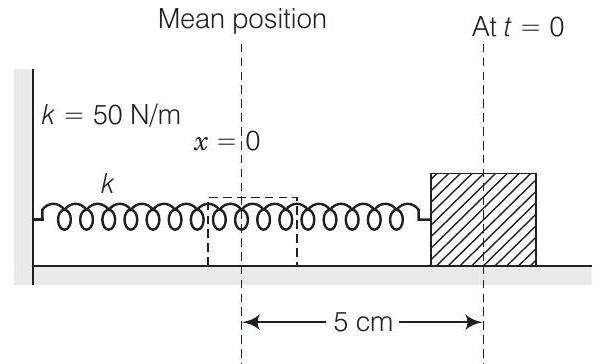
Given,
Assuming the displacement function
where,
But given at
or
Putting
we get,
where,
34. Consider a pair of identical pendulums, which oscillate with equal amplitude independently such that when one pendulum is at its extreme position making an angle of
Show Answer
Answer Consider the situations shown in the diagram (i) and (ii)
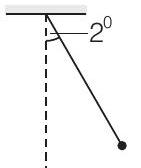
(i)

(ii)
Assuming the two pendulums follow the following functions of their angular displacements
and
As it is given that amplitude and time period being equal but phases being different.
Now, for first pendulum at any time
From Eq. (i), we get
[Right extreme]
or
Similarly, at the same instant
where
From Eq. (ii), then
From Eqs. (iv) and (iii), the difference in phases
or
Long Answer Type Questions
35. A person normally weighing
(a) Will there be any change in weight of the body, during the oscillation?
(b) If answer to part (a) is yes, what will be the maximum and minimum reading in the machine and at which position?
Show Answer
Answer In this case acceleration is variable. In accelerated motion, weight of body depends on the magnitude and direction of acceleration for upward or downward motion.
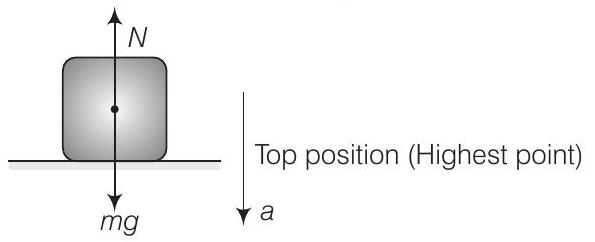
(a) Hence, the weight of the body changes during oscillations
(b) Considering the situation in two extreme positions, as their acceleration is maximum in magnitude.
we have,
When the platform is at the lowest position of its oscillation,
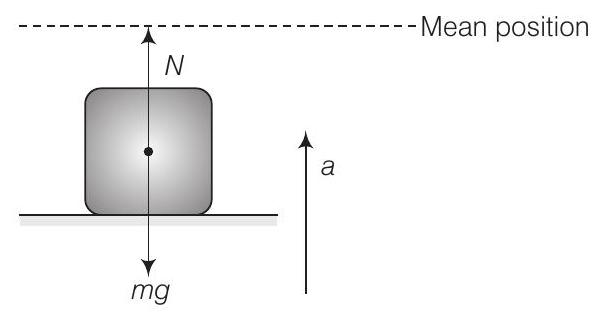
It is accelerating towards mean position that is vertically upwards.
Writing equation of motion
or
Putting the data
Now, the machine reads the normal reaction. It is clear that
maximum weight
(at lowest point)
minimum weight
(at top point)
36. A body of mass
(a) What is the amplitude of oscillation?
(b) Find the frequency of oscillation?
Show Answer
Thinking Process
When we have given the support of hand, net force
Answer (a) When the support of the hand is removed, the body oscillates about a mean position.
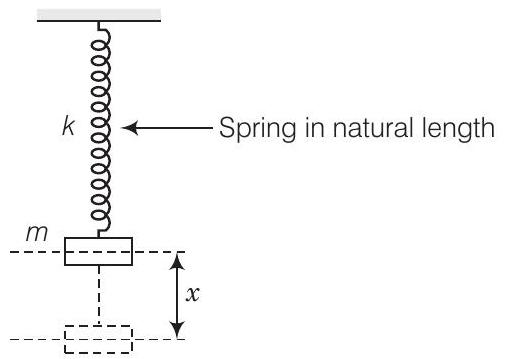
Suppose
Loss in PE of the block
where,
Gain in elastic potential energy of the spring
As the two are equal, conserving the mechanical energy,
we get,
Now, the mean position of oscillation will be, when the block is balanced by the spring.

If
Dividing Eq. (iii) by Eq. (iv),
But given
But the displacement of mass from the mean position to the position when spring attains its natural length is equal to amplitude of the oscillation.
where,
(b) Time period of the oscillating system depends on mass spring constant given by
It does not depend on the amplitude.
But from Eq. (iii),
Note The block during oscillation cannot go above the position it was released, as it is given that there is no velocity in the upward direction either by the system or external agent.
37. A cylindrical log of wood of height
where,
Show Answer
Thinking Process
To find the time period for the SHM displace the log from equilibrium position by a small displacement and then find the restoring force.
Answer Consider the diagram.

Let the log be pressed and let the vertical displacement at the equilibrium position be
When it is displaced by a further displacement
As displacement
where
So, the motion is SHM
Now,
Comparing with
38. One end of a
Show Answer
Answer Consider the diagram shown below
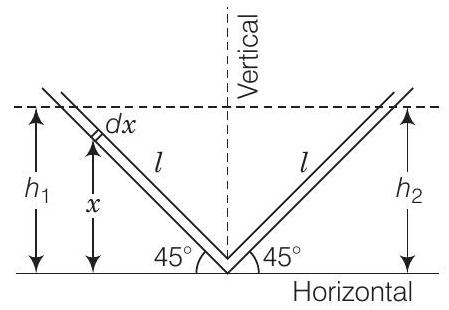
Let us consider an infinitesimal liquid column of length
If
PE of element
where
But,
In a similar way,
If due to pressure difference created y element of left side moves on the right side, then liquid present in the left arm
liquid present in the right arm
or
Changes in P E =
If
But
From Eqs. (iv) and (v),
System being conservative.
From Eq. (vi),
Differentiating both sides with respect to time
But,
[acceleration]
This is the standard differential equation for SHM of the form
39. A tunnel is dug through the centre of the earth. Show that a body of mass
Show Answer
Answer Consider the situation shown in the diagram.
The gravitational force on the particle at a distance
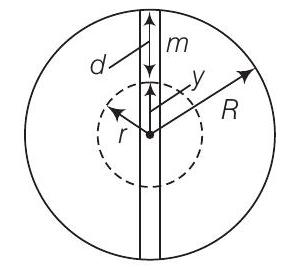
More clearly,
let
So,
From figure,
Force on body at
So, motion is SHM.
For time period, we can write Eq. (i)
As
Comparing with
40. A simple pendulum of time period
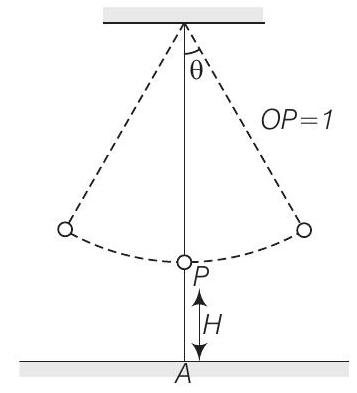
Show Answer
Thinking Process
We have to consider
Answer Consider the diagram.
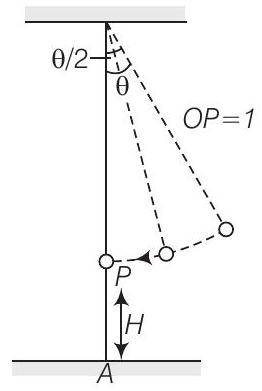
Let us assume
Given a seconds pendulum
At time
At
Negative sign shows that it is going left.
Thus, the linear velocity is
The vertical component is
and the horizontal component is
At the time it snaps, the vertical height is
Let the time required for fall be
Given that
The distance travelled in the
At the time of snapping, the bob was at a horizontal distance of
Thus, the distance of bob from










