Solution

A loaded die is thrown such that

$P(1)=P(2)=0.2, P(3)=P(5)=P(6)=0.1$ and $P(4)=0.3$ and die is thrown two times. Also given that:

$A=$ Same number each time and

$B=$ Total score is 10 or more.

$ \begin{aligned} & \text{ So, } P(A)=[P(1,1)+P(2,2)+P(3,3)+P(4,4)+P(5,5)+P(6,6)] \\ & =P(1) \cdot P(1)+P(2) \cdot P(2)+P(3) \cdot P(3)+P(4) \cdot P(4) \\ & +P(5) \cdot P(5)+P(6) \cdot P(6) \\ & =0.2 \times 0.2+0.2 \times 0.2+0.1 \times 0.1+0.3 \times 0.3+0.1 \times 0.1 \\ & +0.1 \times 0.1 \\ & =0.04+0.04+0.01+0.09+0.01+0.01=0.20 \end{aligned} $

Now $B=[(4,6),(6,4),(5,5),(5,6),(6,5),(6,6)]$

$P(B)=[P(4) \cdot P(6)+P(6) \cdot P(4)+P(5) \cdot P(5)+P(5) \cdot P(6)$ $+P(6) \cdot P(5)+P(6) \cdot P(6)$

$=0.3 \times 0.1+0.1 \times 0.3+0.1 \times 0.1+0.1 \times 0.1+0.1 \times 0.1$ $+0.1 \times 0.1$

$=0.03+0.03+0.01+0.01+0.01+0.01=0.10$

$A$ and $B$ both events will be independent if

$$ \begin{equation*} P(A \cap B)=P(A) \cdot P(B) \tag{i} \end{equation*} $$

Here,

$ (A \cap B)={(5,5),(6,6)} $

$ \therefore \quad P(A \cap B)=P(5,5)+P(6,6)=P(5) \cdot P(5)+P(6) \cdot P(6) $

$ =0.1 \times 0.1+0.1 \times 0.1=0.02 $

From eq. (i) we get

$ \begin{aligned} & 0.02=0.20 \times 0.10 \\ & 0.02=0.02 \end{aligned} $

Hence, $A$ and $B$ are independent events.

Solution

According to the solution of Q. 1 , we have

$A={(1,1),(2,2),(3,3),(4,4),(5,5),(6,6)}$

$\therefore n(A)=6$ and $n(S)=6 \times 6=36$

So, $P(A)=\frac{n(A)}{n(S)}=\frac{6}{36}=\frac{1}{6}$

and $\quad B={(4,6),(6,4),(5,5),(5,6),(6,5),(6,6)}$

$n(B)=6$ and $n(S)=36$

$\therefore \quad P(B)=\frac{n(B)}{n(S)}=\frac{6}{36}=\frac{1}{6}$

$A \cap B={(5,5),(6,6)}$

$\therefore P(A \cap B)=\frac{2}{36}=\frac{1}{18}$

Therefore, if $A$ and $B$ are independent, then

$P(A \cap B)=P(A) \cdot P(B)$

$\Rightarrow \quad \frac{1}{18} \neq \frac{1}{6} \times \frac{1}{6} \Rightarrow \frac{1}{18} \neq \frac{1}{36}$

Hence, $A$ and $B$ are not independent events.

Solution

We know that:

$A \cup B$ denotes that atleast one of the events occurs and $A \cap B$ denotes that the two events occur simultaneously.

$ \begin{matrix} \text{ So, } P(A \cup B) =P(A)+P(B)-P(A \cap B) \\ \Rightarrow 0.6 =P(A)+P(B)-0.3 \\ \Rightarrow 0.9 =P(A)+P(B) \\ \Rightarrow 0.9 =1-P(\overline{A})+1-P(\overline{B}) \\ \Rightarrow P(\overline{A})+P(\overline{B}) =2-0.9=1.1 \end{matrix} $

Hence, the required answer is 1.1.

Solution

Let red marbles be represented with $R$ and black marble with B. The following three conditions are possible, if atleast one of the three marbles drawn be black and the first marble is red.

(i) $E_1$ : II ball is black and III is red

(ii) $E_2$ : II ball is black and III is also black

(iii) $E_3:$ II ball is red and III is black

$ \begin{aligned} & \therefore \quad P(E_1)=P(R_1) \cdot P(B_1 / R_1) \cdot P(R_2 / R_1 B_1)=\frac{5}{8} \cdot \frac{3}{7} \cdot \frac{4}{6}=\frac{60}{336}=\frac{5}{28} \\ & \quad P(E_2)=P(R_1) \cdot P(B_1 / R_1) \cdot P(B_2 / R_1 B_1)=\frac{5}{8} \cdot \frac{3}{7} \cdot \frac{2}{6}=\frac{30}{336}=\frac{5}{56} \\ & \text{ and } P(E_3)=P(R_1) \cdot P(R_2 / R_1) \cdot B(B_1 / R_1 R_2)=\frac{5}{8} \cdot \frac{4}{7} \cdot \frac{3}{6}=\frac{60}{336}=\frac{5}{28} \\ & \therefore \quad P(E)=P(E_1)+P(E_2)+P(E_3)=\frac{5}{28}+\frac{5}{56}+\frac{5}{28}=\frac{25}{56} \end{aligned} $

Hence the required probability is $\frac{25}{56}$.

Solution

Two dice are thrown together

$\therefore n(S)=36$

$E=\text{ A total of } 4={(2,2),(1,3),(3,1)} \quad \therefore n(E)=3$

$F=\text{ A total of } 9 \text{ or more } $

$=\{(3,6),(6,3),(5,4),(4,5),(5,5),(4,6),(6,4),(5,6),(6,5),(6,6)\}$

$\therefore n(F)=10 ; G=\text{ A total divisible by } 5 $

$={(1,4),(4,1),(2,3),(3,2),(4,6),(6,4),(5,5)} \therefore n(G)=7$

Here, we see that $(E \cap F)=\phi$ and $(E \cap G)=\phi$

and $ (F \cap G)={(4,6),(6,4),(5,5)} $

$ \begin{aligned} \therefore \quad n(F & \cap G)=3 \text{ and }(E \cap F \cap G)=\phi \\ \therefore \quad P(E) & =\frac{n(E)}{n(S)}=\frac{3}{36}=\frac{1}{12} \\ P(F) & =\frac{n(F)}{n(S)}=\frac{10}{36}=\frac{5}{18} ; P(G)=\frac{n(G)}{n(S)}=\frac{7}{36} \\ P(F \cap G) & =\frac{3}{36}=\frac{1}{12} \text{ and } P(F) \cdot P(G)=\frac{5}{18} \cdot \frac{7}{36}=\frac{35}{648} \end{aligned} $

Since, $P(F \cap G) \neq P(F) . P(G)$

Hence, there is no pair of independent events.

Solution

We know that random variable $X$ takes values $0,1,2,3, \ldots, n$ is said to be binomial distribution having parameters $n$ and $p$, if the probability is given by $P(X=r)={ }^{n} C_r p^{r} q^{n-r}$, where $q=1-p$ and $r=0,1,2,3, \ldots$

Similarly in case of tossing a coin 3 times,

$n=3$ and $X$ has the values $0,1,2,3$ with $p=\frac{1}{2}, q=\frac{1}{2}$.

Hence, it is said to have a binomial distribution.

Solution

We have $P(A)=\frac{1}{2}, P(B)=\frac{1}{3}$ and $P(A \cap B)=\frac{1}{4}$

$ \begin{aligned} P(A^{\prime}) & =1-\frac{1}{2}=\frac{1}{2}, P(B^{\prime})=1-\frac{1}{3}=\frac{2}{3} \\ P(A^{\prime} \cap B^{\prime}) & =1-P(A \cup B)=1-[P(A)+P(B)-P(A \cap B)] \\ & =1-[\frac{1}{2}+\frac{1}{3}-\frac{1}{4}]=1-[\frac{6+4-3}{12}]=1-\frac{7}{12}=\frac{5}{12} \end{aligned} $

(i) $P(A / B)=\frac{P(A \cap B)}{P(B)}=\frac{1 / 4}{1 / 3}=\frac{3}{4}$

(ii) $P(B / A)=\frac{P(A \cap B)}{P(A)}=\frac{1 / 4}{1 / 2}=\frac{1}{2}$

(iii) $P(A^{\prime} / B)=\frac{P(A^{\prime} \cap B)}{P(B)}=\frac{P(B)-P(A \cap B)}{P(B)}$

$ =1-\frac{P(A \cap B)}{P(B)}=1-\frac{1 / 4}{1 / 3}=1-\frac{3}{4}=\frac{1}{4} $

(iv) $P(A^{\prime} / B^{\prime})=\frac{P(A^{\prime} \cap B^{\prime})}{P(B^{\prime})}=\frac{5 / 12}{2 / 3}=\frac{5}{12} \times \frac{3}{2}=\frac{5}{8}$

Solution

We have $P(A)=\frac{2}{5}, P(B)=\frac{1}{3}$ and $P(C)=\frac{1}{2}$

$ \begin{aligned} & P(A \cap C)=\frac{1}{5} \text{ and } P(B \cap C)=\frac{1}{4} \\ & \therefore \quad P(C / B)=\frac{P(B \cap C)}{P(B)}=\frac{1 / 4}{1 / 3}=\frac{3}{4} \\ & P(A^{\prime} \cap C^{\prime})=1-P(A \cup C) \\ & =1-[P(A)+P(C)-P(A \cap C)] \end{aligned} $

$ =1-[\frac{2}{5}+\frac{1}{2}-\frac{1}{5}]=1-\frac{7}{10}=\frac{3}{10} $

Hence, the required probabilities are $\frac{3}{4}$ and $\frac{3}{10}$.

Solution

Here,

$ P(E_1)=p_1 \text{ and } P(E_2)=p_2 $

(i)

$ p_1 p_2=P(E_1) \cdot P(E_2)=P(E_1 \cap E_2) $

So, $E_1$ and $E_2$ occur.

(ii)

$ (1-p_1) \cdot p_2=P(E_1)^{\prime} \cdot P(E_2)=P(E_1^{\prime} \cap E_2) $

So, $E_1$ does not occur but $E_2$ occurs.

(iii) $1-(1-p_1)(1-p_2)=1-P(E_1)^{\prime} P(E_2)^{\prime}=1-P(E_1^{\prime} \cap E_2^{\prime})$

$ =1-[1-P(E_1 \cup E_2)]=P(E_1 \cup E_2) $

So, either $E_1$ or $E_2$ or both $E_1$ and $E_2$ occur.

(iv)

$ \begin{aligned} p_1+p_2-2 p_1 p_2 & =P(E_1)+P(E_2)-2 P(E_1) \cdot P(E_2) \\ & =P(E_1)+P(E_2)-2 P(E_1 \cap E_2) \\ & =P(E_1 \cup E_2)-2 P(E_1 \cap E_2) \end{aligned} $

So, either $E_1$ or $E_2$ occurs but not both.

Solution

For a probability distribution, we know that if $P_i \geq 0$

(i) $\sum _{i=1}^{n} P_i=1 \Rightarrow k+k^{2}+2 k^{2}+k=1$

$ \begin{aligned} & \Rightarrow \quad 3 k^{2}+2 k-1=0 \Rightarrow 3 k^{2}+3 k-k-1=0 \\ & \Rightarrow \quad 3 k(k+1)-1(k+1)=0 \Rightarrow(3 k-1)(k+1)=0 \\ & \therefore \quad k=\frac{1}{3} \text{ and } k=-1 \end{aligned} $

But $k \geq 0 \quad \therefore k=\frac{1}{3}$

(ii) Mean of the distribution

$ E(X)=\sum _{i=1}^{n} X_i P_i=0.5 k+1 . k^{2}+1.5(2 k^{2})+2 k $

Solution

(i) To prove: $P(A)=P(A \cap B)+P(A \cap \overline{B})$ R.H.S. $=P(A \cap B)+P(A \cap \bar{{}B})$

$ \begin{aligned} & =P(A) \cdot P(B)+P(A) \cdot P(\overline{B})=P(A)[P(B)+P(\overline{B})] \\ & =P(A) \cdot 1 \\ & =P(A)=\text{ L.H.S. Hence proved. } \end{aligned} $

(ii) To prove: $P(A \cup B)=P(A \cap B)+P(A \cap \overline{B})+P(\overline{A} \cap B)$

R.H.S. $=P(A) \cdot P(B)+P(A) \cdot P(\bar{{}B})+P(\bar{{}A}) \cdot P(B)$

$ \begin{aligned} & =P(A) \cdot P(B)+P(A)[1-P(B)]+[1-P(A)] \cdot P(B) \\ & =P(A) \cdot P(B)+P(A)-P(A) \cdot P(B)+P(B)-P(A) \cdot P(B) \\ & =P(A)+P(B)-P(A \cap B) \\ & =P(A \cup B)=\text{ L.H.S. Hence proved. } \end{aligned} $

Solution

Given that: $X=0,1,2,3$

$\therefore P(X=r)={ }^{n} C_r p^{r} q^{n-r}$

where $n=3, p=\frac{1}{2}, q=\frac{1}{2}$ and $r=0,1,2,3$

$P(X=0)=\frac{1}{2} \times \frac{1}{2} \times \frac{1}{2}=\frac{1}{8} ; P(X=1)=3 \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2}=\frac{3}{8}$

$P(X=2)=3 \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2}=\frac{3}{8} ; P(X=3)=\frac{1}{2} \times \frac{1}{2} \times \frac{1}{2}=\frac{1}{8}$

Probability distribution table is:

$\begin{array}{|l|l|l|l|l|} \hline X & 0 & 1 & 2 & 3 \\ \hline P(X) & \frac{1}{8} & \frac{3}{8} & \frac{3}{8} & \frac{1}{8} \\ \hline \end{array}$

$ \begin{aligned} & E(X)=0+1 \times \frac{3}{8}+2 \times \frac{3}{8}+3 \times \frac{1}{8}=\frac{3}{8}+\frac{6}{8}+\frac{3}{8}=\frac{12}{8}=\frac{3}{2} \\ & E(X^{2})=0+1 \times \frac{3}{8}+4 \times \frac{3}{8}+9 \times \frac{1}{8}=\frac{3}{8}+\frac{12}{8}+\frac{9}{8}=\frac{24}{8}=3 \end{aligned} $

We know that $Var(X)=E(X^{2})-[E(X)]^{2}=3-(\frac{3}{2})^{2}=3-\frac{9}{4}=\frac{3}{4}$

$\therefore$ Standard deviation $=\sqrt{Var(X)}=\sqrt{\frac{3}{4}}=\frac{\sqrt{3}}{2}$.

Solution

Let $X$ be the random variable of profit per throw.

$\begin{array}{|l|l|l|l|l|} \hline X & -1 & 1 & 4 \\ \hline P(X) & \frac{1}{2} & \frac{1}{2} & \frac{1}{6} \\ \hline \end{array}$

Since, she loses ₹ 1 for getting any of 2, 4, 5 .

So, $P(X=-1)=\frac{1}{6}+\frac{1}{6}+\frac{1}{6}=\frac{3}{6}=\frac{1}{2}$

$ \begin{matrix} P(X=1)=\frac{1}{6}+\frac{1}{6}=\frac{2}{6}=\frac{1}{3} & (\because \text{ die showing } 1 \text{ or } 6) \\ P(X=4)=\frac{1}{6} & (\because \text{ die shows only a } 3) \end{matrix} $

Player’s expected profit $=\sum p_1 x_i$

$ =-1 \times \frac{1}{2}+1 \times \frac{1}{3}+4 \times \frac{1}{6}=-\frac{1}{2}+\frac{1}{3}+\frac{2}{3}=\frac{1}{2}=₹ 0.50 $

Solution

The dice is thrown three times

$\therefore$ Sample space $n(S)=(6)^{3}=216$

Let $E_1$ be the event when the sum of numbers on the dice was six and $E_2$ be the event when three two’s occur.

$ \begin{aligned} \Rightarrow E_1=\{(1,1,4),(1,2,3),(1,3,2),(1,4,1),(2,1,3),(2,2,2),(2,3,1),(3,1,2),\\(3,2,1),(4,1,1)\}[\therefore E_2=\{2,2,2\}] \end{aligned} $

$\begin{aligned}\Rightarrow n(E_1)=10 \text{ and } n(E_2)=1\end{aligned}$

$\begin{aligned}\therefore P(E_2 / E_1)=\frac{P(E_1 \cap E_2)}{P(E_1)}=\frac{1 / 216}{10 / 216}=\frac{1}{10} . \end{aligned} $

Solution

Let $X$ be the random variable where $X=0,500,2000$ and 3000

$\begin{array}{|l|l|l|l|l|l|} \hline X & 0 & 500 & 2000 & 3000 \\ \hline P(X) & \frac{9995}{10000} & \frac{3}{10000} & \frac{1}{10000} & \frac{1}{10000} \\ \hline \end{array}$

Hence, expectation is ₹ 0.65 .

Solution

Let $W_1$ and $W_2$ be two bags containing ( $4 W, 5 B)$ and (9 W, 7 B) balls respectively.

Let $E_1$ be the event that the

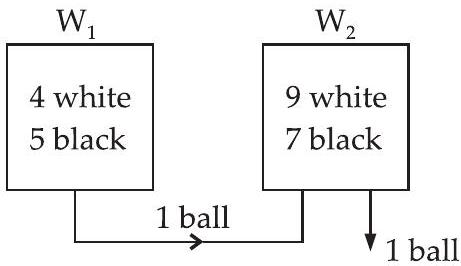
transferred ball from the bag

$W_1$ to $W_2$ is white and $E_2$ the event that the transferred ball is black.

And $E$ be the event that the ball drawn from the second bag is white.

$ \begin{aligned} \therefore P(E / E_1) & =\frac{10}{17}, \quad P(E / E_2)=\frac{9}{17} \\ P(E_1) & =\frac{4}{9} \text{ and } P(E_2)=\frac{5}{9} \\ \therefore \quad P(E) & =P(E_1) \cdot P(E / E_1)+P(E_2) \cdot P(E / E_2) \\ & =\frac{4}{9} \times \frac{10}{17}+\frac{5}{9} \times \frac{9}{17}=\frac{40}{153}+\frac{45}{153}=\frac{85}{153}=\frac{5}{9} \end{aligned} $

Hence, the required probability is $\frac{5}{9}$.

Solution

Given that $bag I={3 B, 2 W}$ and $\quad$ bag II $={2 B, 4 W}$

Let $E_1=$ The event that bag I is selected

$ E_2=\text{ The event that bag II is selected } $

and $E=$ The event that a black ball is selected

$ \begin{aligned} \therefore \quad P(E_1) & =\frac{1}{2}, P(E_2)=\frac{1}{2}, P(E _{/} E_1)=\frac{3}{5} \text{ and } P(E_2 E_2)=\frac{1}{3} \\ P(E) & =P(E_1) \cdot P(E / E_1)+P(E_2) \cdot P(E / E_2) \\ & =\frac{1}{2} \times \frac{3}{5}+\frac{1}{2} \times \frac{1}{3}=\frac{3}{10}+\frac{1}{6}=\frac{9+5}{30}=\frac{14}{30}=\frac{7}{15} \end{aligned} $

Hence, the required probability is $\frac{7}{15}$.

Solution

Given that the box has 5 blue and 4 red balls.

Let $E_1$ be the event that first ball drawn is blue $E_2$ be the event that first ball drawn is red

and $E$ is the event that second ball drawn is blue.

$ \begin{aligned} \therefore \quad P(E) & =P(E_1) \cdot P(E / E_1)+P(E_2) \cdot P(E / E_2) \\ & =\frac{5}{9} \times \frac{4}{8}+\frac{4}{9} \times \frac{5}{8}=\frac{20}{72}+\frac{20}{72}=\frac{40}{72}=\frac{5}{9} \end{aligned} $

Hence, the required probability is $\frac{5}{9}$.

Solution

Let $E_1, E_2, E_3$ and $E_4$ be the events that first, second, third and fourth card is King respectively.

$\therefore P(E_1 \cap E_2 \cap E_3 \cap E_4)$

$=P(E_1) \cdot P(E_2 / E_1) \cdot P[\frac{E_3}{(E_1 \cap E_2)}] \cdot P[\frac{E_4}{(E_1 \cap E_2 \cap E_3 \cap E_4)}]$

$=\frac{4}{52} \times \frac{3}{51} \times \frac{2}{50} \times \frac{1}{49}=\frac{24}{52 \cdot 51 \cdot 50 \cdot 49}=\frac{1}{13 \cdot 17 \cdot 25 \cdot 49}=\frac{1}{27075}$

Hence, the required probability is $\frac{1}{27075}$.

Solution

Here, $p=\frac{1}{6}+\frac{1}{6}+\frac{1}{6}=\frac{1}{2} \Rightarrow q=1-\frac{1}{2}=\frac{1}{2}$ and $n=5$

$ \begin{aligned} \therefore \quad P(x & =r)={ }^{n} C_r p^{r} q^{n-r} \\ & ={ }^{5} C_3(\frac{1}{2})^{3}(\frac{1}{2})^{5-3}=\frac{5 !}{3 ! 2 !} \cdot(\frac{1}{2})^{3} \cdot(\frac{1}{2})^{2}=10 \cdot \frac{1}{8} \cdot \frac{1}{4}=\frac{5}{16} \end{aligned} $

Hence, the required probability is $\frac{5}{16}$.

Solution

Here, $n=10, p=\frac{1}{2}, q=-\frac{1}{2}=\frac{1}{2}$

$ \begin{aligned} & P(X \geq 8)=P(x=8)+P(x=9)+P(x=10) \\ & ={ }^{10} C_8(\frac{1}{2})^{8}(\frac{1}{2})^{10-8}+{ }^{10} C_9(\frac{1}{2})^{9}(\frac{1}{2})^{10-9}+{ }^{10} C _{10}(\frac{1}{2})^{10}(\frac{1}{2})^{0} \\ & =\frac{10 !}{8 ! 2 !} \cdot(\frac{1}{2})^{8} \cdot(\frac{1}{2})^{2}+\frac{10 !}{9 ! 1 !}(\frac{1}{2})^{9}(\frac{1}{2})+(\frac{1}{2})^{10} \\ & =45 \cdot(\frac{1}{2})^{10}+10 \cdot(\frac{1}{2})^{10}+(\frac{1}{2})^{10}=(\frac{1}{2})^{10} \cdot(45+10+1) \\ & =56(\frac{1}{2})^{10}=56 \times \frac{1}{1024}=\frac{7}{128} \end{aligned} $

Hence, the required probability is $\frac{7}{128}$.

Solution

Here $n=7, \quad p=0.25=\frac{25}{100}=\frac{1}{4} \quad$ and $q=1-\frac{1}{4}=\frac{3}{4}$

$ \begin{aligned} P(X \geq 2) & =1-[P(X=0)+P(X=1)] \\ & =1-[{ }^{7} C_0(\frac{1}{4})^{0}(\frac{3}{4})^{7}+{ }^{7} C_1(\frac{1}{4})^{1}(\frac{3}{4})^{6}] \\ & =1-[(\frac{3}{4})^{7}+\frac{7}{4}(\frac{3}{4})^{6}]=1-(\frac{3}{4})^{6}(\frac{3}{4}+\frac{7}{4}) \\ & =1-(\frac{3}{4})^{6}(\frac{10}{4})=1-\frac{729}{4096} \times \frac{10}{4}=1-\frac{7290}{16384} \\ & =\frac{16384-7290}{16384}=\frac{9094}{16384}=\frac{4547}{8192} \end{aligned} $

Hence, the required probability is $\frac{4547}{8192}$.

Solution

Probability of defective watch out of 100 watches $=\frac{10}{100}=\frac{1}{10}$.

Here, $n=8, p=\frac{1}{10}$ and $q=1-\frac{1}{10}=\frac{9}{10}$ and $r \geq 1$

$P(X \geq 1)=1-P(x=0)=1-{ }^{8} C_0(\frac{1}{10})^{0}(\frac{9}{10})^{8-0}=1-(\frac{9}{10})^{8}$

Hence, the required probability is $1-(\frac{9}{10})^{8}$.

Solution

Here, we have

$\begin{array}{|l|l|l|l|l|l|} \hline x & 0 & 1 & 2 & 3 & 4 \\ \hline y & 0.1 & 0.25 & 0.3 & 0.2 & 0.15 \\ \hline \end{array}$

$=E(X^{2})-[E(X)]^{2}$ |

where $E(X)=\sum _{i=1}^{n} x_i p_i$ and $E(X^{2})=\sum _{i=1}^{n} p_i x_i^{2}$

$\therefore \quad E(X)=0 \times 0.1+1 \times 0.25+2 \times 0.3+3 \times 0.2+4 \times 0.15$

$=0+0.25+0.6+0.6+0.6=2.05$

$E(X^{2})=0 \times 0.1+1 \times 0.25+4 \times 0.3+9 \times 0.2+16 \times 0.15$

$=0+0.25+1.2+1.8+2.40=5.65$

(i) $V(\frac{X}{2})=\frac{1}{4} V(X)=\frac{1}{4}[5.65-(2.05)^{2}]=\frac{1}{4}[5.65-4.2025]$

$=\frac{1}{4} \times 1.4475=0.361875$

$[\because V(\frac{X}{2})=\frac{1}{4} V(X)]$

(ii) $\quad Var(X)=1.4475$

Solution

(i) We know that $P(0)+P(1)+P(2)+P(3)=1$

$ \begin{matrix} \Rightarrow k+\frac{k}{2}+\frac{k}{4}+\frac{k}{8} =1 \\ \Rightarrow \quad \frac{8 k+4 k+2 k+k}{8} =1 \Rightarrow 15 k=8 \\ \therefore \quad k =\frac{1}{15} \end{matrix} $

(ii)

$ \begin{aligned} P(X \leq 2) & =P(X=0)+P(X=1)+P(X=2) \\ & =k+\frac{k}{2}+\frac{k}{4}=\frac{7 k}{4}=\frac{7}{4} \times \frac{8}{15}=\frac{14}{15} \end{aligned} $

and $P(X>2)=P(X=3)=\frac{k}{8}=\frac{1}{8} \times \frac{8}{15}=\frac{1}{15}$

(iii) $P(X \leq 2)+P(X>2)=\frac{14}{15}+\frac{1}{15}=\frac{14+1}{15}=\frac{15}{15}=1$.

Solution

We know that: Standard deviation (S.D.) $=\sqrt{\text{ Variance }}$

$ \begin{aligned} & \therefore \quad Var(X)=E(X^{2})-[E(X)]^{2} \\ & E(X)=2 \times 0.2+3 \times 0.5+4 \times 0.3=0.4+1.5+1.2=3.1 \\ & E(X^{2})=4 \times 0.2+9 \times 0.5+16 \times 0.3=0.8+4.5+4.8=10.1 \\ & V(X)=10.1-(3.1)^{2}=10.1-9.61=0.49 \\ & \therefore \quad \text{ S.D. }=\sqrt{Var(X)}=\sqrt{0.49}=0.7 \end{aligned} $

Solution

Here, random variable $X=0,1,2$

$ \begin{aligned} P(4) & =\frac{1}{10}, P(\overline{4})=1-\frac{1}{10}=\frac{9}{10} \\ P(X=0) & =P(\overline{4}) \cdot P(\overline{4})=\frac{9}{10} \times \frac{9}{10}=\frac{81}{100} \\ P(X=1) & =P(\overline{4}) \cdot P(4)+P(4) \cdot P(\overline{4})=\frac{9}{10} \times \frac{1}{10}+\frac{1}{10} \times \frac{9}{10}=\frac{18}{100} \end{aligned} $

$P(X=2)=P(4) \cdot P(4)=\frac{1}{10} \times \frac{1}{10}=\frac{1}{100}$

$\begin{array}{|l|l|l|l|} \hline X & 0 & 1 & 2 \\ \hline P(X) & \frac{81}{100} & \frac{18}{100} & \frac{1}{100} \\ \hline \end{array}$

We know that $V(X)=E(X^{2})-[E(X)]^{2}$

$ \begin{aligned} E(X) & =0 \times \frac{81}{100}+1 \times \frac{18}{100}+\frac{2}{100}=\frac{20}{100}=\frac{1}{5} \\ E(X^{2}) & =0 \times \frac{81}{100}+1 \times \frac{18}{100}+4 \times \frac{1}{100}=\frac{22}{100}=\frac{11}{50} \\ \therefore \quad Var(X) & =\frac{11}{50}-(\frac{1}{5})^{2}=\frac{11}{50}-\frac{1}{25}=\frac{9}{50}=0.18 \end{aligned} $

Hence, the required variance $=0.18$.

Solution

Here, we have $X=0,1,2,3 \quad[\because$ die is thrown 3 times] and $p=\frac{1}{6}, q=\frac{5}{6}$

$\therefore P(X=0)=P(not 2) \cdot P(not 2) \cdot P(not 2)=\frac{5}{6} \cdot \frac{5}{6} \cdot \frac{5}{6}=\frac{125}{216}$

$P(X=1)=P(2) \cdot P(not 2) \cdot P(not 2)+P(not 2) \cdot P(2) \cdot P(not 2)$

$+P($ not 2$) . P(not 2) \cdot P(2)$

$=\frac{1}{6} \cdot \frac{5}{6} \cdot \frac{5}{6}+\frac{5}{6} \cdot \frac{1}{6} \cdot \frac{5}{6}+\frac{5}{6} \cdot \frac{5}{6} \cdot \frac{1}{6}=\frac{25}{216}+\frac{25}{216}+\frac{25}{216}=\frac{75}{216}$

$P(X=2)=P(2) \cdot P(2) \cdot P(not 2)+P(2) \cdot P(not 2) \cdot P(2)$

$+P($ not 2$) \cdot P(2) \cdot P(2)$

$=\frac{1}{6} \cdot \frac{1}{6} \cdot \frac{5}{6}+\frac{1}{6} \cdot \frac{5}{6} \cdot \frac{1}{6}+\frac{5}{6} \cdot \frac{1}{6} \cdot \frac{1}{6}=\frac{5}{216}+\frac{5}{216}+\frac{5}{216}=\frac{15}{216}$

$P(X=3)=P(2) \cdot P(2) \cdot P(2)=\frac{1}{6} \cdot \frac{1}{6} \cdot \frac{1}{6}=\frac{1}{216}$

Now $E(X)=\sum _{i=1}^{n} p_i x_i$

$ \begin{aligned} & i=1 \\ & 0 \times \frac{125}{216}+1 \times \frac{75}{216}+2 \times \frac{15}{216}+3 \times \frac{1}{216} \\ = & 0+\frac{75}{216}+\frac{30}{216}+\frac{3}{216}=\frac{75+30+3}{216}=\frac{108}{216}=\frac{1}{2} \end{aligned} $

Hence, the required expectation is $\frac{1}{2}$.

Solution

Given that: for the first die, $P(6)=\frac{1}{2}$ and $P(\overline{6})=1-\frac{1}{2}=\frac{1}{2}$

$\Rightarrow P(1)+P(2)+P(3)+P(4)+P(5)=\frac{1}{2}$

But $P(1)=P(2)=P(3)=P(4)=P(5)$

$\therefore 5 . P(1)=\frac{1}{2} \Rightarrow P(1)=\frac{1}{10}$ and $P(\overline{1})=1-\frac{1}{10}=\frac{9}{10}$

For the second die, $P(1)=\frac{2}{5}$ and $P(\overline{1})=1-\frac{2}{5}=\frac{3}{5}$

Let $X$ be the number of one’s seen

$ \begin{aligned} \therefore X=0,1,2 \\ \Rightarrow \quad \begin{aligned} P(X=0) =P(\overline{1}) \cdot P _{II}(\overline{1})=\frac{9}{10} \cdot \frac{3}{5}=\frac{27}{50}=0.54 \\ P(X=1) =P(\overline{1}) \cdot P_I(1)+P(1) \cdot P _{(\overline{1})} \\ =\frac{9}{10} \cdot \frac{2}{5}+\frac{1}{10} \cdot \frac{3}{5}=\frac{18+3}{50}=\frac{21}{50}=0.42 \\ P(X=2) =\underset{I \hspace{2mm} II}{P(1) \cdot P(1)}=\frac{1}{10} \cdot \frac{2}{5}=\frac{2}{50}=0.04 \end{aligned} \end{aligned} $

Hence, the required probability distribution is

$\begin{array}{|l|l|l|l|} \hline X & 0 & 1 & 2 \\ \hline P(X) & 0.54 & 0.42 & 0.04 \\ \hline \end{array}$

Solution

First probability distribution is given by

$\begin{array}{|l|l|l|l|l|} \hline X & 0 & 1 & 2 & 3 \\ \hline P(X) & \frac{1}{5} & \frac{2}{5} & \frac{1}{5} & \frac{1}{5} \\ \hline \end{array}$

We know that, $E(X)=\sum _{i=1}^{n} P_i X_i$

$\Rightarrow E(X)=0 \cdot \frac{1}{5}+1 \cdot \frac{2}{5}+2 \cdot \frac{1}{5}+3 \cdot \frac{1}{5}=0+\frac{2}{5}+\frac{2}{5}+\frac{3}{5}=\frac{7}{5}$

For the second probability distribution,

$\begin{array}{|l|l|l|l|l|} \hline (Y) & 0 & 1 & 2 & 3 \\ \hline P(Y) & \frac{1}{5} & \frac{3}{10} & \frac{2}{5} & \frac{1}{10} \\ \hline \end{array}$

$ \begin{aligned} E(Y^{2}) & =0 \cdot \frac{1}{5}+1 \cdot \frac{3}{10}+4 \cdot \frac{2}{5}+9 \cdot \frac{1}{10} \\ & =0+\frac{3}{10}+\frac{8}{5}+\frac{9}{10}=\frac{28}{10}=\frac{14}{5} \end{aligned} $

Now $E(Y^{2})=\frac{14}{5}$ and $2 E(X)=2 \cdot \frac{7}{5}=\frac{14}{5}$

Hence, $E(Y^{2})=2 E(X)$.

Solution

Let $X$ be the random variable denoting a bulb to be defective.

Here, $n=10, p=\frac{1}{50}, q=1-\frac{1}{50}=\frac{49}{50}$

We know that $P(X=r)={ }^{n} C_r p^{r} q^{n-r}$

(i) None of the bulbs is defective, i.e., $r=0$

$ P(x=0)={ }^{10} C_0(\frac{1}{50})^{0}(\frac{49}{50})^{10-0}=(\frac{49}{50})^{10} $

(ii) Exactly two bulbs are defective

$ \begin{aligned} \therefore \quad P(x=2) & ={ }^{10} C_2(\frac{1}{50})^{2}(\frac{49}{50})^{10-2} \\ & =45 \cdot \frac{(49)^{8}}{(50)^{10}}=45 \times(\frac{1}{50})^{10} \times(49)^{8} \end{aligned} $

(iii) More than 8 bulbs work properly

We can say that less than 2 bulbs are defective

$ P(x<2)=P(x=0)+P(x=1) $

$={ }^{10} C_0(\frac{1}{50})^{0}(\frac{49}{50})^{10}+{ }^{10} C_1(\frac{1}{50})^{1}(\frac{49}{50})^{9}=(\frac{49}{50})^{10}+\frac{1}{5}(\frac{49}{50})^{9}$

$ =(\frac{49}{50})^{9}(\frac{49}{50}+\frac{1}{5})=(\frac{49}{50})^{9}(\frac{59}{50})=\frac{59(49)^{9}}{(50)^{10}} . $

Solution

Let $E_1=$ Event that the coin is fair

$ E_2=\text{ Event that the coin is 2-headed } $

and $H=$ Event that the tossed coin gets head.

$ P(E_1)=\frac{1}{2}, \quad P(E_2)=\frac{1}{2}, \quad P(H / E_1)=\frac{1}{2}, \quad P(H / E_2)=1 $

$\therefore$ Using Bayes’ Theorem, we get

$ \begin{aligned} P(E_1 / H) & =\frac{P(E_1) \cdot P(H / E_1)}{P(E_1) \cdot P(H / E_1)+P(E_2) \cdot P(H / E_2)} \\ & =\frac{\frac{1}{2} \cdot \frac{1}{2}}{\frac{1}{2} \cdot \frac{1}{2}+\frac{1}{2} \cdot 1}=\frac{\frac{1}{4}}{\frac{1}{4}+\frac{1}{2}}=\frac{\frac{1}{3}}{\frac{3}{4}}=\frac{1}{3} \end{aligned} $

Hence the required probability is $\frac{1}{3}$.

Solution

Let $E_1=$ The event that a person selected is of blood group $O$ $E_2=$ The event that the person selected is of other group and $H=$ The event that selected person is left handed

$ \begin{matrix} \therefore \quad P(E_1) & =0.30 \text{ and } & P(E_2)=0.70 \\ P(H / E_1) & =0.06 & P(H / E_2)=0.10 \end{matrix} $

So, from Bayes’ Theorem

$ \begin{aligned} & \quad P(\frac{E_1}{H})=\frac{P(E_1) \cdot P(H / E_1)}{P(E_1) \cdot P(H / E_1)+P(E_2) \cdot P(H / E_2)} \\ & =\frac{0.30 \times 0.06}{0.30 \times 0.06+0.70 \times 0.10}=\frac{0.018}{0.018+0.070}=\frac{0.018}{0.088}=\frac{9}{44} \\ & \text{ Hence, the required probability is } \frac{9}{44} . \end{aligned} $

Solution

Given that: $S={1,2,3, \ldots, n}$

$ \therefore \quad P(r \leq p / s \leq p)=\frac{P(P \cap S)}{P(S)}=\frac{p-1}{n} \times \frac{n}{n-1}=\frac{p-1}{n-1} $

Hence, the required probability is $\frac{p-1}{n-1}$.

Solution

Let $X$ be the random variable scores when a die is thrown twice.

and

$ \begin{aligned} X & =1,2,3,4,5,6 \\ S & =\{(1,1),(1,2),(2,1),(2,2),(1,3),(2,3),(3,1),(3,2),(3,3),(3,4),(3,5),..,(6,6)\} \end{aligned} $

So, $\quad P(X=1)=\frac{1}{6} \cdot \frac{1}{6}=\frac{1}{36}$

$ \begin{aligned} & P(X=2)=\frac{1}{6} \cdot \frac{1}{6}+\frac{1}{6} \cdot \frac{1}{6}+\frac{1}{6} \cdot \frac{1}{6}=\frac{3}{36} \\ & P(X=3)=\frac{1}{6} \cdot \frac{1}{6}+\frac{1}{6} \cdot \frac{1}{6}+\frac{1}{6} \cdot \frac{1}{6}+\frac{1}{6} \cdot \frac{1}{6}+\frac{1}{6} \cdot \frac{1}{6}=\frac{5}{36} \end{aligned} $

Similarly

$ P(X=4)=\frac{7}{36} ; P(X=5)=\frac{9}{36} \text{ and } P(X=6)=\frac{11}{36} $

So, the required distribution is

$\begin{array}{|l|l|l|l|l|l|l|} \hline X & 1 & 2 & 3 & 4 & 5 & 6 \\ \hline P(X) & \frac{1}{36} & \frac{3}{36} & \frac{5}{36} & \frac{7}{36} & \frac{9}{36} &\frac{11}{36} \\ \hline \end{array}$

Now, the mean $E(X)=\sum _{i=1}^{n} x_i p_i$

$ \begin{aligned} & =1 \times \frac{1}{36}+2 \times \frac{3}{36}+3 \times \frac{5}{36}+4 \times \frac{7}{36}+5 \times \frac{9}{36}+6 \times \frac{11}{36} \\ & =\frac{1}{36}+\frac{6}{36}+\frac{15}{36}+\frac{28}{36}+\frac{45}{36}+\frac{66}{36}=\frac{161}{36} \end{aligned} $

Hence, the required mean $=\frac{161}{36}$.

Solution

Given that: $\quad X=0,1,2$ and $P(X)$ at $X=0$ and 1 is $p$. Let $P(X)$ at $X=2$ is $x$

$\Rightarrow \quad p+p+x=1 \quad \Rightarrow \quad x=1-2 p$

Now we have the following distributions.

$\begin{array}{|l|l|l|l|} \hline X & 0 & 1 & 2 \\ \hline P(X) & p & p & 1-2p \\ \hline \end{array}$

$\therefore \quad E(X)=0 . p+1 . p+2(1-2 p)=p+2-4 p=2-3 p$

and $\quad E(X^{2})=0 . p+1 . p+4(1-2 p)=p+4-8 p=4-7 p$

Given that: $\quad E(X^{2})=E(X)$

$\therefore \quad 4-7 p=2-3 p \Rightarrow 4 p=2 \Rightarrow p=\frac{1}{2}$

Hence, the required value of $p$ is $\frac{1}{2}$.

Solution

We know that:

$ \begin{aligned} & \text{ Variance }(X)=E(X^{2})-[E(X)]^{2} \\ & E(X)=\sum _{i=1}^{n} p_i x_i \\ &=0 \times \frac{1}{6}+1 \times \frac{5}{18}+2 \times \frac{2}{9}+3 \times \frac{1}{6}+4 \times \frac{1}{9}+5 \times \frac{1}{18} \\ &=0+\frac{5}{18}+\frac{4}{9}+\frac{3}{6}+\frac{4}{9}+\frac{5}{18}=\frac{5+8+9+8+5}{18}=\frac{35}{18} \\ & E(X^{2})=0 \times \frac{1}{6}+1 \times \frac{5}{18}+4 \times \frac{2}{9}+9 \times \frac{1}{6}+16 \times \frac{1}{9}+25 \times \frac{1}{18} \\ &=\frac{5}{18}+\frac{8}{9}+\frac{9}{6}+\frac{16}{9}+\frac{25}{18}=\frac{5+16+27+32+25}{18}=\frac{105}{18} \\ & \therefore \text{ Var }(X)=\frac{105}{18}-\frac{35}{18} \times \frac{35}{18}=\frac{1890-1225}{324}=\frac{665}{324} \\ & \text{ Hence, the required variance is } \frac{665}{324} . \end{aligned} $

Solution

Let $A_1$ be the event of getting a total of 6

$ ={(2,4),(4,2),(1,5),(5,1),(3,3)} $

and $B_1$ be the event of getting a total of 7

$ ={(2,5),(5,2),(1,6),(6,1),(3,4),(4,3)} $

Let $P(A_1)$ is the probability, if $A$ wins in a throw $=\frac{5}{36}$

and $P(B_1)$ is the probability, if $B$ wins in a throw $=\frac{1}{6}$

$\therefore$ The required probability of winning $A$ in his third throw

$ =P(\overline{A}_1) \cdot P(\overline{B}_1) \cdot P(A_1)=\frac{31}{36} \cdot \frac{5}{6} \cdot \frac{5}{36}=\frac{775}{7776} . $

Solution

Given that:

$A=\{(x, y): x+y=11\} \text{ and } B=\{(x, y): x \neq 5\}$

$\therefore \quad A=\{(5,6),(6,5)\},$

$B=\{(1,1),(1,2),(1,3),(1,4),(1,5),(1,6),(2,1),(2,2),(2,3),(2,4),(2,5),(2,6),(3,1),$ $(3,2),(3,3),(3,4),(3,5),(3,6),(4,1),(4,2),(4,3),(4,4),(4,5),(4,6),(6,1),(6,2),$ $ (6,3),(6,4),(6,5),(6,6)\}$

$ \begin{aligned} & \Rightarrow n(A)=2, n(B)=30 \text{ and } n(A \cap B)=1 \\ & \therefore \quad P(A)=\frac{2}{36}=\frac{1}{18} \text{ and } P(B)=\frac{30}{36}=\frac{5}{6} \\ & \Rightarrow \quad P(A) \cdot P(B)=\frac{1}{18} \cdot \frac{5}{6}=\frac{5}{108} \text{ and } P(A \cap B)=\frac{1}{36} \end{aligned} $

Since $P(A).P(B) \neq P(P \cap B)$

Hence, A and B are not independent.

Solution

Let $A$ be the event having $m$ white and $n$ black balls

$ \begin{aligned} & E_1={\text{ first ball drawn of white colour }} \\ & E_2={\text{ first ball drawn of black colour }} \end{aligned} $

$ \begin{aligned} & E_3={\text{ second ball drawn of white colour }} \\ & \therefore \quad P(E_1)=\frac{m}{m+n} \text{ and } P(E_2)=\frac{n}{m+n} \\ & P(E_3 / E_1)=\frac{m+k}{m+n+k} \text{ and } P(E_3 / E_2)=\frac{m}{m+n+k} \\ & \text{ Now } P(E_3)=P(E_1) \cdot P(E_3 / E_1)+P(E_2) \cdot P(E_3 / E_2) \\ &=\frac{m}{m+n} \times \frac{m+k}{m+n+k}+\frac{n}{m+n} \times \frac{m}{m+n+k} \\ &=\frac{m}{m+n+k}[\frac{m+k}{m+n}+\frac{n}{m+n}] \\ &=\frac{m}{m+n+k}[\frac{m+n+k}{m+n}]=\frac{m}{m+n} \end{aligned} $

Hence, the probability of drawing a white ball does not depend upon $k$.

Solution

Given that:

Bag I: 3 red balls and no white ball

Bag II: 2 red balls and 1 white ball

Bag III: no red ball and 3 white balls

Let $E_1, E_2$ and $E_3$ be the events of choosing Bag I, Bag II and Bag III respectively and a ball is drawn from it.

$\therefore P(E_1)=\frac{1}{6}, P(E_2)=\frac{2}{6}$ and $P(E_3)=\frac{3}{6}$

(i) Let $E$ be the event that red ball is selected

$ \begin{aligned} \therefore P(E) & =P(E_1) \cdot P(E / E_1)+P(E_2) \cdot P(E / E_2)+P(E_3) \cdot P(E / E_3) \\ & =\frac{1}{6} \cdot \frac{3}{3}+\frac{2}{6} \cdot \frac{2}{3}+\frac{3}{6} \cdot 0=\frac{3}{18}+\frac{4}{18}=\frac{7}{18} \end{aligned} $

(ii) Let $F$ be the event that a white ball is selected

$ \begin{matrix} \therefore P(F) & =1-P(E) & {[P(E)+P(F)=1]} \\ & =1-\frac{7}{18}=\frac{11}{18} & \end{matrix} $

Hence, the required probabilities are $\frac{7}{18}$ and $\frac{11}{18}$.

Solution

Referring to Exercise Q.41, we will use here Bayes’ Theorem

$ \begin{aligned} & \text{ (i) } \begin{aligned} P(E_2 / F) & =\frac{P(E_2) \cdot P(F / E_2)}{P(E_1) \cdot P(F / E_1)+P(E_2) \cdot P(F / E_2)+P(E_3) \cdot P(F / E_3)} \\ & =\frac{\frac{2}{6} \cdot \frac{1}{3}}{\frac{1}{6} \cdot 0+\frac{2}{6} \cdot \frac{1}{3}+\frac{3}{6} \cdot 1}=\frac{\frac{2}{18}}{\frac{2}{18}+\frac{3}{6}}=\frac{2}{11} \\ \text{ (ii) } P(E_3 / F) & =\frac{P(E_3) \cdot P(F / E_3)}{P(E_1) \cdot P(F / E_1)+P(E_2) \cdot P(F / E_2)+P(E_3) \cdot P(F / E_3)} \\ & =\frac{\frac{3}{6} \cdot 1}{\frac{1}{6} \cdot 0+\frac{2}{6} \cdot \frac{1}{3}+\frac{3}{6} \cdot 1}=\frac{\frac{3}{6}}{\frac{2}{18}+\frac{3}{6}}=\frac{3}{6} \times \frac{18}{11}=\frac{9}{11} \end{aligned} \\ & \text{ Hence, the required probabilities are } \frac{2}{11} \text{ and } \frac{9}{11} . \end{aligned} $

Solution

Given that $A_1: A_2: A_3=4: 4: 2$

$\therefore P(A_1)=\frac{4}{10}, P(A_2)=\frac{4}{10}$ and $P(A_3)=\frac{2}{10}$

where $A_1, A_2$ and $A_3$ are the three types of seeds.

Let $E$ be the event that a seed germinates and $\overline{E}$ be the event that a seed does not germinate

$\therefore P(\frac{E}{A_1})=\frac{45}{100}, P(\frac{E}{A_2})=\frac{60}{100}$ and $P(\frac{E}{A_3})=\frac{35}{100}$

and $P(\frac{\overline{E}}{A_1})=\frac{55}{100}, P(\frac{\overline{E}}{A_2})=\frac{40}{100}$ and $P(\frac{\overline{E}}{A_3})=\frac{65}{100}$ (i)

$ \begin{aligned} P(E) & =P(A_1) \cdot P(\frac{E}{A_1})+P(A_2) \cdot P(\frac{E}{A_2})+P(A_3) \cdot P(\frac{E}{A_3}) \\ & =\frac{4}{10} \cdot \frac{45}{100}+\frac{4}{10} \cdot \frac{60}{100}+\frac{2}{10} \cdot \frac{35}{100} \\ & =\frac{180}{1000}+\frac{240}{1000}+\frac{70}{1000}=\frac{490}{1000}=0.49 \end{aligned} $

(ii) $P(\overline{E} / A_3)=1-P(E / A_3)=1-\frac{35}{100}=\frac{65}{100}=0.65$

(iii) Using Bayes’ Theorem, we get

$ \begin{aligned} & P(A_2 / \overline{E})=\frac{P(A_2) \cdot P(\overline{E} / A_2)}{P(A_1) \cdot P(\overline{E} / A_1)+P(A_2) \cdot P(\overline{E} / A_2)+P(A_3) \cdot P(\overline{E} / A_3)} \\ &= \frac{\frac{4}{10} \cdot \frac{40}{100}}{\frac{4}{10} \cdot \frac{55}{100}+\frac{4}{10} \cdot \frac{40}{100}+\frac{2}{10} \cdot \frac{65}{100}} \\ &=\frac{\frac{160}{1000}}{\frac{220}{1000}+\frac{160}{1000}+\frac{130}{1000}}=\frac{160}{220+160+130}=\frac{160}{510}=\frac{16}{51}=0.314 \end{aligned} $

Hence, the required probability is $\frac{16}{51}$ or 0.314 .

Solution

Let $E_1$ : The event that the letter comes from TATANAGAR and $E_2$ : The event that the letter comes from CALCUTTA Also $E_3$ : The event that on the letter, two consecutive letters TA are visible

$\therefore P(E_1)=\frac{1}{2}$ and $P(E_2)=\frac{1}{2}$ and $P(\frac{E_3}{E_1})=\frac{2}{8}$ and $P(\frac{E_3}{E_2})=\frac{1}{7}$

$[\because$ For TATA NAGAR, the two consecutive letters visible are TA, AT, TA, AN, NA, AG, GA, AR] $\therefore P(E_3 / E_1)=\frac{2}{8}$ and [For CALCUTTA, the two consecutive letters visible are $CA, AL, LC, CU, UT, TT$ and TA] So, $P(E_3 / E_2)=\frac{1}{7}$ Now using Bayes’ Theorem, we have

$ P(E_1 / E_3)=\frac{P(E_1) \cdot P(E_3 / E_1)}{P(E_1) \cdot P(E_3 / E_1)+P(E_2) \cdot P(E_3 / E_2)} $

$ =\frac{\frac{1}{2} \cdot \frac{2}{8}}{\frac{1}{2} \cdot \frac{2}{8}+\frac{1}{2} \cdot \frac{1}{7}}=\frac{\frac{1}{8}}{\frac{1}{8}+\frac{1}{14}}=\frac{\frac{1}{8}}{\frac{7+4}{56}}=\frac{7}{11} $

Hence, the required probability is $\frac{7}{11}$.

Solution

Let $E_1$ be the event of selecting Bag I and $E_2$ be the event of selecting Bag II

Let $E_3$ be the event that black ball is selected

$ \begin{aligned} \therefore \quad P(E_1) & =\frac{2}{6}=\frac{1}{3} \text{ and } P(E_2)=1-\frac{1}{3}=\frac{2}{3} \\ P(E_3 / E_1) & =\frac{3}{7} \text{ and } P(E_3 / E_2)=\frac{4}{7} \\ \therefore \quad P(E_3) & =P(E_1) \cdot P(E_3 / E_1)+P(E_2) \cdot P(E_3 / E_2) \\ & =\frac{1}{3} \cdot \frac{3}{7}+\frac{2}{3} \cdot \frac{4}{7}=\frac{3+8}{21}=\frac{11}{21} \end{aligned} $

Hence, the required probability is $\frac{11}{21}$.

Solution

We have 3 urns:

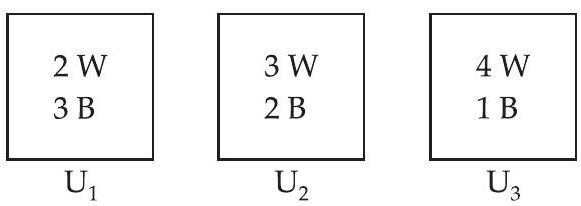
$\therefore$ Probabilities of choosing either of the urns are

$ P(U_1)=P(U_2)=P(U_3)=\frac{1}{3} $

Let $H$ be the event of drawing white ball from the chosen urn.

$ \begin{aligned} & \therefore P(H / U_1)=\frac{2}{5}, P(H / U_2)=\frac{3}{5} \text{ and } P(H / U_3)=\frac{4}{5} \\ & \therefore P(U_2 / H)=\frac{P(U_2) \cdot P(H / U_2)}{P(U_1) \cdot P(H / U_1)+P(U_2) \cdot P(H / U_2)+P(U_3) \cdot P(H / U_3)} \\ & \quad=\frac{\frac{1}{3} \cdot \frac{3}{5}}{\frac{1}{3} \cdot \frac{2}{5}+\frac{1}{3} \cdot \frac{3}{5}+\frac{1}{3} \cdot \frac{4}{5}}=\frac{\frac{3}{5}}{\frac{2}{5}+\frac{3}{5}+\frac{4}{5}}=\frac{3}{9}=\frac{1}{3} \end{aligned} $

Hence, the required probability is $\frac{1}{3}$.

Solution

Let $E_1$ : Event that a person has TB

$ E_2 \text{ : Event that a person does not have TB } $

and $H$ : Event that the person is diagnosed to have TB.

$ \begin{aligned} & \therefore \quad P(E_1)=\frac{1}{1000}=0.001, P(E_2)=1-\frac{1}{1000}=\frac{999}{1000}=0.999 \\ & P(H / E_1)=0.99, P(H / E_2)=0.001 \\ & \therefore P(E_1 / H)=\frac{P(E_1) \cdot P(H / E_1)}{P(E_1) \cdot P(H / E_1)+P(E_2) \cdot P(H / E_2)} \\ & =\frac{0.001 \times 0.99}{0.001 \times 0.99+0.999 \times 0.001}=\frac{0.99}{0.99+0.999} \\ & =\frac{0.990}{0.990+0.999}=\frac{990}{1989}=\frac{110}{221} \end{aligned} $

Hence, the required probability is $\frac{110}{221}$.

Solution

Let $E_1$ : The event that the item is manufactured on machine A

$E_2$ : The event that the item is manufactured on machine $B$

$E_3$ : The event that the item is manufactured on machine C

Let $H$ be the event that the selected item is defective.

$\therefore$ Using Bayes’ Theorem,

$ \begin{aligned} & P(E_1)=\frac{50}{100}, P(E_2)=\frac{30}{100}, P(E_3)=\frac{20}{100} \\ & P(H / E_1)=\frac{2}{100}, P(H / E_2)=\frac{2}{100} \text{ and } P(H / E_3)=\frac{3}{100} \\ & \therefore P(E_1 / H)=\frac{P(E_1) \cdot P(H / E_1)}{P(E_1) \cdot P(H / E_1)+P(E_2) \cdot P(H / E_2)+P(E_3) \cdot P(H / E_3)} \\ & =\frac{\frac{50}{100} \times \frac{2}{100}}{\frac{50}{100} \times \frac{2}{100}+\frac{30}{100} \times \frac{2}{100}+\frac{20}{100} \times \frac{3}{100}} \\ & =\frac{100}{100+60+60}=\frac{100}{220}=\frac{10}{22}=\frac{5}{11} \end{aligned} $

Solution

(i) Here, $P(X=x)=k(x+1)$ for $x=1,2,3,4$

So, $P(X=1)=k(1+1)=2 k ; P(X=2)=k(2+1)=3 k$

$ P(X=3)=k(3+1)=4 k ; P(X=4)=k(4+1)=5 k $

Also, $P(X=x)=2 k x$ for $x=5,6,7$

$ \begin{aligned} & P(X=5)=2(5) k=10 k ; P(X=6)=2(6) k=12 k \\ & P(X=7)=2(7) k=14 k \end{aligned} $

and for otherwise it is 0 .

$\therefore$ The probability distribution is given by

$\begin{array}{|l|l|l|l|l|l|l|l|l|} \hline X & 1 & 2 & 3 & 4 & 5 & 6 & 7 & \text{otherwise} \\ \hline P(X) & 2k & 3k & 4k & 5k & 10k & 12k & 14k & 0 \\ \hline \end{array}$

$ \begin{aligned} & \text{ We know that } \sum _{i=1}^{n} P(X_i)=1 \\ & \text{ So, } 2 k+3 k+4 k+5 k+10 k+12 k+14 k=1 \\ & \Rightarrow \quad 50 k=1 \Rightarrow k=\frac{1}{50} \\ & \text{ Hence, the value of } k \text{ is } \frac{1}{50} \end{aligned} $

(ii) Now the probability distribution is

$\begin{array}{|l|l|l|l|l|l|l|l|} \hline X & 1 & 2 & 3 & 4 & 5 & 6 & 7 \\ \hline P(X) & \frac{2}{50} & \frac{3}{50} & \frac{4}{50} & \frac{5}{50} & \frac{10}{50} & \frac{12}{50} &\frac{14}{50} \\ \hline \end{array}$

$ \begin{aligned} E(X)=1 \times \frac{2}{50}+2 \times \frac{3}{50}+3 \times \frac{4}{50}+4 \times \frac{5}{50}+5 \times \frac{10}{50}+ & 6 \times \frac{12}{50} \\ & +7 \times \frac{14}{50} \end{aligned} $

$ =\frac{2}{50}+\frac{6}{50}+\frac{12}{50}+\frac{20}{50}+\frac{50}{50}+\frac{72}{50}+\frac{98}{50}=\frac{260}{50}=\frac{26}{5}=5.2 $

(iii) We know that Standard deviation (SD) $=\sqrt{\text{ Variance }}$ Variance $=E(X^{2})-[E(X)]^{2}$

$ \begin{aligned} & \begin{aligned} & E(X^{2})= 1 \times \frac{2}{50}+4 \times \frac{3}{50}+9 \times \frac{4}{50}+16 \times \frac{5}{50}+25 \times \frac{10}{50} \\ &=\frac{2}{50}+\frac{12}{50}+\frac{36}{50}+\frac{80}{50}+\frac{250}{50}+\frac{432}{50}+\frac{686}{50}=\frac{1498}{50} \end{aligned} \\ & \therefore \text{ Variance }(X)=\frac{1498}{50}-(\frac{26}{5})^{2} \\ & =\frac{1498}{50}-\frac{676}{25}=\frac{1498-1352}{50}=\frac{146}{50}=2.92 \\ & \therefore \quad \text{ S.D }=\sqrt{2.92}=1.7 \text{ (approx.) } \end{aligned} $

Solution

(i) We know that: $E(X)=\sum _{i=1}^{n} P_i X_i$

$ \begin{matrix} \therefore & E(X) & =1 \times \frac{1}{2}+2 \times \frac{1}{5}+4 \times \frac{3}{25}+2 A \times \frac{1}{10}+3 A \times \frac{1}{25}+5 A \times \frac{1}{25} \\ & 2.94 & =\frac{1}{2}+\frac{2}{5}+\frac{12}{25}+\frac{A}{5}+\frac{3 A}{25}+\frac{A}{5} \\ \Rightarrow & 2.94 & =0.5+0.4+0.48+\frac{13 A}{25}=1.38+\frac{13 A}{25} \\ \Rightarrow & 2.94 & -1.38=\frac{13 A}{25} \Rightarrow 1.56=\frac{13 A}{25} \\ \Rightarrow \quad & A & =\frac{1.56 \times 25}{13}=0.12 \times 25 \\ & \therefore \quad & A & =3 \end{matrix} $

(ii) Now the distribution becomes

$\begin{array}{|l|l|l|l|l|l|l|} \hline X & 1 & 2 & 4 & 6 & 9 & 15 \\ \hline P(X) & \frac{1}{2} & \frac{1}{5} & \frac{3}{25} & \frac{1}{10} & \frac{1}{25} & \frac{1}{25} \\ \hline \end{array}$

$E(X^{2})=1 \times \frac{1}{2}+4 \times \frac{1}{5}+16 \times \frac{3}{25}+36 \times \frac{1}{10}+81 \times \frac{1}{25}+225 \times \frac{1}{25}$

$ \begin{aligned} & =\frac{1}{2}+\frac{4}{5}+\frac{48}{25}+\frac{36}{10}+\frac{81}{25}+\frac{225}{25} \\ & =0.5+0.8+1.92+3.6+3.24+9.00=19.06 \end{aligned} $

Variance $(X)=E(X^{2})-[E(X)]^{2}$

$ =19.06-(2.94)^{2}=19.06-8.64=10.42 $

Solution

Given that: $\quad P(X=x)=\begin{cases} k x^{2} & \text{ for } x=1,2,3 \\ 2 k x & \text{ for } x=4,5,6 \\ 0 & \text{ otherwise } \end{cases} .$

$\therefore$ Probability distribution of random variable $X$ is

$\begin{array}{|l|l|l|l|l|l|l|l|l|} \hline X & 1 & 2 & 3 & 4 & 5 & 6 & \text{otherwise} \\ \hline P(X) & k & 4k & 9k & 8k & 10k & 0 \\ \hline \end{array}$

We know that $\sum _{i=1}^{n} P(X_i)=1$

$\therefore k+4 k+9 k+8 k+10 k+12 k=1 \Rightarrow 44 k=1 \Rightarrow k=\frac{1}{44}$

(i) $E(X)=\sum _{i=1}^{n} P_i X_i=1 \times k+2 \times 4 k+3 \times 9 k+4 \times 8 k+5 \times 10 k+6 \times 12 k$ $=k+8 k+27 k+32 k+50 k+72 k=190 k$ $=190 \times \frac{1}{44}=\frac{95}{22}=4.32$ (approx.)

(ii) $E(3 X^{2})=3[k+4 \times 4 k+9 \times 9 k+16 \times 8 k+25 \times 10 k+36 \times 12 k]$

$=3[k+16 k+81 k+128 k+250 k+432 k]=3[908 k]$

$=3 \times 908 \times \frac{1}{44}=\frac{2724}{44}=61.9$ (approx.)

(iii) $P(X \geq 4)=P(X=4)+P(X=5)+P(X=6)$

$=8 k+10 k+12 k=30 k$

$=30 \times \frac{1}{44}=\frac{15}{22}$.

Solution

Given that $n$ coins are two headed coins and the remaining $(n+1)$ coins are fair.

Let $E_1$ : the event that unfair coin is selected

$E_2$ : the event that the fair coin is selected

$E$ : the event that the toss results in a head

$\therefore \quad P(E_1)=\frac{n}{2 n+1}$ and $P(E_2)=\frac{n+1}{2 n+1}$

$P(E / E_1)=1$ (sure event) and $P(E / E_2)=\frac{1}{2}$

$\therefore \quad P(E)=P(E_1) \cdot P(E / E_1)+P(E_2) \cdot P(E / E_2)$

$=\frac{n}{2 n+1} \cdot 1+\frac{n+1}{2 n+1} \cdot \frac{1}{2}=\frac{1}{2 n+1}(n+\frac{n+1}{2})$

$=\frac{1}{2 n+1}(\frac{2 n+n+1}{2})=\frac{3 n+1}{2(2 n+1)}$

But $\quad P(E)=\frac{31}{42}$ (given)

$ \begin{matrix} \therefore \frac{3 n+1}{2(2 n+1)}=\frac{31}{42} & \Rightarrow & \frac{3 n+1}{2 n+1} & =\frac{31}{21} \\ & \Rightarrow & 63 n+21 & =62 n+31 \\ & \Rightarrow & & n & =10 \end{matrix} $

Hence, the required value of $n$ is 10 .

Solution

Let $X$ be the random variable such that $X=0,1,2$

and $E=$ the event of drawing an ace

and $F=$ the event of drawing non-ace.

$\therefore P(E)=\frac{4}{52}$ and $P(\overline{E})=\frac{48}{52}$

Now $P(X=0)=P(\overline{E}) \cdot P(\overline{E})=\frac{48}{52} \cdot \frac{47}{51}=\frac{188}{221}$

$ \begin{gathered} P(X=1)=P(E) \cdot P(\bar{{}E})+P(\bar{{}E}) \cdot P(E)=\frac{4}{52} \times \frac{48}{51}+\frac{48}{52} \times \frac{4}{51}=\frac{32}{221} \\ P(X=2)=P(E) \cdot P(E)=\frac{4}{52} \cdot \frac{3}{51}=\frac{1}{221} \end{gathered} $

We have Distribution Table:

$\begin{array}{|l|l|l|l|} \hline X & 0 & 1 & 2 \\ \hline P(X) & \frac{188}{221} & \frac{32}{221} & \frac{1}{221} \\ \hline \end{array}$

Now, Mean $E(X)=0 \times \frac{188}{221}+1 \times \frac{32}{221}+2 \times \frac{1}{221}=\frac{32}{221}+\frac{2}{221}=\frac{34}{221}=\frac{2}{13}$

$ E(X^{2})=0 \times \frac{188}{221}+1 \times \frac{32}{221}+4 \times \frac{1}{221}=\frac{32}{221}+\frac{4}{221}=\frac{36}{221} $

$\therefore \quad$ Variance $=E(X^{2})-[E(X)]^{2}$

$ =\frac{36}{221}-(\frac{2}{13})^{2}=\frac{36}{221} \quad \frac{4}{169}=\frac{468-68}{13 \times 221}=\frac{400}{2873} $

Standard deviation $=\sqrt{\frac{400}{2873}}=0.377$ (approx.)

Solution

Let $E$ be the event of getting even number on tossing a die.

$\therefore P(E)=\frac{3}{6}=\frac{1}{2}$ and $P(\overline{E})=1-\frac{1}{2}=\frac{1}{2}$

Here $X=0,1,2$

$ \begin{gathered} P(X=0)=P(\bar{{}E}) \cdot P(\bar{{}E})=\frac{1}{2} \cdot \frac{1}{2}=\frac{1}{4} \\ P(X=1)=P(E) \cdot P(\bar{{}E})+P(\bar{{}E}) \cdot P(E)=\frac{1}{2} \cdot \frac{1}{2}+\frac{1}{2} \cdot \frac{1}{2}=\frac{1}{4}+\frac{1}{4}=\frac{2}{4} \\ P(X=2)=P(E) \cdot P(E)=\frac{1}{2} \cdot \frac{1}{2}=\frac{1}{4} \end{gathered} $

$\therefore$ Probability distribution table is

$\begin{array}{|l|l|l|l|} \hline X & 0 & 1 & 2 \\ \hline P(X) & \frac{1}{4} & \frac{2}{4} & \frac{1}{4} \\ \hline \end{array}$

$ \begin{aligned} E(X) & =0 \times \frac{1}{4}+1 \times \frac{2}{4}+2 \times \frac{1}{4}=\frac{2}{4}+\frac{2}{4}=1 \\ E(X^{2}) & =0 \times \frac{1}{4}+1 \times \frac{2}{4}+4 \times \frac{1}{4}=\frac{3}{2} \end{aligned} $

$\therefore$ Variance $(X)=E(X^{2})-[E(X)]^{2}=\frac{3}{2}-1=\frac{1}{2}=0.5$

Solution

Here, sample space $S=\{(1,2),(2,1),(1,3),(3,1),(2,3),(3,2)$, $(1,4),(4,1),(1,5),(5,1),(2,4),(4,2),(2,5),(5,2),(3,4),(4,3)$, $(3,5),(5,3),(5,4),(4,5)\}$

$\therefore n(S)=20$

Let $X$ be the random variable denoting the sum of the numbers on two cards drawn.

$ \therefore \quad X=3,4,5,6,7,8,9 $

So, $\quad P(X=3)=\frac{2}{20}$

$ \begin{aligned} & P(X=4)=\frac{2}{20} \\ & P(X=6)=\frac{4}{20} \\ & P(X=8)=\frac{2}{20} \end{aligned} $

$ \begin{aligned} & P(X=5)=\frac{4}{20} \\ & P(X=7)=\frac{4}{20} \end{aligned} $

$ \begin{matrix} P(X=9)=\frac{2}{20} \\ \therefore \text{ Mean, } E(X)=3 \times \frac{2}{20}+4 \times \frac{2}{20}+5 \times \frac{4}{20}+6 \times \frac{4}{20}+7 \times \frac{4}{20} \\ +8 \times \frac{2}{20}+9 \times \frac{2}{20} \\ =\frac{6}{20}+\frac{8}{20}+\frac{20}{20}+\frac{24}{20}+\frac{28}{20}+\frac{16}{20}+\frac{18}{20}=\frac{120}{20}=6 \\ E(X^{2})=9 \times \frac{2}{20}+16 \times \frac{2}{20}+25 \times \frac{4}{20}+36 \times \frac{4}{20}+49 \times \frac{4}{20}+64 \times \frac{2}{20}+81 \times \frac{2}{20} \\ =\frac{18}{20}+\frac{32}{20}+\frac{100}{20}+\frac{144}{20}+\frac{196}{20}+\frac{128}{20}+\frac{162}{20}=\frac{780}{20}=39 \\ \therefore \text{ Variance }(X)=E(X^{2})-[E(X)]^{2}=39-(6)^{2}=39-36=3 \end{matrix} $

Solution

Given that: $P(A)=\frac{4}{5}$ and $P(A \cap B)=\frac{7}{10}$

$ \therefore \quad P(B / A)=\frac{P(A \cap B)}{P(A)}=\frac{7 / 10}{4 / 5}=\frac{7}{8} $

Hence, the correct option is (c).

• Option (a) $\frac{1}{10}$: This option is incorrect because it does not satisfy the formula for conditional probability. The correct calculation for $P(B / A)$ is $\frac{P(A \cap B)}{P(A)}$, which results in $\frac{7/10}{4/5} = \frac{7}{8}$, not $\frac{1}{10}$.

• Option (b) $\frac{1}{8}$: This option is incorrect because it also does not satisfy the formula for conditional probability. The correct calculation for $P(B / A)$ is $\frac{P(A \cap B)}{P(A)}$, which results in $\frac{7/10}{4/5} = \frac{7}{8}$, not $\frac{1}{8}$.

• Option (d) $\frac{17}{20}$: This option is incorrect because it does not follow the correct calculation for conditional probability. The correct calculation for $P(B / A)$ is $\frac{P(A \cap B)}{P(A)}$, which results in $\frac{7/10}{4/5} = \frac{7}{8}$, not $\frac{17}{20}$.

Solution

Given that: $P(A \cap B)=\frac{7}{10}$ and $P(B)=\frac{17}{20}$

$ \therefore \quad P(A / B)=\frac{P(A \cap B)}{P(B)}=\frac{7 / 10}{17 / 20}=\frac{14}{17} $

Hence, the correct option is $(a)$.

• Option (b) $\frac{17}{20}$: This option is incorrect because it represents the probability of event B, not the conditional probability ( P(A / B) ). The conditional probability ( P(A / B) ) is calculated using the formula ( \frac{P(A \cap B)}{P(B)} ), which does not simplify to ( \frac{17}{20} ).

• Option (c) $\frac{7}{8}$: This option is incorrect because it does not match the result of the calculation for ( P(A / B) ). The correct calculation is ( \frac{P(A \cap B)}{P(B)} = \frac{7/10}{17/20} = \frac{14}{17} ), not ( \frac{7}{8} ).

• Option (d) $\frac{1}{8}$: This option is incorrect because it is not the result of the calculation for ( P(A / B) ). The correct calculation is ( \frac{P(A \cap B)}{P(B)} = \frac{7/10}{17/20} = \frac{14}{17} ), not ( \frac{1}{8} ).

Solution

Here, $P(A)=\frac{3}{10}, P(B)=\frac{2}{5}$ and $P(A \cup B)=\frac{3}{5}$

$ \begin{aligned} & P(A \cup B)=P(A)+P(B)-P(A \cap B) \\ & \Rightarrow \quad \frac{3}{5}=\frac{3}{10}+\frac{2}{5}-P(A \cap B) \\ & \Rightarrow \quad P(A \cap B)=\frac{3}{10}+\frac{2}{5}-\frac{3}{5}=\frac{3+4-6}{10}=\frac{1}{10} \\ & \text{ Now } P(A / B)+P(B / A)=\frac{P(A \cap B)}{P(B)}+\frac{P(A \cap B)}{P(A)} \\ & =\frac{1 / 10}{2 / 5}+\frac{1 / 10}{3 / 10}=\frac{1}{4}+\frac{1}{3}=\frac{7}{12} \end{aligned} $

Hence, the correct option is $(d)$.

• Option (a) (\frac{1}{4}): This option is incorrect because it does not account for the correct calculation of (P(A / B) + P(B / A)). The correct calculation involves finding the probabilities of (P(A \cap B)) divided by (P(B)) and (P(A \cap B)) divided by (P(A)), which sum to (\frac{7}{12}), not (\frac{1}{4}).

• Option (b) (\frac{1}{3}): This option is incorrect because it also does not match the correct sum of (P(A / B) + P(B / A)). The individual probabilities (\frac{P(A \cap B)}{P(B)}) and (\frac{P(A \cap B)}{P(A)}) do not add up to (\frac{1}{3}).

• Option (c) (\frac{5}{12}): This option is incorrect because it is not the result of the correct calculation. The sum of (\frac{P(A \cap B)}{P(B)}) and (\frac{P(A \cap B)}{P(A)}) is (\frac{7}{12}), not (\frac{5}{12}).

Solution

Given that: $P(A)=\frac{2}{5}, P(B)=\frac{3}{10}$ and $P(A \cap B)=\frac{1}{5}$

$ \begin{aligned} & P(A^{\prime})=1-\frac{2}{5}=\frac{3}{5}, P(B^{\prime})=1-\frac{3}{10}=\frac{7}{10} \\ & \text{ and } P(A^{\prime} \cap B^{\prime})=1-P(A \cup B)=1-[P(A)+P(B)-P(A \cap B)] \\ &=1-[\frac{2}{5}+\frac{3}{10}-\frac{1}{5}]=1-[\frac{1}{5}+\frac{3}{10}]=1-\frac{5}{10}=\frac{1}{2} \\ & \therefore \quad P(A^{\prime} / B^{\prime})=\frac{P(A^{\prime} \cap B^{\prime})}{P(B^{\prime})}=\frac{1 / 2}{7 / 10}=\frac{5}{7} \\ & \text{ and } P(B^{\prime} / A^{\prime})=\frac{P(A^{\prime} \cap B^{\prime})}{P(A^{\prime})}=\frac{1 / 2}{3 / 5}=\frac{5}{6} \\ & \therefore P(A^{\prime} / B^{\prime}) \cdot P(B^{\prime} / A^{\prime})=\frac{5}{7} \times \frac{5}{6}=\frac{25}{42} \end{aligned} $

Hence, the correct option is (c).

• Option (a) (\frac{5}{6}): This option is incorrect because it represents the value of (P(B^{\prime} / A^{\prime})) alone, not the product of (P(A^{\prime} / B^{\prime})) and (P(B^{\prime} / A^{\prime})).

• Option (b) (\frac{5}{7}): This option is incorrect because it represents the value of (P(A^{\prime} / B^{\prime})) alone, not the product of (P(A^{\prime} / B^{\prime})) and (P(B^{\prime} / A^{\prime})).

• Option (d) 1: This option is incorrect because the product of (P(A^{\prime} / B^{\prime})) and (P(B^{\prime} / A^{\prime})) is not equal to 1. The correct product is (\frac{25}{42}).

Solution

Given that: $P(A)=\frac{1}{2}, P(B)=\frac{1}{3}$ and $P(A / B)=\frac{1}{4}$

$ \begin{aligned} P(A / B) & =\frac{P(A \cap B)}{P(B)} \\ \frac{1}{4} & =\frac{P(A \cap B)}{1 / 3} \Rightarrow P(A \cap B)=\frac{1}{4} \times \frac{1}{3}=\frac{1}{12} \end{aligned} $

Now $\quad P(A^{\prime} \cap B^{\prime})=1-P(A \cup B)$

$ =1-[P(A)+P(B)-P(A \cap B)] $

$=1-[\frac{1}{2}+\frac{1}{3}-\frac{1}{12}]=1-[\frac{5}{6}-\frac{1}{12}]=1-\frac{9}{12}=\frac{3}{12}=\frac{1}{4}$

Hence, the correct option is (c).

• Option (a) $\frac{1}{12}$: This option is incorrect because it represents the probability of the intersection of events $A$ and $B$ ($P(A \cap B)$), not the probability of the intersection of their complements ($P(A^{\prime} \cap B^{\prime})$).

• Option (b) $\frac{3}{4}$: This option is incorrect because it does not correctly account for the calculation of $P(A^{\prime} \cap B^{\prime})$. The correct calculation involves subtracting the union of $A$ and $B$ from 1, which results in $\frac{1}{4}$, not $\frac{3}{4}$.

• Option (d) $\frac{3}{16}$: This option is incorrect because it does not follow from the given probabilities and the correct formula for $P(A^{\prime} \cap B^{\prime})$. The correct calculation yields $\frac{1}{4}$, not $\frac{3}{16}$.

Solution

Given that: $P(A)=0.4, P(B)=0.8$ and $P(B / A)=0.6$

$ \begin{aligned} P(B / A)=\frac{P(A \cap B)}{P(A)} & \Rightarrow 0.6=\frac{P(A \cap B)}{0.4} \\ \therefore \quad P(A \cap B) & =0.6 \times 0.4=0.24 \\ P(A \cup B) & =P(A)+P(B)-P(A \cap B) \\ & =0.4+0.8-0.24=1.20-0.24=0.96 \end{aligned} $

Hence, the correct option is (d).

• Option (a) 0.24 is incorrect because it represents the probability of the intersection of events A and B, ( P(A \cap B) ), not the union ( P(A \cup B) ).

• Option (b) 0.3 is incorrect because it does not correspond to any relevant probability calculation given the provided data. It is neither the probability of A, B, nor their intersection or union.

• Option (c) 0.48 is incorrect because it does not match the correct calculation for the union of events A and B. It might be a miscalculation or an unrelated probability.

Solution

Given that: $A=\phi$ and $B \neq \phi$,

then

$ P(A / B)=\frac{P(A \cap B)}{P(B)} $

Hence, the correct option is (b).

• Option (a) $P(A / B)=P(A) \cdot P(B)$: This is incorrect because the conditional probability ( P(A / B) ) is defined as the probability of event ( A ) occurring given that event ( B ) has occurred. It is not simply the product of the probabilities of ( A ) and ( B ). The correct formula for conditional probability is ( P(A / B) = \frac{P(A \cap B)}{P(B)} ).

• Option (c) $P(A / B) \cdot P(B / A)=1$: This is incorrect because the product of the conditional probabilities ( P(A / B) ) and ( P(B / A) ) does not necessarily equal 1. The correct relationship between these probabilities is given by Bayes’ theorem: ( P(A / B) = \frac{P(B / A) \cdot P(A)}{P(B)} ).

• Option (d) $P(A / B)=\frac{P(A)}{P(B)}$: This is incorrect because the conditional probability ( P(A / B) ) is not simply the ratio of the probabilities of ( A ) and ( B ). The correct formula for conditional probability is ( P(A / B) = \frac{P(A \cap B)}{P(B)} ). The given formula would only be correct if ( A ) and ( B ) were independent events, which is not stated in the problem.

Solution

Given that: $P(A)=0.4, P(B)=0.3$ and $P(A \cup B)=0.5$

$ \begin{aligned} P(A \cup B) & =P(A)+P(B)-P(A \cap B) \\ 0.5 & =0.4+0.3-P(A \cap B) \\ P(A \cap B) & =0.4+0.3-0.5=0.2 \\ \therefore \quad P(B^{\prime} \cap A) & =P(A)-P(A \cap B) \\ & =0.4-0.2=0.2=\frac{1}{5} \end{aligned} $

Hence, the correct option is $(d)$.

• Option (a) $\frac{2}{3}$: This option is incorrect because the calculation of $P(B^{\prime} \cap A)$ is based on the given probabilities and the formula $P(B^{\prime} \cap A) = P(A) - P(A \cap B)$. The correct value is $0.2$, which is not equal to $\frac{2}{3}$.

• Option (b) $\frac{1}{2}$: This option is incorrect because, as per the given probabilities and the formula used, $P(B^{\prime} \cap A) = 0.2$. The value $\frac{1}{2}$ (or 0.5) does not match the calculated value of $0.2$.

• Option (c) $\frac{3}{10}$: This option is incorrect because the correct calculation of $P(B^{\prime} \cap A)$ results in $0.2$. The value $\frac{3}{10}$ (or 0.3) is not equal to the calculated value of $0.2$.

Solution

Given that: $P(B)=\frac{3}{5}, P(A / B)=\frac{1}{2}$ and $P(A \cup B)=\frac{4}{5}$

We know that $P(A / B)=\frac{P(A \cap B)}{P(B)} \Rightarrow \frac{1}{2}=\frac{P(A \cap B)}{3 / 5}$

$\therefore \quad P(A \cap B)=\frac{3}{10}$

Now $\quad P(A \cup B)=P(A)+P(B)-P(A \cap B)$

$ \begin{aligned} \frac{4}{5} & =P(A)+\frac{3}{5}-\frac{3}{10} \\ \Rightarrow \quad P(A) & =\frac{4}{5}-\frac{3}{5}+\frac{3}{10}=\frac{1}{5}+\frac{3}{10}=\frac{5}{10}=\frac{1}{2} \end{aligned} $

Hence, the correct option is (c).

• Option (a) $\frac{3}{10}$ is incorrect because when we solve for $P(A)$ using the given probabilities and the formula for the union of two events, we find that $P(A) = \frac{1}{2}$, not $\frac{3}{10}$.

• Option (b) $\frac{1}{5}$ is incorrect because the calculation for $P(A)$ involves subtracting $P(B)$ and adding $P(A \cap B)$ to $P(A \cup B)$. This results in $P(A) = \frac{1}{2}$, not $\frac{1}{5}$.

• Option (d) $\frac{3}{5}$ is incorrect because $P(A)$ is derived from the equation $P(A \cup B) = P(A) + P(B) - P(A \cap B)$. Solving this equation with the given values results in $P(A) = \frac{1}{2}$, not $\frac{3}{5}$.

Solution

According to Exercise 64, we have

$ \begin{aligned} & P(B)=\frac{3}{5}, P(A / B)=\frac{1}{2}, P(A \cup B)=\frac{4}{5} \\ & P(B / A^{\prime})=\frac{P(B \cap A^{\prime})}{P(A^{\prime})}=\frac{P(B)-P(A \cap B)}{1-P(A)}=\frac{\frac{3}{5}-\frac{3}{10}}{1-\frac{1}{2}}=\frac{\frac{3}{10}}{\frac{1}{2}}=\frac{3}{5} \end{aligned} $

Hence, the correct option is $(d)$.

• Option (a) $\frac{1}{5}$ is incorrect because the calculation of $P(B / A^{\prime})$ involves the difference $P(B) - P(A \cap B)$ and the division by $1 - P(A)$. The correct calculation yields $\frac{3}{5}$, not $\frac{1}{5}$.

• Option (b) $\frac{3}{10}$ is incorrect because it represents the value of $P(A \cap B)$, not $P(B / A^{\prime})$. The correct calculation for $P(B / A^{\prime})$ yields $\frac{3}{5}$.

• Option (c) $\frac{1}{2}$ is incorrect because it does not match the result of the calculation for $P(B / A^{\prime})$. The correct calculation yields $\frac{3}{5}$, not $\frac{1}{2}$.

Solution

Given that: $P(B)=\frac{3}{5}, P(A / B)=\frac{1}{2}$ and $P(A \cup B)=\frac{4}{5}$

$ \begin{aligned} P(A / B) & =\frac{P(A \cap B)}{P(B)} \\ \Rightarrow \quad \frac{1}{2} & =\frac{P(A \cap B)}{3 / 5} \Rightarrow P(A \cap B)=\frac{3}{10} \\ P(A \cup B) & =P(A)+P(B)-P(A \cap B) \\ \frac{4}{5} & =P(A)+\frac{3}{5}-\frac{3}{10} \\ \therefore \quad P(A) & =\frac{4}{5}-\frac{3}{5}+\frac{3}{10}=\frac{1}{5}+\frac{3}{10}=\frac{5}{10}=\frac{1}{2} \end{aligned} $

Now $P(A \cup B)^{\prime}+P(A^{\prime} \cup B)$

$ \begin{aligned} & =1-P(A \cup B)+1-P(A \cap B^{\prime}) \\ & =2-\frac{4}{5}-P(A) \cdot P(B^{\prime}) \\ & =\frac{6}{5}-\frac{1}{2} \cdot(1-\frac{3}{5})=\frac{6}{5}-\frac{1}{2} \times \frac{2}{5}=\frac{6}{5}-\frac{1}{5}=\frac{5}{5}=1 \end{aligned} $

Hence, the correct option is $(d)$.

• Option (a) $\frac{1}{5}$: This option is incorrect because the calculation of $P(A \cup B)^{\prime} + P(A^{\prime} \cup B)$ involves subtracting the probabilities and adding the complements, which results in a value of 1, not $\frac{1}{5}$.

• Option (b) $\frac{4}{5}$: This option is incorrect because it does not account for the correct combination of probabilities and their complements. The correct calculation shows that the sum is 1, not $\frac{4}{5}$.

• Option (c) $\frac{1}{2}$: This option is incorrect because it misrepresents the result of the combined probabilities and their complements. The correct calculation yields a total of 1, not $\frac{1}{2}$.

Solution

Given that: $P(A)=\frac{7}{13}, P(B)=\frac{9}{13}$ and $P(A \cap B)=\frac{4}{13}$

$ P(A^{\prime} / B)=\frac{P(A^{\prime} \cap B)}{P(B)}=\frac{P(B)-P(A \cap B)}{P(B)}=\frac{\frac{9}{13}-\frac{4}{13}}{\frac{9}{13}}=\frac{\frac{5}{13}}{\frac{9}{13}}=\frac{5}{9} $

Hence, the correct option is $(d)$.

• Option (a) $\frac{6}{13}$: This option is incorrect because it does not account for the conditional probability formula. The correct calculation involves dividing the difference between $P(B)$ and $P(A \cap B)$ by $P(B)$, which results in $\frac{5}{9}$, not $\frac{6}{13}$.

• Option (b) $\frac{4}{13}$: This option is incorrect because it represents the probability of $A \cap B$ rather than the conditional probability $P(A^{\prime} / B)$. The correct calculation for $P(A^{\prime} / B)$ involves using the formula $\frac{P(B) - P(A \cap B)}{P(B)}$.

• Option (c) $\frac{4}{9}$: This option is incorrect because it results from an incorrect calculation. The correct calculation for $P(A^{\prime} / B)$ is $\frac{P(B) - P(A \cap B)}{P(B)} = \frac{5}{9}$, not $\frac{4}{9}$.

Solution

Given that: $P(A)>0$ and $P(B) \neq 1$

$ \therefore \quad P(A^{\prime} / B^{\prime})=\frac{P(A^{\prime} \cap B^{\prime})}{P(B^{\prime})}=\frac{1-P(A \cup B)}{P(B^{\prime})} $

Hence, the correct option is (c).

• Option (a): (1 - P(A / B)) is incorrect because (P(A^{\prime} / B^{\prime})) is not simply the complement of (P(A / B)). The relationship between (P(A^{\prime} / B^{\prime})) and (P(A / B)) involves the joint probabilities of (A) and (B) and their complements, not just a straightforward complement operation.

• Option (b): (1 - P(A^{\prime} / B)) is incorrect because (P(A^{\prime} / B^{\prime})) is not the complement of (P(A^{\prime} / B)). The conditional probabilities involving complements of events do not follow this simple complement rule.

• Option (d): (\frac{P(A^{\prime})}{P(B^{\prime})}) is incorrect because (P(A^{\prime} / B^{\prime})) is the conditional probability of (A^{\prime}) given (B^{\prime}), which depends on the joint probability of (A^{\prime}) and (B^{\prime}). The correct expression involves the joint probability (P(A^{\prime} \cap B^{\prime})), not just the marginal probability (P(A^{\prime})).

Solution

Given that: A and B are independent events

such that

$ \begin{aligned} & P(A)=\frac{3}{5} \quad \therefore P(A^{\prime})=1-\frac{3}{5}=\frac{2}{5} \\ & P(B)=\frac{4}{9} \quad \therefore P(B^{\prime})=1-\frac{4}{9}=\frac{5}{9} \end{aligned} $

$ \therefore \quad P(A^{\prime} \cap B^{\prime})=P(A^{\prime}) \cdot P(B^{\prime})=\frac{2}{5} \cdot \frac{5}{9}=\frac{2}{9} $

Hence, the correct option is $(d)$.

• Option (a) (\frac{4}{15}): This option is incorrect because it does not correctly represent the product of the probabilities of the complements of (A) and (B). The correct calculation is (\frac{2}{5} \cdot \frac{5}{9} = \frac{2}{9}), not (\frac{4}{15}).

• Option (b) (\frac{8}{45}): This option is incorrect because it also does not correctly represent the product of the probabilities of the complements of (A) and (B). The correct calculation is (\frac{2}{5} \cdot \frac{5}{9} = \frac{2}{9}), not (\frac{8}{45}).

• Option (c) (\frac{1}{3}): This option is incorrect because it does not correctly represent the product of the probabilities of the complements of (A) and (B). The correct calculation is (\frac{2}{5} \cdot \frac{5}{9} = \frac{2}{9}), not (\frac{1}{3}).

Solution

For independent events $A$ and $B, P(A) \cdot P(B)=P(A \cap B)$

So, they will not be mutually exclusive.

If $P(A)+P(B)=1$, they are exhaustive events and for independent events $A$ and $P(A \cap B) \neq 0$.

Hence, the correct option is $(d)$.

• Option (a): If two events are mutually exclusive, it means that they cannot occur simultaneously, i.e., ( P(A \cap B) = 0 ). However, for independent events, ( P(A \cap B) = P(A) \cdot P(B) ). Therefore, if ( P(A) \cdot P(B) \neq 0 ), the events cannot be mutually exclusive. Hence, independence does not imply mutual exclusivity.

• Option (b): The sum of the probabilities of two independent events does not necessarily equal 1. The sum of their probabilities can be any value between 0 and 2, depending on the individual probabilities of the events. Therefore, independence does not imply that ( P(A) + P(B) = 1 ).

• Option (c): Since both (a) and (b) are incorrect, this option is also incorrect.

Solution

Given that: $P(A)=\frac{3}{8}, P(B)=\frac{5}{8}$ and $P(A \cup B)=\frac{3}{4}$

$ \begin{matrix} \therefore & P(A \cup B) & =P(A)+P(B)-P(A \cap B) \\ \frac{3}{4} & =\frac{3}{8}+\frac{5}{8}-P(A \cap B) \\ \Rightarrow \quad P(A \cap B) & =\frac{3}{8}+\frac{5}{8}-\frac{3}{4}=\frac{1}{4} \end{matrix} $

$ \begin{aligned} \text{ Now } P(A / B) \cdot P(A^{\prime} / B) & =\frac{P(A \cap B)}{P(B)} \cdot \frac{P(A^{\prime} \cap B)}{P(B)} \\ & =\frac{P(A \cap B)}{P(B)} \cdot \frac{P(B)-P(A \cap B)}{P(B)} \\ & =\frac{\frac{1}{5}}{\frac{5}{8}} \cdot \frac{(\frac{5}{8}-\frac{1}{4})}{\frac{5}{8}}=\frac{2}{5} \cdot \frac{3}{5}=\frac{6}{25} \end{aligned} $

Hence, the correct option is $(d)$.

• Option (a) $\frac{2}{5}$: This option is incorrect because it does not account for the correct calculation of ( P(A / B) \cdot P(A^{\prime} / B) ). The correct calculation involves multiplying ( \frac{1/4}{5/8} ) by ( \frac{(5/8 - 1/4)}{5/8} ), which results in ( \frac{6}{25} ), not ( \frac{2}{5} ).

• Option (b) $\frac{3}{8}$: This option is incorrect because it does not follow from the correct application of the formula for conditional probabilities. The correct product of ( P(A / B) ) and ( P(A^{\prime} / B) ) is ( \frac{6}{25} ), not ( \frac{3}{8} ).

• Option (c) $\frac{3}{20}$: This option is incorrect because it results from an incorrect calculation of the probabilities involved. The correct product of ( P(A / B) ) and ( P(A^{\prime} / B) ) is ( \frac{6}{25} ), not ( \frac{3}{20} ).

Solution

Since $A$ and $B$ are two independent events

$ \therefore \quad P(A \cap B)=P(A) \cdot P(B) $

Hence, the correct option is (c).

• Option (a) $P(A) + P(B)$: This option is incorrect because the sum of the probabilities of two events does not account for their intersection. For independent events, the probability of both events occurring together is the product of their individual probabilities, not the sum.

• Option (b) $P(A) - P(B)$: This option is incorrect because subtracting the probability of one event from another does not represent the probability of their intersection. The intersection of two independent events is calculated by multiplying their probabilities, not by subtraction.

• Option (d) $\frac{P(A)}{P(B)}$: This option is incorrect because dividing the probability of one event by the probability of another does not yield the probability of their intersection. For independent events, the correct operation is multiplication, not division.

Solution

Given that: $E$ and $F$ are independent events such that $P(E)=0.3$ and $P(E \cup F)=0.5$

$ \begin{matrix} P(E \cup F) =P(E)+P(F)-P(E \cap F) \\ 0.5 =0.3+P(F)-P(E) \cdot P(F) \\ \Rightarrow \quad 0.5-0.3 =P(F)[1-P(E)] \Rightarrow 0.2=P(F)(1-0.3) \\ \Rightarrow \quad 0.2 =P(F) \cdot(0.7) \\ \therefore \quad P(F) =\frac{0.2}{0.7}=\frac{2}{7} \\ \\ \text{ Now } P(E / F)-P(F / E) =\frac{P(E \cap F)}{P(F)}-\frac{P(E \cap F)}{P(E)} \\ =\frac{P(E) \cdot P(F)}{P(F)}-\frac{P(E) \cdot P(F)}{P(E)} =P(E)-P(F)=\frac{3}{10}-\frac{2}{7}=\frac{1}{70} \end{matrix} $

Hence, the correct option is (c).

• Option (a) (\frac{2}{7}): This option is incorrect because it represents the probability (P(F)), not the difference (P(E / F) - P(F / E)). The calculation shows that (P(F) = \frac{2}{7}), but this is not the value we are looking for.

• Option (b) (\frac{3}{35}): This option is incorrect because it does not match the calculated difference (P(E / F) - P(F / E)). The correct difference is (\frac{1}{70}), and (\frac{3}{35}) is a different value that does not correspond to any part of the given problem.

• Option (d) (\frac{1}{7}): This option is incorrect because it is not the result of the difference (P(E / F) - P(F / E)). The correct calculation shows that the difference is (\frac{1}{70}), and (\frac{1}{7}) is a larger value that does not fit the derived result.

Solution

Given that: Bag contains 5 red and 3 blue balls.

Probability of getting exactly one red ball if 3 balls are randomly drawn without replacement

$=P(R) \cdot P(B) \cdot P(B)+P(B) \cdot P(R) \cdot P(B)+P(B) \cdot P(B) \cdot P(R)$

$=\frac{5}{8} \cdot \frac{3}{7} \cdot \frac{2}{6}+\frac{3}{8} \cdot \frac{5}{7} \cdot \frac{2}{6}+\frac{3}{8} \cdot \frac{2}{7} \cdot \frac{5}{6}=\frac{30}{336}+\frac{30}{336}+\frac{30}{336}=\frac{90}{336}=\frac{15}{56}$

Hence, the correct option is (c).

• Option (a) $\frac{45}{196}$ is incorrect because the correct probability calculation yields $\frac{15}{56}$, not $\frac{45}{196}$. The denominator 196 does not align with the total number of ways to draw 3 balls from 8, which is 56.

• Option (b) $\frac{135}{392}$ is incorrect because the correct probability calculation yields $\frac{15}{56}$. The numerator 135 and the denominator 392 do not match the correct values derived from the combination calculations.

• Option (d) $\frac{15}{29}$ is incorrect because the correct probability calculation yields $\frac{15}{56}$. The denominator 29 is not the correct total number of ways to draw 3 balls from 8, which is 56.

Solution

According to Question 74,

Let $E_1$ be the event that first ball is red.

$E_2$ be the event that exactly two of the three balls are red.

$\therefore \quad P(E_1)=P(R) \cdot P(R) \cdot P(B)+P(R) \cdot P(R) \cdot P(R)+P(R) \cdot P(B) \cdot P(R)$ $+P(R) \cdot P(B) \cdot P(B)$

$=\frac{5}{8} \cdot \frac{4}{7} \cdot \frac{3}{6}+\frac{5}{8} \cdot \frac{4}{7} \cdot \frac{3}{6}+\frac{5}{8} \cdot \frac{3}{7} \cdot \frac{4}{6}+\frac{5}{8} \cdot \frac{3}{7} \cdot \frac{2}{6}$

$=\frac{60}{336}+\frac{60}{336}+\frac{60}{336}+\frac{30}{336}=\frac{210}{336}$

$P(E_1 \cap E_2)=P(R) \cdot P(B) \cdot P(R)+P(R) \cdot P(R) \cdot P(B)$

$=\frac{5}{8} \cdot \frac{3}{7} \cdot \frac{4}{6}+\frac{5}{8} \cdot \frac{4}{7} \cdot \frac{3}{6}=\frac{60}{336}+\frac{60}{336}=\frac{120}{336}$

$\therefore P(E_2 / E_1)=\frac{P(E_1 \cap E_2)}{P(E_1)}=\frac{120 / 336}{210 / 336}=\frac{4}{7}$

Hence, the correct option is $(b)$.

• Option (a) $\frac{1}{3}$: This option is incorrect because the calculated probability of exactly two of the three balls being red, given that the first ball is red, is $\frac{4}{7}$. The value $\frac{1}{3}$ does not match this probability and is not derived from the given conditions and calculations.

• Option (c) $\frac{15}{28}$: This option is incorrect because the correct probability, as calculated, is $\frac{4}{7}$. The fraction $\frac{15}{28}$ simplifies to $\frac{15}{28}$, which is not equal to $\frac{4}{7}$. Therefore, it does not represent the correct probability.

• Option (d) $\frac{5}{28}$: This option is incorrect because the correct probability is $\frac{4}{7}$. The fraction $\frac{5}{28}$ does not match the calculated probability and is not derived from the given conditions and calculations.

Solution

Given that: $P(A)=0.4, P(B)=0.3$ and $P(C)=0.2$

Also $P(\overline{A})=1-0.4=0.6, P(\overline{B})=1-0.3=0.7$

and $P(\bar{{}C})=1-0.2=0.8$

$\therefore$ Probabilities of two hits

$=P(A) \cdot P(B) \cdot P(\overline{C})+P(A) \cdot P(\overline{B}) \cdot P(C)+P(\overline{A}) \cdot P(B) \cdot P(C)$

$=0.4 \times 0.3 \times 0.8+0.4 \times 0.7 \times 0.2+0.6 \times 0.3 \times 0.2$

$=0.096+0.056+0.036=0.188$

Hence, the correct option is $(b)$.

• Option (a) 0.024: This value is too low to represent the probability of two hits given the probabilities of hitting the target for A, B, and C. The calculation of two hits involves multiple combinations of hits and misses, and the sum of these probabilities is significantly higher than 0.024.

• Option (c) 0.336: This value is too high. The correct calculation involves summing the probabilities of three different scenarios where exactly two out of the three persons hit the target. The sum of these probabilities is 0.188, which is much lower than 0.336.

• Option (d) 0.452: This value is also too high. The combined probability of two hits from the three shooters, considering their individual probabilities of hitting and missing the target, results in a total probability of 0.188, which is significantly less than 0.452.

Solution

Let $G$ denotes the girl and $B$ denotes the boy of the given family.

$\text{So}n(S)=\{(BGG),(GBG),(GGB),(GBB),(BGB),(BBG),(BBB), (GGG)\}$

Let $E_1$ be the event that the family has alteast one girl.

$\therefore E_1=\{(BGG),(GBG),(GGB),(GBB),(BGB),(BBG),(GGG)\}$

Let $E_2$ be the event that the eldest child is a girl.

$ \begin{aligned} & \therefore \quad E_2={(GBG),(GGB),(GBB),(GGG)} \\ & (E_1 \cap E_2)={(GBB),(GGB),(GBG),(GGG)} \\ & \therefore P(E_2 / E_1)=\frac{P(E_1 \cap E_2)}{P(E_1)}=\frac{4 / 8}{7 / 8}=\frac{4}{7} \end{aligned} $

Hence, the correct option is $(d)$.

• Option (a) $\frac{1}{2}$: This option is incorrect because it assumes that the probability of the eldest child being a girl is independent of the condition that the family has at least one girl. However, the condition changes the sample space, and the correct probability is not simply $\frac{1}{2}$.

• Option (b) $\frac{1}{3}$: This option is incorrect because it does not correctly account for the conditional probability given that there is at least one girl in the family. The correct calculation involves considering the reduced sample space where at least one girl is present, which leads to a different probability.

• Option (c) $\frac{2}{3}$: This option is incorrect because it likely misinterprets the problem or miscalculates the conditional probability. The correct approach involves finding the intersection of the events and then dividing by the probability of having at least one girl, which results in $\frac{4}{7}$, not $\frac{2}{3}$.

Solution

Let $E_1$ be the event of getting even number on the die. $E_2$ be the event of selecting a spade card.

$\therefore P(E_1)=\frac{3}{6}=\frac{1}{2}$ and $P(E_2)=\frac{13}{52}=\frac{1}{4}$

So $\quad P(E_1 \cap E_2)=P(E_1) \cdot P(E_2)=\frac{1}{2} \cdot \frac{1}{4}=\frac{1}{8}$

Hence, the correction option is (c).

• Option (a) $\frac{1}{2}$ is incorrect because it assumes that the probability of both events happening together is the same as the probability of one of the events happening alone, which is not the case. The correct probability is the product of the individual probabilities of the two independent events.

• Option (b) $\frac{1}{4}$ is incorrect because it only considers the probability of one of the events (selecting a spade card) and does not account for the combined probability of both events (getting an even number on the die and selecting a spade card).

• Option (d) $\frac{3}{4}$ is incorrect because it overestimates the combined probability of the two independent events. The correct combined probability is much lower, as calculated by multiplying the individual probabilities of the two events.

Solution

Probability of drawing 2 green and 1 blue balls $=P(G) \cdot P(G) \cdot P(B)+P(G) \cdot P(B) \cdot P(G)+P(B) \cdot P(G) \cdot P(G)$

$=\frac{3}{8} \cdot \frac{2}{7} \cdot \frac{2}{6}+\frac{3}{8} \cdot \frac{2}{7} \cdot \frac{2}{6}+\frac{2}{8} \cdot \frac{3}{7} \cdot \frac{2}{6}=\frac{12}{336}+\frac{12}{336}+\frac{12}{336}=\frac{36}{336}=\frac{3}{28}$

Hence, the correct option is $(a)$.

• Option (b) $\frac{2}{21}$ is incorrect because the calculated probability of drawing 2 green balls and 1 blue ball is $\frac{3}{28}$, not $\frac{2}{21}$. The value $\frac{2}{21}$ does not match the correct probability derived from the combinations and calculations.

• Option (c) $\frac{1}{28}$ is incorrect because the correct probability of drawing 2 green balls and 1 blue ball is $\frac{3}{28}$. The value $\frac{1}{28}$ is too low and does not match the correct probability derived from the combinations and calculations.

• Option (d) $\frac{167}{168}$ is incorrect because it is significantly higher than the correct probability of $\frac{3}{28}$. The value $\frac{167}{168}$ is almost 1, which would imply an almost certain event, whereas the actual probability is much lower.

Solution

Required probability $=P($ dead $) \cdot P($ dead $)$

$ =\frac{3}{8} \cdot \frac{2}{7}=\frac{3}{28} $

Hence, the correct option is $(d)$.

• Option (a) $\frac{33}{56}$: This option is incorrect because it does not correctly represent the probability of selecting two dead batteries out of 8, where 3 are dead. The correct calculation involves multiplying the probabilities of selecting a dead battery first and then another dead battery without replacement, which results in $\frac{3}{28}$, not $\frac{33}{56}$.

• Option (b) $\frac{9}{64}$: This option is incorrect because it incorrectly assumes that the probability of selecting two dead batteries is the product of $\frac{3}{8}$ and $\frac{3}{8}$, which would be the case if the batteries were replaced after each selection. However, since the selection is without replacement, the correct probability is $\frac{3}{8} \cdot \frac{2}{7} = \frac{3}{28}$.

• Option (c) $\frac{1}{14}$: This option is incorrect because it does not accurately reflect the probability of selecting two dead batteries without replacement. The correct probability is $\frac{3}{8} \cdot \frac{2}{7} = \frac{3}{28}$, which simplifies to $\frac{3}{28}$, not $\frac{1}{14}$.

Solution

Here, $n=8, p=\frac{1}{2}, q=1-\frac{1}{2}=\frac{1}{2}$ and $r=3$

We know that $P(x=r)={ }^{n} C_r p^{r} . q^{n-r}$

$ \begin{aligned} \therefore \quad P(x=3) & ={ }^{8} C_3(\frac{1}{2})^{3}(\frac{1}{2})^{8-3} \\ & =\frac{8 !}{3 ! \cdot 5 !} \cdot(\frac{1}{2})^{3}(\frac{1}{2})^{5}=56 \cdot(\frac{1}{2})^{8}=56 \cdot \frac{1}{256}=\frac{7}{32} \end{aligned} $

Hence, the correct option is $(b)$.

• Option (a) $\frac{1}{256}$ is incorrect because it represents the probability of getting exactly 0 heads or 8 heads when tossing 8 coins, not 3 heads.

• Option (c) $\frac{5}{32}$ is incorrect because it does not match the calculated probability of getting exactly 3 heads, which is $\frac{7}{32}$.

• Option (d) $\frac{3}{32}$ is incorrect because it also does not match the calculated probability of getting exactly 3 heads, which is $\frac{7}{32}$.

Solution

Let $E_1$ be the event showing the sum of the numbers on the two dice was less than 6 and $E_2$ be the event that the sum of the numbers is 3 .

$ \begin{aligned} & \therefore \quad E_1=\{(1,1),(1,2),(2,1),(1,3),(3,1),(1,4),(4,1), \\ &(2,2),(2,3),(3,2)\} \\ & \text{ and } \quad n(E_1)=10 \\ & E_2={(1,2),(2,1)} \Rightarrow n(E_2)=2 \text{ and } n(E_1 \cap E_2)=2 \end{aligned} $

$\therefore$ Required probability

$ P(E_2 / E_1)=\frac{n(E_1 \cap E_2)}{n(E_1)}=\frac{2}{10}=\frac{1}{5} $

Hence, the correct option is (c).

• Option (a) $\frac{1}{18}$ is incorrect because it does not correctly represent the ratio of favorable outcomes to the total outcomes where the sum of the numbers on the dice is less than 6. The correct ratio is $\frac{2}{10}$, which simplifies to $\frac{1}{5}$.

• Option (b) $\frac{5}{18}$ is incorrect because it overestimates the probability. The correct probability is $\frac{1}{5}$, which is derived from the ratio of favorable outcomes (sum of 3) to the total outcomes where the sum is less than 6.

• Option (d) $\frac{2}{5}$ is incorrect because it overestimates the probability. The correct probability is $\frac{1}{5}$, which is derived from the ratio of favorable outcomes (sum of 3) to the total outcomes where the sum is less than 6.

Solution

We know that for a Binomial distribution, the outcomes must not be dependent on each other.

Hence, the correct option is (c).

• (a) There are two outcomes for each trial.

  • This is a requirement of a Binomial distribution because each trial must result in one of two possible outcomes, typically labeled as “success” and “failure.”

• (b) There is a fixed number of trials.

  • This is a requirement of a Binomial distribution because the number of trials must be predetermined and constant.

• (d) The probability of success must be the same for all trials.

  • This is a requirement of a Binomial distribution because the probability of success must remain constant across all trials to ensure the trials are identically distributed.

Solution

Probability of getting Queen $=\frac{4}{52}$

So, the required probability

$ \begin{aligned} & =P(\text{ Queen }) \cdot P(\text{ Queen }) \\ & =\frac{4}{52} \cdot \frac{4}{52}=\frac{1}{13} \cdot \frac{1}{13} \quad \text{ (with replacement) } \end{aligned} $

Hence, the correct option is $(a)$.

• Option (b) $\frac{1}{13}+\frac{1}{13}$: This option is incorrect because it represents the sum of probabilities rather than the product. When calculating the probability of two independent events both occurring, you multiply their probabilities, not add them.

• Option (c) $\frac{1}{13} \times \frac{1}{17}$: This option is incorrect because it uses an incorrect probability for the second card. The probability of drawing a queen remains $\frac{1}{13}$ for both draws since the cards are drawn with replacement, meaning the deck is reset to its original state after each draw.

• Option (d) $\frac{1}{13} \times \frac{4}{51}$: This option is incorrect because it assumes the cards are drawn without replacement. If the cards were drawn without replacement, the probability of drawing a queen on the second draw would be $\frac{3}{51}$ after drawing a queen on the first draw. However, since the problem states the cards are drawn with replacement, the probability for each draw remains $\frac{1}{13}$.

Solution

Here, $n=10, p=\frac{1}{2}$ and $q=\frac{1}{2} \quad$ (for true/false questions) and $r \geq 8$ i.e. $8,9,10$

$ \begin{aligned} \therefore P(X \geq 8) & =P(x=8)+P(x=9)+P(x=10) \\ & ={ }^{10} C_8(\frac{1}{2})^{8}(\frac{1}{2})^{2}+{ }^{10} C_9(\frac{1}{2})^{9}(\frac{1}{2})+{ }^{10} C _{10}(\frac{1}{2})^{10}(\frac{1}{2})^{0} \\ & =45 \cdot(\frac{1}{2})^{10}+10 \cdot(\frac{1}{2})^{10}+(\frac{1}{2})^{10}=(\frac{1}{2})^{10}(45+10+1) \\ & =56 \times \frac{1}{1024}=\frac{7}{128} \end{aligned} $

Hence, the correct option is (b).

• Option (a) $\frac{7}{64}$: This option is incorrect because the calculated probability of guessing correctly at least 8 out of 10 answers is $\frac{7}{128}$, not $\frac{7}{64}$. The value $\frac{7}{64}$ is twice the correct probability, indicating a miscalculation.

• Option (c) $\frac{45}{1024}$: This option is incorrect because it represents only the probability of guessing exactly 8 out of 10 answers correctly. The problem requires the probability of guessing at least 8 answers correctly, which includes the probabilities of guessing 8, 9, and 10 answers correctly.

• Option (d) $\frac{7}{41}$: This option is incorrect because it does not match the calculated probability of $\frac{7}{128}$. The value $\frac{7}{41}$ is not derived from the binomial probability formula used in the solution and is not a simplified form of the correct probability.

Solution

Given that: $\overline{P}=0.3 \therefore p=0.7$ and $q=1-0.7=0.3$ $n=5$ and $r=4$

We know that

$ \begin{aligned} & P(X=r)={ }^{n} C_r(p)^{r} \cdot(q)^{n-r} \\ \therefore \quad & P(x=4)={ }^{5} C_4(0.7)^{4}(0.3)^{5-4}={ }^{5} C_4(0.7)^{4}(0.3) \end{aligned} $

Hence, the correct option is (a).

• Option (b) is incorrect because it incorrectly places the power of 4 on the probability of not being a swimmer (0.3) instead of the probability of being a swimmer (0.7). The correct formula should have the power of 4 on the probability of being a swimmer.

• Option (c) is incorrect because it incorrectly places the power of 4 on the probability of not being a swimmer (0.3) instead of the probability of being a swimmer (0.7). The correct formula should have the power of 4 on the probability of being a swimmer.

• Option (d) is incorrect because it does not include the binomial coefficient ${ }^{5} C_4$, which is necessary to account for the different ways 4 swimmers can be chosen out of 5 people.

Solution

We know that $\sum _{i=1}^{n} P(X_i)=1$

$ \begin{aligned} \therefore \quad \frac{5}{k}+\frac{7}{k}+\frac{9}{k}+\frac{11}{k} & =1 \\ \frac{32}{k} & =1 \Rightarrow k=32 . \end{aligned} $

Hence, the correct option is (c).

• Option (a) 8 is incorrect because if ( k = 8 ), then the sum of the probabilities would be (\frac{5}{8} + \frac{7}{8} + \frac{9}{8} + \frac{11}{8} = \frac{32}{8} = 4 ), which is not equal to 1.

• Option (b) 16 is incorrect because if ( k = 16 ), then the sum of the probabilities would be (\frac{5}{16} + \frac{7}{16} + \frac{9}{16} + \frac{11}{16} = \frac{32}{16} = 2 ), which is not equal to 1.

• Option (d) 48 is incorrect because if ( k = 48 ), then the sum of the probabilities would be (\frac{5}{48} + \frac{7}{48} + \frac{9}{48} + \frac{11}{48} = \frac{32}{48} = \frac{2}{3} ), which is not equal to 1.

Solution

We know that:

$ \begin{aligned} E(X) & =\sum _{i=1}^{n} X_i P_i \\ & =(-4)(0.1)+(-3)(0.2)+(-2)(0.3)+(-1)(0.2)+0(0.2) \\ & =-0.4-0.6-0.6-0.2=-1.8 \end{aligned} $

Hence, the correct option is $(d)$.

• Option (a) 0 is incorrect because the expected value ( E(X) ) is calculated as a weighted average of the values of ( X ) with their respective probabilities. The calculation shows that the sum is (-1.8), not 0.

• Option (b) -1 is incorrect because the expected value ( E(X) ) is calculated as (-1.8), not (-1). The sum of the products of each value of ( X ) and its probability does not equal (-1).

• Option (c) -2 is incorrect because the expected value ( E(X) ) is calculated as (-1.8), not (-2). The sum of the products of each value of ( X ) and its probability does not equal (-2).

Solution

We know that

$ \begin{aligned} E(X^{2}) & =\sum _{i=1}^{n} P_i X_i^{2} \\ & =1 \times \frac{1}{10}+4 \times \frac{1}{5}+9 \times \frac{3}{10}+16 \times \frac{2}{5} \end{aligned} $

$ =\frac{1}{10}+\frac{4}{5}+\frac{27}{10}+\frac{32}{5}=\frac{28}{10}+\frac{36}{5}=\frac{100}{10}=10 $

Hence, the correct option is (d).

• Option (a) 3: This option is incorrect because the calculation of (E(X^2)) involves squaring each value of (X) and multiplying by its corresponding probability. The sum of these products does not equal 3. Specifically, the correct calculation yields a value of 10, not 3.

• Option (b) 5: This option is incorrect because, similar to option (a), the correct calculation of (E(X^2)) involves summing the products of each squared value of (X) and its corresponding probability. The sum of these products is 10, not 5.

• Option (c) 7: This option is incorrect because the correct calculation of (E(X^2)) results in a value of 10. The sum of the products of each squared value of (X) and its corresponding probability does not equal 7.

Solution

$\quad P(X=r)={ }^{n} C_r p^{r} q^{n-r}=\frac{n !}{r !(n-r) !} p^{r} \cdot(1-p)^{n-r}$

$ \begin{aligned} P(X=n-r) & ={ }^{n} C _{n-r} p^{n-r} \cdot(q)^{n-(n-r)}={ }^{n} C _{n-r} p^{n-r} \cdot q^{r} \\ & =\frac{n !}{(n-r) !(n-n+r) !} p^{n-r} q^{r}=\frac{n !}{(n-r) ! r !} \cdot p^{n-r} \cdot q^{r} \end{aligned} $

Now $\frac{P(x=r)}{P(x=n-r)}=\frac{\frac{n !}{r !(n-r) !} \cdot p^{r} \cdot(1-p)^{n-r}}{\frac{n !}{r !(n-r) !} \cdot p^{n-r} \cdot(1-p)^{r}}=\frac{(\frac{1-p}{p})^{n-r}}{(\frac{1-p}{p})^{r}}$

The above expression will be independent of $n$ and $r$ if

$ (\frac{1-p}{p})=1 \Rightarrow \frac{1}{p}=2 \Rightarrow p=\frac{1}{2} $

Hence, the correct option is (a).

• Option (b) $\frac{1}{3}$: If ( p = \frac{1}{3} ), then ( 1 - p = \frac{2}{3} ). The ratio (\frac{P(X=r)}{P(X=n-r)}) would be (\left(\frac{\frac{2}{3}}{\frac{1}{3}}\right)^{n-2r} = 2^{n-2r}). This expression is dependent on ( n ) and ( r ), which contradicts the condition that the ratio should be independent of ( n ) and ( r ).

• Option (c) $\frac{1}{5}$: If ( p = \frac{1}{5} ), then ( 1 - p = \frac{4}{5} ). The ratio (\frac{P(X=r)}{P(X=n-r)}) would be (\left(\frac{\frac{4}{5}}{\frac{1}{5}}\right)^{n-2r} = 4^{n-2r}). This expression is dependent on ( n ) and ( r ), which contradicts the condition that the ratio should be independent of ( n ) and ( r ).

• Option (d) $\frac{1}{7}$: If ( p = \frac{1}{7} ), then ( 1 - p = \frac{6}{7} ). The ratio (\frac{P(X=r)}{P(X=n-r)}) would be (\left(\frac{\frac{6}{7}}{\frac{1}{7}}\right)^{n-2r} = 6^{n-2r}). This expression is dependent on ( n ) and ( r ), which contradicts the condition that the ratio should be independent of ( n ) and ( r ).

Solution

Let $E_1$ be the event that the student fails in Physics and $E_2$ be the event that she fails in Mathematics.

$\therefore \quad P(E_1)=\frac{30}{100}, P(E_2)=\frac{25}{100}$ and $P(E_1 \cap E_2)=\frac{10}{100}$

$\therefore P(E_1 / E_2)=\frac{P(E_1 \cap E_2)}{P(E_2)}=\frac{10 / 100}{25 / 100}=\frac{2}{5}$

Hence, the correct option is (b).

• Option (a) $\frac{1}{10}$ is incorrect because it does not match the calculated conditional probability $\frac{2}{5}$ for the event that a student fails in Physics given that they have failed in Mathematics. The correct calculation is $\frac{10/100}{25/100} = \frac{2}{5}$, not $\frac{1}{10}$.

• Option (c) $\frac{9}{20}$ is incorrect because it is not the result of the conditional probability calculation. The correct probability is $\frac{2}{5}$, which simplifies to $\frac{4}{10}$, not $\frac{9}{20}$.

• Option (d) $\frac{1}{3}$ is incorrect because it does not represent the correct conditional probability. The correct calculation yields $\frac{2}{5}$, not $\frac{1}{3}$.

Solution

Let $E_1$ be the event that both of them solve the problem.

$\therefore \quad P(E_1)=\frac{1}{3} \times \frac{1}{4}=\frac{1}{12}$

and $E_2$ be the event that both of them same incorrectly the problem.

$\therefore \quad P(E_2)=(1-\frac{1}{3}) \times(1-\frac{1}{4})=\frac{2}{3} \times \frac{3}{4}=\frac{1}{2}$

Let $H$ be the event that both of them get the same answer.

Here, $P(H / E_1)=1, P(H / E_2)=\frac{1}{20}$

$\therefore P(E_1 / H)=\frac{P(E_1) \cdot P(H / E_1)}{P(E_1) \cdot P(H / E_1)+P(E_2) \cdot P(H / E_2)}$

$=\frac{\frac{1}{12} \times 1}{\frac{1}{12} \times 1+\frac{1}{2} \times \frac{1}{20}}=\frac{\frac{1}{12}}{\frac{1}{12}+\frac{1}{40}}=\frac{\frac{1}{12}}{\frac{10+3}{120}}=\frac{1 / 12}{13 / 120}=\frac{10}{13}$

Hence, the correct option is (d).

• Option (a) $\frac{1}{12}$: This option is incorrect because it represents the probability that both A and B solve the problem correctly independently, not the conditional probability given that they obtained the same answer.

• Option (b) $\frac{1}{40}$: This option is incorrect because it represents the probability that both A and B make a common error independently, not the conditional probability given that they obtained the same answer.

• Option (c) $\frac{13}{120}$: This option is incorrect because it is derived from the sum of the probabilities of both solving the problem correctly and both making a common error, but it does not account for the conditional probability given that they obtained the same answer.

Solution

Here, $n=5, p=\frac{10}{100}=\frac{1}{10}$ and $q=1-\frac{1}{10}=\frac{9}{10}$ and $r \leq 1$

We know that

$ \text{ So } \quad \begin{aligned} P(X=r) & ={ }^{n} C_r p^{r} q^{n-r} \\ P(x \leq 1) & =P(x=0)+P(x=1) \\ & ={ }^{5} C_0(\frac{1}{10})^{0}(\frac{9}{10})^{5}+{ }^{5} C_1(\frac{1}{10})(\frac{9}{10})^{4} \end{aligned} $

$ =(\frac{9}{10})^{5}+\frac{5}{10}(\frac{9}{10})^{4}=(\frac{9}{10})^{5}+\frac{1}{2}(\frac{9}{10})^{4} $

Hence, the correct option is (d).

• Option (a) $(\frac{9}{10})^{5}$ is incorrect because it only accounts for the probability that all 5 pens drawn are non-defective. It does not include the scenario where exactly one pen is defective.

• Option (b) $\frac{1}{2}(\frac{9}{10})^{4}$ is incorrect because it only considers the probability of drawing exactly one defective pen and does not include the probability of drawing no defective pens at all.

• Option (c) $\frac{1}{2}(\frac{9}{10})^{5}$ is incorrect because it incorrectly scales the probability of drawing no defective pens by $\frac{1}{2}$, which is not part of the correct calculation.

Solution

False

• If events ( A ) and ( B ) are mutually exclusive, it means that ( P(A \cap B) = 0 ). However, for events to be independent, the probability of their intersection must equal the product of their individual probabilities, i.e., ( P(A \cap B) = P(A) \cdot P(B) ). Since ( P(A) > 0 ) and ( P(B) > 0 ), ( P(A) \cdot P(B) > 0 ). Therefore, ( P(A \cap B) ) cannot be both 0 and greater than 0 simultaneously. Hence, events ( A ) and ( B ) cannot be both mutually exclusive and independent.

Solution

True

• The statement is true because if ( A ) and ( B ) are independent events, then the probability of their complements ( A’ ) and ( B’ ) occurring together can be shown to be the product of their individual probabilities. Specifically, ( P(A’ \cap B’) = P(A’) \cdot P(B’) ). This follows from the fact that ( P(A’) = 1 - P(A) ) and ( P(B’) = 1 - P(B) ), and the independence of ( A ) and ( B ) implies ( P(A \cap B) = P(A) \cdot P(B) ). Therefore, the complements ( A’ ) and ( B’ ) are also independent.

Solution

False

• Reason 1: Mutually exclusive events cannot be independent because if one event occurs, the probability of the other event occurring is zero. For two events to be independent, the occurrence of one event should not affect the probability of the other event occurring.

• Reason 2: For mutually exclusive events A and B, P(A ∩ B) = 0. However, for independent events, P(A ∩ B) should equal P(A) * P(B). Since P(A ∩ B) = 0 for mutually exclusive events, and P(A) * P(B) is generally not zero unless one of the events has a probability of zero, mutually exclusive events cannot be independent.

• Reason 3: Independence implies that the occurrence of one event does not provide any information about the occurrence of the other event. In contrast, if events are mutually exclusive, the occurrence of one event completely rules out the occurrence of the other event, which means they are not independent.

Solution

False

• Independent events are events where the occurrence of one event does not affect the probability of the other event occurring. Mutually exclusive events, on the other hand, are events that cannot occur at the same time. If two events are mutually exclusive, the occurrence of one event means the other event cannot occur, which directly contradicts the definition of independent events. Therefore, independent events cannot be mutually exclusive.

Solution

True

• The statement is true because, by definition, if two events (A) and (B) are independent, the probability of both events occurring together (i.e., (P(A \text{ and } B))) is the product of their individual probabilities, (P(A) \cdot P(B)). This is a fundamental property of independent events in probability theory.

Solution

True $\qquad [\because E(X)=\sum X_i P(X_i)]$

• The statement is true because the mean of a probability distribution is indeed referred to as the expected value. The expected value, denoted as ( E(X) ), is calculated as the sum of each possible value of the random variable ( X ) multiplied by its corresponding probability ( P(X_i) ). This is mathematically represented as ( E(X) = \sum X_i P(X_i) ).

Solution

True $\quad[\because P(A^{\prime} \cup B)=1-P(A \cap B^{\prime})=1-P(A) \cdot P(B^{\prime})]$

To provide reasons why each of the other options is incorrect, we need to consider the context of the problem and the given answer. The problem states that if ( A ) and ( B’ ) are independent events, then ( P(A’ \cup B) = 1 - P(A) \cdot P(B’) ). The given answer is “True” with the explanation provided.

Here are the reasons why other options (i.e., “False”) would be incorrect:

1. Incorrect Reasoning for “False” Option:
   • Reason 1: The given statement is mathematically correct based on the properties of probability and independence of events. Specifically, for independent events ( A ) and ( B’ ), the probability of their intersection is ( P(A \cap B’) = P(A) \cdot P(B’) ). Using the complement rule and the union rule, we get ( P(A’ \cup B) = 1 - P(A \cap B’) = 1 - P(A) \cdot P(B’) ). Therefore, stating “False” would contradict these established probability rules.
   • Reason 2: The derivation provided in the answer is accurate. It correctly applies the complement rule ( P(A’) = 1 - P(A) ) and the fact that ( P(A \cup B) = 1 - P(A’ \cap B’) ) for independent events. Hence, any claim that the statement is “False” would ignore these correct applications of probability laws.
   • Reason 3: The independence of ( A ) and ( B’ ) is crucial in the given problem. If someone claims “False,” they might be misunderstanding or misapplying the concept of independence. The independence ensures that ( P(A \cap B’) = P(A) \cdot P(B’) ), which is a key step in the provided solution. Ignoring this would lead to an incorrect conclusion.

In summary, the reasons why the “False” option is incorrect are rooted in the proper application of probability rules and the correct understanding of event independence. The given statement is mathematically sound, and any claim to the contrary would be based on a misunderstanding of these principles.

Solution

True

To determine why each of the other options is incorrect, we need to understand the given statement and the nature of independent events.

Given:

• ( A ) and ( B ) are independent events.
• The statement to verify is: ( P(\text{exactly one of } A \text{ or } B \text{ occurs}) = P(A) \cdot P(B’) + P(B) \cdot P(A’) ).

Reasoning:

1. Incorrect Option: ( P(A \cup B) = P(A) + P(B) )

   • This is incorrect because ( P(A \cup B) ) represents the probability that either event ( A ) or event ( B ) or both occur. For independent events, the correct formula is: [ P(A \cup B) = P(A) + P(B) - P(A \cap B) ]
   • Since ( A ) and ( B ) are independent, ( P(A \cap B) = P(A) \cdot P(B) ). Therefore: [ P(A \cup B) = P(A) + P(B) - P(A) \cdot P(B) ]
   • The given option does not account for the intersection term ( P(A \cap B) ), making it incorrect.

2. Incorrect Option: ( P(A \cap B) = P(A) + P(B) )

   • This is incorrect because ( P(A \cap B) ) represents the probability that both events ( A ) and ( B ) occur simultaneously. For independent events, the correct formula is: [ P(A \cap B) = P(A) \cdot P(B) ]
   • The given option incorrectly suggests that the probability of both events occurring is the sum of their individual probabilities, which is not true for any events, independent or not.

3. Incorrect Option: ( P(A \cap B’) = P(A) \cdot P(B) )

   • This is incorrect because ( P(A \cap B’) ) represents the probability that event ( A ) occurs and event ( B ) does not occur. For independent events, the correct formula is: [ P(A \cap B’) = P(A) \cdot P(B’) ]
   • Since ( B’ ) (the complement of ( B )) is independent of ( A ), we have: [ P(B’) = 1 - P(B) ]
   • Therefore: [ P(A \cap B’) = P(A) \cdot (1 - P(B)) ]
   • The given option incorrectly uses ( P(B) ) instead of ( P(B’) ).

4. Incorrect Option: ( P(A \cup B’) = P(A) \cdot P(B’) )

   • This is incorrect because ( P(A \cup B’) ) represents the probability that either event ( A ) occurs or event ( B ) does not occur. For independent events, the correct formula is: [ P(A \cup B’) = P(A) + P(B’) - P(A \cap B’) ]
   • Since ( A ) and ( B’ ) are independent, ( P(A \cap B’) = P(A) \cdot P(B’) ). Therefore: [ P(A \cup B’) = P(A) + P(B’) - P(A) \cdot P(B’) ]
   • The given option incorrectly suggests that the probability of the union of ( A ) and ( B’ ) is the product of their probabilities, which is not true.

Conclusion:

The given statement ( P(\text{exactly one of } A \text{ or } B \text{ occurs}) = P(A) \cdot P(B’) + P(B) \cdot P(A’) ) is true, and the reasons for the incorrectness of each of the other options are provided above.

Solution

False

$ \begin{matrix} {[\because P(B / A)=\frac{P(A \cap B)}{P(A)}=\frac{P(A)+P(B)-P(A \cup B)}{P(A)}}>\frac{1-P(A \cup B)}{P(A)}] \end{matrix} $

• The given inequality ( P(B / A) \geq 1 - \frac{P(B’)}{P(A)} ) is incorrect because the correct expression for ( P(B / A) ) is ( \frac{P(A \cap B)}{P(A)} ). The inequality ( P(B / A) > \frac{1 - P(A \cup B)}{P(A)} ) does not necessarily imply ( P(B / A) \geq 1 - \frac{P(B’)}{P(A)} ).

• The expression ( 1 - \frac{P(B’)}{P(A)} ) simplifies to ( 1 - \frac{1 - P(B)}{P(A)} = \frac{P(A) + P(B) - 1}{P(A)} ). This does not directly relate to the probability ( P(B / A) ) in a straightforward manner.

• The inequality ( P(A) + P(B) > 1 ) implies that ( P(A \cup B) ) is at least ( P(A) + P(B) - 1 ), but this does not directly lead to the given inequality involving conditional probability.

• The correct approach to conditional probability involves understanding the relationship between ( P(A \cap B) ) and ( P(A) ), not the complement of ( B ).

Solution

True

Since $P$ (atleast two of $A, B$ and $C$ occur)

$ \begin{aligned} & =p \times p \times(1-p)+(1-p) \cdot p \cdot p+p(1-p) \cdot p+p \cdot p \cdot p \\ & =3 p^{2}(1-p)+p^{3}=3 p^{2}-3 p^{3}+p^{3}=3 p^{2}-2 p^{3} \end{aligned} $

To determine why other options are incorrect, let’s analyze the given problem and the provided solution in detail.

Analysis of the Problem

We are given three independent events ( A ), ( B ), and ( C ) with equal probabilities ( P(A) = P(B) = P(C) = p ). We need to find the probability that at least two of these events occur.

Correct Solution

The correct solution is given as: [ P(\text{at least two of } A, B, \text{ and } C \text{ occur}) = 3p^2 - 2p^3 ]

Detailed Calculation

To find the probability that at least two of the events occur, we can use the principle of inclusion-exclusion:

1. Calculate the probability of exactly two events occurring:

   • ( P(A \cap B \cap \neg C) = p \cdot p \cdot (1 - p) = p^2(1 - p) )
   • ( P(A \cap \neg B \cap C) = p \cdot (1 - p) \cdot p = p^2(1 - p) )
   • ( P(\neg A \cap B \cap C) = (1 - p) \cdot p \cdot p = p^2(1 - p) )

   Summing these probabilities: [ 3p^2(1 - p) ]

2. Calculate the probability of all three events occurring:

   • ( P(A \cap B \cap C) = p \cdot p \cdot p = p^3 )

3. Sum the probabilities of exactly two events and all three events: [ 3p^2(1 - p) + p^3 = 3p^2 - 3p^3 + p^3 = 3p^2 - 2p^3 ]

Conclusion

The provided solution is correct. Now, let’s consider why other options might be incorrect.

Reasons for Incorrect Options

1. Option: ( 3p^2 )

   • This option does not account for the overlap where all three events occur. It only considers the probability of exactly two events occurring, which is ( 3p^2(1 - p) ). It misses the ( -2p^3 ) term that adjusts for the overcounting of the scenario where all three events occur.

2. Option: ( 2p^2 - p^3 )

   • This option underestimates the probability. It does not correctly account for the number of ways two events can occur and the adjustment for all three events occurring. The correct formula should be ( 3p^2 - 2p^3 ), not ( 2p^2 - p^3 ).

3. Option: ( p^2(3 - 2p) )

   • While this option looks similar to the correct answer, it is not in the simplified form. The correct simplified form is ( 3p^2 - 2p^3 ). This option might be a result of incorrect algebraic manipulation or misunderstanding of the inclusion-exclusion principle.

4. Option: ( p^3 + 3p^2(1 - p) )

   • This option is actually correct in its expanded form but is not simplified. It represents the same calculation as the correct answer but is not presented in the simplified form ( 3p^2 - 2p^3 ). Therefore, while not incorrect in calculation, it is not the simplified version required.

Summary

• Option ( 3p^2 ): Incorrect because it does not account for the overlap of all three events occurring.
• Option ( 2p^2 - p^3 ): Incorrect because it underestimates the probability.
• Option ( p^2(3 - 2p) ): Incorrect because it is not in the simplified form.
• Option ( p^3 + 3p^2(1 - p) ): Correct in calculation but not in the simplified form.

These are the reasons why each of the other options is incorrect.

Solution

Given that: $P(A)=p, P(B)=\frac{1}{3}$ and $P(A \cup B)=\frac{5}{9}$

$ \begin{aligned} P(A / B) & =\frac{P(A \cap B)}{P(B)}=p \Rightarrow P(A \cap B)=p \cdot P(B)=p \cdot \frac{1}{3} \\ \text{ and } P(A \cup B) & =P(A)+P(B)-P(A \cap B) \\ \frac{5}{9}=p+\frac{1}{3} & -\frac{p}{3} \Rightarrow \frac{5}{9}-\frac{1}{3}=\frac{2 p}{3} \Rightarrow \frac{2}{9}=\frac{2 p}{3} \Rightarrow p=\frac{1}{3} \end{aligned} $

Hence, $p$ is equal to $\frac{1}{3}$.

Solution

Here,

$ P(A^{\prime} \cup B^{\prime})=\frac{2}{3} \text{ and } P(A \cup B)=\frac{5}{9} $

$ \begin{aligned} & \therefore & 1-P(A \cap B) & =\frac{2}{3} \\ & \Rightarrow & P(A \cap B) & =1-\frac{2}{3}=\frac{1}{3} \end{aligned} $

Now $\quad P(A^{\prime})+P(B^{\prime})=1-P(A)+1-P(B)=2-[P(A)+P(B)]$

$ \begin{aligned} & =2-[P(A \cup B)+P(A \cap B)] \\ & =2-[\frac{5}{9}+\frac{1}{3}]=2-\frac{8}{9}=\frac{10}{9} \end{aligned} $

Hence, the value of the filler is $\frac{10}{9}$.

Solution

Given that: $P(X=2)=9 P(X=3)$

$ \Rightarrow{ }^{5} C_2 p^{2} q^{3}=9 .{ }^{5} C_3 p^{3} q^{2} $

$ \begin{matrix} \Rightarrow \frac{1}{9} =\frac{{ }^{5} C_3 p^{3} q^{2}}{{ }^{5} C_2 p^{2} q^{3}} \Rightarrow \frac{1}{9}=\frac{p}{q} \quad[\because{ }^{5} C_3={ }^{5} C_2] \\ \Rightarrow 9 p =q \\ \Rightarrow 9 p =1-p \Rightarrow 9 p+p=1 \Rightarrow 10 p=1 \therefore p=\frac{1}{10} \end{matrix} $

Hence, the value of the filler is $\frac{1}{10}$.

Solution

$\quad Var(X)=E(X^{2})-[E(X)]^{2}$

$=\sum X^{2} P(X)-[\sum X . P(X)]^{2}=\sum p_i x_i^{2}-(\sum p_i x_i)^{2}$

Hence, $Var(X)$ is equal to $\sum p_i x_i^{2}-(\sum p_i x_i)^{2}$.

Solution

$ \begin{matrix} \because \qquad P(A / B) =\frac{P(A \cap B)}{P(B)} \\ \Rightarrow P(A) =\frac{P(A \cap B)}{P(B)} \\ \Rightarrow P(A \cap B) =P(A) \cdot P(B) \end{matrix} $

So, $A$ is independent of $B$.