Solution

We know that $\frac{5 \pi}{6} \notin(-\frac{\pi}{2}, \frac{\pi}{2})$ and $\frac{13 \pi}{6} \notin[0, \pi]$

$\therefore \tan ^{-1}(\tan \frac{5 \pi}{6})+\cos ^{-1}(\cos \frac{13 \pi}{6})$

$=\tan ^{-1}[\tan (\pi-\frac{\pi}{6})]+\cos ^{-1}[\cos (2 \pi+\frac{\pi}{6})]$

$=\tan ^{-1}[\tan (-\frac{\pi}{6})]+\cos ^{-1}(\cos \frac{\pi}{6})$

$=\tan ^{-1}(-\tan \frac{\pi}{6})+\cos ^{-1}(\cos \frac{\pi}{6})$

$=-\tan ^{-1}(\tan \frac{\pi}{6})+\cos ^{-1}(\cos \frac{\pi}{6}) \quad[\because \tan ^{-1}(-x)=-\tan ^{-1} x]$

$=-\frac{\pi}{6}+\frac{\pi}{6}=0$

Hence, $\tan ^{-1}(\tan \frac{5 \pi}{6})+\cos ^{-1}(\cos \frac{13 \pi}{6})=0$

Solution

$\cos [\cos ^{-1}(\frac{-\sqrt{3}}{2})+\frac{\pi}{6}]$

$=\cos [\pi-\cos ^{-1} \frac{\sqrt{3}}{2}+\frac{\pi}{6}][\because \cos ^{-1}(-x)=\pi-\cos ^{-1} x]$

$=\cos [\pi-\frac{\pi /}{6}+\frac{\pi /}{6}]=\cos \pi=-1$

$ \text{ Hence, } \cos [\cos ^{-1}(\frac{-\sqrt{3}}{2})+\frac{\pi}{6}]=-1 \text{. } $

Solution

L.H.S. $\cot (\frac{\pi}{4}-2 \cot ^{-1} 3)$

$=\cot [\tan ^{-1}(1)-2 \tan ^{-1} \frac{1}{3}] \quad[\because \cot ^{-1} x=\tan ^{-1} \frac{1}{x}]$

$=\cot [\tan ^{-1}(1)-\tan ^{-1} \frac{2 \times \frac{1}{3}}{1-(\frac{1}{3})^{2}}][\because 2 \tan ^{-1} x=\tan ^{-1} \frac{2 x}{1-x^{2}}]$

$=\cot [\tan ^{-1}(1)-\tan ^{-1} \frac{\frac{2}{3}}{\frac{8}{9}}]$

$=\cot [\tan ^{-1}(1)-\tan ^{-1} \frac{3}{4}]$

$=\cot [\tan ^{-1}(\frac{1-\frac{3}{4}}{1+1 \times \frac{3}{4}})]=\cot [\tan ^{-1}(\frac{\frac{1}{4}}{\frac{7}{4}})]$

$=\cot [\tan ^{-1} \frac{1}{7}] \quad[\because \tan ^{-1} \frac{1}{x}=\cot ^{-1} x]$

$=\cot [\cot ^{-1}(7)]=7$ R.H.S.

Hence proved.

Solution

$\tan ^{-1}(\frac{-1}{\sqrt{3}})+\cot ^{-1}(\frac{1}{\sqrt{3}})+\tan ^{-1}[\sin (\frac{-\pi}{2})]$ $=-\tan ^{-1}(\frac{1}{\sqrt{3}})+\tan ^{-1}(\sqrt{3})+\tan ^{-1}(-1)$

$ \begin{bmatrix} \because \tan ^{-1}(-x)=-\tan ^{-1} x \\ \tan ^{-1} x=\cot ^{-1}(\frac{1}{x}) \\ \sin (\frac{-\pi}{2})=-1 \end{bmatrix} $

$ =-\frac{\pi}{6}+\frac{\pi}{3}-\frac{\pi}{4}=\frac{-\pi}{12} \quad[\because \tan ^{-1}(-1)=\frac{-\pi}{4}] $

Hence, $\tan ^{-1}(\frac{-1}{\sqrt{3}})+\cot ^{-1}(\frac{1}{\sqrt{3}})+\tan ^{-1}[\sin (\frac{-\pi}{2})]=\frac{-\pi}{12}$

Solution

We know that $\frac{2 \pi}{3} \notin[\frac{-\pi}{2}, \frac{\pi}{2}]$

$ \begin{aligned} \therefore \tan ^{-1}(\tan \frac{2 \pi}{3}) & =\tan ^{-1}[\tan (\pi-\frac{\pi}{3})]=\tan ^{-1}(-\tan \frac{\pi}{3}) \\ & =-\tan ^{-1}(\tan \frac{\pi}{3})[\because \tan ^{-1}(-x)=-\tan ^{-1} x] \\ & =-\frac{\pi}{3} \in[-\frac{\pi}{2}, \frac{\pi}{2}] \end{aligned} $

Hence, $\tan ^{-1}(\tan \frac{2 \pi}{3})=\frac{-\pi}{3}$.

Solution

L.H.S. $2 \tan ^{-1}(-3)=-2 \tan ^{-1}(3)$

$ \begin{aligned} & =-\cos ^{-1}[\frac{1-(3)^{2}}{1+(3)^{2}}][\because 2 \tan ^{-1} x=\cos ^{-1}(\frac{1-x^{2}}{1+x^{2}})] \\ & =-\cos ^{-1}(\frac{1-9}{1+9})=-\cos ^{-1}(\frac{-8}{10}) \\ & =-\cos ^{-1}(\frac{-4}{5})=-[\pi-\cos ^{-1}(\frac{4}{5})]=-\pi+\cos ^{-1} \frac{4}{5} \\ & =-\pi+\tan ^{-1}(\frac{3}{4}) \quad[\because \cos ^{-1} \frac{4}{5}=\tan ^{-1} \frac{3}{4}] \end{aligned} $

$ \begin{matrix} =-\pi+\frac{\pi}{2}-\cot ^{-1}(\frac{3}{4}) & {[\tan ^{-1} x=\frac{\pi}{2}-\cot ^{-1} x]} \\ =\frac{-\pi}{2}-\cot ^{-1}(\frac{3}{4}) & {[\because \tan ^{-1} x=\cot ^{-1} \frac{1}{x}]} \\ =\frac{-\pi}{2}-\tan ^{-1}(\frac{4}{3}) & \\ =\frac{-\pi}{2}+\tan ^{-1}(-\frac{4}{3}) \text{ R.H.S. } & \end{matrix} $

Hence proved.

Solution

$\tan ^{-1} \sqrt{x(x+1)}+\sin ^{-1} \sqrt{x^{2}+x+1}=\frac{\pi}{2}$

$ \text{ Let } \quad \theta=\sin ^{-1} \sqrt{x^{2}+x+1} $

$\therefore \quad \sin \theta=\sqrt{x^{2}+x+1}$

$\Rightarrow \tan \theta=\frac{\sqrt{x^{2}+x+1}}{\sqrt{-x^{2}-x}} \Rightarrow \theta=\tan ^{-1}(\frac{\sqrt{x^{2}+x+1}}{\sqrt{-x^{2}-x}})$

$\Rightarrow \sin ^{-1} \sqrt{x^{2}+x+1}=\tan ^{-1}(\sqrt{\frac{x^{2}+x+1}{-x^{2}-x}})$

So, $\tan ^{-1} \sqrt{x(x+1)}+\tan ^{-1}(\sqrt{\frac{x^{2}+x+1}{-x^{2}-x}})=\frac{\pi}{2}$

$\Rightarrow \tan ^{-1}[\frac{\sqrt{x(x+1)}+\sqrt{\frac{x^{2}+x+1}{-x(x+1)}}}{1-\sqrt{x(x+1)} \times \sqrt{\frac{x^{2}+x+1}{-x(x+1)}}}]=\frac{\pi}{2}$

$\Rightarrow \tan ^{-1}[\frac{\frac{x(x+1)+\sqrt{-(x^{2}+x+1)}}{\sqrt{x(x+1)}}}{1-\sqrt{-(x^{2}+x+1)}}]=\frac{\pi}{2}$ $\Rightarrow \frac{x^{2}+x-\sqrt{-(x^{2}+x+1)}}{[1-\sqrt{-(x^{2}+x+1)}] \sqrt{x^{2}+x}}=\tan \frac{\pi}{2}=\frac{1}{0}$ $\Rightarrow[1-\sqrt{-(x^{2}+x+1)}] \sqrt{x^{2}+x}=0$ $\Rightarrow[1-\sqrt{-(x^{2}+x+1)}]=0$ or $\sqrt{x^{2}+x}=0$

Here, $\sqrt{-(x^{2}+x+1)} \notin R$

$\therefore \sqrt{x^{2}+x}=0$

$\Rightarrow \quad x^{2}+x=0 \Rightarrow x(x+1)=0$

$\Rightarrow \quad x=0$ or $x+1=0 \Rightarrow x=0$ or $x=-1$

Hence the real solutions are $x=0$ and $x=-1$.

Alternate Method

$ \begin{aligned} & \tan ^{-1} \sqrt{x(x+1)}+\sin ^{-1} \sqrt{x^{2}+x+1}=\frac{\pi}{2} \\ & \Rightarrow \tan ^{-1} \sqrt{x^{2}+x}=\frac{\pi}{2}-\sin ^{-1} \sqrt{x^{2}+x+1} \\ & \Rightarrow \tan ^{-1} \sqrt{x^{2}+x}=\cos ^{-1} \sqrt{x^{2}+x+1}[\because \sin ^{-1} x+\cos ^{-1} x=\frac{\pi}{2}] \\ & \Rightarrow \cos ^{-1}[\frac{1}{\sqrt{1+x^{2}+x}}]=\cos ^{-1} \sqrt{x^{2}+x+1} \\ & \Rightarrow \quad \frac{1}{\sqrt{x^{2}+x+1}}=\sqrt{x^{2}+x+1} \quad[\because \tan ^{-1} x=\cos ^{-1} \frac{1}{\sqrt{1+x^{2}}}] \\ & \Rightarrow \quad x^{2}+x+1=1 \quad \Rightarrow x^{2}+x=0 \\ & \Rightarrow \quad x(x+1)=0 \quad \Rightarrow x=0 \text{ or } x+1=0 \\ & \therefore \quad x=0, x=-1 \end{aligned} $

Solution

$\sin (2 \tan ^{-1} \frac{1}{3})+\cos (\tan ^{-1} 2 \sqrt{2})$

$ \begin{aligned} & \Rightarrow \sin [\tan ^{-1}(\frac{2 \times \frac{1}{3}}{1-(\frac{1}{3})^{2}})]+\cos [\cos ^{-1} \frac{1}{\sqrt{1+(2 \sqrt{2})^{2}}}] \\ & {[\because \tan ^{-1} x=\cos ^{-1}(\frac{1}{\sqrt{1+x^{2}}})]} \\ & \Rightarrow \sin [\tan ^{-1}(\frac{\frac{2}{3}}{1-\frac{1}{9}})]+\cos [\cos ^{-1}(\frac{1}{3})] \\ & .\Rightarrow \sin [\tan ^{-1}(\frac{3}{4})]+\frac{1}{3} \Rightarrow \sin ^{-1}(\frac{3}{5})]+\frac{1}{3} \\ & \Rightarrow \frac{3}{5}+\frac{1}{3} \Rightarrow \frac{14}{15} \\ & \text{ Hence, } \sin (2 \tan ^{-1} \frac{1}{3})+\cos (\tan ^{-1} 2 \sqrt{2})=\frac{14}{15} . \end{aligned} $

Solution

$2 \tan ^{-1}(\cos \theta)=\tan ^{-1}(2 cosec \theta)$

$ \begin{aligned} & \Rightarrow \tan ^{-1}(\frac{2 \cos \theta}{1-\cos ^{2} \theta})=\tan ^{-1}(2 cosec \theta) \\ & \Rightarrow \quad[\because 2 \tan ^{-1} x=\tan ^{-1} \frac{2 x}{1-x^{2}}] \\ & \Rightarrow \quad \frac{2 \cos \theta}{1-\cos ^{2} \theta}=2 cosec \theta \Rightarrow \frac{2 \cos \theta}{\sin ^{2} \theta}=\frac{2}{\sin \theta} \\ & \Rightarrow \quad \cos \theta \sin \theta-\sin ^{2} \theta=0 \Rightarrow \sin \theta(\cos \theta-\sin \theta)=0 \\ & \Rightarrow \quad \sin \theta=0 \text{ or } \quad \cos \theta-\sin \theta=0 \\ & \Rightarrow \quad \sin \theta=0 \quad \text{ or } \quad 1-\tan \theta=0 \\ & \Rightarrow \quad \theta=0 \text{ or } \quad \tan \theta=1 \\ & \Rightarrow \quad \theta=0^{\circ} \text{ or } \quad \theta=\frac{\pi}{4} \text{ Hence proved. } \end{aligned} $

Solution

L.H.S. $\cos (2 \tan ^{-1} \frac{1}{7})$

$ \begin{aligned} & =\cos [\cos ^{-1} \frac{1-\frac{1}{49}}{1+\frac{1}{49}}] \quad[\because 2 \tan ^{-1} x=\cos ^{-1} \frac{1-x^{2}}{1+x^{2}}] \\ & =\cos [\cos ^{-1} \frac{48}{50}]=\cos [\cos ^{-1} \frac{24}{25}]=\frac{24}{25} \end{aligned} $

R.H.S. $\sin [4 \tan ^{-1} \frac{1}{3}]$

$ \begin{aligned} & =\sin [2 \tan ^{-1}(\frac{2 \times \frac{1}{3}}{1-\frac{1}{9}})][\because 2 \tan ^{-1} x=\tan ^{-1} \frac{2 x}{1-x^{2}}] \\ & =\sin [2 \tan ^{-1}(\frac{\frac{2}{3}}{\frac{3}{9}})]=\sin [2 \tan ^{-1} \frac{3}{4}] \\ & =\sin [\sin ^{-1} \frac{2 \times \frac{3}{4}}{1+\frac{9}{16}}][\because 2 \tan ^{-1} x=\sin ^{-1} \frac{2 x}{1+x^{2}}] \\ & =\sin [\sin ^{-1} \frac{24}{25}] \Rightarrow \frac{24}{25} \end{aligned} $

L.H.S. = R.H.S. Hence proved.

Solution

Given that $\cos (\tan ^{-1} x)=\sin (\cot ^{-1} \frac{3}{4})$

$ \begin{aligned} \Rightarrow \cos [\cos ^{-1} \frac{1}{\sqrt{1+x^{2}}}]=\sin [\sin ^{-1} \frac{4}{5}] \\ { \begin{bmatrix} \because \tan ^{-1} x=\cos ^{-1}(\frac{1}{\sqrt{1+x^{2}}}) \\ \cot ^{-1} x=\sin ^{-1}(\frac{1}{\sqrt{1+x^{2}}}) \end{bmatrix} } \end{aligned} $

$ \Rightarrow \quad \frac{1}{\sqrt{1+x^{2}}}=\frac{4}{5} $

Squaring both sides we get,

$ \begin{aligned} & \frac{1}{1+x^{2}}=\frac{16}{25} \Rightarrow 1+x^{2}=\frac{25}{16} \\ & \Rightarrow \quad x^{2}=\frac{25}{16}-1=\frac{9}{16} \Rightarrow x= \pm \frac{3}{4} \\ & \text{ Hence, } \quad x=\frac{-3}{4}, \frac{3}{4} \text{. } \end{aligned} $

Solution

L.H.S. $\tan ^{-1}[\frac{\sqrt{1+x^{2}}+\sqrt{1-x^{2}}}{\sqrt{1+x^{2}}-\sqrt{1-x^{2}}}]$

Put $x^{2}=\cos \theta \quad \therefore \theta=\cos ^{-1} x^{2}$

$\Rightarrow \tan ^{-1}[\frac{\sqrt{1+\cos \theta}+\sqrt{1-\cos \theta}}{\sqrt{1+\cos \theta}-\sqrt{1-\cos \theta}}]$

$\Rightarrow \tan ^{-1}[\frac{\sqrt{2 \cos ^{2} \theta / 2}+\sqrt{2 \sin ^{2} \theta / 2}}{\sqrt{2 \cos ^{2} \theta / 2}-\sqrt{2 \sin ^{2} \theta / 2}}] \begin{cases} \because 1+\cos \theta=2 \cos ^{2} \theta / 2 \\ 1-\cos \theta=2 \sin ^{2} \theta / 2 \end{cases} $

$\Rightarrow \tan ^{-1}[\frac{\cos \theta / 2+\sin \theta / 2}{\cos \theta / 2-\sin \theta / 2}]$

$\Rightarrow \tan ^{-1}[\frac{1+\tan \theta / 2}{1-\tan \theta / 2}] \quad$ [Dividing the Nr. and Den. by $\cos \theta / 2$ ]

$\Rightarrow \tan [\tan (\frac{\pi}{4} \quad \frac{\theta}{2})] \quad[\because \frac{1+\tan \theta}{1-\tan \theta}=\tan (\frac{\pi}{4}+\theta)]$

$\Rightarrow \frac{\pi}{4}+\frac{\theta}{2} \Rightarrow \frac{\pi}{4}+\frac{1}{2} \cos ^{-1} x^{2}$ R.H.S. $\quad$ [Putting $\theta=\cos ^{-1} x^{2}$ ]

Hence proved.

Solution

Given that $\cos ^{-1}(\frac{3}{5} \cos x+\frac{4}{5} \sin x)$

Put $\quad \frac{3}{5}=\cos y$

$\therefore \quad \sqrt{1-\cos ^{2} y}=\sin y \Rightarrow \sqrt{1-\frac{9}{25}}=\sin y \Rightarrow \frac{4}{5}=\sin y$

$\therefore \cos ^{-1}[\frac{3}{5} \cos x+\frac{4}{5} \sin x]=\cos ^{-1}[\cos y \cos x+\sin y \sin x]$

$=\cos ^{-1}[\cos (y-x)]=y-x$

$=\tan ^{-1} \frac{4}{3}-x$ $\frac{8}{7}+\sin ^{-1} \frac{3}{5}=\sin ^{-1} \frac{77}{85}$

Solution

L.H.S. $\sin ^{-1} \frac{8}{17}+\sin ^{-1} \frac{3}{5}$

$ \begin{aligned} & \text{ Using } \sin ^{-1} x+\sin ^{-1} y=\sin ^{-1}[x \sqrt{1-y^{2}}+y \sqrt{1-x^{2}}] \\ & \begin{aligned} \sin ^{-1} \frac{8}{17}+ & \sin ^{-1} \frac{3}{5}=\sin ^{-1}[\frac{8}{17} \cdot \sqrt{1-(\frac{3}{5})^{2}}+\frac{3}{5} \cdot \sqrt{1-(\frac{8}{17})^{2}}] \\ & =\sin ^{-1}[\frac{8}{17} \cdot \sqrt{1-\frac{9}{25}}+\frac{3}{5} \cdot \sqrt{1-\frac{64}{289}}] \\ & =\sin ^{-1}[\frac{8}{17} \cdot \sqrt{\frac{16}{25}}+\frac{3}{5} \cdot \sqrt{\frac{225}{289}}] \\ & =\sin ^{-1}[\frac{8}{17} \cdot \frac{4}{5}+\frac{3}{5} \cdot \frac{15}{17}]=\sin ^{-1}[\frac{32}{85}+\frac{45}{85}] \\ & =\sin ^{-1} \frac{77}{85} \text{ R.H.S. Hence proved. } \end{aligned} \end{aligned} $

Solution

Let $\sin ^{-1} \frac{5}{13}=x \quad \Rightarrow \quad \sin x=\frac{5}{13}$

$\Rightarrow \quad \tan x=\frac{5}{12}$

Let $\cos ^{-1} \frac{3}{5}=y \quad \Rightarrow \quad \cos y=\frac{3}{5}$

$\Rightarrow \quad \tan y=\frac{4}{3}$

Now $\quad \tan (x+y)=\frac{\tan x+\tan y}{1-\tan x \tan y}$

$\Rightarrow \quad \tan (x+y)=\frac{\frac{5}{12}+\frac{4}{3}}{1-\frac{5}{12} \times \frac{4}{3}}=\frac{\frac{15+48}{36}}{\frac{36-20}{36}}=\frac{63}{16}$

$\Rightarrow \quad x+y=\tan ^{-1} \frac{63}{16}$

$\therefore \sin ^{-1} \frac{5}{13}+\cos ^{-1} \frac{3}{5}=\tan ^{-1} \frac{63}{16}$ Hence proved.

Solution

$\tan ^{-1} \frac{1}{4}+\tan ^{-1} \frac{2}{9}=\tan ^{-1}[\frac{\frac{1}{4}+\frac{2}{9}}{1-\frac{1}{4} \times \frac{2}{9}}]$

$[\because \tan ^{-1} x+\tan ^{-1} y=\tan ^{-1}(\frac{x+y}{1-x y})]$

$\Rightarrow \tan ^{-1}[\frac{\frac{9+8}{36}}{\frac{36-2}{36}}]=\tan ^{-1}[\frac{17}{34}]$

Let $\quad \tan ^{-1}[\frac{17}{34}]=x$

$\therefore \quad \tan x=\frac{17}{34}=\frac{1}{2}$

$ \begin{aligned} \sin x & =\frac{1}{\sqrt{5}} \\ \therefore \quad \tan ^{-1} \frac{1}{2} & =\sin ^{-1} \frac{1}{\sqrt{5}} \text{ R.H.S. } \end{aligned} $

Hence, $\tan ^{-1} \frac{1}{4}+\tan ^{-1} \frac{2}{9}=\sin ^{-1} \frac{1}{\sqrt{5}}$

Solution

$4 \tan ^{-1} \frac{1}{5}-\tan ^{-1} \frac{1}{239}$

$\Rightarrow 2 \cdot(2 \tan ^{-1} \frac{1}{5})-\tan ^{-1} \frac{1}{239}$

$\Rightarrow 2[\tan ^{-1} \frac{2 \times \frac{1}{5}}{1-\frac{1}{25}}]-\tan ^{-1} \frac{1}{239} \quad[2 \tan ^{-1} x=\tan ^{-1} \frac{2 x}{1-x^{2}}]$

$\Rightarrow 2 \tan ^{-1} \frac{5}{12}-\tan ^{-1} \frac{1}{239} \Rightarrow \tan ^{-1}(\frac{2 \times \frac{5}{12}}{1-\frac{25}{144}})-\tan ^{-1}(\frac{1}{239})$

$\Rightarrow \tan ^{-1}(\frac{120}{119})-\tan ^{-1}(\frac{1}{239})$

$\Rightarrow \tan ^{-1}[\frac{\frac{120}{119}-\frac{1}{239}}{1+\frac{120}{119} \times \frac{1}{239}}][\because \tan ^{-1} x-\tan ^{-1} y=\tan ^{-1} \frac{x-y}{1+x y}]$

$\Rightarrow \tan ^{-1}[\frac{120 \times 239-119}{119 \times 239+120}] \Rightarrow \tan ^{-1}[\frac{28680-119}{28441+120}]$

$\Rightarrow \tan ^{-1}[\frac{28561}{28561}]=\tan ^{-1}(1)=\frac{\pi}{4}$

Solution

To prove that $\tan (\frac{1}{2} \sin ^{-1} \frac{3}{4})=\frac{4-\sqrt{7}}{3}$ L.H.S. Let $\frac{1}{2} \sin ^{-1} \frac{3}{4}=\tan ^{-1} \theta$ $[\therefore \tan (\tan ^{-1} \theta)=\theta]$

$ \begin{aligned} & \Rightarrow \quad \sin ^{-1} \frac{3}{4}=2 \tan ^{-1} \theta \Rightarrow \sin ^{-1} \frac{3}{4}=\sin ^{-1}(\frac{2 \theta}{1+\theta^{2}}) \\ & {[\because 2 \tan ^{-1} x=\sin ^{-1} \frac{2 x}{1+x^{2}}]} \\ & \Rightarrow \quad \frac{2 \theta}{1+\theta^{2}}=\frac{3}{4} \Rightarrow 3+3 \theta^{2}=8 \theta \\ & \Rightarrow \quad 3 \theta^{2}-8 \theta+3=0 \\ & \Rightarrow \quad \theta=\frac{-(-8) \pm \sqrt{(-8)^{2}-4 \times 3 \times 3}}{2 \times 3} \\ & =\frac{8 \pm \sqrt{64-36}}{6}=\frac{8 \pm \sqrt{28}}{6}=\frac{8 \pm 2 \sqrt{7}}{6}=\frac{2(4 \pm \sqrt{7})}{6} \\ & \Rightarrow \quad \theta=\frac{4 \pm \sqrt{7}}{3} \\ & \therefore \quad \theta=\frac{4+\sqrt{7}}{3} \text{ or } \frac{4-\sqrt{7}}{3} \\ & \theta=\frac{4+\sqrt{7}}{3} \text{ is ignored. } \\ & \text{ Because } \frac{-\pi}{2} \leq \sin ^{-1} \frac{3}{4} \leq \frac{\pi}{2} \\ & \Rightarrow \frac{-\pi}{4} \leq \frac{1}{2} \sin ^{-1} \frac{3}{4} \leq \frac{\pi}{4} \\ & \Rightarrow \tan (\frac{-\pi}{4}) \leq \tan (\frac{1}{2} \sin ^{-1} \frac{3}{4}) \leq \tan (\frac{\pi}{4}) \\ & \Rightarrow-1 \leq \tan (\frac{1}{2} \sin ^{-1} \frac{3}{4}) \leq 1 \end{aligned} $

Solution

If $a_1, a_2, a_3, \ldots, a_n$ are the terms of an arithmetic progression $\therefore d=a_2-a_1=a_3-a_2=a_4-a_3 \ldots$

$ \begin{matrix} \therefore \tan [\tan ^{-1}(\frac{a_2-a_1}{1+a_1 a_2})+\tan ^{-1}(\frac{a_3-a_2}{1+a_2 a_3})+\tan ^{-1}(\frac{a_4-a_3}{1+a_3 a_4})+\ldots. \\ .\ldots+\tan ^{-1}(\frac{a_n-a _{n-1}}{1+a _{n-1} \cdot a_n})] \\ \Rightarrow \tan [(\tan ^{-1} a_2-\tan ^{-1} a_1)+(\tan ^{-1} a_3-\tan ^{-1} a_2)+(\tan ^{-1} a_4-\tan ^{-1} a_3). \\ .+\ldots+(\tan ^{-1} a_n-\tan ^{-1} a _{n-1})] \\ \Rightarrow \tan [\tan ^{-1} a_2-\tan ^{-1} a_1+\tan ^{-1} a_3-\tan ^{-1} a_2+\tan ^{-1} a_4-\tan ^{-1} a_3. \\ \quad[\because \tan ^{-1} \frac{x-y}{1+x y}=\tan ^{-1} x-\tan ^{-1} y] \\ \Rightarrow \tan [\tan ^{-1} a_n-\tan ^{-1} a_1] \quad \quad[\because \tan (\tan ^{-1} x)=x] \\ \Rightarrow \tan [\tan ^{-1}(\frac{a_n-a_1}{1+a_1 a_n})] \Rightarrow \frac{a_n-a_1}{1+a_1 a_n \quad} \end{matrix} $

Solution

Principal value branch of $\cos ^{-1} x$ is $[0, \pi]$. Hence the correct answer is (c).

• Option (a) $[\frac{-\pi}{2}, \frac{\pi}{2}]$ is incorrect because this interval is the principal value branch of $\sin^{-1} x$, not $\cos^{-1} x$.
• Option (b) $(0, \pi)$ is incorrect because the principal value branch of $\cos^{-1} x$ includes the endpoints 0 and $\pi$, making it a closed interval $[0, \pi]$ rather than an open interval.
• Option (d) $(0, \pi)-{\frac{\pi}{2}}$ is incorrect because the principal value branch of $\cos^{-1} x$ is a continuous interval $[0, \pi]$ and does not exclude $\frac{\pi}{2}$.

Solution

Principal value branch of $cosec^{-1} x$ is $[\frac{-\pi}{2}, \frac{\pi}{2}]-{0}$ as $cosec^{-1}(0)=\infty$ (not defined).

Hence, the correct answer is (d).

• Option (a) $(\frac{-\pi}{2}, \frac{\pi}{2})$ is incorrect because the interval does not include the endpoints $-\frac{\pi}{2}$ and $\frac{\pi}{2}$, which are necessary for the principal value branch of $cosec^{-1} x$.

• Option (b) $[0, \pi]-{\frac{\pi}{2}}$ is incorrect because the principal value branch of $cosec^{-1} x$ should include negative values, and this interval only covers the positive half of the range.

• Option (c) $[\frac{-\pi}{2}, \frac{\pi}{2}]$ is incorrect because it includes $0$, where $cosec^{-1}(0)$ is not defined.

Solution

Given that $3 \tan ^{-1} x+\cot ^{-1} x=\pi$

$\Rightarrow 2 \tan ^{-1} x+\tan ^{-1} x+\cot ^{-1} x=\pi$

$\Rightarrow 2 \tan ^{-1} x+\frac{\pi}{2}=\pi$

$[\because \tan ^{-1} x+\cot ^{-1} x=\frac{\pi}{2}]$

$\Rightarrow \quad 2 \tan ^{-1} x=\pi-\frac{\pi}{2} \quad \Rightarrow 2 \tan ^{-1} x=\frac{\pi}{2}$

$\Rightarrow \quad \tan ^{-1} x=\frac{\pi}{4} \quad \Rightarrow \tan ^{-1} x=\tan ^{-1}(1)$

$\therefore \quad x=1$

Hence, the correct answer is $(b)$.

• Option (a) 0: If ( x = 0 ), then ( \tan^{-1}(0) = 0 ). Substituting into the given equation, we get ( 3 \cdot 0 + \cot^{-1}(0) = \pi ). However, ( \cot^{-1}(0) = \frac{\pi}{2} ), so the equation becomes ( \frac{\pi}{2} \neq \pi ). Therefore, ( x = 0 ) does not satisfy the equation.

• Option (c) -1: If ( x = -1 ), then ( \tan^{-1}(-1) = -\frac{\pi}{4} ). Substituting into the given equation, we get ( 3 \cdot -\frac{\pi}{4} + \cot^{-1}(-1) = \pi ). However, ( \cot^{-1}(-1) = \frac{3\pi}{4} ), so the equation becomes ( -\frac{3\pi}{4} + \frac{3\pi}{4} = 0 \neq \pi ). Therefore, ( x = -1 ) does not satisfy the equation.

• Option (d) (\frac{1}{2}): If ( x = \frac{1}{2} ), then ( \tan^{-1}\left(\frac{1}{2}\right) ) is some angle (\theta) such that (\tan(\theta) = \frac{1}{2}). Substituting into the given equation, we get ( 3 \cdot \tan^{-1}\left(\frac{1}{2}\right) + \cot^{-1}\left(\frac{1}{2}\right) = \pi ). However, (\cot^{-1}\left(\frac{1}{2}\right) = \tan^{-1}(2)), so the equation becomes ( 3 \cdot \tan^{-1}\left(\frac{1}{2}\right) + \tan^{-1}(2) \neq \pi ). Therefore, ( x = \frac{1}{2} ) does not satisfy the equation.

Solution

$\sin ^{-1}[\cos (\frac{33 \pi}{5})]=\sin ^{-1}[\cos (6 \pi+\frac{3 \pi}{5})]$

$ \begin{aligned} & =\sin ^{-1}[\cos \frac{3 \pi}{5}] \quad[\because \cos (2 n \pi+x)=\cos x] \\ & =\sin ^{-1}[\cos (\frac{\pi}{2}+\frac{\pi}{10})] \\ & =\sin ^{-1}[-\sin (\frac{\pi}{10})][\because \cos (\frac{\pi}{2}+\theta)=-\sin \theta] \\ & =\sin ^{-1}[\sin (\frac{-\pi}{10})]=\frac{-\pi}{10} \end{aligned} $

Hence, the correct answer is $(d)$.

• Option (a) $\frac{3 \pi}{5}$ is incorrect because $\sin^{-1}[\cos(\frac{33 \pi}{5})]$ simplifies to $\sin^{-1}[\cos(\frac{3 \pi}{5})]$, which is not equal to $\frac{3 \pi}{5}$ since $\cos(\frac{3 \pi}{5})$ is not in the range of the inverse sine function.

• Option (b) $\frac{-7 \pi}{5}$ is incorrect because $\sin^{-1}[\cos(\frac{33 \pi}{5})]$ simplifies to $\sin^{-1}[\cos(\frac{3 \pi}{5})]$, and $\frac{-7 \pi}{5}$ is not a valid angle in the principal range of the inverse sine function, which is $[-\frac{\pi}{2}, \frac{\pi}{2}]$.

• Option (c) $\frac{\pi}{10}$ is incorrect because $\sin^{-1}[\cos(\frac{33 \pi}{5})]$ simplifies to $\sin^{-1}[\cos(\frac{\pi}{2} + \frac{\pi}{10})]$, which further simplifies to $\sin^{-1}[-\sin(\frac{\pi}{10})]$. The correct value is $\frac{-\pi}{10}$, not $\frac{\pi}{10}$.

Solution

The given function is $\cos ^{-1}(2 x-1)$

Let

$ \begin{aligned} f(x) & =\cos ^{-1}(2 x-1) \\ -1 & \leq 2 x-1 \leq 1 \Rightarrow-1+1 \leq 2 x \leq 1+1 \\ 0 & \leq 2 x \leq 2 \quad \Rightarrow 0 \leq x \leq 1 \end{aligned} $

$\therefore$ domain of the given function is $[0,1]$.

Hence, the correct answer is (a)

• Option (b) $[-1,1]$: This option is incorrect because the domain of the function $\cos^{-1}(2x-1)$ is determined by the range of the expression $2x-1$. For $2x-1$ to be within the range of the $\cos^{-1}$ function, it must lie between -1 and 1. Solving $-1 \leq 2x-1 \leq 1$ gives $0 \leq x \leq 1$, not $-1 \leq x \leq 1$.

• Option (c) $(-1,1)$: This option is incorrect because the domain of the function $\cos^{-1}(2x-1)$ includes the endpoints 0 and 1. The correct domain is $[0,1]$, not $(-1,1)$, which excludes the endpoints.

• Option (d) $[0, \pi]$: This option is incorrect because it represents the range of the $\cos^{-1}$ function, not the domain. The domain of the function $\cos^{-1}(2x-1)$ is the set of $x$ values for which $2x-1$ lies within the interval $[-1, 1]$, which is $[0,1]$.

Solution

Let

$ f(x)=\sin ^{-1} \sqrt{x-1} $

$\because \sqrt{x-1} \geq 0$ and $-1 \leq \sqrt{x-1} \leq 1$

$ \Rightarrow 0 \leq x-1 \leq 1 \Rightarrow 1 \leq x \leq 2 \Rightarrow x \in[1,2] $

Hence, the correct answer is (a).

• Option (b) $[-1,1]$: This is incorrect because the expression $\sqrt{x-1}$ represents a square root, which is always non-negative. Therefore, $x-1$ must be non-negative, implying $x \geq 1$. Additionally, for $\sqrt{x-1}$ to be within the range of the inverse sine function, $x-1$ must be less than or equal to 1, implying $x \leq 2$. Thus, $x$ must be in the interval $[1,2]$, not $[-1,1]$.

• Option (c) $[0,1]$: This is incorrect because for $f(x) = \sin^{-1} \sqrt{x-1}$ to be defined, $\sqrt{x-1}$ must be within the range $[0,1]$. This requires $x-1$ to be in the range $[0,1]$, which implies $x$ must be in the interval $[1,2]$. The interval $[0,1]$ does not satisfy this condition as it includes values less than 1, which would make $\sqrt{x-1}$ undefined or imaginary.

• Option (d) None of these: This is incorrect because the correct domain of the function $f(x) = \sin^{-1} \sqrt{x-1}$ is indeed $[1,2]$, which is provided in option (a). Therefore, option (d) is not applicable.

Solution

Given that $\cos [\sin ^{-1} \frac{2}{5}+\cos ^{-1} x]=0$

$ \begin{matrix} \Rightarrow \sin ^{-1} \frac{2}{5}+\cos ^{-1} x =\cos ^{-1}(0) \\ \Rightarrow \sin ^{-1} \frac{2}{5}+\cos ^{-1} x =\frac{\pi}{2} \Rightarrow \sin ^{-1} \frac{2}{5}=\frac{\pi}{2}-\cos ^{-1} x \\ \Rightarrow \sin ^{-1} \frac{2}{5} =\sin ^{-1} x[\because \sin ^{-1} x+\cos ^{-1} x=\frac{\pi}{2}] \\ \Rightarrow x =\frac{2}{5} \end{matrix} $

Hence, the correct answer is (b).

• Option (a) $\frac{1}{5}$ is incorrect because if $x = \frac{1}{5}$, then $\cos^{-1} x = \cos^{-1} \frac{1}{5}$. Substituting this into the equation $\cos [\sin^{-1} \frac{2}{5} + \cos^{-1} x] = 0$ would not satisfy the equation since $\sin^{-1} \frac{2}{5} + \cos^{-1} \frac{1}{5} \neq \frac{\pi}{2}$.

• Option (c) 0 is incorrect because if $x = 0$, then $\cos^{-1} x = \cos^{-1} 0 = \frac{\pi}{2}$. Substituting this into the equation $\cos [\sin^{-1} \frac{2}{5} + \cos^{-1} x] = 0$ would result in $\cos [\sin^{-1} \frac{2}{5} + \frac{\pi}{2}] = 0$, which is not true since $\sin^{-1} \frac{2}{5} + \frac{\pi}{2}$ is not an angle whose cosine is zero.

• Option (d) 1 is incorrect because if $x = 1$, then $\cos^{-1} x = \cos^{-1} 1 = 0$. Substituting this into the equation $\cos [\sin^{-1} \frac{2}{5} + \cos^{-1} x] = 0$ would result in $\cos [\sin^{-1} \frac{2}{5} + 0] = \cos [\sin^{-1} \frac{2}{5}]$, which is not zero since $\cos [\sin^{-1} \frac{2}{5}] = \sqrt{1 - (\frac{2}{5})^2} \neq 0$.

Solution

Given that $\sin [2 \tan ^{-1}(0.75)]$

$ \begin{aligned} & =\sin [2 \tan ^{-1} \frac{3}{4}] \\ & =\sin [\sin ^{-1} \frac{2 \times \frac{3}{4}}{1+\frac{9}{16}}][\because 2 \tan ^{-1} x=\sin ^{-1} \frac{2 x}{1+x^{2}}] \\ & =\sin [\sin ^{-1} \frac{\frac{3}{2}}{\frac{25}{16}}]=\sin [\sin ^{-1} \frac{24}{25}] \\ & =\sin [\sin ^{-1}(0.96)] \end{aligned} $

$ =0.96 $

Hence, the correct answer is (c).

• Option (a) 0.75 is incorrect because the value of $\sin [2 \tan^{-1}(0.75)]$ is not equal to 0.75. The calculation shows that it simplifies to 0.96.

• Option (b) 1.5 is incorrect because the value of $\sin [2 \tan^{-1}(0.75)]$ does not simplify to 1.5. The correct value is 0.96.

• Option (d) $\sin 1.5$ is incorrect because $\sin [2 \tan^{-1}(0.75)]$ simplifies to $\sin [\sin^{-1}(0.96)]$, which is 0.96, not $\sin 1.5$.

Solution

$ \cos ^{-1}(\cos \frac{\pi}{2}) \neq \frac{3 \pi}{2} \quad \because \frac{3 \pi}{2} \notin[0, \pi] $

$\Rightarrow \cos ^{-1}[\cos (\pi+\frac{\pi}{2})] \Rightarrow \cos ^{-1}[-\cos \frac{\pi}{2}] \Rightarrow \cos ^{-1}[0]=\frac{\pi}{2}$

Hence, the correct answer is (a).

• Option (b) $\frac{3 \pi}{2}$ is incorrect because $\frac{3 \pi}{2}$ is not within the principal range of the inverse cosine function, which is $[0, \pi]$.

• Option (c) $\frac{5 \pi}{2}$ is incorrect because $\frac{5 \pi}{2}$ is not within the principal range of the inverse cosine function, which is $[0, \pi]$.

• Option (d) $\frac{7 \pi}{2}$ is incorrect because $\frac{7 \pi}{2}$ is not within the principal range of the inverse cosine function, which is $[0, \pi]$.

Solution

$2 \sec ^{-1} 2+\sin ^{-1} \frac{1}{2}=2 \sec ^{-1}(\sec \frac{\pi}{3})+\sin ^{-1}(\sin \frac{\pi}{6})$

$ =2 \cdot \frac{\pi}{3}+\frac{\pi}{6}=\frac{2 \pi}{3}+\frac{\pi}{6}=\frac{5 \pi}{6} $

Hence, the correct answer is $(b)$.

• Option (a) $\frac{\pi}{6}$ is incorrect because the calculated value of the expression $2 \sec^{-1} 2 + \sin^{-1} \frac{1}{2}$ is $\frac{5\pi}{6}$, not $\frac{\pi}{6}$. The value $\frac{\pi}{6}$ is too small compared to the correct value.

• Option (c) $\frac{7\pi}{6}$ is incorrect because the calculated value of the expression $2 \sec^{-1} 2 + \sin^{-1} \frac{1}{2}$ is $\frac{5\pi}{6}$, not $\frac{7\pi}{6}$. The value $\frac{7\pi}{6}$ is too large compared to the correct value.

• Option (d) 1 is incorrect because the calculated value of the expression $2 \sec^{-1} 2 + \sin^{-1} \frac{1}{2}$ is $\frac{5\pi}{6}$, not 1. The value 1 is not in the same form as the correct value, which is in terms of $\pi$.

Solution

Given that $\tan ^{-1} x+\tan ^{-1} y=\frac{4 \pi}{5}$

$ \begin{matrix} \Rightarrow & \frac{\pi}{2}-\cot ^{-1} x+\frac{\pi}{2}-\cot ^{-1} y=\frac{4 \pi}{5} \quad[\because \tan ^{-1} x+\cot ^{-1} x=\frac{\pi}{2}] \\ \Rightarrow & \pi-(\cot ^{-1} x+\cot ^{-1} y)=\frac{4 \pi}{5} \\ \Rightarrow & \quad \cot ^{-1} x+\cot ^{-1} y=\pi-\frac{4 \pi}{5} \\ \Rightarrow & \quad \cot ^{-1} x+\cot ^{-1} y=\frac{\pi}{5} \end{matrix} $

Hence, the correct answer is (a).

• Option (b) $\frac{2 \pi}{5}$ is incorrect because the correct calculation shows that $\cot^{-1} x + \cot^{-1} y = \frac{\pi}{5}$, not $\frac{2 \pi}{5}$. The given equation $\tan^{-1} x + \tan^{-1} y = \frac{4 \pi}{5}$ leads to $\pi - \frac{4 \pi}{5}$, which simplifies to $\frac{\pi}{5}$.

• Option (c) $\frac{3 \pi}{5}$ is incorrect because the correct calculation shows that $\cot^{-1} x + \cot^{-1} y = \frac{\pi}{5}$, not $\frac{3 \pi}{5}$. The given equation $\tan^{-1} x + \tan^{-1} y = \frac{4 \pi}{5}$ leads to $\pi - \frac{4 \pi}{5}$, which simplifies to $\frac{\pi}{5}$.

• Option (d) $\pi$ is incorrect because the correct calculation shows that $\cot^{-1} x + \cot^{-1} y = \frac{\pi}{5}$, not $\pi$. The given equation $\tan^{-1} x + \tan^{-1} y = \frac{4 \pi}{5}$ leads to $\pi - \frac{4 \pi}{5}$, which simplifies to $\frac{\pi}{5}$.

Solution

$\sin ^{-1}(\frac{2 a}{1+a^{2}})+\cos ^{-1}(\frac{1-a^{2}}{1+a^{2}})=\tan ^{-1}(\frac{2 x}{1-x^{2}})$

$ \Rightarrow 2 \tan ^{-1} a+2 \tan ^{-1} a=2 \tan ^{-1} x $

$[\because 2 \tan ^{-1} x=\sin ^{-1} \frac{2 x}{1+x^{2}}=\cos ^{-1} \frac{1-x^{2}}{1+x^{2}}=\tan ^{-1} \frac{2 x}{1-x^{2}}]$

$\Rightarrow \quad 4 \tan ^{-1} a=2 \tan ^{-1} x \Rightarrow 2 \tan ^{-1} a=\tan ^{-1} x$

Hence, the correct answer is (d).

• Option (a) 0: This option is incorrect because if ( x = 0 ), then ( \tan^{-1}(0) = 0 ). However, the given equation ( \sin^{-1}(\frac{2a}{1+a^2}) + \cos^{-1}(\frac{1-a^2}{1+a^2}) ) does not simplify to zero for ( a \in (0, 1) ).

• Option (b) (\frac{a}{2}): This option is incorrect because if ( x = \frac{a}{2} ), then ( \tan^{-1}(\frac{2(\frac{a}{2})}{1-(\frac{a}{2})^2}) = \tan^{-1}(\frac{a}{1-\frac{a^2}{4}}) ). This does not match the simplified form ( 2 \tan^{-1} a ) derived from the given equation.

• Option (c) (a): This option is incorrect because if ( x = a ), then ( \tan^{-1}(\frac{2a}{1-a^2}) ) would need to equal ( 2 \tan^{-1} a ). However, ( \tan^{-1}(\frac{2a}{1-a^2}) ) is not equal to ( 2 \tan^{-1} a ) for ( a \in (0, 1) ).

Solution

We have, $\cot [\cos ^{-1}(\frac{7}{25})]$

Let $\quad \cos ^{-1} \frac{7}{25}=\theta$

$\therefore \quad \cos \theta=\frac{7}{25} \Rightarrow \cot \theta=\frac{7}{24}$

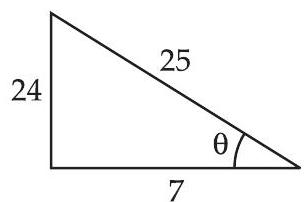
$\therefore \cot [\cos ^{-1}(\frac{7}{25})]=\cot [\cot ^{-1}(\frac{7}{24})]=\frac{}{24}$

Hence, the correct answer is $(d)$.

• Option (a) $\frac{25}{24}$: This option is incorrect because it represents the reciprocal of the sine of the angle, not the cotangent. The cotangent of the angle is $\frac{7}{24}$, not $\frac{25}{24}$.

• Option (b) $\frac{25}{7}$: This option is incorrect because it represents the reciprocal of the cosine of the angle, not the cotangent. The cotangent of the angle is $\frac{7}{24}$, not $\frac{25}{7}$.

• Option (c) $\frac{24}{25}$: This option is incorrect because it represents the tangent of the angle, not the cotangent. The cotangent of the angle is $\frac{7}{24}$, not $\frac{24}{25}$.

Solution

We have, $\tan [\frac{1}{2} \cos ^{-1} \frac{2}{\sqrt{5}}]$

$ \begin{aligned} & \text{ Let } \\ & \theta=\frac{1}{2} \cos ^{-1} \frac{2}{\sqrt{5}} \\ & \Rightarrow \quad 2 \theta=\cos ^{-1} \frac{2}{\sqrt{5}} \Rightarrow \cos 2 \theta=\frac{2}{\sqrt{5}} \\ & \Rightarrow \quad \frac{1-\tan ^{2} \theta}{1+\tan ^{2} \theta}=\frac{2}{\sqrt{5}} \quad[\because \cos 2 \theta=\frac{1-\tan ^{2} \theta}{1+\tan ^{2} \theta}] \\ & \Rightarrow \quad 2+2 \tan ^{2} \theta=\sqrt{5}-\sqrt{5} \tan ^{2} \theta \\ & \Rightarrow \sqrt{5} \tan ^{2} \theta+2 \tan ^{2} \theta=\sqrt{5}-2 \Rightarrow(\sqrt{5}+2) \tan ^{2} \theta=\sqrt{5}-2 \end{aligned} $

$ \begin{matrix} \Rightarrow & \tan ^{2} \theta=\frac{\sqrt{5}-2}{\sqrt{5}+2} \\ \Rightarrow & \tan ^{2} \theta=\frac{(\sqrt{5}-2)(\sqrt{5}-2)}{(\sqrt{5}+2)(\sqrt{5}-2)} \Rightarrow \tan ^{2} \theta=\frac{(\sqrt{5}-2)^{2}}{5-4} \\ \Rightarrow & \tan \theta= \pm(\sqrt{5}-2) \\ \Rightarrow \quad & \tan \theta=\sqrt{5}-2,[-(\sqrt{5}-2) \text{ is not required }] \end{matrix} $

Hence, the correct answer is $(b)$.

• Option (a) $2+\sqrt{5}$: This option is incorrect because the derived value of $\tan \theta$ is $\sqrt{5}-2$, not $2+\sqrt{5}$. The expression $\tan [\frac{1}{2} \cos ^{-1} \frac{2}{\sqrt{5}}]$ simplifies to $\sqrt{5}-2$, which is not equal to $2+\sqrt{5}$.

• Option (c) $\frac{\sqrt{5}+2}{2}$: This option is incorrect because the derived value of $\tan \theta$ is $\sqrt{5}-2$. The expression $\tan [\frac{1}{2} \cos ^{-1} \frac{2}{\sqrt{5}}]$ does not simplify to $\frac{\sqrt{5}+2}{2}$.

• Option (d) $5+\sqrt{2}$: This option is incorrect because the derived value of $\tan \theta$ is $\sqrt{5}-2$. The expression $\tan [\frac{1}{2} \cos ^{-1} \frac{2}{\sqrt{5}}]$ does not simplify to $5+\sqrt{2}$.

Solution

Here, we have $2 \tan ^{-1} x+\sin ^{-1}(\frac{2 x}{1+x^{2}})$

$ \begin{aligned} & =2 \tan ^{-1} x+2 \tan ^{-1} x \quad[\because 2 \tan ^{-1} x=\sin ^{-1} \frac{2 x}{1+x^{2}}] \\ & =4 \tan ^{-1} x \end{aligned} $

Hence, the correct answer is $(a)$.

• Option (b) 0: This option is incorrect because the expression (2 \tan^{-1} x + \sin^{-1} \left( \frac{2x}{1+x^2} \right)) simplifies to (4 \tan^{-1} x), not 0. The given trigonometric identities and simplifications do not lead to a result of 0.

• Option (c) (\frac{\pi}{2}): This option is incorrect because the expression (2 \tan^{-1} x + \sin^{-1} \left( \frac{2x}{1+x^2} \right)) simplifies to (4 \tan^{-1} x), not (\frac{\pi}{2}). The simplification process does not yield (\frac{\pi}{2}) for any value of (x) within the given range (|x| \leq 1).

• Option (d) (\pi): This option is incorrect because the expression (2 \tan^{-1} x + \sin^{-1} \left( \frac{2x}{1+x^2} \right)) simplifies to (4 \tan^{-1} x), not (\pi). The trigonometric identities and the range of (x) do not support a result of (\pi).

Solution

We have $\cos ^{-1} \alpha+\cos ^{-1} \beta+\cos ^{-1} \gamma=3 \pi$

$ \begin{aligned} & \Rightarrow \quad \cos ^{-1} \alpha+\cos ^{-1} \beta+\cos ^{-1} \gamma=\pi+\pi+\pi \\ & \Rightarrow \quad \cos ^{-1} \alpha=\pi, \cos ^{-1} \beta=\pi \text{ and } \cos ^{-1} \gamma=\pi \\ & \Rightarrow \quad \alpha=\cos \pi, \beta=\cos \pi \text{ and } \gamma=\cos \pi \\ & \therefore \quad \alpha=-1, \beta=-1 \text{ and } \gamma=-1 \\ & \text{ Which gives } \alpha=\beta=\gamma=-1 \\ & \text{ So } \quad \alpha(\beta+\gamma)+\beta(\gamma+\alpha)+\gamma(\alpha+\beta) \\ & \Rightarrow \quad(-1)(-1-1)+(-1)(-1-1)+(-1)(-1-1) \\ & \Rightarrow \quad(-1)(-2)+(-1)(-2)+(-1)(-2) \Rightarrow 2+2+2 \Rightarrow 6 \end{aligned} $

Hence, the correct answer is (c).

• Option (a) 0: This option is incorrect because the calculation of (\alpha(\beta+\gamma) + \beta(\gamma+\alpha) + \gamma(\alpha+\beta)) with (\alpha = \beta = \gamma = -1) results in 6, not 0. The expression simplifies to (2 + 2 + 2 = 6).

• Option (b) 1: This option is incorrect because the expression (\alpha(\beta+\gamma) + \beta(\gamma+\alpha) + \gamma(\alpha+\beta)) does not simplify to 1 when (\alpha = \beta = \gamma = -1). The correct simplification is (2 + 2 + 2 = 6).

• Option (d) 12: This option is incorrect because the expression (\alpha(\beta+\gamma) + \beta(\gamma+\alpha) + \gamma(\alpha+\beta)) simplifies to 6, not 12, when (\alpha = \beta = \gamma = -1). The correct calculation is (2 + 2 + 2 = 6).

Solution

We have $\sqrt{1+\cos 2 x}=\sqrt{2} \cos ^{-1}(\cos x)$

$ \begin{matrix} \Rightarrow & \sqrt{2 \cos ^{2} x}=\sqrt{2} x \\ \Rightarrow & \sqrt{2} \cos x & =\sqrt{2} x \Rightarrow \cos x=x \end{matrix} $

Which does not satisfy for any value of $x$.

Hence, the correct answer is $(d)$.

• Option (a) 0: This option is incorrect because the equation $\cos x = x$ does not have any real solutions in the interval $[\frac{\pi}{2}, \pi]$. Therefore, stating that there are zero solutions is incorrect.

• Option (b) 1: This option is incorrect because the equation $\cos x = x$ does not have exactly one real solution in the interval $[\frac{\pi}{2}, \pi]$. Hence, stating that there is one solution is incorrect.

• Option (c) 2: This option is incorrect because the equation $\cos x = x$ does not have exactly two real solutions in the interval $[\frac{\pi}{2}, \pi]$. Therefore, stating that there are two solutions is incorrect.

• Option (d) infinite: This option is incorrect because the equation $\cos x = x$ does not have any real solutions in the interval $[\frac{\pi}{2}, \pi]$. Therefore, stating that there are infinite solutions is incorrect.

Solution

Here, given that $\cos ^{-1} x>\sin ^{-1} x$

$ \begin{matrix} \Rightarrow & \sin [\cos ^{-1} x]>x \\ \Rightarrow & \sin [\sin ^{-1} \sqrt{1-x^{2}}]>x \Rightarrow \sqrt{1-x^{2}}>x \\ \Rightarrow & x<\sqrt{1-x^{2}} \Rightarrow x^{2}<1-x^{2} \Rightarrow 2 x^{2}<1 \\ \Rightarrow & x^{2}<\frac{1}{2} \Rightarrow x< \pm \frac{1}{\sqrt{2}} \end{matrix} $

We know that $-1 \leq x \leq 1$

So $-1 \leq x<\frac{1}{\sqrt{2}}$.

Hence, the correct answer is (c).

• Option (a) $\frac{1}{\sqrt{2}}<x \leq 1$: This option is incorrect because it suggests that ( x ) is greater than (\frac{1}{\sqrt{2}}). However, from the solution, we derived that ( x ) must be less than (\frac{1}{\sqrt{2}}). Therefore, this range does not satisfy the condition ( \cos^{-1} x > \sin^{-1} x ).

• Option (b) $0 \leq x<\frac{1}{\sqrt{2}}$: This option is incorrect because it only considers non-negative values of ( x ). The correct range derived from the solution is ( -1 \leq x < \frac{1}{\sqrt{2}} ), which includes negative values as well. Hence, this option is too restrictive.

• Option (d) $x>0$: This option is incorrect because it implies that ( x ) must be positive. However, the correct range derived from the solution includes negative values as well, specifically ( -1 \leq x < \frac{1}{\sqrt{2}} ). Therefore, this option does not cover the entire valid range of ( x ).

Solution

Let $\cos ^{-1}(-\frac{1}{2})=x \quad \Rightarrow \quad \cos x=-\frac{1}{2}$

$ \begin{aligned} & \Rightarrow & \cos x & =\cos (-\frac{\pi}{3}) \Rightarrow \cos x=\cos (\pi-\frac{\pi}{3})=\cos \frac{2 \pi}{3} \\ & \therefore & x & =\frac{2 \pi}{3} \in[0, \pi] \end{aligned} $

Hence, Principal value of $\cos ^{-1}(-\frac{1}{2})=\frac{2 \pi}{3}$.

Solution

$\quad \sin ^{-1}(\sin \frac{3 \pi}{5}) \neq \frac{3 \pi}{5}$ as $\frac{3 \pi}{5} \notin[\frac{-\pi}{2}, \frac{\pi}{2}]$

So $\sin ^{-1}(\sin \frac{3 \pi}{5})=\sin ^{-1} \sin (\pi-\frac{2 \pi}{5})$

$ =\sin ^{-1} \sin (\frac{2 \pi}{5})=\frac{2 \pi}{5} \in[\frac{-\pi}{2}, \frac{\pi}{2}] $

Hence, the value of $\sin ^{-1}(\sin \frac{3 \pi}{5})=\frac{2 \pi}{5}$

Solution

Given that

$ \begin{aligned} & \cos [\tan ^{-1} x+\cot ^{-1} \sqrt{3}]=0 \\ \Rightarrow & \tan ^{-1} x+\cot ^{-1} \sqrt{3}=\cos ^{-1}(0) \\ \Rightarrow & \tan ^{-1} x+\cot ^{-1} \sqrt{3}=\frac{\pi}{2} \\ \Rightarrow & \tan ^{-1} x=\frac{\pi}{2}-\cot ^{-1} \sqrt{3} \\ \Rightarrow & \tan ^{-1} x=\tan ^{-1} \sqrt{3} \Rightarrow x=\sqrt{3} \end{aligned} \quad[\because \tan ^{-1} x+\cot ^{-1} x=\frac{\pi}{2}] $

Hence, the value of $x$ is $\sqrt{3}$.

Solution

Let $\sec ^{-1}(\frac{1}{2})=x \Rightarrow \sec x=\frac{1}{2}$

Since, the domain of $\sec ^{-1} x$ is $R-{-1,1}$ and $\frac{1}{2} \notin R-{-1,1}$.

Hence, $\sec ^{-1}(\frac{1}{2})$ has no set of values.

Solution

$\tan ^{-1} \sqrt{3}=\tan ^{-1}(\tan \frac{\pi}{3})=\frac{\pi}{3} \in(\frac{-\pi}{2}, \frac{\pi}{2})$

Hence the principal value of $\tan ^{-1} \sqrt{3}$ is $\frac{\pi}{3}$.

Solution

$\quad \cos ^{-1}(\cos \frac{14 \pi}{3})=\cos ^{-1}[\cos (5 \pi-\frac{\pi}{3})]$

$ \begin{aligned} & =\cos ^{-1}[\cos (\frac{-\pi}{3})]=\cos ^{-1}[\cos (\pi-\frac{\pi}{3})] \\ & =\cos ^{-1}[\cos \frac{2 \pi}{3}]=\frac{2 \pi}{3} \in[0, \pi] \end{aligned} $

Hence, the value of $\cos ^{-1}[\cos \frac{14 \pi}{3}]=\frac{2 \pi}{3}$.

Solution

$\cos [\sin ^{-1} x+\cos ^{-1} x]=\cos \frac{\pi}{2}=0 \quad[\because \sin ^{-1} x+\cos ^{-1} x=\frac{\pi}{2}]$ Hence, the value of $\cos (\sin ^{-1} x+\cos ^{-1} x)=0$.

Solution

$\tan (\frac{\sin ^{-1} x+\cos ^{-1} x}{2})=\tan (\frac{\pi}{4})=1[\because \sin ^{-1} x+\cos ^{-1} x=\frac{\pi}{2}]$ Hence, the value of the given expression is 1 .

Solution

$ y=2 \tan ^{-1} x+\sin ^{-1}(\frac{2 x}{1+x^{2}}) $

$\begin{matrix} \Rightarrow & y=2 \tan ^{-1} x+2 \tan ^{-1} x \\ \Rightarrow & y=4 \tan ^{-1} x\end{matrix} [\because \sin ^{-1}(\frac{2 x}{1+x^{2}})=2 \tan ^{-1} x]$

Now $\frac{-\pi}{2}<\tan ^{-1} x<\frac{\pi}{2}$

$\Rightarrow \quad-4 \times \frac{\pi}{2}<4 \tan ^{-1} x<4 \times \frac{\pi}{2} \Rightarrow-2 \pi<y<2 \pi$

Hence, the value of $y$ is $(-2 \pi, 2 \pi)$.

Solution

The given result is true when $x y>-1$.

Solution

$\cot ^{-1}(-x)=\pi-\cot ^{-1} x, x \in R \quad[\because as^{-1}(-x)=\pi-\cot ^{-1} x]$

Solution

False.

We know that all inverse trigonometric functions are restricted over their domains.

Solution

False.

We know that $\cos ^{-1} x=\sec ^{-1}(\frac{1}{x}) \neq \sec x$ So

$ (\cos ^{-1} x)^{2} \neq \sec ^{2} x $

Solution

True.

We know that all trigonometric functions are restricted over their domains to obtain their inverse functions.

Solution

True.

Solution

True.

We know that the domain and range are interchanged in the graph of inverse trigonometric functions to that of their corresponding trigonometric functions.

Solution

False.

Given that $\tan ^{-1} \frac{n}{\pi}>\frac{\pi}{4}$

$ \begin{matrix} \Rightarrow & \frac{n}{\pi}>\tan \frac{\pi}{4} \Rightarrow \frac{n}{\pi}>1 \\ \Rightarrow & n>\pi \Rightarrow n>3.14 \end{matrix} $

Hence, the value of $n$ is 4 .

Solution

True.

$ \begin{aligned} \sin ^{-1}[\cos (\sin ^{-1} \frac{1}{2})] & =\sin ^{-1}[\cos (\sin ^{-1} \sin \frac{\pi}{6})] \\ \sin ^{-1}[\cos \frac{\pi}{6}] & =\sin ^{-1}(\frac{\sqrt{3}}{2})=\sin ^{-1}(\sin \frac{\pi}{3})=\frac{\pi}{3} \end{aligned} $