Applications of Integrals
Short Answer Type Questions
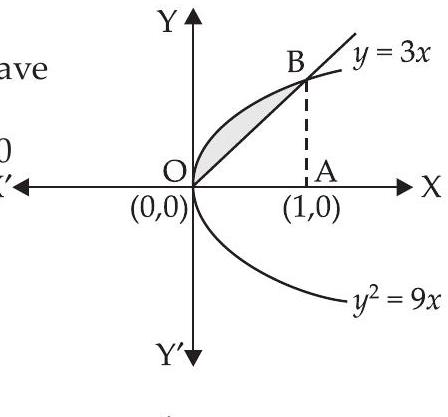
1. Find the area of the region bounded by the curves $y^{2}=9 x, y=3 x$
Show Answer
Solution
We have, $y^{2}=9 x, y=3 x$
Solving the two equations, we have
$(3 x)^{2}=9 x$
$\Rightarrow \quad 9 x^{2}-9 x=0 \Rightarrow 9 x(x-1)=0$
$\therefore \quad x=0,1$
Area of the shaded region
$=ar($ region $OAB)-ar(\triangle OAB)$
$=-\int_0^{1} y_l \cdot d x=\int_0^{1} \sqrt{9 x} d x-\int_0^{1} 3 x d x$
$=3 \int_0^{1} \sqrt{x} d x-3 \int_0^{1} x d x=3 \times \frac{2}{3}[x^{3 / 2}]_0^{1}-3[\frac{x^{2}}{2}]_0^{1}$
$=2[(1)^{3 / 2}-0]-\frac{3}{2}[(1)^{2}-0]=2(1)-\frac{3}{2}(1)=2-\frac{3}{2}=\frac{1}{2}$ sq. units
Hence, the required area $=\frac{1}{2}$ sq. units.
2. Find the area of the region bounded by the parabola $y^{2}=2 p x$ and $x^{2}=2 p y$.
Show Answer
Solution
We are given that: $x^{2}=2 p y \ldots(i)$
and $y^{2}=2 p x$
From eqn. (i) we get $y=\frac{x^{2}}{2 p}$
Putting the value of $y$ in eqn.
(ii) we have
$ \begin{aligned} & (\frac{x^{2}}{2 p})^{2}=2 p x \Rightarrow \frac{x^{4}}{4 p^{2}}=2 p x \\ & \Rightarrow x^{4}=8 p^{3} x \Rightarrow x^{4}-8 p^{3} x=0 \\ & \Rightarrow x(x^{3}-8 p^{3})=0 \quad \therefore x=0,2 p \end{aligned} $
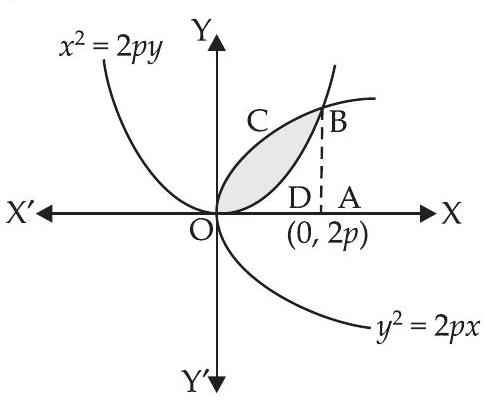
$ \begin{aligned} & \text{ Required area }=\text{ Area of the region }(\text{ OCBA - ODBA) } \\ & =\int_0^{2 p} \sqrt{2 p x} d x-\int_0^{2 p} \frac{x^{2}}{2 p} d x=\sqrt{2 p} \int_0^{2 p} \sqrt{x} d x-\frac{1}{2 p} \int_0^{2 p} x^{2} d x \\ & =\sqrt{2 p} \cdot \frac{2}{3}[x^{3 / 2}]_0^{2 p}-\frac{1}{2 p} \cdot \frac{1}{3}[x^{3}]_0^{2 p} \\ & =\frac{2 \sqrt{2}}{3} \sqrt{p}[(2 p)^{3 / 2}-0]-\frac{1}{6 p}[(2 p)^{3}-0] \\ & =\frac{2 \sqrt{2}}{3} \sqrt{p} \cdot 2 \sqrt{2} p^{\frac{3}{2}}-\frac{1}{6 p} \cdot 8 p^{3} \\ & =\frac{8}{3} \cdot p^{2}-\frac{8}{6} p^{2}=\frac{8}{6} p^{2}=\frac{4}{3} p^{2} \text{ sq. units } \end{aligned} $
Hence, the required area $=\frac{4}{3} p^{2}$ sq. units.
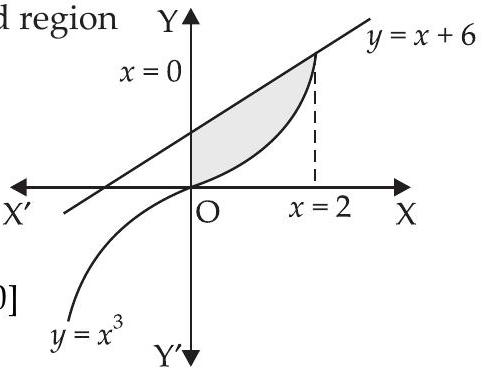
3. Find the area of the region bounded by the curve $y=x^{3}$, $y=x+6$ and $x=0$.
Show Answer
Solution
We are given that: $y=x^{3}, y=x+6$ and $x=0$
Solving $y=x^{3}$ and $y=x+6$, we get
$x+6 =x^{3}$
$\Rightarrow x^{3}-x-6 =0$
$\Rightarrow x^{2}(x-2)+2 x(x-2)+3(x-2) =0$
$\Rightarrow (x-2)(x^{2}+2 x+3) =0$
$x^{2}+2 x+3=0 \text{ has no real roots. } \quad \therefore x=2$
$\therefore$ Required area of the shaded region
$ \begin{aligned} & =\int_0^{2}(x+6) d x-\int_0^{2} x^{3} d x \\ & =[\frac{x^{2}}{2}+6 x]_0^{2}-\frac{1}{4}[x^{4}]_0^{2} \end{aligned} $
$ \begin{aligned} & =(\frac{4}{2}+12)-(0+0)-\frac{1}{4}[(2)^{4}-0] \\ & =14-\frac{1}{4} \times 16=14-4=10 \text{ sq. units. } \end{aligned} $
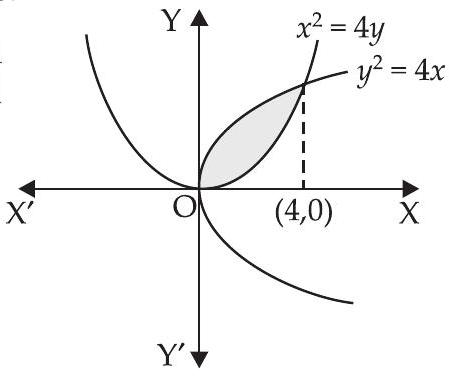
4. Find the area of the region bounded by the curve $y^{2}=4 x$ and $x^{2}=4 y$.
Show Answer
Solution
We have $y^{2}=4 x$ and $x^{2}=4 y$.
$ \begin{aligned} y & =\frac{x^{2}}{4} \\ \Rightarrow \quad(\frac{x^{2}}{4})^{2} & =4 x \end{aligned} $
$ \begin{aligned} & \Rightarrow \quad \frac{x^{4}}{16}=4 x \\ & \Rightarrow \quad x^{4}=64 x \Rightarrow x^{4}-64 x=0 \\ & \Rightarrow \quad x(x^{3}-64)=0 \\ & \therefore x=0, x=4 \end{aligned} $
Required area $=\int_0^{4} \sqrt{4 x} d x-\int_0^{4} \frac{x^{2}}{4} d x=2 \int_0^{4} \sqrt{x} d x-\frac{1}{4} \int_0^{4} x^{2} d x$
$ \begin{aligned} & =2 \cdot \frac{2}{3}[x^{3 / 2}]_0^{4}-\frac{1}{4} \cdot \frac{1}{3}[x^{3}]_0^{4} \\ & =\frac{4}{3}[(4)^{3 / 2}-0]-\frac{1}{12}[(4)^{3}-0]=\frac{4}{3}[8]-\frac{1}{12}[64] \\ & =\frac{32}{3}-\frac{16}{3}=\frac{16}{3} \text{ sq. units } \end{aligned} $
Hence, the required area $=\frac{16}{3}$ sq. units.
5. Find the area of the region included between $y^{2}=9 x$ and $y=x$.
Show Answer
Solution
Given that: $\quad y^{2}=9 x$
and $\quad y=x$
Solving eqns. (i) and (ii) we have
$$ \begin{align*} x^{2} & =9 x \Rightarrow x^{2}-9 x=0 \\ x(x-9) & =0 \quad \therefore x=0,9 \end{align*} $$
Required area
$ \begin{aligned} & =\int_0^{9} \sqrt{9 x} d x-\int_0^{9} x d x=3 \int_0^{9} \sqrt{x} d x-\int_0^{9} x d x \\ & =3 \cdot \frac{2}{3}[x^{3 / 2}]_0^{9}-\frac{1}{2}[x^{2}]_0^{9} \\ & =2[(9)^{3 / 2}-0]-\frac{1}{2}[(9)^{2}-0] \\ & =2(27)-\frac{1}{2}(81)=54-\frac{81}{2}=\frac{108-81}{2} \\ & =\frac{27}{2} \text{ sq. units } \end{aligned} $
Hence, the required area $=\frac{27}{2}$ sq. units.
6. Find the area of the region enclosed by the parabola $x^{2}=y$ and the line $y=x+2$.
Show Answer
Solution
Here, $x^{2}=y$ and $y=x+2$
$\therefore \quad x^{2}=x+2$
$\Rightarrow \quad x^{2}-x-2=0$
$\Rightarrow \quad x^{2}-2 x+x-2=0$
$\Rightarrow \quad x(x-2)+1(x-2)=0$
$\Rightarrow \quad(x-2)(x+1)=0$
$\therefore x=-1,2$
Graph of $y=x+2$
$\begin{array}{|c|c|c|} \hline x & 0 & -2 \\ \hline y & 2 & 0 \\ \hline \end{array}$
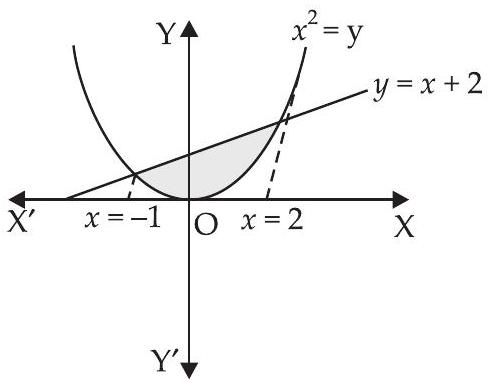
Area of the required region
$ \begin{aligned} & =\int _{-1}^{2}(x+2) d x-\int _{-1}^{2} x^{2} d x=[\frac{x^{2}}{2}+2 x] _{-1}^{2}-\frac{1}{3}[x^{3}] _{-1}^{2} \\ & =[(\frac{4}{2}+4)-(\frac{1}{2}-2)]-\frac{1}{3}[8-(-1)] \\ & =(6+\frac{3}{2})-\frac{1}{3}(9)=\frac{15}{2}-3=\frac{9}{2} \text{ sq. units } \end{aligned} $
Hence, the required area $=\frac{9}{2}$ sq. units.
7. Find the area of the region bounded by the line $x=2$ and parabola $y^{2}=8 x$.
Show Answer
Solution
Here,
$ \begin{aligned} & y^{2}=8 x \text{ and } x=2 \\ & y^{2}=8(2)=16 \end{aligned} $
$ \therefore \quad y= \pm 4 $
Required area
$=2 \int_0^{2} \sqrt{8 x} d x=2 \times 2 \sqrt{2} \int_0^{2} \sqrt{x} d x$
$=4 \sqrt{2} \times \frac{2}{3}[x^{3 / 2}]_0^{2}$
$=\frac{8 \sqrt{2}}{3}[(2)^{3 / 2}]=\frac{8 \sqrt{2}}{3} \times 2 \sqrt{2}=\frac{32}{3}$ sq. units
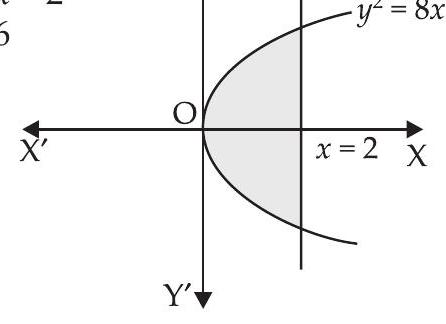
Hence, the area of the region $=\frac{32}{3}$ sq. units.
8. Sketch the region ${(x, 0): y=\sqrt{4-x^{2}}}$ and $x$-axis. Find the area of the region using integration.
Show Answer
Solution
Given that ${(x, 0): y=\sqrt{4-x^{2}}}$
$ \begin{matrix} \Rightarrow & y^{2}=4-x^{2} \\ \Rightarrow & x^{2}+y^{2}=4 \text{ which is a circle. } \end{matrix} $
Required area
$ =2 \cdot \int_0^{2} \sqrt{4-x^{2}} d x $
[Since circle is symmetrical about $y$-axis]
$ \begin{aligned} & =2 \cdot \int_0^{2} \sqrt{(2)^{2}-x^{2}} d x \\ & =2 \cdot[\frac{x}{2} \sqrt{4-x^{2}}+\frac{4}{2} \sin ^{-1} \frac{x}{2}]_0^{2} \\ & =2[(\frac{2}{2} \sqrt{4-4}+2 \sin ^{-1}(1))-(0+0)] \\ & =2[2 \cdot \frac{\pi}{2}]=2 \pi \text{ sq. units } \end{aligned} $
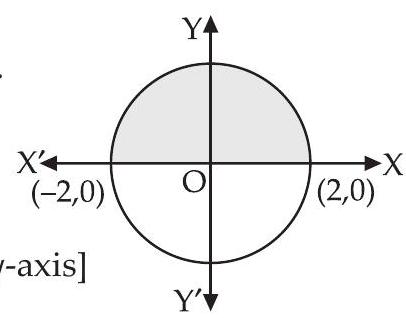
Hence, the required area $=2 \pi$ sq. units.
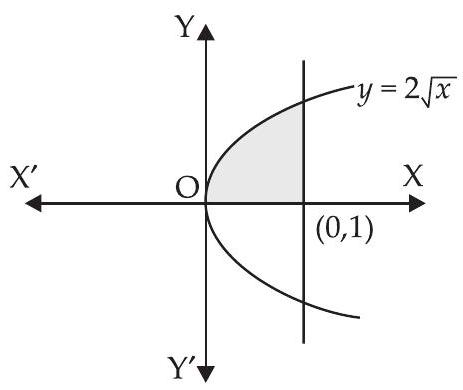
9. Calculate the area under the curve $y=2 \sqrt{x}$ included between the lines $x=0$ and $x=1$.
Show Answer
Solution
Given the curves $y=2 \sqrt{x}, x=0$ and $x=1$.
$ \begin{aligned} \text{ Required area } & =\int_0^{1}(2 \sqrt{x}) d x \\ & =2 \times \frac{2}{3}[x^{3 / 2}]_0^{1} \\ & =\frac{4}{3}[(1)^{3 / 2}-0] \\ & =\frac{4}{3} \text{ sq. units } \end{aligned} $
$ y=2 \sqrt{x} \Rightarrow y^{2}=4 x \text{ (Parabola) } $
Hence, required area $=\frac{4}{3}$ sq. units.
10. Using integration, find the area of the region bounded by the line $2 y=5 x+7, x$-axis and the lines $x=2$ and $x=8$.
Show Answer
Solution
Given that: $2 y=5 x+7, x$-axis, $x=2$ and $x=8$.
Let us draw the graph of $2 y=5 x+7 \Rightarrow y=\frac{5 x+7}{2}$
| $x$ | 1 | -1 |
|---|---|---|
| $y$ | 6 | 1 |
Area of the required shaded region
$=\int_2^{8}(\frac{5 x+7}{2}) d x=\frac{1}{2}[\frac{5}{2} x^{2}+7 x]_2^{8}$
$=\frac{1}{2}[\frac{5}{2}(64-4)+7(8-2)]$
$=\frac{1}{2}[\frac{5}{2} \times 60+7 \times 6]=\frac{1}{2}[150+42]$
$=\frac{1}{2} \times 192=96$ sq. units
Hence, the required area $=96$ sq. units.
11. Draw a rough sketch of the curve $y=\sqrt{x-1}$ in the interval $[1,5]$. Find the area under the curve and between the lines $x=1$ and $x=5$.
Show Answer
Solution
Here, we have $y=\sqrt{x-1}$
$\Rightarrow \quad y^{2}=x-1$ (Parabola)
Area of the required region
$=\int_1^{5} \sqrt{x-1} d x$
$=\frac{2}{3}[(x-1)^{3 / 2}]_1^{5}$
$=\frac{2}{3}[(5-1)^{3 / 2}-0]=\frac{2}{3} \times(4)^{3 / 2}$
$=\frac{2}{3} \times 8=\frac{16}{3}$ sq. units
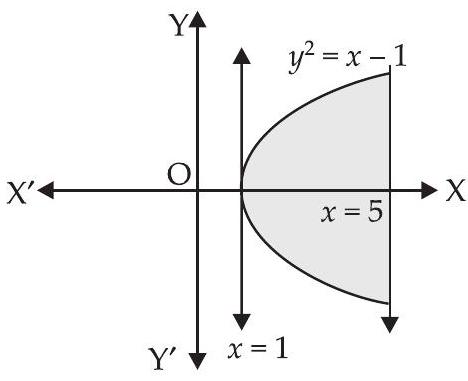
Hence, the required area $=\frac{16}{3}$ sq. units.
12. Determine the area under the curve $y=\sqrt{a^{2}-x^{2}}$ included between the lines $x=0$ and $x=a$.
Show Answer
Solution
Here, we are given $y=\sqrt{a^{2}-x^{2}}$
$ \begin{matrix} \Rightarrow & y^{2}=a^{2}-x^{2} \\ \Rightarrow & x^{2}+y^{2}=a^{2} \end{matrix} $
Area of the shaded region
$=2[(1)^{3 / 2}-0]-\frac{3}{2}[(1)^{2}-0]$
$=[\frac{x}{2} \sqrt{a^{2}-x^{2}}+\frac{a^{2}}{2} \sin ^{-1} \frac{x}{a}]_0^{a}$
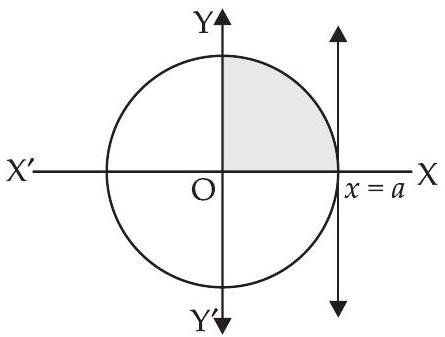
$ \begin{aligned} & =[\frac{a}{2} \sqrt{a^{2}-a^{2}}+\frac{a^{2}}{2} \sin ^{-1} \frac{a}{a}-0-0] \\ & =\frac{a^{2}}{2} \sin ^{-1}(1)=\frac{a^{2}}{2} \cdot \frac{\pi}{2}=\frac{\pi a^{2}}{4} \end{aligned} $
Hence, the required area $=\frac{\pi a^{2}}{4}$ sq. units.
13. Find the area of the region bounded by $y=\sqrt{x}$ and $y=x$.
Show Answer
Solution
We are given the equations of curve $y=\sqrt{x}$ and line $y=x$.
Solving $y=\sqrt{x} \Rightarrow y^{2}=x$ and $y=x$, we get
$ \begin{aligned} x^{2} & =x \Rightarrow x^{2}-x=0 \\ \Rightarrow \quad x(x-1) & =0 \quad \therefore x=0,1 \end{aligned} $
Required area of the shaded region
$ \begin{aligned} & =\int_0^{1} \sqrt{x} d x-\int_0^{1} x d x \\ & =\frac{2}{3}[x^{3 / 2}]_0^{1}-\frac{1}{2}[x^{2}]_0^{1} \\ & =\frac{2}{3}[(1)^{3 / 2}-0]-\frac{1}{2}[(1)^{2}-0] \\ & =\frac{2}{3}-\frac{1}{2} \Rightarrow \frac{4-3}{6} \Rightarrow \frac{1}{6} \text{ sq. units } \end{aligned} $
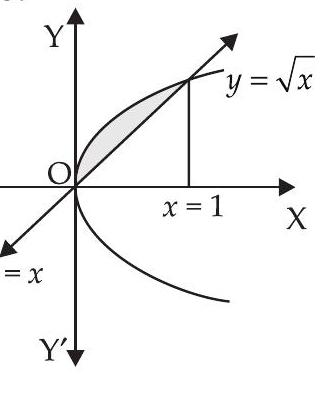
Hence, the required area $=\frac{1}{6}$ sq. units.
14. Find the area enclosed by the curve $y=-x^{2}$ and the straight line $x+y+2=0$.
Show Answer
Solution
We are given that $y=-x^{2}$ or $x^{2}=-y$ and the line $x+y+2=0$
Solving the two equations, we get
$x-x^{2}+2 =0$
$\Rightarrow x^{2}-x-2 =0$
$\Rightarrow x^{2}-2 x+x-2 =0$
$\Rightarrow x(x-2)+1(x-2) =0$
$\Rightarrow (x-2)(x+1) =0$
$\therefore x =-1,2$
Area of the required shaded region
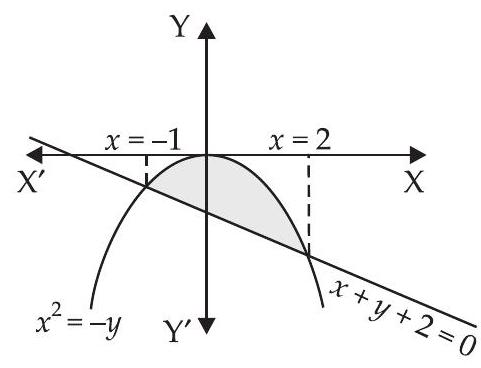
$=|\int _{-1}^{2}(-x-2) d x-\int _{-1}^{2}-x^{2} d x|$
$\Rightarrow \quad |-[\frac{x^{2}}{2}+2 x] _{-1}^{2} +\frac{1}{3}[x^{3}] _{-1}^{2}|$
$\Rightarrow \quad |-[(\frac{4}{2}+4)-(\frac{1}{2}-2)]+\frac{1}{3}(8+1)|$
$\Rightarrow \quad |-(6+\frac{3}{2})+\frac{1}{3}(9)|\Rightarrow|-\frac{15}{2}+3|$
$\Rightarrow \quad |\frac{-15+6}{2}|=|\frac{-9}{2}|=\frac{9}{2} \text{ sq. units }$
15. Find the area bounded by the curve $y=\sqrt{x}, x=2 y+3$ in the first quadrant and $x$-axis.
Show Answer
Solution
Given that: $y=\sqrt{x}, x=2 y+3$, first quadrant and $x$-axis.
Solving $y=\sqrt{x}$ and $x=2 y+3$, we get
$ \begin{aligned} & y=\sqrt{2 y+3} \Rightarrow y^{2}=2 y+3 \\ & \Rightarrow \quad y^{2}-2 y-3=0 \Rightarrow y^{2}-3 y+y-3=0 \\ & \Rightarrow \quad y(y-3)+1(y-3)=0 \\ & \Rightarrow \quad(y+1)(y-3)=0 \\ & \therefore \quad y=-1,3 \end{aligned} $
Hence, the required area $=9$ sq. units.
Long Answer Type Questions
16. Find the area of the region bounded by the curve $y^{2}=2 x$ and $x^{2}+y^{2}=4 x$.
Show Answer
Solution
Equations of the curves are given by
$$ \begin{equation*} x^{2}+y^{2}=4 x \tag{i} \end{equation*} $$
$$ \begin{equation*} \text{ and } \quad y^{2}=2 x \tag{ii} \end{equation*} $$
$ \begin{matrix} \Rightarrow & x^{2}-4 x+y^{2}=0 \\ \Rightarrow & x^{2}-4 x+4-4+y^{2}=0 \\ \Rightarrow & (x-2)^{2}+y^{2}=4 \end{matrix} $
Clearly it is the equation of a circle having its centre $(2,0)$ and radius 2 .
Solving $x^{2}+y^{2}=4 x$ and $y^{2}=2 x$
$ x^{2}+2 x=4 x $
$\Rightarrow x^{2}+2 x-4 x=0$
$\Rightarrow \quad x^{2}-2 x=0$
$\Rightarrow \quad x(x-2)=0$
$\therefore \quad x=0,2$
Area of the required region
$=2[\int_0^{2} \sqrt{4-(x-2)^{2}} d x-\int_0^{2} \sqrt{2 x} d x]$
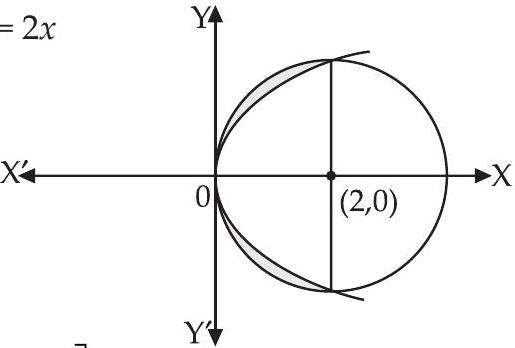
[ $\therefore$ Parabola and circle both are symmetrical about $x$-axis.]
$=2[\frac{x-2}{2} \sqrt{4-(x-2)^{2}}+\frac{4}{2} \sin ^{-1} \frac{x-2}{2}]_0^{2}-2 \cdot \sqrt{2} \cdot \frac{2}{3}[x^{3 / 2}]_0^{2}$
$=2[(0+0)-(0+2 \sin ^{-1}(-1)]-\frac{4 \sqrt{2}}{3}[2^{3 / 2}-0].$
$=-2 \times 2 \cdot(-\frac{\pi}{2})-\frac{4 \sqrt{2}}{3} \cdot 2 \sqrt{2}$
$=2 \pi-\frac{16}{3}=2(\pi-\frac{8}{3})$ sq.units
Hence, the required area $=2(\pi-\frac{8}{3})$ sq. units.
17. Find the area bounded by the curve $y=\sin x$ between $x=0$ and $x=2 \pi$.
Show Answer
Solution
Required area $=\int_0^{\pi} \sin x d x+\int _{\pi}^{2 \pi}|\sin x| d x$
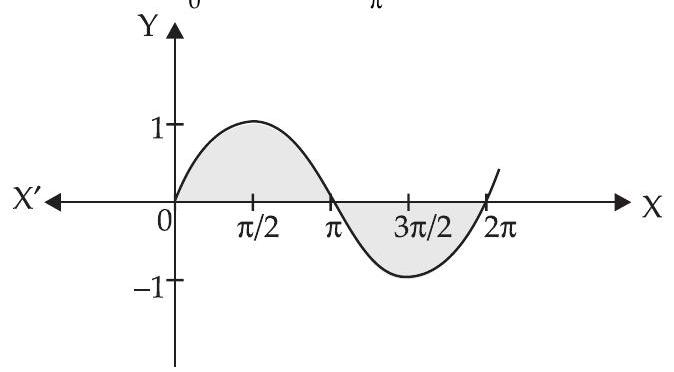
$ \begin{aligned} & =-[\cos x]_0^{\pi}+|(-\cos x)| _{\pi}^{2 \pi}=-[\cos \pi-\cos 0]+[\cos 2 \pi-\cos \pi] \\ & =-[-1-1]+[1+1]=2+2=4 \text{ sq. units } \end{aligned} $
18. Find the area of the region bounded by the triangle whose vertices are $(-1,1),(0,5)$ and $(3,2)$, using integration.
Show Answer
Solution
The coordinates of the vertices of $\triangle ABC$ are given by $A(-1,1)$, B $(0,5)$ and $C(3,2)$.
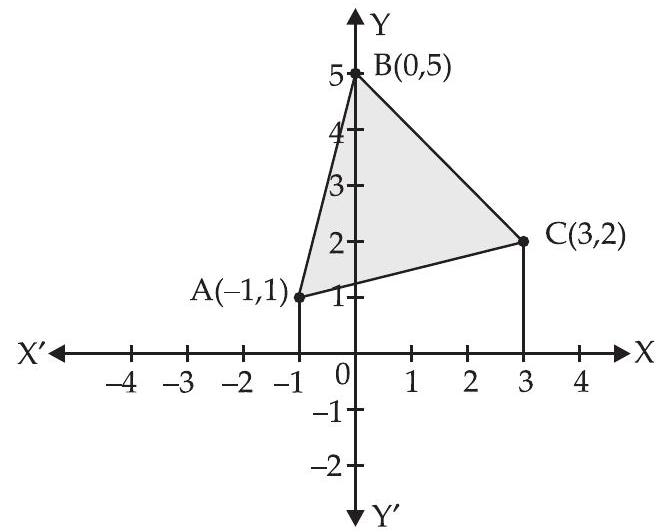
Equation of $AB$ is $\quad y-1=\frac{5-1}{0+1}(x+1)$
$$\Rightarrow y-1 =4 x+4$$
$$\therefore y =4 x+4+1 \Rightarrow y=4 x+5 \tag{i}$$
Equation of $BC$ is $\quad y-5=\frac{2-5}{3-0}(x-0)$
$ \Rightarrow \quad y-5=-x $
$$ \begin{equation*} \therefore \quad y=5-x \tag{ii} \end{equation*} $$
Equation of $CA$ is
$ \begin{aligned} y-1 & =\frac{2-1}{3+1}(x+1) \\ \Rightarrow \quad y-1 & =\frac{1}{4} x+\frac{1}{4} \Rightarrow y=\frac{1}{4} x+\frac{1}{4}+1 \\ \therefore \quad y & =\frac{1}{4} x+\frac{5}{4}=\frac{1}{4}(5+x) \end{aligned} $
Area of $\triangle ABC$
$ \begin{aligned} & =\int _{-1}^{0}(4 x+5) d x+\int_0^{3}(5-x) d x-\int _{-1}^{3} \frac{1}{4}(5+x) d x \\ & =\frac{4}{2}[x^{2}] _{-1}^{0}+5[x] _{-1}^{0}+5[x]_0^{3}-\frac{1}{2}[x^{2}]_0^{3}-\frac{1}{4}[5 x+\frac{x^{2}}{2}] _{-1}^{3} \end{aligned} $
$ \begin{aligned} & =2(0-1)+5(0+1)+5(3-0)-\frac{1}{2}(9-0) \\ & -\frac{1}{4}[(15+\frac{9}{2})-(-5+\frac{1}{2})] \\ & =-2+5+15-\frac{9}{2}-\frac{1}{4}(\frac{39}{2}+\frac{9}{2}) \\ & =18-\frac{9}{2}-\frac{1}{4} \times \frac{48}{2}=18-\frac{9}{2}-6=12-\frac{9}{2}=\frac{15}{2} \text{ sq. units } \end{aligned} $
Hence, the required area $=\frac{15}{2}$ sq. units.
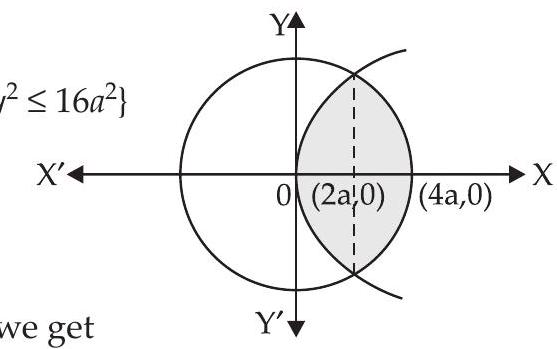
19. Draw a rough sketch of the region $\{(x, y): y^{2} \leq 6 a x\text{ and } x^{2}+y^{2} \leq 16 a^{2}\}$. Also find the area of the region sketched using method of integration.
Show Answer
Solution
Given that:
$\{(x, y): y^{2} \leq 6 a x.$ and $.x^{2}+y^{2} \leq 16 a^{2}\}$
Equation of Parabola is
$$ \begin{equation*} y^{2}=6 a x \tag{i} \end{equation*} $$
and equation of circle is
$$ \begin{equation*} x^{2}+y^{2} \leq 16 a^{2} \tag{ii} \end{equation*} $$
Solving eqns. (i) and (ii) we get
$ \begin{matrix} \Rightarrow & x^{2}+6 a x-16 a^{2}=0 \\ \Rightarrow & x^{2}+8 a x-2 a x-16 a^{2}=0 \\ \Rightarrow & x(x+8 a)-2 a(x+8 a)=0 \\ \Rightarrow & (x+8 a)(x-2 a)=0 \end{matrix} $
$\therefore x=2 a$ and $x=-8 a$. (Rejected as it is out of region)
Area of the required shaded region
$ \begin{aligned} & =2[\int_0^{2 a} \sqrt{6 a x} d x+\int _{2 a}^{4 a} \sqrt{16 a^{2}-x^{2}} d x] \\ & =2[\sqrt{6 a} \int_0^{2 a} \sqrt{x} d x+\int _{2 a}^{4 a} \sqrt{(4 a)^{2}-x^{2}} d x] \\ & =2 \sqrt{6 a} \cdot \frac{2}{3} \cdot[x^{3 / 2}]_0^{2 a}+2[\frac{x}{2} \sqrt{(4 a)^{2}-x^{2}}+\frac{16 a^{2}}{2} \sin ^{-1} \frac{x}{4 a}] _{2 a}^{4 a} \\ & =\frac{4 \sqrt{6}}{3} \cdot \sqrt{a}[(2 a)^{3 / 2}-0]+[x \sqrt{(4 a)^{2}-x^{2}}+16 a^{2} \sin ^{-1} \frac{x}{4 a}] _{2 a}^{4 a} \end{aligned} $
$ \begin{aligned} & =\frac{4 \sqrt{6}}{3} \sqrt{a} \cdot 2 \sqrt{2} \cdot a^{3 / 2}+[0+16 a^{2} \sin ^{-1}(\frac{4 a}{4 a})-2 a \sqrt{16 a^{2}-4 a^{2}} \\ & .-16 a^{2} \sin ^{-1} \frac{2 a}{4 a}] \\ & =\frac{8 \sqrt{12}}{3} a^{2}+[16 a^{2} \cdot \sin ^{-1}(1)-2 a \sqrt{12 a^{2}}-16 a^{2} \sin ^{-1} \frac{1}{2}] \\ & =\frac{16 \sqrt{3}}{3} a^{2}+[16 a^{2} \cdot \frac{\pi}{2}-2 a \cdot 2 \sqrt{3} a-16 a^{2} \cdot \frac{\pi}{6}] \\ & =\frac{16 \sqrt{3}}{3} a^{2}+8 \pi a^{2}-4 \sqrt{3} a^{2}-\frac{8}{3} \pi a^{2} \\ & =(\frac{16 \sqrt{3}}{3}-4 \sqrt{3}) a^{2}+\frac{16}{3} \pi a^{2}=\frac{4 \sqrt{3}}{3} a^{2}+\frac{16}{3} \pi a^{2} \\ & =\frac{4}{3}(\sqrt{3}+4 \pi) a^{2} \end{aligned} $
Hence, required area $=\frac{4}{3}(\sqrt{3}+4 \pi) a^{2}$ sq. units.
20. Compute the area bounded by the lines $x+2 y=2, y-x=1$ and $2 x+y=7$.
Show Answer
Solution
Given that: $$x+2 y=2 \tag{i}$$
$$ y-x=1 \tag{ii} $$
$$\text{ and } \qquad 2 x+y=7 \tag{iii}$$
$\begin{array}{|c|c|c|} \hline x & 0 & 2 \\ \hline y & 1 & 0 \\ \hline \end{array}$
$\begin{array}{|c|c|c|} \hline x & 0 & -1 \\ \hline y & 1 & 0 \\ \hline \end{array}$
$\begin{array}{|c|c|c|} \hline x & 0 & 7 / 2 \\ \hline y & 7 & 0 \\ \hline \end{array}$
Solving eqns. (ii) and (iii) we get
$y =1+x$
$\therefore 2 x+1+x =7$
$3 x =6$
$\Rightarrow x =2$
$\therefore y =1+2$
$=3$
Coordinates of $B=(2,3)$
Solving eqns. (i) and (iii) we get
$x +2 y =2$
$\therefore x =2-2 y$
$2 x+y =7$
$ 2(2-2 y)+y =7$
$\Rightarrow 4-4 y+y =7 \Rightarrow-3 y=3$
$\therefore y =-1 \text{ and } x=4$
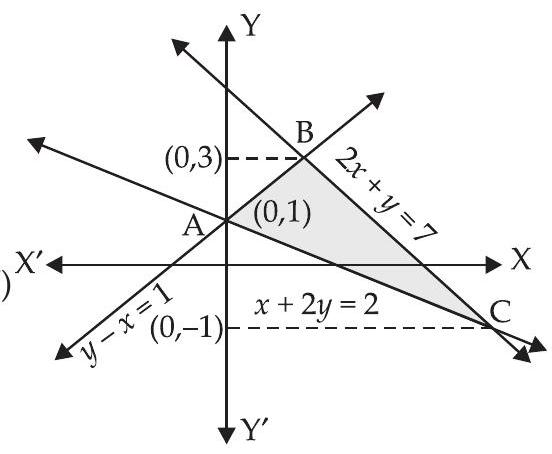
$\therefore$ Coordinates of $C=(4,-1)$ and coordinates of $A=(0,1)$.
Taking the limits on y-axis, we get
$ \begin{matrix} \int _{-1}^{3} x _{BC} d y-\int _{-1}^{1} x _{AC} d y-\int_1^{3} x _{AB} d y \\ =\int _{-1}^{3} \frac{7-y}{2} d y-\int _{-1}^{1}(2-2 y) d y-\int_1^{3}(y-1) d y \\ =\frac{1}{2}[7 y-\frac{y^{2}}{2}] _{-1}^{3}-2[y-\frac{y^{2}}{2}] _{-1}^{1}-[\frac{y^{2}}{2}-y]_1^{3} \\ =\frac{1}{2}[(21-\frac{9}{2})-(-7-\frac{1}{2})]-2[(1-\frac{1}{2})-(-1-\frac{1}{2})] \\ \quad-[(\frac{9}{2}-3)-(\frac{1}{2}-1)] \\ =\frac{1}{2} \times 24-2 \times 2-2 \Rightarrow 12-4-2=6 \text{ sq.units } \end{matrix} $
Hence, the required area $=6$ sq. units.
21. Find the area bounded by the lines $y=4 x+5, y=5-x$ and $4 y=x+5$.
Show Answer
Solution
Given that
and
$$ \begin{align*} y & =4 x+5 \tag{i}\\ y & =5-x \tag{ii}\\ 4 y & =x+5 \tag{iii} \end{align*} $$
$\begin{array}{|c|c|c|} \hline x & 0 & -5 / 4 \\ \hline y & 5 & 0 \\ \hline \end{array}$
$\begin{array}{|c|c|c|} \hline x & 0 & 5 \\ \hline y & 5 & 0 \\ \hline \end{array}$
$\begin{array}{|c|c|c|} \hline x & 0 & -5 \\ \hline y & 5 /4 & 0 \\ \hline \end{array}$
Solving eq. (i) and (ii) we get
$ \begin{matrix} 4 x+5 & =5-x \\ \Rightarrow & x & =0 \text{ and } y=5 \end{matrix} $
$\therefore$ Coordinates of $A=(0,5)$
Solving eq. (ii) and (iii)
$ \begin{aligned} y & =5-x \\ 4 y & =x+5 \\ 5 y & =10 \end{aligned} $
$\therefore y=2$ and $x=3$
$\therefore$ Coordinates of $B=(3,2)$
Solving eq. (i) and (iii)

$ y=4 x+5 $
$ \begin{aligned} & 4 y=x+5 \\ & \Rightarrow \quad 4(4 x+5)=x+5 \\ & \Rightarrow \quad 16 x+20=x+5 \Rightarrow 15 x=-15 \\ & \therefore \quad x=-1 \text{ and } y=1 \end{aligned} $
$\therefore$ Coordinates of $C=(-1,1)$.
$\therefore$ Area of required regions
$=\int _{-1}^{0} y _{AC} d x+\int_0^{3} y _{AB} d x-\int _{-1}^{3} y _{CB} d x$
$=\int _{-1}^{0}(4 x+5) d x+\int_0^{3}(5-x) d x-\int _{-1}^{3} \frac{x+5}{4} d x$
$=[4 \frac{x^{2}}{2}+5 x] _{-1}^{0}+[5 x-\frac{x^{2}}{2}]_0^{3}-\frac{1}{4}[\frac{x^{2}}{2}+5 x] _{-1}^{3}$
$=[(0+0)-(2-5)]+[(15-\frac{9}{2})-(0-0)]-\frac{1}{4}[(\frac{9}{2}+15)-(\frac{1}{2}-5)]$
$=3+\frac{21}{2}-\frac{1}{4}[\frac{39}{2}+\frac{9}{2}]=3+\frac{21}{2}-\frac{1}{4} \times 24 \Rightarrow 3+\frac{21}{2}-6$
$=\frac{15}{2}$ sq. units
Hence, the required area $=\frac{15}{2}$ sq. units.
22. Find the area bounded by the curve $y=2 \cos x$ and the $x$-axis from $x=0$ to $x=2 \pi$.
Show Answer
Solution
Given equation of the curve is $y=2 \cos x$
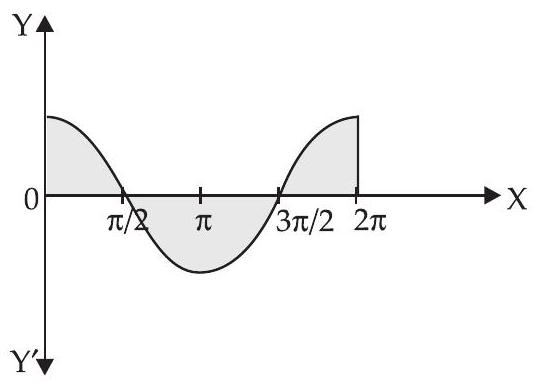
$\therefore$ Area of the shaded region
$ \int_0^{2 \pi} 2 \cos x d x=\int_0^{\pi / 2} 2 \cos x d x+\int _{\pi / 2}^{3 \pi / 2}|2 \cos x| d x+\int _{3 \pi / 2}^{2 \pi} 2 \cos x d x $
$ \begin{aligned} & =2[\sin x]_0^{\pi / 2}+|[2 \sin x] _{\pi / 2}^{3 \pi / 2}|+2[\sin x] _{3 \pi / 2}^{2 \pi} \\ & =2[\sin \frac{\pi}{2}-\sin 0]+|2(\sin \frac{3 \pi}{2}-\sin \frac{\pi}{2})| \\ & +2[\sin 2 \pi-\sin \frac{3 \pi}{2}] \\ & =2(1)+|2(-1-1)|+2(0+1)=2+4+2=8 \text{ sq. units } \end{aligned} $
23. Draw a rough sketch of the given curve $y=1+|x+1|, x=-3$, $x=3, y=0$ and find the area of the region bounded by them, using integration.
Show Answer
Solution
Given equations are
$y=1+|x+1|, x=-3$
and $x=3, y=0$
Taking $y=1+|x+1|$
$\Rightarrow \quad y=1+x+1$
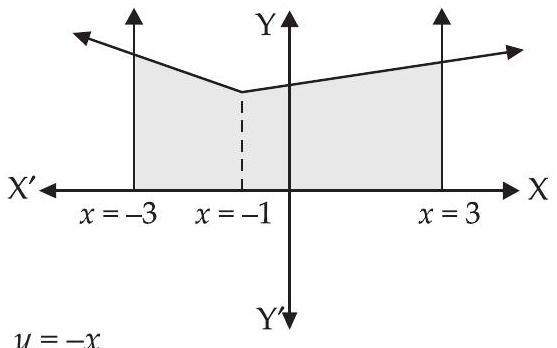
$\Rightarrow \quad y=x+2$
and $y=1-x-1 \Rightarrow y=-x$
On solving we get $x=-1$
Area of the required regions
$ \begin{aligned} & =\int _{-3}^{-1}-x d x+\int _{-1}^{3}(x+2) d x \\ & =-[\frac{x^{2}}{2}] _{-3}^{-1}+[\frac{x^{2}}{2}+2 x] _{-1}^{3}=-[\frac{1}{2}-\frac{9}{2}]+[(\frac{9}{2}+6)-(\frac{1}{2}-2)] \\ & =-(-4)+[\frac{21}{2}+\frac{3}{2}]=4+12=16 \text{ sq. units } \end{aligned} $
Hence, the required area $=16$ sq. units.
Objective Type Questions
24. The area of the region bounded by the $y$-axis, $y=\cos x$ and $y=\sin x$, where $0 \leq x \leq \frac{\pi}{2}$ is
(a) $\sqrt{2}$ sq. units
(b) $(\sqrt{2}+1)$ sq. units
(c) $(\sqrt{2}-1)$ sq. units
(d) $(2 \sqrt{2}-1)$ sq. units
Show Answer
Solution
Given that $y$-axis, $y=\cos x, y=\sin x, 0 \leq x \leq \frac{\pi}{2}$

$ \begin{aligned} \text{ Required area } & =\int_0^{\pi / 4} \cos x d x-\int_0^{\pi / 4} \sin x d x \\ & =[\sin x]_0^{\pi / 4}-[-\cos x]_0^{\pi / 4} \\ & =[\sin \frac{\pi}{4}-\sin 0]+[\cos \frac{\pi}{4}-\cos 0] \\ & =[\frac{1}{\sqrt{2}}-0+\frac{1}{\sqrt{2}}-1]=\frac{2}{\sqrt{2}}-1 \\ & =(\sqrt{2}-1) \text{ sq. units } \end{aligned} $
Hence, the correct option is (c).
-
Option (a) $\sqrt{2}$ sq. units: This option is incorrect because the calculated area is $\sqrt{2} - 1$ sq. units, not $\sqrt{2}$ sq. units. The subtraction of 1 is essential in the final result.
-
Option (b) $(\sqrt{2}+1)$ sq. units: This option is incorrect because the calculated area is $\sqrt{2} - 1$ sq. units, not $\sqrt{2} + 1$ sq. units. The addition of 1 is not part of the correct area calculation.
-
Option (d) $(2 \sqrt{2}-1)$ sq. units: This option is incorrect because the calculated area is $\sqrt{2} - 1$ sq. units, not $2 \sqrt{2} - 1$ sq. units. The factor of 2 in front of $\sqrt{2}$ is not part of the correct area calculation.
25. The area of the region bounded by the curve $x^{2}=4 y$ and the straight line $x=4 y-2$ is
(a) $\frac{3}{8}$ sq. units
(b) $\frac{5}{8}$ sq. units
(c) $\frac{7}{8}$ sq. units
(d) $\frac{9}{8}$ sq. units
Show Answer
Solution
Given that: The equation of parabola is $x^{2}=4 y$ and equation of straight line is $x=4 y-2$

Solving eqn. (i) and (ii) we get
$ y=\frac{x^{2}}{4} $
$ \begin{aligned} & x=4(\frac{x^{2}}{4})-2 \\ & \Rightarrow \quad x=x^{2}-2 \\ & \Rightarrow \quad x^{2}-x-2=0 \Rightarrow x^{2}-2 x+x-2=0 \\ & \Rightarrow x(x-2)+1(x-2)=0 \Rightarrow(x-2)(x+1)=0 \therefore x=-1, x=2 \\ & \text{ Required area }=\int _{-1}^{2} \frac{x+2}{4} d x-\int _{-1}^{2} \frac{x^{2}}{4} d x \\ & =\frac{1}{4}[\frac{x^{2}}{2}+2 x] _{-1}^{2}-\frac{1}{4} \cdot \frac{1}{3}[x^{3}] _{-1}^{2} \\ & =\frac{1}{4}[(\frac{4}{2}+4)-(\frac{1}{2}-2)]-\frac{1}{12}[8+1] \\ & =\frac{1}{4}[6+\frac{3}{2}]-\frac{1}{12}[9]=\frac{1}{4} \times \frac{15}{2}-\frac{3}{4} \\ & =\frac{15}{8}-\frac{3}{4}=\frac{9}{8} \text{ sq. units } \end{aligned} $
Hence, the correct option is $(d)$.
-
Option (a) $\frac{3}{8}$ sq. units: This option is incorrect because the calculated area of the region bounded by the curve and the straight line is $\frac{9}{8}$ sq. units, which is significantly larger than $\frac{3}{8}$ sq. units. The integration and algebraic steps show that the area is not as small as $\frac{3}{8}$ sq. units.
-
Option (b) $\frac{5}{8}$ sq. units: This option is incorrect because the calculated area of the region bounded by the curve and the straight line is $\frac{9}{8}$ sq. units. The detailed integration process and algebraic simplification confirm that the area is larger than $\frac{5}{8}$ sq. units.
-
Option (c) $\frac{7}{8}$ sq. units: This option is incorrect because the calculated area of the region bounded by the curve and the straight line is $\frac{9}{8}$ sq. units. The integration and algebraic steps clearly show that the area is slightly larger than $\frac{7}{8}$ sq. units.
26. The area of the region bounded by the curve $y=\sqrt{16-x^{2}}$ and $x$-axis is
(a) $8 \pi$ sq. units
(b) $20 \pi$ sq. units
(c) $16 \pi$ sq. units
(d) $256 \pi$ sq. units
Show Answer
Solution
Here, equation of curve is $y=\sqrt{16-x^{2}}$
Required area
$ \begin{aligned} & =2[\int_0^{4} \sqrt{16-x^{2}} d x] \\ & =2[\frac{x}{2} \sqrt{16-x^{2}}+\frac{16}{2} \sin ^{-1} \frac{x}{4}]_0^{4} \\ & =2[(0+8 \sin ^{-1} \frac{4}{4})-(0+0)] \\ & =2[8 \sin ^{-1}(1)]=16 \cdot \frac{\pi}{2}=8 \pi \text{ sq. units } \end{aligned} $
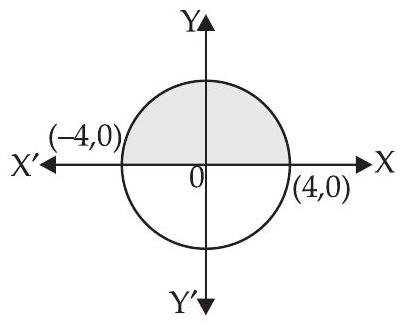
Hence, the correct option is $(a)$.
-
Option (b) $20 \pi$ sq. units is incorrect because the integral calculation and the geometric interpretation of the area under the curve $y=\sqrt{16-x^{2}}$ yield an area of $8 \pi$ sq. units, not $20 \pi$ sq. units.
-
Option (c) $16 \pi$ sq. units is incorrect because the integral calculation and the geometric interpretation of the area under the curve $y=\sqrt{16-x^{2}}$ yield an area of $8 \pi$ sq. units, not $16 \pi$ sq. units.
-
Option (d) $256 \pi$ sq. units is incorrect because the integral calculation and the geometric interpretation of the area under the curve $y=\sqrt{16-x^{2}}$ yield an area of $8 \pi$ sq. units, not $256 \pi$ sq. units.
27. Area of the region in the first quadrant enclosed by the $x$-axis, the line $y=x$ and the circle $x^{2}+y^{2}=32$ is
(a) $16 \pi$ sq. units
(c) $32 \pi$ sq. units
(b) $4 \pi$ sq. units
(d) 24 sq. units
Show Answer
Solution
Given equation of circle is $x^{2}+y^{2}=32 \Rightarrow x^{2}+y^{2}=(4 \sqrt{2})^{2}$ and the line is $y=x$ and the $x$-axis.
Solving the two equations we have
$x^{2}+x^{2} =32$
$\Rightarrow 2 x^{2} =32$
$\Rightarrow x^{2} =16$
$\therefore x = \pm 4$
Required area
$ \begin{aligned} & =\int_0^{4} x d x+\int_4^{4 \sqrt{2}} \sqrt{(4 \sqrt{2})^{2}-x^{2}} d x \\ & =\frac{1}{2}[x^{2}]_0^{4}+[\frac{x}{2} \sqrt{(4 \sqrt{2})^{2}-x^{2}}+\frac{32}{2} \sin ^{-1} \frac{x}{4 \sqrt{2}}]_4^{4 \sqrt{2}} \\ & =\frac{1}{2}[16-0]+[0+16 \sin ^{-1}(\frac{4 \sqrt{2}}{4 \sqrt{2}})-2 \sqrt{32-16}-16 \sin ^{-1} \frac{4}{4 \sqrt{2}}] \\ & =8+[16 \sin ^{-1}(1)-8-16 \sin ^{-1} \frac{1}{\sqrt{2}}] \\ & =8+16 \cdot \frac{\pi}{2}-8-16 \cdot \frac{\pi}{4}=8 \pi-4 \pi=4 \pi \text{ sq. units } \end{aligned} $
Hence, the correct option is $(b)$.
-
Option (a) $16 \pi$ sq. units: This option is incorrect because it overestimates the area. The actual area calculation involves integrating the region bounded by the line $y=x$ and the circle $x^2 + y^2 = 32$, which results in a smaller area of $4 \pi$ sq. units.
-
Option (c) $32 \pi$ sq. units: This option is incorrect because it significantly overestimates the area. The total area of the circle itself is $32 \pi$ sq. units, but the region in question is only a small part of the circle, specifically in the first quadrant and bounded by the line $y=x$ and the $x$-axis.
-
Option (d) 24 sq. units: This option is incorrect because it does not account for the circular segment’s area correctly. The area calculation involves both the triangular region under the line $y=x$ and the segment of the circle, which results in an area of $4 \pi$ sq. units, not 24 sq. units.
28. Area of the region bounded by the curve $y=\cos x$ between $x=0$ and $x=\pi$ is
(a) 2 sq. units
(b) 4 sq. units
(c) 3 sq. units
(d) 1 sq. unit
Show Answer
Solution
Given that: $y=\cos x, x=0, x=\pi$
Required area
$ \begin{aligned} & =\int_0^{\pi / 2} \cos x d x+|\int _{\pi / 2}^{\pi} \cos x d x| \\ & =[\sin x]_0^{\pi / 2}+|(\sin x) _{\pi / 2}^{\pi}| \\ & =[\sin \frac{\pi}{2}-\sin 0]+|[\sin \pi-\sin \frac{\pi}{2}]| \\ & =(1-0)+|0-1|=1+1=2 \text{ sq. units } \end{aligned} $
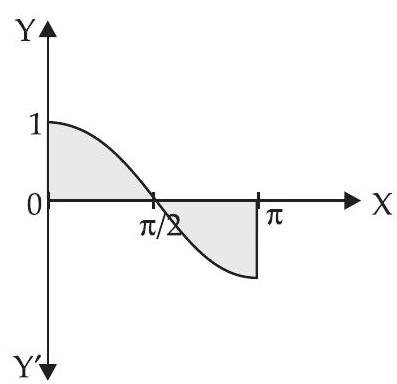
Hence, the correct option is $(a)$.
-
Option (b) 4 sq. units: This option is incorrect because the integral of the cosine function from (x = 0) to (x = \pi) results in an area of 2 square units, not 4. The calculation shows that the total area under the curve (y = \cos x) between these limits is 2 square units.
-
Option (c) 3 sq. units: This option is incorrect because the integral of the cosine function from (x = 0) to (x = \pi) results in an area of 2 square units, not 3. The calculation confirms that the total area under the curve (y = \cos x) between these limits is 2 square units.
-
Option (d) 1 sq. unit: This option is incorrect because the integral of the cosine function from (x = 0) to (x = \pi) results in an area of 2 square units, not 1. The calculation demonstrates that the total area under the curve (y = \cos x) between these limits is 2 square units.
29. The area of the region bounded by parabola $y^{2}=x$ and the straight line $2 y=x$ is
(a) $\frac{4}{3} sq$. units
(b) 1 sq. unit
(c) $\frac{2}{3} sq \cdot$ units
(d) $\frac{1}{3}$ sq. units
Show Answer
Solution
Given equation of parabola is $y^{2}=x$ and equation of straight line is $2 y=x$
Solving eqns. (i) and (ii) we get
$ \begin{aligned} & (\frac{x}{2})^{2}=x \Rightarrow \frac{x^{2}}{4}=x \Rightarrow x^{2}=4 x \\ & \Rightarrow x(x-4)=0 \quad \therefore x=0,4 \end{aligned} $
Required area
$ \begin{aligned} & =\int_0^{4} \sqrt{x} d x-\int_0^{4} \frac{x}{2} d x \\ & =\frac{2}{3}[x^{3 / 2}]_0^{4}-\frac{1}{2} \cdot \frac{1}{2}[x^{2}]_0^{4} \end{aligned} $
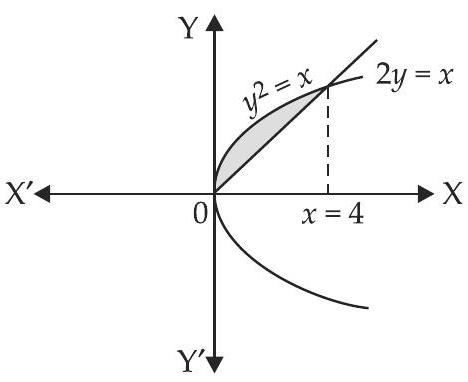
$=\frac{2}{3}[(4)^{3 / 2}-0]-\frac{1}{4}[(4)^{2}-0]=\frac{2}{3} \times 8-\frac{1}{4} \times 16$
$=\frac{16}{3}-4=\frac{4}{3}$ sq. units
Hence, the correct answer is $(a)$.
-
Option (b) 1 sq. unit: This option is incorrect because the calculated area of the region bounded by the parabola ( y^2 = x ) and the line ( 2y = x ) is (\frac{4}{3}) sq. units, not 1 sq. unit. The integration and subtraction of the areas under the curves do not result in 1 sq. unit.
-
Option (c) (\frac{2}{3}) sq. units: This option is incorrect because the area calculation involves integrating the functions (\sqrt{x}) and (\frac{x}{2}) over the interval from 0 to 4. The correct result of this integration and subtraction is (\frac{4}{3}) sq. units, not (\frac{2}{3}) sq. units.
-
Option (d) (\frac{1}{3}) sq. units: This option is incorrect because the area derived from the integration process is (\frac{4}{3}) sq. units. The value (\frac{1}{3}) sq. units does not match the result of the correct mathematical operations performed to find the area between the given curves.
30. The area of the region bounded by the curve $y=\sin x$, between the ordinates $x=0$ and $x=\frac{\pi}{2}$ and the $x$-axis is
(a) 2 sq. units
(b) 4 sq. units
(c) 3 sq. units
(d) 1 sq. units
Show Answer
Solution
Given equation of curve is $y=\sin x$ between $x=0$ and $x=\frac{\pi}{2}$ Area of required region
$ \begin{aligned} & =\int_0^{\pi / 2} \sin x d x=-[\cos x]_0^{\pi / 2} \\ & =-[\cos \frac{\pi}{2}-\cos 0] \\ & =-[0-1]=1 \text{ sq. unit } \end{aligned} $
- Option (a) is incorrect because the integral of (\sin x) from (0) to (\frac{\pi}{2}) does not yield 2 sq. units. The correct integral calculation results in 1 sq. unit.
- Option (b) is incorrect because the integral of (\sin x) from (0) to (\frac{\pi}{2}) does not yield 4 sq. units. The correct integral calculation results in 1 sq. unit.
- Option (c) is incorrect because the integral of (\sin x) from (0) to (\frac{\pi}{2}) does not yield 3 sq. units. The correct integral calculation results in 1 sq. unit.
31. The area of the region bounded by the ellipse $\frac{x^{2}}{25}+\frac{y^{2}}{16}=1$ is
(a) $20 \pi$ sq. units
(b) $20 \pi^{2}$ sq. units
(c) $16 \pi^{2}$ sq. units
(d) $25 \pi$ sq. units
Show Answer
Solution
Given equation of ellipse is $\frac{x^{2}}{25}+\frac{y^{2}}{16}=1$
$\Rightarrow \frac{y^{2}}{16}=1-\frac{x^{2}}{25} \Rightarrow y^{2}=\frac{16}{25}(25-x^{2})$
$\therefore \quad y=\frac{4}{5} \sqrt{25-x^{2}}$
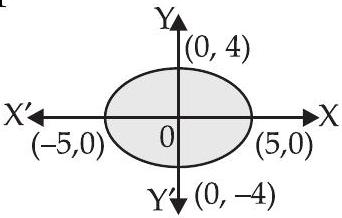
$\therefore$ Since the ellipse is symmetrical about the axes.
$\therefore$ Required area $=4 \times \int_0^{5} \frac{4}{5} \sqrt{25-x^{2}} d x=4 \times \frac{4}{5} \int_0^{5} \sqrt{(5)^{2}-x^{2}} d x$
$ \begin{aligned} & =\frac{16}{5}[\frac{x}{2} \sqrt{(5)^{2}-x^{2}}+\frac{25}{2} \sin ^{-1} \frac{x}{5}]_0^{5} \\ & =\frac{16}{5}[0+\frac{25}{2} \cdot \sin ^{-1}(\frac{5}{5})-0-0]=\frac{16}{5}[\frac{25}{2} \cdot \sin ^{-1}(1)] \\ & =\frac{16}{5}[\frac{25}{2} \cdot \frac{\pi}{2}]=20 \pi \text{ sq. units } \end{aligned} $
Hence, the correct answer is (a).
-
Option (b) $20 \pi^{2}$ sq. units: This option is incorrect because the area of an ellipse is given by the formula $\pi \times a \times b$, where $a$ and $b$ are the semi-major and semi-minor axes, respectively. The correct calculation yields $20 \pi$ sq. units, not $20 \pi^{2}$ sq. units. The presence of $\pi^2$ is a mathematical error.
-
Option (c) $16 \pi^{2}$ sq. units: This option is incorrect for similar reasons as option (b). The area of the ellipse should be calculated using the formula $\pi \times a \times b$. The correct area is $20 \pi$ sq. units, and the inclusion of $\pi^2$ and the incorrect numerical factor of 16 instead of 20 makes this option incorrect.
-
Option (d) $25 \pi$ sq. units: This option is incorrect because it miscalculates the area of the ellipse. The correct area is $20 \pi$ sq. units, derived from the semi-major axis $a = 5$ and the semi-minor axis $b = 4$. The factor of 25 is incorrect and does not align with the correct formula for the area of an ellipse.
32. The area of the region bounded by the circle $x^{2}+y^{2}=1$ is
(a) $2 \pi$ sq. units
(b) $\pi$ sq. units
(c) $3 \pi$ sq. units
(d) $4 \pi$ sq. units
Show Answer
Solution
Given equation of circle is
$x^{2}+y^{2}=1 \Rightarrow y=\sqrt{1-x^{2}}$
Since the circle is symmetrical about the axes.
$\therefore$ Required area $=4 \times \int_0^{1} \sqrt{1-x^{2}} d x$
$=4[\frac{x}{2} \sqrt{1-x^{2}}+\frac{1}{2} \sin ^{-1} x]_0^{1}$
$=4[0+\frac{1}{2} \sin ^{-1}(1)-0-0]$
$=4 \times \frac{1}{2} \times \frac{\pi}{2}=\pi$ sq. units
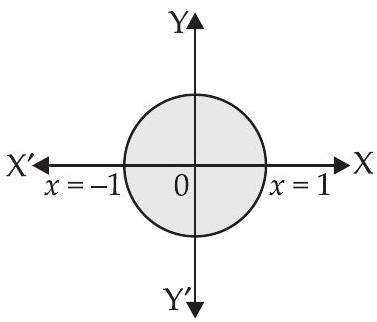
Hence, the correct answer is $(b)$.
-
Option (a) $2 \pi$ sq. units is incorrect because the area of a circle with radius 1 is given by the formula $\pi r^2$. For a circle with radius 1, the area is $\pi \times 1^2 = \pi$ sq. units, not $2 \pi$ sq. units.
-
Option (c) $3 \pi$ sq. units is incorrect because, as mentioned, the area of a circle with radius 1 is $\pi$ sq. units. There is no mathematical basis for the area being $3 \pi$ sq. units.
-
Option (d) $4 \pi$ sq. units is incorrect because the area of a circle with radius 1 is $\pi$ sq. units. The value $4 \pi$ sq. units would correspond to a circle with a radius of 2, not 1.
33. The area of the region bounded by the curve $y=x+1$ and the lines $x=2$ and $x=3$ is
(a) $\frac{7}{2}$ sq. units
(b) $\frac{9}{2}$ sq. units
(c) $\frac{11}{2}$ sq. units
(d) $\frac{13}{2}$ sq. units
Show Answer
Solution
Given equation of lines are
$ y=x+1, x=2 \text{ and } x=3 $
Required area
$ \begin{aligned} & =\int_2^{3}(x+1) d x=[\frac{x^{2}}{2}+x]_2^{3} \\ & =(\frac{9}{2}+3)-(\frac{4}{2}+2) \\ & =\frac{15}{2}-4=\frac{7}{2} \text{ sq.units } \end{aligned} $
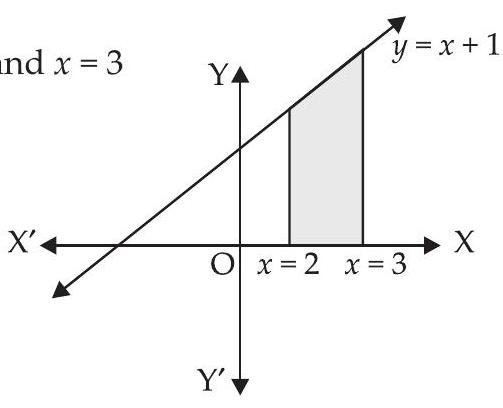
Hence, the correct option is (a).
-
Option (b) $\frac{9}{2}$ sq. units is incorrect because the integral calculation of the area between $x=2$ and $x=3$ for the function $y=x+1$ results in $\frac{7}{2}$ sq. units, not $\frac{9}{2}$ sq. units.
-
Option (c) $\frac{11}{2}$ sq. units is incorrect because the integral calculation of the area between $x=2$ and $x=3$ for the function $y=x+1$ results in $\frac{7}{2}$ sq. units, not $\frac{11}{2}$ sq. units.
-
Option (d) $\frac{13}{2}$ sq. units is incorrect because the integral calculation of the area between $x=2$ and $x=3$ for the function $y=x+1$ results in $\frac{7}{2}$ sq. units, not $\frac{13}{2}$ sq. units.
34. The area of the region bounded by the curve $x=2 y+3$ and the lines $y=1$ and $y=-1$ is
(a) 4 sq. units
(b) $\frac{3}{2}$ sq.units
(c) 6 sq. units
(d) 8 sq. units
Show Answer
Solution
Given equations of lines are $x=2 y+3, y=1$ and $y=-1$
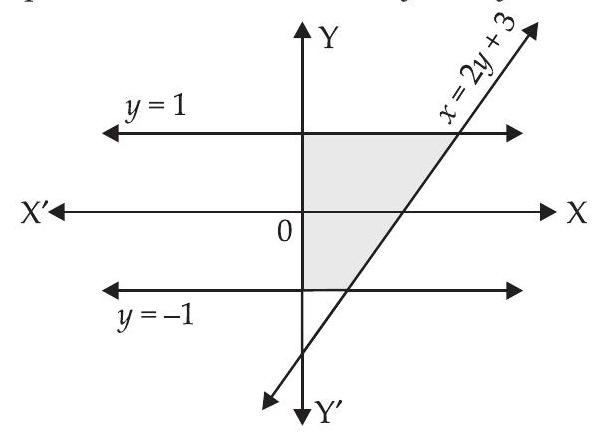
$ \begin{aligned} \text{ Required area } & =\int _{-1}^{1}(2 y+3) d y \\ & =2 \cdot \frac{1}{2}[y^{2}] _{-1}^{1}+3[y] _{-1}^{1} \\ & =(1-1)+3(1+1)=6 \text{ sq. units } \end{aligned} $
Hence, the correct answer is (c).
-
Option (a) 4 sq. units: This option is incorrect because the integral calculation of the area between the given curve and the lines ( y = 1 ) and ( y = -1 ) results in 6 sq. units, not 4 sq. units. The integral (\int_{-1}^{1} (2y + 3) , dy) evaluates to 6, as shown in the solution.
-
Option (b) (\frac{3}{2}) sq. units: This option is incorrect because the integral calculation of the area between the given curve and the lines ( y = 1 ) and ( y = -1 ) results in 6 sq. units, not (\frac{3}{2}) sq. units. The integral (\int_{-1}^{1} (2y + 3) , dy) evaluates to 6, as shown in the solution.
-
Option (d) 8 sq. units: This option is incorrect because the integral calculation of the area between the given curve and the lines ( y = 1 ) and ( y = -1 ) results in 6 sq. units, not 8 sq. units. The integral (\int_{-1}^{1} (2y + 3) , dy) evaluates to 6, as shown in the solution.










