SATHEE: Sequence and Series

Sequence and Series

Short Answer Type Questions

1. The first term of an AP is a and the sum of the first $p$ terms is zero, show that the sum of its next $q$ terms is $\frac{- a (p + q) q}{p - 1}$ .

Show Answer

Solution

Let the common difference of an AP is $d$ . According to the question,

$S_{p} = 0$

$\Rightarrow \frac{p}{2} [2 a + (p - 1) d] = 0 [∵ S_{n} = \frac{n}{2} 2 a + (n - 1) d]$

$\Rightarrow 2 a + (p - 1) d = 0$

$∴ d = \frac{- 2 a}{p - 1}$

$Now, sum of next q terms = S_{p + q} - S_{p} = S_{p + q} - 0$

$= \frac{p + q}{2} [2 a + (p + q - 1) d]$

$= \frac{p + q}{2} [2 a + (p - 1) d + q d]$

$= \frac{p + q}{2} [2 a + (p - 1) \cdot \frac{- 2 a}{p - 1} + \frac{q (- 2 a)}{p - 1}]$

$= \frac{p + q}{2} [2 a + (- 2 a) - \frac{2 a q}{p - 1}]$

$= \frac{p + q}{2} [\frac{- 2 a q}{p - 1}]$

$= \frac{- a (p + q) q}{(p - 1)}$

$\frac{p}{2} [2 a + (p - 1) d] = 0 ∵ S_{n} = \frac{n}{2} 2 a + (n - 1) d$

2. A man saved ₹ 66000 in $20 y r$ . In each succeeding year after the first year, he saved ₹ 200 more than what he saved in the previous year. How much did he save in the first year?

Show Answer

Solution

Let saved in first year ₹ a. Since, each succeeding year an increment ₹ 200 has made. So,it forms an AP whose

First term $= a$ , common difference $(d) = 200$ and $n = 20 y r$

$\begin{aligned} ∴ S_{20} = \frac{20}{2} [2 a + (20 - 1) d] [∵ S_{n} = \frac{n}{2} 2 a + (n - 1) d] \\ \Rightarrow 66000 = 10 [2 a + 19 d] \\ \Rightarrow 66000 = 20 a + 190 d \\ \Rightarrow 66000 = 20 a + 190 \times 200 \\ \Rightarrow 20 a = 66000 - 38000 \\ \Rightarrow 20 a = 28000 \\ ∴ a = \frac{28000}{20} = 1400 \end{aligned}$

Hence, he saved ₹ 1400 in the first year.

3. A man accepts a position with an initial salary of ₹ 5200 per month. It is understood that he will receive an automatic increase of ₹ 320 in the very next month and each month thereafter.

(i) Find his salary for the tenth month.

(ii) What is his total earnings during the first year?

Show Answer

Solution

Since, the man get a fixed increment of ₹ 320 each month. Therefore, this forms an AP whose First term $= 5200$ and Common difference $(d) = 320$

(i) Salary for tenth month i.e., for $n = 10$ ,

$\begin{matrix} \Rightarrow & a_{10} = a + (n - 1) d \\ \Rightarrow & a_{10} = 5200 + (10 - 1) \times 320 \\ ∴ & a_{10} = 5200 + 9 \times 320 \\ ∴ & a_{10} = 5200 + 2880 \\ a_{10} = 8080 \end{matrix}$

(ii) Total earning during the first year.

In a year there are 12 month i.e., $n = 12$ ,

$\begin{aligned} S_{12} & = \frac{12}{2} [2 \times 5200 + (12 - 1) 320] \\ = 6 [10400 + 11 \times 320] \\ = 6 [10400 + 3520] = 6 \times 13920 = 83520 \end{aligned}$

4. If the $p$ th and $q$ th terms of a GP are $q$ and $p$ respectively, then show that its $(p + q)$ th term is $\frac{q^{p}}{p^{q}} \frac{1}{p - q}$ .

Show Answer

Solution

Let the first term and common ratio of GP be a and $r$ , respectively.

According to the question, $p$ th term $= q$

$\Rightarrow a \cdot r^{p - 1} = q \dots (i)$

and

$q$ th term $= p$

$\Rightarrow$

$a r^{q - 1} = p \dots (i i)$

On dividing Eq. (i) by Eq. (ii), we get

$\begin{aligned} \frac{a r^{p - 1}}{a r^{q - 1}} = \frac{q}{p} \\ \Rightarrow r^{p - 1 - q + 1} = \frac{q}{p} \\ \Rightarrow r^{p - q} = \frac{q}{p} \Rightarrow r = (\frac{q}{p})^{\frac{1}{p - q}} \end{aligned}$

On substituting the value of $r$ in Eq. (i), we get

$\begin{aligned} a (\frac{q}{p})^{\frac{p - 1}{p - q}} = q \Rightarrow a = \frac{q}{(\frac{q}{p})^{\frac{p - 1}{p - q}}} = q \cdot (\frac{p}{q})^{\frac{p - 1}{p - q}} \\ ∴ (p + q) th term, T_{p + q} = a \cdot r^{p + q - 1} = q \cdot {\frac{p}{q}}^{\frac{p - 1}{p - q}} \cdot (r)^{p + q - 1} \\ = q \cdot (\frac{p}{q})^{\frac{p - 1}{p - q}} (\frac{q}{p})^{\frac{1}{p - q}}^{p + q - 1} = q \cdot (\frac{p}{q})^{\frac{p - 1}{p - q}} \frac{q}{\frac{p}{p}} \\ = q \cdot (\frac{p}{q})^{\frac{p - q - 1}{p - q}} {\frac{p}{q}}^{\frac{- (p + q - 1)}{p - q}} = q \cdot (\frac{p}{q})^{\frac{p - 1}{p - q} - \frac{(p + q - 1)}{p - q}} \\ = q \cdot (\frac{p}{q})^{\frac{p - 1 - p - q + 1}{p - q}} = q \cdot (\frac{p}{q})^{\frac{- q}{p - q}} \\ a = q \cdot (\frac{p}{q})^{\frac{p - 1}{p - q}} \end{aligned}$

Now, $(p + q)$ th term i.e., $a_{p + q} = a r^{p + q - 1}$

$\begin{aligned} = q \cdot (\frac{p}{q})^{\frac{p - 1}{p - q}} \cdot (\frac{q}{p})^{\frac{p + q - 1}{p - q}} \\ = q \cdot \frac{q \frac{p + q - 1 - p + 1}{p - q}}{p \frac{p + q - 1 - p + 1}{p - q}} = q \cdot (\frac{q^{\frac{q}{p - q}}}{p^{\frac{q}{p - q}}}) \end{aligned}$

5. A carpenter was hired to build 192 window frames. The first day he made five frames and each day, thereafter he made two more frames than he made the day before. How many days did it take him to finish the job?

Show Answer

Solution

Here, $a = 5$ and $d = 2$

Let he finished the job in $n$ days.

Then,

$\begin{aligned} S_{n} = 192 \\ S_{n} = \frac{n}{2} [2 a + (n - 1) d] \end{aligned}$

$\begin{matrix} \Rightarrow & 192 = \frac{n}{2} [2 \times 5 + (n - 1) 2] \\ \Rightarrow & 192 = \frac{n}{2} [10 + 2 n - 2] \end{matrix}$

$\Rightarrow$	192	$= \frac{n}{2} [8 + 2 n]$
$\Rightarrow$	192	$= 4 n + n^{2}$
$\Rightarrow$	$n^{2} + 4 n - 192$	$= 0$
$\Rightarrow$	$(n - 12) (n + 16)$	$= 0$
$\Rightarrow$	$n$	$n$	$= 12, - 16$
	$n$		$= 12$

6. The sum of interior angles of a triangle is $180^{\circ}$ . Show that the sum of the interior angles of polygons with $3, 4, 5, 6, \dots$ sides form an arithmetic progression. Find the sum of the interior angles for a 21 sided polygon.

Show Answer

Solution

We know that, sum of interior angles of a polygon of side $n = (2 n - 4) \times 90^{\circ} = (n - 2) \times 180^{\circ}$ Sum of interior angles of a polygon with sides 3 is 180 .

Sum of interior angles of polygon with side $4 = (4 - 2) \times 180^{\circ} = 360^{\circ}$

Similarly, sum of interior angles of polygon with side $5, 6, 7 \dots$ are $540^{\circ}, 720^{\circ}, 900^{\circ}, \dots$

The series will be $180^{\circ}, 360^{\circ} 540^{\circ}, 720^{\circ}, 900^{\circ}, .$ .

Here, $a = 180^{\circ}$

and $d = 360^{\circ} - 180^{\circ} = 180^{\circ}$

Since, common difference is same between two consecutive terms of the series.

So, it form an AP.

We have to find the sum of interior angles of a 21 sides polygon.

It means, we have to find the 19th term of the above series.

$\begin{aligned} ∴ a_{19} = a + (19 - 1) d \\ = 180 + 18 \times 180 = 3420 \end{aligned}$

7. A side of an equilateral triangle is $20 c m$ long. A second equilateral triangle is inscribed in it by joining the mid-points of the sides of the first triangle. The process is continued as shown in the accompanying diagram. Find the perimeter of the sixth inscribed equilateral triangle.

Show Answer

Solution

Side of equilateral $△ A B C = 20 c m$ . By joining the mid-points of this triangle, we get another equilateral triangle of side equal to half of the length of side of $△ A B C$ .

Continuing in this way, we get a set of equilateral triangles with side equal to half of the side of the previous triangle.

$∴$ Perimeter of first triangle $= 20 \times 3 = 60 c m$

Perimeter of second triangle $= 10 \times 3 = 30 c m$

Perimeter of third triangle $= 5 \times 3 = 15 c m$

Now, the series will be $60, 30, 15, \dots$

Here,

$a = 60$

$∴ r = \frac{30}{60} = \frac{1}{2} [∵ \frac{second term}{first term} = r$ ]

We have, to find perimeter of sixth inscribed triangle. It is the sixth term of the series.

$\begin{aligned} ∴ & a_{6} & = a r^{6 - 1} \\ = 60 \times {\frac{1}{2}}^{5} = \frac{60}{32} = \frac{15}{8} c m & [∵ a_{n} = a r^{n - 1}] \end{aligned}$

8. In a potato race 20 potatoes are placed in a line at intervals of $4 m$ with the first potato $24 m$ from the starting point. A contestant is required to bring the potatoes back to the starting place one at a time. How far would he run in bringing back all the potatoes?

Show Answer

Solution

According to the given information, we have following diagram.

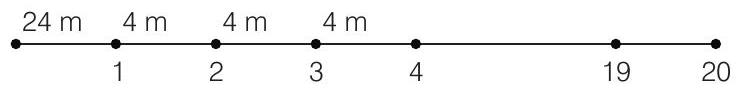
Distance travelled to bring first potato $= 24 + 24 = 2 \times 24 = 48 m$

Distance travelled to bring second potato $= 2 (24 + 4) = 2 \times 28 = 56 m$

Distance travelled to bring third potato $= 2 (24 + 4 + 4) = 2 \times 32 = 64 m$

Then, the series of distances are $48, 56, 64, \dots$

Here,

$\begin{aligned} a = 48 \\ d = 56 - 48 = 8 \end{aligned}$

$and n = 20$

To find the total distance that he run in bringing back all potatoes, we have to find the sum of 20 terms of the above series.

$\begin{matrix} ∴ S_{20} & = \frac{20}{2} [2 \times 48 + 19 \times 8] ∵ S_{n} = \frac{n}{2} 2 a + (n - 1) d \\ = 10 [96 + 152] \\ = 10 \times 248 = 2480 m \end{matrix}$

9. In a cricket tournament 16 school teams participated. A sum of ₹ 8000 is to be awarded among themselves as prize money. If the last placed team is awarded ₹ 275 in prize money and the award increases by the same amount for successive finishing places, how much amount will the first place team receive?

Show Answer

Solution

Let the first place team got ₹ a.

Since, award money increases by the same amount for successive finishing places. Therefore series is an AP.

Let the constant amount be $d$ .

Here, l = 275, n = 16 and $S_{16} = 8000$

$∴$ l = a+(n-)d

$\Rightarrow$ l = a+(16-1)(-d)

[we take common difference (−ve) because series is decreasing]

$\Rightarrow$ 275 = a-15d

and

$\Rightarrow 8000 = 8 [2 a + (16 - 1) (- d)]$

$\Rightarrow 8000 = 8 [2 a - 15 d]$

$\Rightarrow 1000 = 2 a - 15 d$

On subtracting Eq. (i) from Eq. (ii), we get

$(2 a - 15 d) - (a - 15 d) = 1000 - 275$

$\Rightarrow 2 a - 15 d - a + 15 d = 725$

$∴ a = 725$

Hence, first place team receive ₹ 725 .

10. If $a_{1}, a_{2}, a_{3}, \dots, a_{n}$ are in AP, where $a_{i} > 0$ for all $i$ , show that

$\frac{1}{\sqrt{a_{1}} + \sqrt{a_{2}}} + \frac{1}{\sqrt{a_{2}} + \sqrt{a_{3}}} + \dots + \frac{1}{\sqrt{a_{n - 1}} + \sqrt{a_{n}}} = \frac{n - 1}{\sqrt{a_{1}} + \sqrt{a_{n}}}$

Show Answer

Solution

Since, $a_{1}, a_{2}, a_{3}, \dots, a_{n}$ are in AP.

$\begin{aligned} \Rightarrow a_{2} - a_{1} = a_{3} - a_{2} = \dots = a_{n} - a_{n - 1} = d [common difference] \\ If a_{2} - a_{1} = d, then (\sqrt{a_{2}})^{2} - (\sqrt{a_{1}})^{2} = d \\ \Rightarrow (\sqrt{a_{2}} - \sqrt{a_{1}}) (\sqrt{a_{2}} + \sqrt{a_{1}}) = d \\ \Rightarrow \frac{1}{\sqrt{a_{1}} + \sqrt{a_{2}}} = \frac{\sqrt{a_{2}} - \sqrt{a_{1}}}{d} \\ \frac{1}{\sqrt{a_{2}} + \sqrt{a_{3}}} = \frac{\sqrt{a_{3}} - \sqrt{a_{2}}}{d} \\ \frac{1}{\sqrt{a_{n - 1}} + \sqrt{a_{n}}} = \frac{\sqrt{a_{n}} - \sqrt{a_{n - 1}}}{d} \end{aligned}$

On adding these terms, we get

$\begin{aligned} \frac{1}{\sqrt{a_{1}} + \sqrt{a_{2}}} + \frac{1}{\sqrt{a_{2}} + \sqrt{a_{3}}} + \dots + \frac{1}{\sqrt{a_{n - 1}} + \sqrt{a_{n}}} \\ = & \frac{1}{d} [\sqrt{a_{2}} - \sqrt{a_{1}} + \sqrt{a_{3}} - \sqrt{a_{2}} + \dots + \sqrt{a_{n}} - \sqrt{a_{n - 1}}] [using above relations] \\ = & \frac{1}{d} [\sqrt{a_{n}} - \sqrt{a_{1}}] \end{aligned}$

Again,

$a_{n} = a_{1} + (n - 1) d [∵ T_{n} = a + (n - 1) d]$

$\Rightarrow$

$a_{n} - a_{1} = (n - 1) d$

$\begin{matrix} \Rightarrow & (\sqrt{a_{n}})^{2} - (\sqrt{a_{1}})^{2} = (n - 1) d \\ \Rightarrow & (\sqrt{a_{n}} - \sqrt{a_{1}}) (\sqrt{a_{n}} + \sqrt{a_{1}}) = (n - 1) d \Rightarrow \sqrt{a_{n}} - \sqrt{a_{1}} = \frac{(n - 1) d}{\sqrt{a_{n}} + \sqrt{a_{1}}} \end{matrix}$

On putting this value in Eq. (i), we get

$\begin{matrix} \frac{1}{\sqrt{a_{1}} + \sqrt{a_{2}}} + \frac{1}{\sqrt{a_{2}} + \sqrt{a_{3}}} + \dots + \frac{1}{\sqrt{a_{n - 1}} + \sqrt{a_{n}}} \\ = \frac{(n - 1) d}{d (\sqrt{a_{n}} + \sqrt{a_{1}})} = \frac{n - 1}{\sqrt{a_{n}} + \sqrt{a_{1}}} \end{matrix}$

11. Find the sum of the series

$(3^{3} - 2^{3}) + (5^{3} - 4^{3}) + (7^{3} - 6^{3}) + \dots to (i) n terms. (ii) 10 terms.$

Show Answer

Solution

Given series, $(3^{3} - 2^{3}) + (5^{3} - 4^{3}) + (7^{3} - 6^{3}) +$

$= (3^{3} + 5^{3} + 7^{3} + \dots) - (2^{3} + 4^{3} + 6^{3} + \dots)$

Let $T_{n}$ be the $n$ th term of the series (i),

then $T_{n} = (n .$ th term of ${.3}^{3}, 5^{3}, 7^{3}, \dots) - (n .$ th term of ${.2}^{3}, 4^{3}, 6^{3}, \dots) = (2 n + 1)^{3} - (2 n)^{3}$

$\begin{aligned} = (2 n + 1 - 2 n) [(2 n + 1)^{2} + (2 n + 1) 2 n + (2 n)^{2}] [∵ a^{3} - b^{3} = (a - b) (a^{2} + a b + b^{2})] \\ = [4 n^{2} + 1 + 4 n + 4 n^{2} + 2 n + 4 n^{2}] = [12 n^{2} + 6 n] + 1 \end{aligned}$

(i) Let $S_{n}$ denote the sum of $n$ term of series (i). Then,

$\begin{aligned} S_{n} & = Σ T_{n} = Σ (12 n^{2} + 6 n) \\ = 12 Σ n^{2} + 6 Σ n + Σ n \\ = 12 \cdot \frac{n (n + 1) (2 n + 1)}{6} + \frac{6 n (n + 1)}{2} + n \\ = 2 n (n + 1) (2 n + 1) + 3 n (n + 1) + n \\ = 2 n (n + 1) (2 n + 1) + 3 n (n + 1) + n \\ = (2 n^{2} + 2 n) (2 n + 1) + 3 n^{2} + 3 n + n \\ = 4 n^{3} + 2 n^{2} + 4 n^{2} + 2 n + 3 n^{2} + 3 n + n \\ = 4 n^{3} + 9 n^{2} + 6 n \\ S_{10} & = 4 \times (10)^{3} + 9 \times (10)^{2} + 6 \times 10 \\ = 4 \times 1000 + 9 \times 100 + 60 \\ = 4000 + 900 + 60 = 4960 \end{aligned}$

(ii) Sum of 10 terms,

12. Find the $r$ th term of an AP sum of whose first $n$ terms is $2 n + 3 n^{2}$ .

Show Answer

Solution

Given that, sum of $n$ terms of an AP,

$\begin{aligned} S_{n} & = 2 n + 3 n^{2} \\ T_{n} & = S_{n} - S_{n - 1} \\ = (2 n + 3 n^{2}) - [2 (n - 1) + 3 (n - 1)^{2}] \\ = (2 n + 3 n^{2}) - [2 n - 2 + 3 (n^{2} + 1 - 2 n)] \\ = (2 n + 3 n^{2}) - (2 n - 2 + 3 n^{2} + 3 - 6 n) \\ = 2 n + 3 n^{2} - 2 n + 2 - 3 n^{2} - 3 + 6 n \\ ∴ & = 6 n - 1 \\ ∴ rth term T_{r} & = 6 r - 1 \end{aligned}$

Long Answer Type Questions

13. If $A$ is the arithmetic mean and $G_{1}, G_{2}$ be two geometric mean between any two numbers, then prove that $2 A = \frac{G_{1}^{2}}{G_{2}} + \frac{G_{2}^{2}}{G_{1}}$ .

Show Answer

Solution

Let the numbers be $a$ and $b$ .

Then,

$A = \frac{a + b}{2}$

$\Rightarrow 2 A = a + b$

and $G_{1}, G_{2}$ be geometric mean between $a$ and $b$ , then $a, G_{1}, G_{2}, b$ are in GP.

Let $r$ be the common ratio.

$\begin{aligned} Then, \\ b = a r^{4 - 1} \\ \Rightarrow b = a r^{3} \Rightarrow \frac{b}{a} = r^{3} \\ ∴ r = {\frac{b}{a}}^{1 / 3} \end{aligned}$

$\begin{aligned} Now, \\ G_{1} = a r = a {\frac{b}{a}}^{1 / 3} \\ and \\ G_{2} = a r^{2} = a {\frac{b}{a}}^{2 / 3} \\ R H S = \frac{G_{1}^{2}}{G_{2}} + \frac{G_{2}^{2}}{G_{1}} = \frac{a {\frac{b}{a}}^{1 / 3^{2}}}{a \frac{b}{a}} + \frac{a {\frac{b}{a}}^{2 / 3}}{a \frac{b}{a}} \\ = \frac{a^{2} \frac{b^{2 / 3}}{a}}{a \frac{b}{a}} + \frac{a^{2} \frac{b}{a}}{a \frac{b}{a}} \\ = a + a \frac{b}{a} = a + b = 2 A \\ = L H S \end{aligned}$

14. If $θ_{1}, θ_{2}, θ_{3}, \dots, θ_{n}$ are in AP whose common difference is $d$ , show that $\sec θ_{1} \sec θ_{2} + \sec θ_{2} \sec θ_{3} + \dots + \sec θ_{n - 1} \sec θ_{n} = \frac{\tan θ_{n} - \tan θ_{1}}{\sin d}$ .

Show Answer

Solution

Since, $θ_{1}, θ_{2}, θ_{3}, \dots, θ_{n}$ are in AP.

$\Rightarrow θ_{2} - θ_{1} = θ_{3} - θ_{2} = \dots = θ_{n} - θ_{n - 1} = d$

$∵ r = {\frac{b}{a}}^{1 / 3}$

Now, we have to prove

$\sec q_{1} \sec q_{2} + \sec q_{2} \sec q_{3} + \dots + \sec q_{n - 1} \sec θ_{n} = \frac{\tan θ_{n} - \tan θ_{1}}{\sin d}$

or it can be written as

sind $[\sec θ_{1} \sec θ_{2} + \sec θ_{2} \sec θ_{3} + \dots + \sec θ_{n - 1} \sec θ_{n}] = \tan θ_{n} - \tan θ_{1}$

Now, taking only first term of LHS

$\begin{aligned} \sin d \sec θ_{1} \sec θ_{2} & = \frac{\sin d}{\cos θ_{1} \cos θ_{2}} = \frac{\sin (θ_{2} - θ_{1})}{\cos θ_{1} \cos θ_{2}} \\ = \frac{\sin θ_{2} \cos θ_{1} - \cos θ_{2} \sin θ_{1}}{\cos θ_{1} \cos θ_{2}} \\ = \frac{\sin θ_{2} \cos θ_{1}}{\cos θ_{1} \cos θ_{2}} - \frac{\cos θ_{2} \sin θ_{1}}{\cos θ_{1} \cos θ_{2}} = \tan θ_{2} - \tan θ_{1} \end{aligned}$

Similarly, we can solve other terms which will be $\tan θ_{3} - \tan θ_{2}, \tan θ_{4} - \tan θ_{3}, \dots$

$\begin{aligned} ∴ L H S & = \tan θ_{2} - \tan θ_{1} + \tan θ_{3} - \tan θ_{2} + \dots + \tan θ_{n} - \tan θ_{n - 1} \\ = - \tan θ_{1} + \tan θ_{n} = \tan θ_{n} - \tan θ_{1} \\ = RHS Hence proved. \end{aligned}$

15. If the sum of $p$ terms of an AP is $q$ and the sum of $q$ terms is $p$ , then show that the sum of $p + q$ terms is $- (p + q)$ . Also, find the sum of first $p - q$ terms (where, $p > q$ ).

Show Answer

Solution

Let first term and common difference of the AP be a and $d$ , respectively. Then,

$S_{p} = q$

$\Rightarrow$

$\begin{aligned} \frac{p}{2} [2 a + (p - 1) d] & = q \\ 2 a + (p - 1) d & = \frac{2 q}{p} \end{aligned}$

and

$S_{q} = p$

$\begin{matrix} \Rightarrow & \frac{q}{2} [2 a + (q - 1) d] & = p \\ \Rightarrow & 2 a + (q - 1) d & = \frac{2 p}{q} \end{matrix}$

On subtracting Eq. (ii) from Eq. (i), we get

$2 a + (p - 1) d - 2 a - (q - 1) d = \frac{2 q}{p} - \frac{2 p}{q}$

$\Rightarrow [(p - 1) - (q - 1)] d = \frac{2 q^{2} - 2 p^{2}}{p q}$

$\Rightarrow [p - 1 - q + 1] d = \frac{2 (q^{2} - p^{2})}{p q}$

$∴ d = \frac{- 2 (p + q)}{p q}$

On substituting the value of $d$ in Eq. (i), we get

$\begin{aligned} 2 a + (p - 1) \frac{- 2 (p + q)}{p q} = \frac{2 q}{p} \\ \Rightarrow 2 a = \frac{2 q}{p} + \frac{2 (p + q) (p - 1)}{p q} \\ \Rightarrow a = \frac{q}{p} + \frac{(p + q) (p - 1)}{p q} \\ Now, S_{p + q} = \frac{p + q}{2} [2 a + (p + q - 1) d] \\ = \frac{p + q}{2} \frac{2 q}{p} + \frac{2 (p + q) (p - 1)}{p q} - \frac{(p + q - 1) 2 (p + q)}{p q} \\ = (p + q) \frac{q}{p} + \frac{(p + q) (p - 1) - (p + q - 1) (p + q)}{p q} \\ = (p + q) \frac{q}{p} + \frac{(p + q) (p - 1 - p - q + 1)}{p q} \\ = p + q \frac{q}{p} - \frac{p + q}{p} = (p + q) \frac{q - p - q}{p} \\ S_{p + q} = - (p + q) \\ S_{p - q} = \frac{p - q}{2} [2 a + (p - q - 1) d] \end{aligned}$

$\begin{aligned} = \frac{p - q}{2} \frac{2 q}{p} + \frac{2 (p + q) (p - 1)}{p q} - \frac{(p - q - 1) 2 (p + q)}{p q} \\ = (p - q) \frac{q}{p} + \frac{p + q (p - 1 - p + q + 1)}{p q} \\ = (p - q) \frac{q}{p} + \frac{(p + q) q}{p q} \\ = (p - q) \frac{q}{p} + \frac{p + q}{p} = (p - q) \frac{(p + 2 q)}{p} \end{aligned}$

16. If $p$ th, $q$ th and $r$ th terms of an AP and GP are both and $c$ respectively, then show that $a^{b - c} \cdot b^{c - a} \cdot c^{a - b} = 1$ .

Show Answer

Solution

Let $A, d$ are the first term and common difference of AP and $x, R$ are the first term and common ratio of GP, respectively.

According to the given condition,

and

$\begin{aligned} A + (p - 1) d & = a \\ A + (q - 1) d & = b \\ A + (r - 1) d & = c \\ a & = x R^{p - 1} \\ b & = x R^{q - 1} \\ c & = x R^{r - 1} \end{aligned}$

On subtracting Eq. (ii) from Eq. (i), we get

$d (p - 1 - q + 1) = a - b$

$\Rightarrow a - b = d (p - q)$

On subtracting Eq. (iii) from Eq. (ii), we get

$d (q - 1 - r + 1) = b - c$

$\Rightarrow b - c = d (q - r)$

On subtracting Eq. (i) from Eq. (iii), we get

$d (r - 1 - p + 1) = c - a$

$\Rightarrow c - a = d (r - p)$

Now, we have to prove $a^{b - c} b^{c - a} c^{a - b} = 1$

$Taking LHS = a^{b - c} b^{c - a} c^{a - b}$

Using Eqs. (iv), (v), (vi) and (vii), (viii), (ix),

$\begin{aligned} L H S & = (x R^{p - 1})^{d} (q - r) (x R^{q - 1})^{d} (r - p) (x R^{r - 1})^{d (p - q)} \\ = x^{d (q - r) + d (r - p) + d (p - q)} R^{(p - 1) d (q - r) + (q - 1) d (r - p) + (r - 1) d (p - q)} \\ = x^{d (q - r + r - p + p - q)} \end{aligned}$

$\begin{aligned} R^{d (p q - p r - q + r + q r - p q - r + p + r p - r q - p + q)} & = x^{0} R^{0} = 1 \\ = R H S \end{aligned}$

Objective Type Questions

17. If the sum of $n$ terms of an AP is given by $S_{n} = 3 n + 2 n^{2}$ , then the common difference of the AP is

(a) 3

(b) 2

(d) 4

Show Answer

Solution

(d) Given, $S_{n} = 3 n + 2 n^{2}$

First term of the AP,

$\begin{aligned} ∴ T_{1} = 3 \times 1 + 2 (1)^{2} = 3 + 2 = 5 \\ and T_{2} = S_{2} - S_{1} \\ = [3 \times 2 + 2 \times (2)^{2}] - [3 \times 1 + 2 \times (1)^{2}] \\ = 14 - 5 = 9 \end{aligned}$

$∴$ Common difference $(d) = T_{2} - T_{1} = 9 - 5 = 4$

Option (a) 3: The common difference is calculated as ( T_2 - T_1 ). Given ( S_n = 3n + 2n^2 ), the first term ( T_1 = 5 ) and the second term ( T_2 = 9 ). Therefore, the common difference ( d = 9 - 5 = 4 ), not 3.
Option (b) 2: The common difference is calculated as ( T_2 - T_1 ). Given ( S_n = 3n + 2n^2 ), the first term ( T_1 = 5 ) and the second term ( T_2 = 9 ). Therefore, the common difference ( d = 9 - 5 = 4 ), not 2.
Option (c) 6: The common difference is calculated as ( T_2 - T_1 ). Given ( S_n = 3n + 2n^2 ), the first term ( T_1 = 5 ) and the second term ( T_2 = 9 ). Therefore, the common difference ( d = 9 - 5 = 4 ), not 6.

18. If the third term of GP is 4 , then the product of its first 5 terms is

(a) $4^{3}$

(b) $4^{4}$

(d) None of these

Show Answer

Solution

Let $a$ and $r$ the first term and common ratio, respectively.

Then,

$a r^{2} = 4$

Product of first 5 terms $= a \cdot a r \cdot a r^{2} \cdot a r^{3} \cdot a r^{4}$

$= a^{5} r^{10} = (a r^{2})^{5} = (4)^{5}$

Option (a) $4^{3}$ is incorrect because the product of the first 5 terms of the GP is calculated as $(a r^{2})^{5}$ , which equals $4^{5}$ , not $4^{3}$ .
Option (b) $4^{4}$ is incorrect because the product of the first 5 terms of the GP is calculated as $(a r^{2})^{5}$ , which equals $4^{5}$ , not $4^{4}$ .
Option (d) None of these is incorrect because the correct answer is indeed one of the given options, specifically $4^{5}$ .

19. If 9 times the 9 th term of an AP is equal to 13 times the 13 th term, then the 22 nd term of the AP is

(a) 0

(b) 22

(d) 220

Show Answer

Solution

(a) Let the first term be a and common difference be $d$ .

According to the question, $9 \cdot T_{9} = 13 \cdot T_{13}$

$\begin{matrix} \Rightarrow & 9 (a + 8 d) & = 13 (a + 12 d) \\ \Rightarrow & 9 a + 72 d & = 13 a + 156 d \\ \Rightarrow & (9 a - 13 a) & = 156 d - 72 d \\ \Rightarrow & - 4 a & = 84 d \\ \Rightarrow & a & = - 21 d \\ \Rightarrow & a + 21 d & = 0 \\ ∴ & 22nd term i.e., T_{22} & = [a + 21 d] \\ T_{22} & = 0 [using Eq. (i)] \end{matrix}$

Option (b) 22: This option is incorrect because, based on the derived equation ( a = -21d ), the 22nd term ( T_{22} ) is calculated as ( a + 21d ). Substituting ( a = -21d ) into this equation results in ( T_{22} = -21d + 21d = 0 ), not 22.
Option (c) 198: This option is incorrect because the calculation of the 22nd term ( T_{22} ) using the derived equation ( a = -21d ) results in ( T_{22} = 0 ). There is no scenario in the given arithmetic progression where the 22nd term equals 198.
Option (d) 220: This option is incorrect because, similar to the previous options, the calculation of the 22nd term ( T_{22} ) using ( a = -21d ) results in ( T_{22} = 0 ). The 22nd term cannot be 220 based on the given conditions and derived equations.

20. If $x, 2 y$ and $3 z$ are in AP where the distinct numbers $x, y$ and $z$ are in GP, then the common ratio of the GP is

(a) 3

(b) $\frac{1}{3}$

(d) $\frac{1}{2}$

Show Answer

Solution

(b) Given, $x, 2 y$ and $3 z$ are in AP.

Then,

$2 y = \frac{x + 3 z}{2}$

$\Rightarrow y = \frac{x + 3 z}{4}$

$\Rightarrow 4 y = x + 3 z$

and $x, y, z$ are in GP

$\begin{matrix} Then, & \frac{y}{x} = \frac{z}{y} = λ \\ \Rightarrow & y = x λ and z = λ y = λ^{2} x \end{matrix}$

On substituting these values in Eq. (i), we get

$4 (x λ) = x + 3 (λ^{2} x)$

$\Rightarrow 4 λ x = x + 3 λ^{2} x$

$\Rightarrow 4 λ = 1 + 3 λ^{2}$

$\Rightarrow 3 λ^{2} - 4 λ + 1 = 0$

$\Rightarrow (3 λ - 1) (λ - 1) = 0$

$∴ λ = \frac{1}{3}, λ = 1$

Option (a) 3: If the common ratio of the GP were 3, then ( y = 3x ) and ( z = 9x ). Substituting these into the AP condition ( 4y = x + 3z ) would yield ( 4(3x) = x + 3(9x) ), which simplifies to ( 12x = 28x ), a contradiction.
Option (c) 2: If the common ratio of the GP were 2, then ( y = 2x ) and ( z = 4x ). Substituting these into the AP condition ( 4y = x + 3z ) would yield ( 4(2x) = x + 3(4x) ), which simplifies to ( 8x = 13x ), a contradiction.
Option (d) (\frac{1}{2}): If the common ratio of the GP were (\frac{1}{2}), then ( y = \frac{x}{2} ) and ( z = \frac{x}{4} ). Substituting these into the AP condition ( 4y = x + 3z ) would yield ( 4(\frac{x}{2}) = x + 3(\frac{x}{4}) ), which simplifies to ( 2x = \frac{5x}{4} ), a contradiction.

21. If in an AP, $S_{n} = q n^{2}$ and $S_{m} = q m^{2}$ , where $S_{r}$ denotes the sum of $r$ terms of the AP, then $S_{q}$ equals to

(a) $\frac{q^{3}}{2}$

(b) $m n q$

(d) $(m + n) q^{2}$

Show Answer

Solution

$\begin{aligned} ∴ S_{1} = q, S_{2} = 4 q, S_{3} = 9 q and S_{4} = 16 q \\ Now, T_{1} = q \\ ∴ T_{2} = S_{2} - S_{1} = 4 q - q = 3 q \\ T_{3} = S_{3} - S_{2} = 9 q - 4 q = 5 q \\ T_{4} = S_{4} - S_{3} = 16 q - 9 q = 7 q \end{aligned}$

So, the series is $q, 3 q, 5 q, 7 q, \dots$

$\begin{aligned} Here, a = q and d = 3 q - q = 2 q \\ ∴ S_{q} = \frac{q}{2} [2 \times q + (q - 1) 2 q] \\ = \frac{q}{2} \times [2 q + 2 q^{2} - 2 q] = \frac{q}{2} \times 2 q^{2} = q^{3} \end{aligned}$

Option (a) $\frac{q^{3}}{2}$ : This option is incorrect because the formula for the sum of the first $q$ terms of an arithmetic progression (AP) is given by $S_{q} = \frac{q}{2} [2 a + (q - 1) d]$ . When we substitute $a = q$ and $d = 2 q$ into this formula, we get $S_{q} = q^{3}$ , not $\frac{q^{3}}{2}$ .
Option (b) $m n q$ : This option is incorrect because the sum of the first $q$ terms of the AP does not depend on the variables $m$ and $n$ . The correct sum $S_{q}$ is derived solely from the given relationship $S_{n} = q n^{2}$ and the properties of the AP, leading to $S_{q} = q^{3}$ .
Option (d) $(m + n) q^{2}$ : This option is incorrect because, similar to option (b), the sum of the first $q$ terms of the AP does not involve the variables $m$ and $n$ . The correct sum $S_{q}$ is determined by the given relationship $S_{n} = q n^{2}$ and the properties of the AP, resulting in $S_{q} = q^{3}$ .

22. Let $S_{n}$ denote the sum of the first $n$ terms of an AP, if $S_{2 n} = 3 S_{n}$ , then $S_{3 n} : S_{n}$ is equal to

(a) 4

(b) 6

(d) 10

Show Answer

Solution

(b) Let first term be a and common difference be $d$ .

Then, $S_{n} = \frac{n}{2} [2 a + (n - 1) d]$

$∴ S_{2 n} = \frac{2 n}{2} [2 a + (2 n - 1) d]$

$S_{2 n} = n [2 a + (2 n - 1) d]$

$S_{3 n} = \frac{3 n}{2} [2 a + (3 n - 1) d]$

According to the question, $S_{2 n} = 3 S_{n}$

$\begin{aligned} \Rightarrow & n [2 a + (2 n - 1) d] & = 3 \frac{n}{2} [2 a + (n - 1) d] \\ \Rightarrow & 4 a + (4 n - 2) d & = 6 a + (3 n - 3) d \\ \Rightarrow & - 2 a + (4 n - 2 - 3 n + 3) d & = 0 \\ \Rightarrow & - 2 a + (n + 1) d & = 0 \\ \Rightarrow & d & = \frac{2 a}{n + 1} \end{aligned}$

Now,

$\begin{aligned} \frac{S_{3 n}}{S_{n}} & = \frac{\frac{3 n}{2} [2 a + (3 n - 1) d]}{\frac{n}{2} [2 a + (n - 1) d]} = \frac{6 a + (9 n - 3) \frac{2 a}{n + 1}}{2 a + (n - 1) \frac{2 a}{n + 1}} \\ = \frac{6 a n + 6 a + 18 a n - 6 a}{2 a n + 2 a + 2 a n - 2 a} \\ = \frac{24 a n}{4 a n} = \frac{S_{3 n}}{S_{n}} = 6 \end{aligned}$

Option (a) 4: This option is incorrect because the ratio ( \frac{S_{3n}}{S_n} ) does not simplify to 4. The correct ratio, as derived, is 6, not 4.
Option (c) 8: This option is incorrect because the ratio ( \frac{S_{3n}}{S_n} ) does not simplify to 8. The correct ratio, as derived, is 6, not 8.
Option (d) 10: This option is incorrect because the ratio ( \frac{S_{3n}}{S_n} ) does not simplify to 10. The correct ratio, as derived, is 6, not 10.

23. The minimum value of $4^{x} + 4^{1 - x}, x \in R$ is

(a) 2

(b) 4

(d) 0

Show Answer

Solution

(b) We know that,

$A M \geq G M$

$\begin{matrix} \Rightarrow & \frac{4^{x} + 4^{1 - x}}{2} \geq \sqrt{4^{x} \cdot 4^{1 - x}} \\ \Rightarrow & 4^{x} + 4^{1 - x} \geq 2 \sqrt{4} \\ \Rightarrow & 4^{x} + 4^{1 - x} \geq 2 \cdot 2 \\ \Rightarrow & 4^{x} + 4^{1 - x} \geq 4 \end{matrix}$

Option (a) 2: This option is incorrect because the minimum value of (4^x + 4^{1-x}) is 4, as shown by the application of the AM-GM inequality. The expression (4^x + 4^{1-x}) cannot be less than 4.
Option (c) 1: This option is incorrect because the minimum value of (4^x + 4^{1-x}) is 4, not 1. The AM-GM inequality demonstrates that the expression is always at least 4.
Option (d) 0: This option is incorrect because the minimum value of (4^x + 4^{1-x}) is 4, not 0. The AM-GM inequality shows that the expression cannot be zero or any value less than 4.

24. Let $S_{n}$ denote the sum of the cubes of the first $n$ natural numbers and $s_{n}$ denote the sum of the first $n$ natural numbers, then $\sum_{r = 1}^{n} \frac{S_{r}}{S_{4}}$ equals to (a) $\frac{n (n + 1) (n + 2)}{6}$ (b) $\frac{n (n + 1)}{2}$ (c) $\frac{n^{2} + 3 n + 2}{2}$ (d) None of these

Show Answer

Solution

(a) $\sum_{r = 1}^{n} \frac{S_{r}}{S_{r}} = \frac{S_{1}}{S_{1}} + \frac{S_{2}}{S_{2}} + \frac{S_{3}}{S_{3}} + \dots + \frac{S_{n}}{S_{n}}$

Let $T_{n}$ be the $n$ th term of the above series.

$\begin{aligned} ∴ T_{n} & = \frac{S_{n}}{S_{n}} = \frac{\frac{n (n + 1)^{2}}{2}}{\frac{n (n + 1)}{2}} \\ = \frac{n (n + 1)}{2} = \frac{1}{2} [n^{2} + n] \end{aligned}$

$∴$ Sum of the above series $= Σ T_{n} = \frac{1}{2} [Σ n^{2} + Σ n]$

$\begin{aligned} = \frac{1}{2} \frac{n (n + 1) (2 n + 1)}{6} + \frac{n (n + 1)}{2} = \frac{1}{2} \cdot \frac{n (n + 1)}{2} \frac{(2 n + 1)}{3} + 1 \\ = \frac{1}{4} n (n + 1) \frac{2 n + 1 + 3}{3} = \frac{1}{4 \times 3} n (n + 1) (2 n + 4) \\ = \frac{1}{12} n (n + 1) (2 n + 4) = \frac{1}{6} n (n + 1) (n + 2) \end{aligned}$

Option (b) $\frac{n (n + 1)}{2}$ : This option represents the sum of the first ( n ) natural numbers, denoted as ( s_n ). However, the given problem involves the sum of the cubes of the first ( n ) natural numbers, which is a different series. Therefore, this option does not correctly represent the sum of the given series.
Option (c) $\frac{n^{2} + 3 n + 2}{2}$ : This option does not correspond to any known formula related to the sum of cubes or the sum of natural numbers. It appears to be an arbitrary expression that does not fit the context of the problem, which involves the sum of the cubes of the first ( n ) natural numbers.
Option (d) None of these: This option is incorrect because the correct answer is provided in option (a). The correct sum of the series ( \sum_{r=1}^{n} \frac{S_{r}}{S_4} ) is indeed ( \frac{n(n+1)(n+2)}{6} ), as derived in the solution.

25. If $t_{n}$ denotes the $n$ th term of the series $2 + 3 + 6 + 11 + 18 + \dots$ , then $t_{50}$ is

(a) $49^{2} - 1$

(b) $49^{2}$

(d) $49^{2} + 2$

Show Answer

Solution

(d) Let $S_{n}$ be sum of the series $2 + 3 + 6 + 11 + 18 + \dots + t_{50}$ .

$\begin{matrix} ∴ & S_{n} = 2 + 3 + 6 + 11 + 18 + \dots + t_{50} \\ and & S_{n} = 0 + 2 + 3 + 6 + 11 + 18 + \dots + t_{49} + t_{50} \end{matrix}$

On subtracting Eq. (ii) from Eq. (i), we get

$\begin{aligned} 0 & = 2 + 1 + 3 + 5 + 7 + \dots + t_{50} \\ \Rightarrow & t_{50} & = 2 + 1 + 3 + 5 + 7 + \dots upto 49 terms \\ ∴ & t_{50} & = 2 + [1 + 3 + 5 + 7 + \dots upto 49 terms] \\ = 2 + \frac{49}{2} [2 \times 1 + 48 \times 2] \\ = 2 + \frac{49}{2} \times [2 + 96] \\ = 2 + [49 + 49 \times 48] \\ = 2 + 49 \times 49 = 2 + (49)^{2} \end{aligned}$

Option (a) $49^{2} - 1$ : This option is incorrect because the calculation of the 50th term, $t_{50}$ , involves adding 2 to the square of 49, not subtracting 1 from it. The correct formula derived from the series pattern is $t_{50} = 49^{2} + 2$ .
Option (b) $49^{2}$ : This option is incorrect because it does not account for the additional 2 that is added to the square of 49 in the correct formula. The correct term is $t_{50} = 49^{2} + 2$ , not just $49^{2}$ .
Option (c) $50^{2} + 1$ : This option is incorrect because it uses the wrong base for the square. The correct term is based on 49, not 50. The correct formula is $t_{50} = 49^{2} + 2$ , not $50^{2} + 1$ .

26. The lengths of three unequal edges of a rectangular solid block are in GP. If the volume of the block is $216 c m^{3}$ and the total surface area is $252 c m^{2}$ , then the length of the longest edge is

(a) $12 c m$

(b) $6 c m$

(d) $3 c m$

Show Answer

Solution

(a) Let the length, breadth and height of rectangular solid block is $\frac{a}{r}$ , a and ar, respectively.

$\begin{aligned} ∴ Volume = \frac{a}{r} \times a \times a r = 216 c m^{3} \\ \Rightarrow a^{3} = 216 \Rightarrow a^{3} = 6^{3} \\ ∴ a = 6 \\ Surface area = 2 \frac{a^{2}}{r} + a^{2} r + a^{2} = 252 \\ \Rightarrow 2 a^{2} \frac{1}{r} + r + 1 = 252 \\ \Rightarrow 2 \times 36 \frac{1 + r^{2} + r}{r} = 252 \\ \Rightarrow \frac{1 + r^{2} + r}{r} = \frac{252}{2 \times 36} \end{aligned}$

$\begin{aligned} \Rightarrow 1 + r^{2} + r = \frac{126}{36} r \Rightarrow 1 + r^{2} + r = \frac{21}{6} r \\ \Rightarrow 6 + 6 r^{2} + 6 r = 21 r \Rightarrow 6 r^{2} - 15 r + 6 = 0 \\ \Rightarrow 2 r^{2} - 5 r + 2 = 0 \Rightarrow (2 r - 1) (r - 2) = 0 \\ ∴ r = \frac{1}{2}, 2 \\ For r = \frac{1}{2} : Length = \frac{a}{r} = \frac{6 \times 2}{1} = 12 \\ Breadth = a = 6 \\ Height = a r = 6 \times \frac{1}{2} = 3 \\ For r = 2 : Length = \frac{a}{r} = \frac{6}{2} = 3 \\ Breadth = a = 6 \\ Height = a r = 6 \times 2 = 12 \end{aligned}$

Option (b) $6 c m$ : This option is incorrect because $6 c m$ is the length of the middle edge (breadth) of the rectangular solid block, not the longest edge. The longest edge must be either $12 c m$ or $3 c m$ depending on the value of $r$ .
Option (c) $18 c m$ : This option is incorrect because the calculations show that the possible lengths of the edges are $12 c m$ , $6 c m$ , and $3 c m$ . There is no edge with a length of $18 c m$ .
Option (d) $3 c m$ : This option is incorrect because $3 c m$ is the length of the shortest edge of the rectangular solid block, not the longest edge. The longest edge must be either $12 c m$ or $3 c m$ depending on the value of $r$ .

Fillers

27. If $a, b$ and $c$ are in GP, then the value of $\frac{a - b}{b - c}$ is equal to ……

Show Answer

Solution

Given that, $a, b$ and $c$ are in GP.

$\begin{aligned} Then, \\ \frac{b}{a} = \frac{c}{b} = r \\ \Rightarrow b = a r \Rightarrow c = b r \\ \begin{array}{c} \Rightarrow & \frac{a - b}{b - c} = \frac{a - a r}{a r - b r} = \frac{a (1 - r)}{r (a - b)} = \frac{a (1 - r)}{r (a - a r)} \end{array} \\ = \frac{a (1 - r)}{a r (1 - r)} = \frac{1}{r} \\ ∴ \frac{a - b}{b - c} = \frac{1}{r} = \frac{a}{b} or \frac{b}{c} \end{aligned}$

28. The sum of terms equidistant from the beginning and end in an AP is equal to ……

Show Answer

Solution

Let AP be $a, a + d, a + 2 d \dots a + (n - 1) d$

$\begin{aligned} ∴ a_{1} + a_{n} = a + a + (n - 1) d \\ = 2 a + (n - 1) d \\ a_{2} + a_{n - 1} = (a + d) + [a + (n - 2) d] \\ = 2 a + (n - 1) d \\ a_{2} + a_{n - 1} = a_{1} + a_{n} \\ a_{3} + a_{n - 2} = (a + 2 d) + [a + (n - 3) d] \\ = 2 a + (n - 1) d \\ = a_{1} + a_{n} \end{aligned}$

an $A P$ is equal to [first term +last term].

29. The third term of a GP is 4 , the product of the first five terms is …..

Show Answer

Solution

It is given that, $T_{3} = 4$

Let $a$ and $r$ the first term and common ration, respectively.

Then,

$a r^{2} = 4$

Product of first 5 terms $= a r \cdot a r \cdot a r^{2} \cdot a r^{3} \cdot a r^{4}$

$= a^{5} r^{10} = (a r^{2})^{5} = (4)^{5}$

[using Eq. (i)]

True/False

30. Two sequences cannot be in both AP and GP together.

Show Answer

Solution

False

Consider an AP $a, a + d, a + 2 d, \dots$

Now,

$\frac{a_{2}}{a_{1}} = \frac{a + d}{a} \neq \frac{a + 2 d}{a + d}$

Thus, AP is not a GP.

31. Every progression is a sequence but the converse, i.e., every sequence is also a progression need not necessarily be true.

Show Answer

Solution

True

Consider the progression $a, a + d, a + 2 d, \dots$

and sequence of prime number $2, 3, 5, 7, 11, \dots$

Clearly, progression is a sequence but sequence is not progression because it does not follow a specific pattern.

32. Any term of an AP (except first) is equal to half the sum of terms which are equidistant from it.

Show Answer

Solution

True

Consider an AP $a, a + d, a + 2 d, \dots$

Now,

$\begin{aligned} a_{2} + a_{4} & = a + d + a + 3 d \\ = 2 a + 4 d = 2 a_{3} \\ a_{3} & = \frac{a_{2} + a_{4}}{2} \\ \frac{a_{3} + a_{5}}{2} & = \frac{a + 2 d + a + 4 d}{2} = \frac{2 a + 6 d}{2} \\ = a + 3 d = a_{4} \end{aligned}$

$\Rightarrow a_{3} = \frac{a_{2} + a_{4}}{2}$

Again

Hence, the statement is true.

33. The sum or difference of two GP, is again a GP.

Show Answer

Solution

False

Let two GP are $a, a r_{1}, a r_{1}^{2}, a r_{2}^{3}, \dots$ and $b, b r_{2}, b r_{2}^{2}, b r_{2}^{3}, \dots$

Now, sum of two GP $a + b, (a r_{1} + b r_{2}), (a r_{1}^{2} + b r_{2}^{2}), \dots$

Now, $\frac{T_{2}}{T_{1}} = \frac{a r_{1} + b r_{2}}{a + b}$ and $\frac{T_{3}}{T_{2}} = \frac{a r_{1}^{2} + b r_{2}^{2}}{a r_{1} + b r_{2}}$

$∴ \frac{T_{2}}{T_{1}} \neq \frac{T_{3}}{T_{2}}$

Again, difference of two GP is $a - b, a r_{1} - b r_{2}, a r_{1}^{2} - b r_{2}^{2}, \dots$

Now,

$\frac{T_{2}}{T_{1}} = \frac{a r_{1} - b r_{2}}{a - b} and \frac{T_{3}}{T_{2}} = \frac{a r_{1}^{2} - b r_{2}^{2}}{a r_{1} - b r_{2}}$

$∴ \frac{T_{2}}{T_{1}} \neq \frac{T_{3}}{T_{2}}$

So, the sum or difference of two GP is not a GP. Hence, the statement is false.

34. If the sum of $n$ terms of a sequence is quadratic expression, then it always represents an AP.

Show Answer

Solution

False

Let

$\begin{aligned} S_{n} & = a n^{2} + b n + c \\ S_{1} & = a + b + c \\ a_{1} & = a + b + c \\ S_{2} & = 4 a + 2 b + c \\ a_{2} & = S_{2} - S_{1} \\ = 4 a + 4 b + c - (a + b + c) = 3 a + b \\ S_{3} & = 9 a + 3 b + c \\ a_{3} & = S_{3} - S_{2} = 5 a + b \end{aligned}$

Now, $a_{2} - a_{1} = (3 a + b) - (a + b + c) = 2 a - c$

$a_{3} - a_{2} = (5 a + b) - (3 a + b) = 2 a$

Now, $a_{2} - a_{1} \neq a_{3} - a_{2}$

Hence, the statement is false.

Matching The Columns

35. Match the following.

	Column I		Column II
(i)	$4, 1, \frac{1}{4}, \frac{1}{16}$	(a)	AP
(ii) $2, 3, 5, 7$	(b)	Sequence
(iii)	$13, 8, 3, - 2, - 7$	(c)	GP

Show Answer

Solution

(i) $4, 1, \frac{1}{4}, \frac{1}{16}$

$\Rightarrow$

$\frac{T_{2}}{T_{1}} = \frac{1}{4} \Rightarrow \frac{T_{3}}{T_{2}} = \frac{1}{4} \Rightarrow \frac{T_{4}}{T_{3}} = \frac{1 / 16}{1 / 4} = \frac{1}{4}$

Hence, it is a GP.

(ii) $2, 3, 5, 7$

$\begin{matrix} ∵ & T_{2} - T_{1} = 3 - 2 = 1 \\ ∵ & T_{3} - T_{2} = 5 - 3 = 2 \\ T_{2} - T_{1} \neq T_{3} - T_{2} \end{matrix}$

Hence, it is not an AP.

Again,

$\frac{T_{2}}{T_{1}} = 3 / 2 \Rightarrow \frac{T_{3}}{T_{2}} = 5 / 3$

$∵ \frac{T_{2}}{T_{1}} \neq \frac{T_{3}}{T_{2}}$

It is not a GP.

Hence, it is a sequence.

(iii) $13, 8, 3, - 2, - 7$

$∵ T_{2} - T_{1} = T_{3} - T_{2}$

Hence, it is an AP.

$\begin{aligned} T_{2} - T_{1} = 8 - 13 = - 5 \\ T_{3} - T_{2} = 3 - 8 = - 5 \\ T_{2} - T_{1} = T_{3} - T_{2} \end{aligned}$

36. Match the following.

Column I	Column II
(i) $1^{2} + 2^{2} + 3^{2} + \dots + n^{2}$	(a) $\frac{n (n + 1)^{2}}{2}$
(ii) $1^{3} + 2^{3} + 3^{3} + \dots + n^{3}$	(b) $n (n + 1)$
(iii) $2 + 4 + 6 + \dots + 2 n$	(c) $\frac{n (n + 1) (2 n + 1)}{6}$
(iv) $1 + 2 + 3 + \dots + n$	(d) $\frac{n (n + 1)}{2}$

Show Answer

Solution

(i) $1^{2} + 2^{2} + 3^{2} + \dots + n^{2}$

Consider the identity, $(k + 1)^{3} - k^{3} = 3 k^{2} + 3 k + 1$

On putting $k = 1, 2, 3, \dots, (n - 1), n$ successively, we get

$\begin{aligned} 2^{3} - 1^{3} = 3 \cdot 1^{2} + 3 \cdot 1 + 1 \\ 3^{3} - 2^{3} = 3 \cdot 2^{2} + 3 \cdot 2 + 1 \\ 4^{3} - 3^{3} = 3 \cdot 3^{2} + 3 \cdot 3 + 1 \end{aligned}$

$\begin{aligned} n^{3} - (n - 1)^{3} = 3 \cdot (n - 1)^{2} + 3 \cdot (n - 1) + 1 \\ (n + 1)^{3} - n^{3} = 3 \cdot n^{2} + 3 \cdot n + 1 \end{aligned}$

Adding columnwise, we get

$n^{3} + 3 n^{2} + 3 n = 3 \sum_{r = 1}^{n} r^{2} + 3 \frac{n (n + 1)}{2} + n ∵ \sum_{r = 1}^{n} r^{2} = \frac{n (n + 1)}{2}$

$\Rightarrow 3 \sum_{r = 1}^{n} r^{2} = n^{3} + 3 n^{2} + 3 n - \frac{3 n (n + 1)}{2} + n$

$\Rightarrow \sum_{r = 1}^{n} r^{2} = \frac{2 n^{3} + 3 n^{2} + n}{2} = \frac{n (n + 1) (2 n + 1)}{2}$

$\Rightarrow \sum_{r = 1}^{n} r^{2} = \frac{n (n + 1) (2 n + 1)}{6}$

Hence, $\sum_{r = 1}^{n} r^{2} = 1^{2} + 2^{2} + \dots + n^{2} = \frac{n (n + 1) (2 n + 1)}{6}$ (ii) $1^{3} + 2^{3} + 3^{3} + \dots + n^{3}$

Consider the identity $(k + 1)^{4} - k^{4} = 4 k^{3} + 6 k^{2} + 4 k + 1$

On putting $k = 1, 2, 3, \dots (n - 1), n$ successively, we get

$\begin{aligned} 2^{4} - 1^{4} = 4 \cdot 1^{3} + 6 \cdot 1^{2} + 4 \cdot 1 + 1 \\ 3^{4} - 2^{4} = 4 \cdot 2^{3} + 6 \cdot 2^{2} + 4 \cdot 2 + 1 \\ 4^{4} - 3^{4} = 4 \cdot 3^{3} + 6 \cdot 3^{2} + 4 \cdot 3 + 1 \end{aligned}$

$\begin{aligned} n^{4} - (n - 1)^{4} = 4 (n - 1)^{3} + 6 (n - 1)^{2} + 4 (n - 1) + 1 \\ (n + 1)^{4} - n^{4} = 4 \cdot n^{3} + 6 \cdot n^{2} + 4 \cdot n + 1 \end{aligned}$

Adding columnwise, we get

$(n + 1)^{4} - 1^{4} = 4 \cdot (1^{3} + 2^{3} + \dots + n^{3}) + 6 (1^{2} + 2^{2} + 3^{3} + \dots + n^{2})$

$+ 4 (1 + 2 + 3 + \dots + n) + (1 + 1 + \dots + 1) n terms$

$\Rightarrow n^{4} + 4 n^{3} + 6 n^{2} + 4 n = 4 \sum_{r = 1}^{n} r^{3} + 6 \sum_{r = 1}^{n} r^{2} + 4 \sum_{r = 1}^{n} r + n$

$\Rightarrow n^{4} + 4 n^{3} + 6 n^{2} + 4 n = 4 \sum_{r = 1}^{n} r^{3} + 6 \frac{n (n + 1) (2 n + 1)}{6} + 4 \frac{n (n + 1)}{2} + n$

$\Rightarrow \sum_{r = 1}^{n} r^{3} = \frac{n^{2} (n + 1)^{2}}{4}$

$\Rightarrow \sum_{r = 1}^{n} r^{3} = \frac{n (n + 1)}{2} = \sum_{r = 1}^{n} r^{2}$

$Hence, \sum_{r = 1}^{n} r^{3} = 1^{3} + 2^{3} + \dots + n^{3} = \frac{n (n + 1)^{2}}{2} = \sum_{r = 1}^{n} r^{2}$

(iii)

$\begin{aligned} 2 + 4 + 6 + \dots + 2 n & = 2 [1 + 2 + 3 + \dots + n] \\ = 2 \times \frac{n (n + 1)}{2} = n (n + 1) \end{aligned}$

(iv) Let

$S_{n} = 1 + 2 + 3 + \dots + n$

Clearly, it is an arithmetic series with first term, $a = 1$ ,

common difference,

$d = 1$

and

last term $= n$

$S_{n} = \frac{n}{2} (1 + n) = \frac{n (n + 1)}{2}$

Hence, $1 + 2 + 3 + \dots + n = \frac{n (n + 1)}{2}$ .

Binomial Theorem

Straight Lines

Sequence and Series

Short Answer Type Questions

Long Answer Type Questions

Objective Type Questions

Fillers

True/False

Matching The Columns

Table of Contents

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Mentorship

NCERT Exercise Video Solution

Sequence and Series

Short Answer Type Questions

Long Answer Type Questions

Objective Type Questions

Fillers

True/False

Matching The Columns

Table of Contents

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Mentorship

NCERT Exercise Video Solution

Menu