Introduction to Three Dimensional Geometry
Short Answer Type Questions
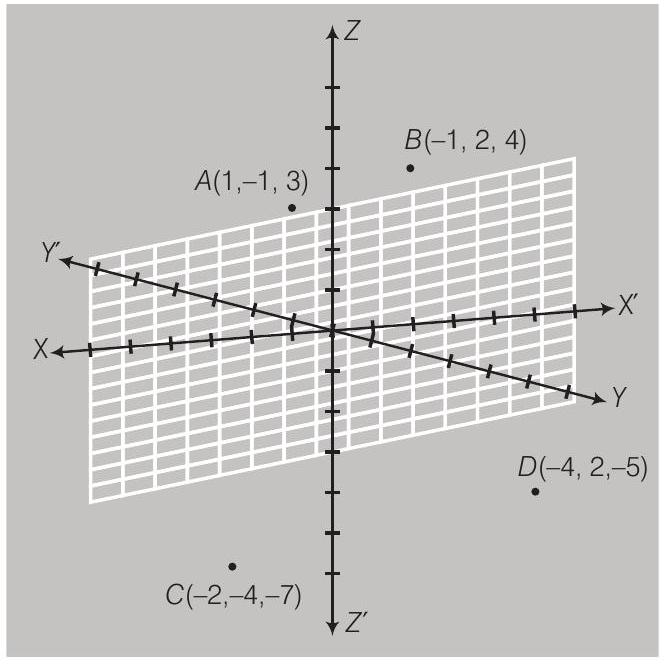
1. Locate the following points
(i)
(ii)
(iii)
(iv)
Show Answer
Solution
Given, coordinates are
(i)
(ii)
(iii)
(iv)
X-increment
2. Name the octant in which each of the following points lies.
(i)
(ii)
(iii)
(iv)
(v)
(iv)
(vii)
(viii)
Show Answer
Solution
(i) Point
(ii)
(iii)
(iv)
(v)
(vi)
(vii)
(viii)
3. If
(i)
(iii)
(ii)
Show Answer
Solution
The coordinates of
(i)
(ii)
(iii)
4. If
(i)
(ii)
(iii)
Show Answer
Solution
We know that, on
(i)
(ii)
(iii)
5. How far apart are the points
Show Answer
Thinking Process
Distance between two points
Solution
Given points,
6. Find the distance from the origin to
Show Answer
Solution
Distance from origin to the point
7. Show that, if
Show Answer
Solution
Given that,
Hence proved.
8. Show that the point
Show Answer
Thinking Process
If the three points
Solution
Given points,
So, the points
9. Three consecutive vertices of a parallelogram
Show Answer
Solution
Let the coordinates of the fourth vertices
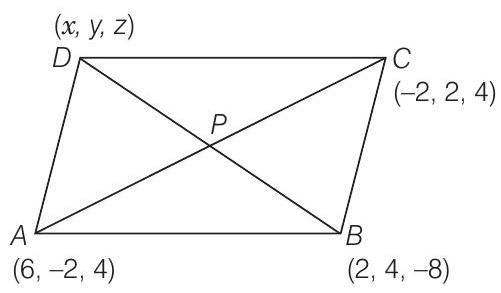
Mid-points of diagonal
and
Since, the mid-point of
Now,
So, the coordinates of fourth vertex
10. Show that the
Show Answer
Thinking Process
In a right angled triangle sum of the square of two sides is equal to square of third side.
Solution
Given that, the vertices of the
Hence, vertices
11. Find the third vertex of triangle whose centroid is origin and two vertices are
Show Answer
Thinking Process
The vertices of the
coordinates of the centroid Gare
Solution
Let third vertex of
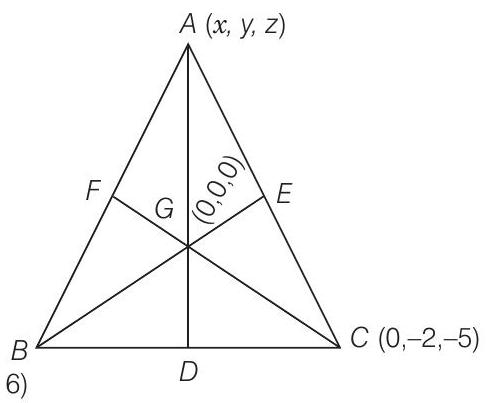
Given that, the coordinate of centroid
Hence, the third vertex of triangle is
12. Find the centroid of a triangle, the mid-point of whose sides are
Show Answer
Solution
Given that, mid-points of sides are
Let the vertices of the
Then, mid-point of
Similarly for the sides
On adding Eqs. (i) and (iv), we get
On adding Eqs. (ii) and (v), we get
On adding Eqs. (iii) and (vi), we get
From Eqs. (vii) and (x),
If
If
From Eqs. (xi) and (viii),
If
From Eqs. (xii) and (ix),
So, the points are
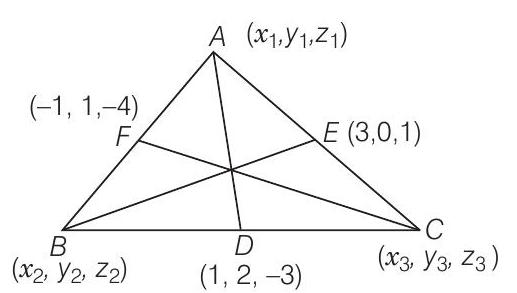
13. The mid-points of the sides of a triangle are
Show Answer
Solution
Let vertices of the
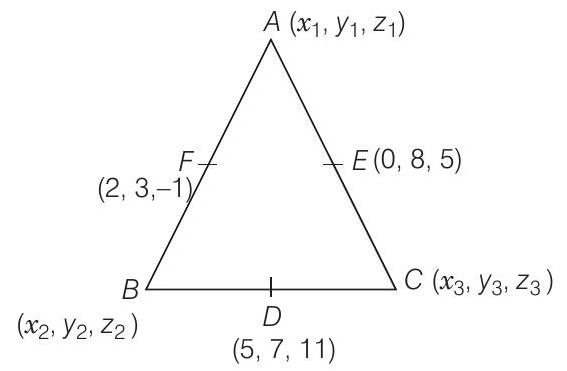
Similarly for the sides
From Eqs. (i) and (iv),
From Eqs. (ii) and (v),
From Eqs. (iii) and (vi),
From Eqs. (vii) and (x),
From Eqs. (viii) and (xi),
From Eqs. (ix) and (xii),
So, the vertices are
14. If the vertices of a parallelogram
Show Answer
Thinking Process
The diagonal of a parallelogram have the same vertices. Use this property to solve the problem.
Solution
Let the fourth vertex of the parallelogram
Now, mid-point of
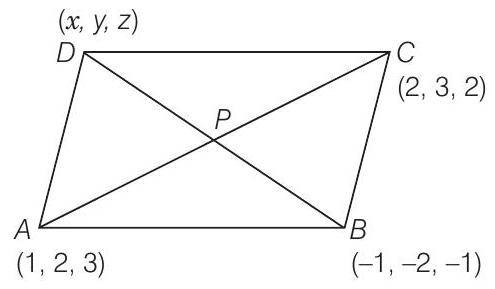
So, the coordinates of fourth vertex is
15. Find the coordinate of the points which trisect the line segment joining the points
Show Answer
Thinking Process
If point
Solution
Let the
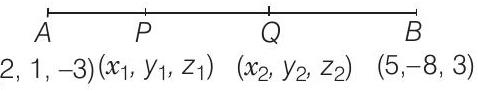
Since, the point
Since, the point
So, the coordinates of
16. If the origin is the centroid of a
Show Answer
Solution
Given that origin is the centroid of the
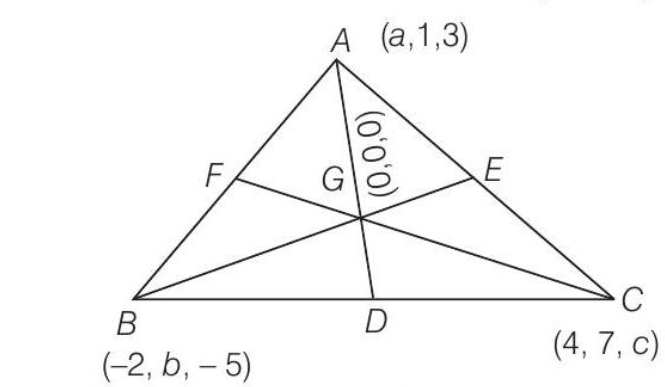
17. If
Show Answer
Solution
Let the coordinates of
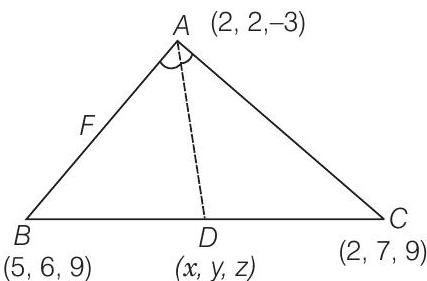
Since,
So, the coordinates of
Long Answer Type Questions
18. Show that the three points
Show Answer
Solution
Given points are
19. The mid-point of the sides of a triangle are
Show Answer
Solution
Let the vertices of

Since, the mid-point of side
Then,
Similarly, the mid-points of
and
Now,
From Eqs. (i) and (iv),
From Eqs. (ii) and (v),
From Eqs. (iii) and (vi),
From Eqs. (vii) and (x),
Then,
From Eqs. (viii) and (xi),
Then,
From Eqs. (ix) and (xii),
Then,
Then,
So, the vertices of the triangle
Hence, centroid of the triangle
20. Prove that the points
Show Answer
Thinking Process
First of all find the value of
Solution
Given points are
So, point
21. What are the coordinates of the vertices of a cube whose edge is 2 units, one of whose vertices coincides with the origin and the three edges passing through the origin, coincides with the positive direction of the axes through the origin?
Show Answer
Solution
The coordinates of the cube which edge is 2 units, are
Objective Type Questions
22. The distance of point
(a) 3 units
(b) 4 units
(c) 5 units
(d) 550
Show Answer
Solution
(a) Given, point is
Distance of
-
Option (b) 4 units: This is incorrect because the distance of a point from the YZ-plane is determined by the x-coordinate of the point. In this case, the x-coordinate is 3, not 4.
-
Option (c) 5 units: This is incorrect because the distance of a point from the YZ-plane is determined by the x-coordinate of the point. In this case, the x-coordinate is 3, not 5.
-
Option (d) 550: This is incorrect because the distance of a point from the YZ-plane is determined by the x-coordinate of the point. In this case, the x-coordinate is 3, and 550 is an unrelated and excessively large number.
23. What is the length of foot of perpendicular drawn from the point
(a)
(b)
(c) 5
(d) None of these
Show Answer
Solution
(b) We know that, on the
-
Option (a)
-
Option (c) 5 is incorrect because the distance from point
-
Option (d) None of these is incorrect because there is a correct option provided, which is (b)
24. Distance of the point
(a)
(b) 3
(c) 4
(d) 5
Show Answer
Solution
(a) Given, points
- Option (b) is incorrect because the distance calculated as 3 would imply that the sum of the squares of the differences in coordinates is 9, which is not the case here.
- Option (c) is incorrect because the distance calculated as 4 would imply that the sum of the squares of the differences in coordinates is 16, which is not the case here.
- Option (d) is incorrect because the distance calculated as 5 would imply that the sum of the squares of the differences in coordinates is 25, which is not the case here.
25. If the distance between the points
(a) 5
(b)
(c) -5
(d) None of these
Show Answer
Solution
(b) Given, the points are
-
Option (a) 5: This option is partially correct because it only considers the positive root of the equation (a^2 = 25). However, the equation has two solutions, (a = 5) and (a = -5).
-
Option (c) -5: This option is also partially correct because it only considers the negative root of the equation (a^2 = 25). The equation has two solutions, (a = 5) and (a = -5).
-
Option (d) None of these: This option is incorrect because the correct value of (a) is (\pm 5), which is provided in option (b).
26.
(a)
(b)
(c)
(d) None of these
Show Answer
Solution
(a) We know that, on the
- Option (b) is incorrect because the intersection of the planes ( YZ ) and ( ZX ) is the ( Z )-axis, not the ( X )-axis.
- Option (c) is incorrect because the intersection of the planes ( XY ) and ( YZ ) is the ( Y )-axis, not the ( X )-axis.
- Option (d) is incorrect because option (a) is correct, making “None of these” an invalid choice.
27. Equation of
(a)
(b)
(c)
(d) None of these
Show Answer
Solution
(c) On the Y-axis,
- Option (a)
- Option (b)
- Option (d) None of these: This option is incorrect because there is a correct option provided, which is (c)
28. The point
(a) first octant
(b) seventh octant
(c) second octant
(d) eight octant
Show Answer
Solution
(b) The point
- The point
- The point
- The point
29. A plane is parallel to
(a)
(b)
(c) Z-axis
(d) None of these
Show Answer
Solution
(a) A plane is parallel to YZ-plane, so it is perpendicular to
- (b) A plane parallel to the YZ-plane is not perpendicular to the Y-axis because it lies parallel to both the Y and Z axes.
- (c) A plane parallel to the YZ-plane is not perpendicular to the Z-axis because it lies parallel to both the Y and Z axes.
- (d) The option “None of these” is incorrect because the plane is indeed perpendicular to the X-axis.
30. The locus of a point for which
(a) equation of
(b) equation of
(c) equation at Z-axis
(d) None of these
Show Answer
Solution
(a) We know that, equation on the
- (b) The equation of the
- (c) The equation of the
- (d) The given conditions
31. The locus of a point for which
(a)
(b) YZ-plane
(c) ZX-plane
(d) None of these
Show Answer
Solution
(b) On the YZ-plane,
-
(a)
-
(c)
-
(d) None of these: This option is incorrect because there is a correct option, which is the
32. If a parallelopiped is formed by planes drawn through the points
(a)
(b)
(c)
(d)
Show Answer
Solution
(a) Given points of the parallelopiped are
-
Option (b)
-
Option (c)
-
Option (d)
33.
(a)
(b)
(c)
(d) None of these
Show Answer
Solution
(d) We know that, on the
- Option (a)
- Option (b)
- Option (c)
34.
(a)
(b)
(c)
(d) None of these
Show Answer
Solution
(a) On the X-axis,
Hence, the required coordinates are
- Option (b)
- Option (c)
- Option (d) None of these is incorrect because the correct coordinates
Fillers
35. The three axes
Show Answer
Solution
The three axes
36. The three planes determine a rectangular parallelopiped which has …… of rectangular faces.
Show Answer
Solution
Three points
37. The coordinates of a point are the perpendicular distance from the …… on the respectives axes.
Show Answer
Solution
Given points
38. The three coordinate planes divide the space into …… parts.
Show Answer
Solution
Eight parts
39. If a point
Show Answer
Solution
We know that, on YZ-plane,
40. The equation of
Show Answer
Solution
The equation of YZ-plane is
41. If the point
Show Answer
Solution
On the
42. The equation of
Show Answer
Solution
The equation of
43.
Show Answer
Solution
z-coordinates.
44.
Show Answer
Solution
45.
Show Answer
Solution
46. The plane parallel to
Show Answer
Solution
The plane parallel to
47. The length of the longest piece of a string that can be stretched straight in a rectangular room whose dimensions are 10,13 and 8 units are ……
Show Answer
Solution
Given dimensions are
48. If the distance between the points
Show Answer
Solution
Given points are
49. If the mid-points of the sides of a triangle
Show Answer
Solution
Let the vertices of

Since,
Similarly,
From Eqs. (i) and (iv),
From Eqs. (ii) and (v),
From Eqs. (iii) and (vi),
From Eqs. (vii) and (x),
If
If
From Eqs. (ix) and (xii),
So, the vertices of
i.e.,
50. Match each item given under the Column I to its correct answer given under Column II.
| Column I | Column II | ||
|---|---|---|---|
| (i) | In-XY-plane | (a) | Ist octant |
| (ii) | Point |
(b) | YZ-plane |
| (iii) | Locus of the points having 0 is |
(c) | |
| (iv) | A line is parallel to |
(d) | Z-axis |
| If represent the |
(e) | plane parallel to |
|
| (vi) | (f) | if all the points on the line have equal |
|
| (vii) | Planes |
(f) | from the point on the respective |
| (viii) | Coordinates of a point are the distances from the origin to the feet of perpendiculars |
(h) | parallel to Z-axis |
| (ix) | A ball is the solid region in the space enclosed by a |
(i) | disc |
| Region in the plane enclosed by a circle is known as a |
(j) | sphere |
Show Answer
Solution
(i) In XY-plane, z-coordinates is zero.
(ii) The point
(iii) Locus of the points having
(iv)
(v)
(vi)
(vii) The planes
(viii) Coordinates of a point are the distances from the origin to the feet of perpendicular from the point on the respective.
(ix) A ball is the solid region in the space enclosed by a sphere.
(x) The region in the plane enclosed by a circle is known as a disc.










