Chapter 02 Structure of Atom
Multiple Choice Questions (MCQs)
1. Which of the following conclusions could not be derived from Rutherford’s
(a) Most of the space in the atom is empty
(b) The radius of the atom is about
(c) Electrons move in a circular path of fixed energy called orbits
(d) Electrons and the nucleus are held together by electrostatic forces of attraction
Show Answer
Answer
(c) Concept of electrons move in a circular path of fixed energy called orbits was put forward by Bohr and not derived from Rutherford’s scattering experiment.
Out of a large number of circular orbits theoretically possible around the nucleus, the electron revolve only in those orbits which have a tired value of energy. Hence, these orbits are called energy level or stationary states.
-
(a) Most of the space in the atom is empty: This conclusion is correct and was derived from Rutherford’s experiment. The experiment showed that most
-
(b) The radius of the atom is about
-
(d) Electrons and the nucleus are held together by electrostatic forces of attraction: This conclusion is correct and was derived from Rutherford’s experiment. The deflection patterns of the
2. Which of the following options does not represent ground state electronic configuration of an atom?
(a)
(b)
(c)
(d)
Show Answer
Answer
(b) Correct configuration should be
-
Option (a): The configuration
-
Option (c): The configuration
-
Option (d): The configuration
3. The probability density plots of

1

2
The density of dots in a region represents the probability density of finding electrons in the region.
On the basis of above diagram which of the following statements is incorrect?
(a)
(b) The probability of finding the electron is maximum near the nucleus
(c) The probability of finding the electron at a given distance is equal in all directions
(d) The probability density of electrons for
Show Answer
Thinking Process
(i) To solve the problem, it keep in mind that s-orbital has spherical shape.
(ii) Probability density represents the probability of finding an electron at a point within an atom is proportional to the square of the orbital wave function i.e.,
Answer
(d) The probability density of electrons in
-
Option (a): This option is correct. Both
-
Option (b): This option is incorrect. For the
-
Option (c): This option is correct. The probability of finding the electron at a given distance is indeed equal in all directions for both
4. Which of the following statement is not correct about the characteristics of cathode rays?
(a) They start from the cathode and move towards the anode
(b) They travel in straight line in the absence of an external electrical or magnetic field
(c) Characteristics of cathode rays do not depend upon the material of electrodes in cathode ray tube
(d) Characteristics of cathode rays depend upon the nature of gas present in the cathode ray tube
Show Answer
Answer
(d) Cathode rays consist of negatively charged material particles called electrons. It was discovered by William Crookes. The characteristics of cathode rays do not depend upon the material of electrodes and the nature of the gas present in the cathode ray tube.
-
Option (a): This statement is correct. Cathode rays do indeed start from the cathode and move towards the anode in a cathode ray tube.
-
Option (b): This statement is correct. Cathode rays travel in straight lines when there is no external electrical or magnetic field influencing their path.
-
Option (c): This statement is correct. The characteristics of cathode rays are independent of the material of the electrodes in the cathode ray tube.
5. Which of the following statements about the electron is incorrect?
(a) It is a negatively charged particle
(b) The mass of electron is equal to the mass of neutron
(c) It is a basic constituent of all atoms
(d) It is a constituent of cathode rays
Show Answer
Answer
(b) The mass of electron is very small as compared to the mass of the neutron.
Mass of electron
Mass of neutron
-
(a) It is a negatively charged particle: This statement is correct. Electrons are indeed negatively charged particles.
-
(c) It is a basic constituent of all atoms: This statement is correct. Electrons are fundamental components of all atoms, orbiting the nucleus.
-
(d) It is a constituent of cathode rays: This statement is correct. Cathode rays are streams of electrons observed in vacuum tubes.
6. Which of the following properties of atom could be explained correctly by Thomson model of atom?
(a) Overall neutrality of atom
(b) Spectra of hydrogen atom
(c) Position of electrons, protons and neutrons in atom
(d) Stability of atom
Show Answer
Answer
(a)
-
(b) Spectra of hydrogen atom: Thomson’s model could not explain the discrete spectral lines observed in the hydrogen atom. The model did not account for the quantized energy levels of electrons, which are necessary to explain the emission and absorption spectra.
-
(c) Position of electrons, protons and neutrons in atom: Thomson’s model did not provide a clear picture of the positions of electrons, protons, and neutrons within the atom. It only suggested that electrons were embedded in a positively charged ‘pudding,’ without detailing the specific arrangement or positions of these subatomic particles.
-
(d) Stability of atom: Thomson’s model could not explain the stability of the atom. It did not address why electrons, which are negatively charged, do not spiral into the positively charged ‘pudding’ due to electrostatic attraction, leading to the collapse of the atom.
7. Two atoms are said to be isobars if
(a) they have same atomic number but different mass number
(b) they have same number of electrons but different number of neutrons
(c) they have same number of neutrons but different number of electrons
(d) sum of the number of protons and neutrons is same but the number of protons is different
Show Answer
Answer
(d) Isobars have the same mass number (i.e., sum of protons and neutrons) but different atomic number (i.e., number of protons). e.g.,
| Atomic number |
Atomic number |
| Mass number |
Mass number |
-
(a) they have same atomic number but different mass number: This describes isotopes, not isobars. Isotopes have the same number of protons (atomic number) but different numbers of neutrons, leading to different mass numbers.
-
(b) they have same number of electrons but different number of neutrons: This describes ions or neutral atoms of different elements, not isobars. The number of electrons can vary due to ionization, but this does not define isobars.
-
(c) they have same number of neutrons but different number of electrons: This describes neither isobars nor any specific category of atoms. The number of neutrons being the same with different numbers of electrons does not fit the definition of isobars.
8. The number of radial nodes for
(a) 3
(b) 4
(c) 2
(d) 1
Show Answer
Answer
(d) For a hydrogen atom wave function, there are
Number of radial nodes
-
Option (a) is incorrect because the number of radial nodes for a
-
Option (b) is incorrect because the number of radial nodes for a
-
Option (c) is incorrect because the number of radial nodes for a
9. Number of angular nodes for
(a) 4
(b) 3
(c) 2
(d) 1
Show Answer
Answer
(c) Number of angular nodes
For
- Option (a) is incorrect because the number of angular nodes is given by the azimuthal quantum number ( l ). For a ( d )-orbital, ( l = 2 ), not 4.
- Option (b) is incorrect because the number of angular nodes for a ( d )-orbital is determined by ( l ), which is 2, not 3.
- Option (d) is incorrect because the number of angular nodes for a ( d )-orbital is given by ( l = 2 ), not 1.
10. Which of the following is responsible to rule out the existence of definite paths or trajectories of electrons?
(a) Pauli’s exclusion principle
(b) Heisenberg’s uncertainty principle
(c) Hund’s rule of maximum multiplicity
(d) Aufbau principle
Show Answer
Answer
(b) Werner Heisenberg, a German physicist in 1927, stated uncertainty principle which states that it is impossible to determine simultaneously, the exact position and exact momentum of an electron.
Mathematically,
The important implications of the Heisenberg uncertainty principle is that it rules out existence of definite paths or trajectories of electrons and other similar particles.
-
Pauli’s exclusion principle: This principle states that no two electrons in an atom can have the same set of four quantum numbers. It is related to the arrangement of electrons in an atom and their unique quantum states, but it does not address the concept of definite paths or trajectories of electrons.
-
Hund’s rule of maximum multiplicity: This rule states that electrons will fill degenerate orbitals (orbitals with the same energy) singly as far as possible before pairing up. It is concerned with the distribution of electrons among orbitals to maximize total spin, but it does not address the existence of definite paths or trajectories of electrons.
-
Aufbau principle: This principle states that electrons fill atomic orbitals of the lowest available energy levels before occupying higher levels. It is a guideline for the order in which electrons are added to orbitals, but it does not address the concept of definite paths or trajectories of electrons.
11. Total number of orbitals associated with third shell will be
(a) 2
(b) 4
(c) 9
(d) 3
Show Answer
Answer
(c) Total number of orbitals associated with
-
Option (a) is incorrect because the total number of orbitals associated with the third shell is not 2. The formula for the total number of orbitals in the
-
Option (b) is incorrect because the total number of orbitals associated with the third shell is not 4. Using the formula (n^2), for (n = 3), the total number of orbitals is
-
Option (d) is incorrect because the total number of orbitals associated with the third shell is not 3. According to the formula (n^2), for (n = 3), the total number of orbitals is
12. Orbital angular momentum depends on
(a)
(b)
(c)
(d)
Show Answer
Answer
(a) Orbital angular momentum
(a) When
(b) When
(c) When
(d) When
-
Option (b) is incorrect because the principal quantum number ( n ) does not directly affect the orbital angular momentum. Orbital angular momentum depends solely on the azimuthal quantum number ( l ).
-
Option (c) is incorrect because the magnetic quantum number ( m ) does not affect the magnitude of the orbital angular momentum. It only determines the orientation of the orbital angular momentum in space.
-
Option (d) is incorrect because the spin quantum number ( s ) and the magnetic quantum number ( m ) do not influence the orbital angular momentum. The spin quantum number ( s ) pertains to the intrinsic spin of the electron, not its orbital motion.
13. Chlorine exists in two isotopic forms.
(a)
(b)
(c)
(d)
Show Answer
Answer
(c) The fractional atomic mass ( 35.5 ) of chlorine is due to the fact that in ordinary chlorine atom,
-
Option (a)
This value is not equal to the given atomic mass of 35.5 amu. -
Option (b)
This value is not equal to the given atomic mass of 35.5 amu. -
Option (d)
This value is not equal to the given atomic mass of 35.5 amu.
14. The pair of ions having same electronic configuration is
(a)
(b)
(c)
(d)
Show Answer
Answer
(b)
Thus,
-
(a)
-
(c)
-
(d)
15. For the electrons of oxygen atom, which of the following statements is correct?
(a)
(b) An electron in the
(c)
(d) The two electrons present in the
Show Answer
Answer
(d)
(a) Electrons in
(b) Energy of
(c)
(d) For the two electrons of
Hence, it is correct.
-
(a) Electrons in
-
(b) Energy of
-
(c)
16. If travelling at same speeds, which of the following matter waves have the shortest wavelength?
(a) Electron
(b) Alpha particle
(c) Neutron
(d) Proton
Show Answer
Answer
(b) From de-Broglie equation,
For same speed of different particles, i.e., electron, proton, neutron and
As
-
Electron: Electrons have a much smaller mass compared to alpha particles. According to the de-Broglie equation, a smaller mass results in a longer wavelength for the same speed.
-
Neutron: Neutrons have a greater mass than electrons but still have a smaller mass compared to alpha particles. Therefore, their wavelength will be longer than that of alpha particles for the same speed.
-
Proton: Protons have a mass that is greater than electrons but less than alpha particles. Consequently, their wavelength will be longer than that of alpha particles for the same speed.
Multiple Choice Questions (More Than One Options)
17. Identify the pairs which are not of isotopes?
(a)
(b)
(c)
(d)
Show Answer
Thinking Process
(i) Isotopes are the elements that have same atomic number
(ii) The digit written as subscript represents the atomic number
Answer
(c,
(a)
(b)
(c)
(d)
Both these pairs are isobars to each other.
- (a)
- (b)
18. Out of the following pairs of electrons, identify the pairs of electrons present in degenerate orbitals.
(a) (i)
(b) (i)
(c) (i)
(d) (i)
Show Answer
Answer
Degenerate orbitals means the orbitals of the same subshell of the same main shell, i.e., their
(a) (i)
(b) (i)
(c) (i)
(d) (i)
Thus,
-
(b): The electrons are in different subshells. One electron is in the 3p subshell (
-
(c): The electrons are in different principal quantum levels. One electron is in the 4s subshell (
19. Which of the following sets of quantum numbers are correct?
| (a) 1 | 1 | +2 | (b) 2 | 1 | +1 |
| (c) 3 | 2 | -2 | (d) 3 | 4 | -2 |
Show Answer
Answer
(
| If |
|
|---|---|
| Hence, (a) is incorrect. | |
| If |
|
| Hence, (b) is correct. | |
| If |
|
| For |
|
| Hence, (c) is correct. | |
| If |
|
| Hence, (d) is incorrect. |
-
For option (a): If ( n = 1 ), ( l \neq 1 ) because ( l ) must be less than ( n ). Therefore, ( l ) can only be 0 when ( n = 1 ). Hence, (a) is incorrect.
-
For option (d): If ( n = 3 ), ( l ) cannot be 4 because ( l ) must be less than ( n ). Therefore, ( l ) can only be 0, 1, or 2 when ( n = 3 ). Hence, (d) is incorrect.
20. In which of the following pairs, the ions are isoelectronic?
(a)
(b)
(c)
(d)
Show Answer
Thinking Process
To solve this problem, it should kept in mind that isoelectronic are those species which have same number of electrons.
Answer
For,
Thus, they have same number of electrons.
For,
They do not have same number of electrons.
For,
They have same number of electrons.
They do not have same number of electrons.
Thus,
-
For option (b):
- They do not have the same number of electrons.
-
For option (d):
- They do not have the same number of electrons.
21. Which of the following statements concerning the quantum numbers are correct?
(a) Angular quantum number determines the three dimensional shape of the orbital
(b) The principal quantum number determines the orientation and energy of the orbital
(c) Magnetic quantum number determines the size of the orbital
(d) Spin quantum number of an electron determines the orientation of the spin of electron relative to the chosen axis
Show Answer
Answer
(a) Azimuthal quantum number ’
(b) The principal quantum number determines the size of the orbit.
(c) Magnetic quantum number determines the orientation of the electron cloud in a subshell.
(d) An electron spins around its own axis, much in a similar way as earth spins around its own axis while revolving around the sun. In other words, an electron has, besides charge and mass, intrinsic spin angular quantum number.
- (b) The principal quantum number determines the size of the orbit, not the orientation and energy of the orbital.
- (c) Magnetic quantum number determines the orientation of the electron cloud in a subshell, not the size of the orbital.
Short Answer Type Questions
22. Arrange
Show Answer
Answer
s-orbital is spherical in shape, it shields the electrons from the nucleus more effectively than
Therefore, the effective nuclear charge
Show Answer
Answer

From the orbital diagram, it is seen that there are two unpaired electrons.
Show Answer
Answer
Show Answer
Answer
The orbitals which belongs to same subshell and same shell are called degenerate orbitals.
Show Answer
Thinking Process
To calculate the total number and radial nodes of principal quantum number
(i) Radial nodes (or spherical nodes)
(ii) Angular nodes (or non-spherical nodes)
(iii) Total nodes
Answer
For
Number of angular nodes
Number of radial nodes
I. Based upon the above information, arrange the following orbitals in the increasing order of energy.
(a)
(b)
(c)
(d)
II. Based upon the above information. Solve the questions given below.
(a) Which of the following orbitals has the lowest energy?
(b) Which of the following orbitals has the highest energy?
Show Answer
Answer
I.(a)
Hence, increasing order of their energy is
(b)
Hence,
(c)
Hence,
(d)
Hence,
II. (a)
Hence,
(b)
Hence,
Proton, cathode rays, electron, neutron.
Show Answer
Answer
Neutron being neutral will not show deflection from the path on passing through an electric field.
Proton, cathode rays and electron being the charged particle will show deflection from the path on passing through an electric field.
Show Answer
Thinking Process
(i) Atomic mass
(ii) Number of proton is equal to the atomic number of the atom.
Answer
An atom having atomic mass number 13 and number of neutrons 7 .
(B)
(D)
Arrange these radiations in the increasing order of their energies.
Show Answer
Answer
Increasing order of energy is
Show Answer
Answer
Configurations either exactly half-filled or fully filled orbitals are more stable due to symmetrical distribution of electrons and maximum exchange energy. In
Show Answer
Answer
From Rydberg formula,
Wave number,
Show Answer
Answer
Given,
From de-Broglie equation, wavelength,
As the wavelength is very small so wave nature cannot be detected.
Show Answer
Answer
The line spectrum of any element has lines corresponding to definite wavelengths. Lines are obtained as a result of electronic transitions between the energy levels. Hence, the electrons in these levels have fixed energy, i.e., quantized values.
Show Answer
Answer
From de-Broglie equation, wavelength,
For same wavelength for two different particles, i.e., electron and proton,
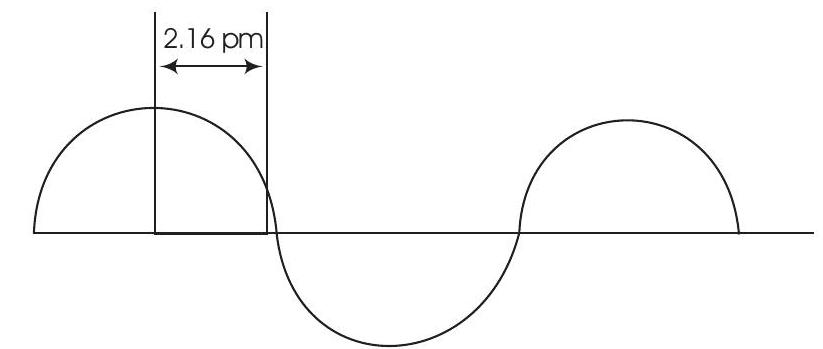
Show Answer
Answer
Wavelength is the distance between two successive peaks or two successive throughs of a wave.
Therefore,
Which part of the electromagnetic spectrum does it belong to?
Show Answer
Answer
Thus, it belongs to visible region.
Show Answer
Answer
Difference between the terms orbit and orbital are as below
| Orbit | Orbital |
|---|---|
| An orbit is a well defined circular path around the nucleus in which the electrons revolve. |
An orbital is the three dimensional space around the nucleus within which the probability of finding an electron is maximum (upto 90%). All orbits are circular and disc like. |
| The concept of an orbit is not in accordance orbitals have different shapes. with the wave character of electrons with |
The concept of an orbital is in accordance with the wave character of electrons and uncertainty principle. |
| The maximum number of electrons in any orbit is given by of the orbit. |
The maximum number of electrons present in any orbital is two. |
Show Answer
Answer
Given that, speed
mass
Uncertainty in speed
From Heisenberg uncertainty principle,
Uncertainty in position,
Show Answer
Answer
If uncertainty principle is applied to an object of mass, say about a milligram
The value of
Show Answer
Answer
In hydrogen atom, the energy of an electron is determined by the value of
Matching The Columns
42. Match the following species with their corresponding ground state electronic configuration.
| Atom / Ion | Electronic configuration | ||
|---|---|---|---|
| A. | 1. | ||
| B. | 2. | ||
| C. | 3. | ||
| D. | 4. | ||
| 5. |
Show Answer
Answer
A.
B.
C.
D.
A.
B.
C.
D.
| Quantum number | Information provided | ||
|---|---|---|---|
| A. | Principal quantum number | 1. | Orientation of the orbital |
| B. | Azimuthal quantum number | 2. | Energy and size of orbital |
| C. | Magnetic quantum number | 3. | Spin of electron |
| D. | Spin quantum number | 4. | Shape of the orbital |
Show Answer
Answer
A.
B.
C.
D.
A. Principal quantum number is the most important quantum number as it determines the size and to large extent the energy of the orbital.
B. Azimuthal quantum number determines the angular momentum of the electron and defines the three-dimensional shape of the orbital.
C. Magnetic quantum number gives information about the spatial orientation of orbitals with respect to a standard set of coordinate axes.
D. Spin quantum number arises from the spectral evidence that an electron in its motion around the nucleus in an orbit also rotates or spin about its own axis.
| Rules | Statements | |
|---|---|---|
| A. | Hund’s Rule | 1. No two electrons in an atom can have the same set of four quantum numbers. |
| B. | Aufbau Principle | 2. Half-filled and completely filled orbitals have extra stability. |
| C. | Pauli Exclusion Principle | 3. Pairing of electrons in the orbitals belonging to the same subshell does not take place until each orbital is singly occupied. |
| D. | Heisenberg’s Uncertainty Principle |
4. It is impossible to determine the exact position and exact momentum of a subatomic particle simultaneously. 5. In the ground state of atoms, orbitals are filled in the order of their increasing energies. |
Show Answer
Answer
A.
B.
C.
D.
A. Hund’s rule states that pairing of electrons in the orbitals belonging to the same subshell (
B. Aufbau principle states that in the ground state of the atoms, the orbitals are filled in order of their increasing energies.
C. According to Pauli exclusion principle, no two electrons in an atom can have the same set of four quantum numbers.
D. Heisenberg’s uncertainty principle states that it is impossible to determine the exact position and exact momentum of a subatomic particle simultaneously.
| Column I | Column II | ||
|---|---|---|---|
| A. | X-rays | 1. | |
| B. | Ultraviolet wave (UV) | 2. | |
| C. | Long radio waves | 3. | |
| D. | Microwave | 4. |
Show Answer
Answer
A.
B.
C.
D.
| Name | Frequency | Uses | |
|---|---|---|---|
| A. | X-rays | Medical pictures, material testing | |
| B. | Ultraviolet wave |
Germisidal lamp | |
| C. | Long radio waves | Signal transmission | |
| D. | Microwave | Cooking radar |
| Column I | Column II | ||
|---|---|---|---|
| A. | Photon | 1. | Value is 4 for |
| B. | Electron | 2. | Probability density |
| C. | 3. | Always positive value | |
| D. | Principal quantum number |
4. | Exhibits both momentum and wavelength |
Show Answer
Answer
A.
B.
C.
D.
A. Photon has particle nature as well as wave nature. It exhibits both momentum and wavelength.
B. Electron also has particle nature as well as wave nature. Thus, it also exhibits both momentum and wavelength.
C.
D. Principal quantum number
It always has positive values.
| Column I | Column II | ||
|---|---|---|---|
| A. | 1. | ||
| B. | 2. | ||
| C. | 3. | ||
| D. | 4. | ||
| 5. |
Show Answer
Answer
A.
B.
C.
D.
A.
B.
C.
D.
In the following questions a statement of Assertion (A) followed by a statement of Reason
(A) All isotopes of a given element show the same type of chemical behaviour.
Reason (R) The chemical properties of an atom are controlled by the number of electrons in the atom.
(a) Both
(b) Both
(c)
(d) Both
Show Answer
Answer
(a) Both assertion and reason are true and reason is the correct explanation of assertion. Isotopes have the same atomic number i.e., same number of electrons which are responsible for their chemical behaviour. Hence, these exhibit similar chemical properties.
Reason (R) The frequency of radiation emitted by a body goes from a lower frequency to higher frequency with an increase in temperature.
(a) Both
(b) Both
(c)
(d) Both
Show Answer
Answer
(c) Assertion is true and reason is false.
A body which absorbs and emits all radiations falling on it is called perfect black body. With rise in temperature, frequency increases.
Reason (R) The path of an electron in an atom is clearly defined.
(a) Both
(b) Both
(c)
(d) Both
Show Answer
Answer
(c) Assertion is true and reason is false.
According to Heisenberg’s uncertainty principle, the exact position and exact momentum of an electron cannot be determined simultaneously. Thus, the path of electron in an atom is not clearly defined.
Long Answer Type Questions
51. What is photoelectric effect? State the result of photoelectric effect experiment that could not be explained on the basis of laws of classical physics. Explain this effect on the basis of quantum theory of electromagnetic radiations.
Show Answer
Answer
Photoelectric effect When radiation with certain minimum frequency

Equipment for studying the photoelectric effect. Light of a particular frequency strikes a clean metal surface inside a vacuum chamber.
Electrons are ejected from the metal and are counted by a detector that measures their kinetic energy.
The result observed in this experiment were
(i) The electrons are ejected from the metal surface as soon as the beam of light strikes the surface, i.e., there is no time lag between the striking of light beam and the ejection of electrons from the metal surface.
(ii) The number of electrons ejected is proportional to the intensity or brightness of light.
(iii) For each metal, there is a characteristic minimum frequency,
The above observation cannot be explained by the electromagnetic wave theory. According to this theory, since radiations were continuous, therefore, it should be possible to accumulate energy on the surface of the metal, irrespective of its frequency and thus, radiations of all frequencies should be able to eject electrons.
Similarly, according to this theory, the energy of the electrons ejected should depend upon the intensity of the incident radiation.
Particle Nature of Electromagnetic Radiation
To explain the phenomena of ‘black body radiation’ and ‘photoelectric effect’, Max Planck in 1900 , put forward a theory known after his name as Planck’s quantum theory. This theory was further extended by Einstein in 1905.
The important points of this theory are as follows
(i) The radiant energy is emitted or absorbed not continuously but discontinuously in the form of small discrete packets of energy. Each such packet of energy is called a ‘quantum’. In case of light, the quantum of energy is called a ‘photon’.
(ii) The energy of each quantum is directly proportional to the frequency of the radiation, i.e.,
where,
(iii) The total amount of energy emitted or absorbed by a body will be some whole number quanta.
Hence,
Note The energy possessed by one mole of quanta (or photons), i.e., Avogadro’s number
1 Einstein of energy
Show that an electron will not be emitted if a photon with a wavelength equal to
Show Answer
Answer
We know that,
Thus,
What points of Bohr’s model of an atom can be used to arrive at this formula? Based on these points derive the above formula giving description of each step and each term.
Show Answer
Answer
The two important points of Bohr’s model that can be used to derive the given formula are as follows
(i) Electrons revolve around the nucleus in a circular path of fixed radius and energy. These paths are called orbits. stationarv states or allowed enerav states
(ii) Energy is emitted or absorbed when an electron moves from higher stationary state to lower stationary state or from lower stationary state to higher stationary state respectively.
Derivation The energy of the electron in the
where,
The energy gap between the two orbits is given by the equation,
On combining Eqs. (i) and (iii)
Where,
Frequency,
Show Answer
Answer
In hydrogen spectrum, the spectral lines are expressed in term of wave number
Note When an electron returns from
Show Answer
Answer
In Bohr model, an electron is regarded as a charged particle moving in well defined circular orbits about the nucleus. An orbit can completely be defined only if both the position and the velocity of the electron are known exactly at the same time.
This is not possible according to the Heisenberg uncertainty principle. Further more, the wave character of the electron is not considered in Bohr model.
Therefore, concept of movement of an electron in an orbit was replaced by the concept of probabitlity of finding electron in an orbital due to de-Broglie concept of dual nature of electron and Heisenberg’s uncertainty principle. The changed model is called quantum mechanical model of the atom.










