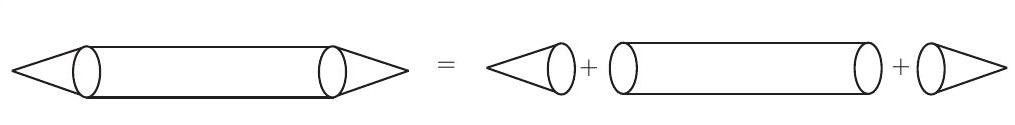Multiple Choice Questions (MCQs)
1 A cylindrical pencil sharpened at one edge is the combination of
(a) a cone and a cylinder
(b) frustum of a cone and a cylinder
(c) a hemisphere and a cylinder
(d) two cylinders
Show Answer
Solution
(a) Because the shape of sharpened pencil is

2 A surahi is the combination of
(a) a sphere and a cylinder
(b) a hemisphere and a cylinder
(c) two hemispheres
(d) a cylinder and a cone
Show Answer
Solution
(a) Because the shape of surahi is

3 A plumbline (sahul) is the combination of (see figure)
(a) a cone and a cylinder
(b) a hemisphere and a cone
(c) frustum of a cone and a cylinder
(d) sphere and cylinder
Show Answer
Solution
(b)

4 The shape of a glass (tumbler) (see figure) is usually in the form of
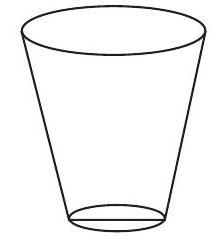
(a) a cone
(b) frustum of a cone
(c) a cylinder
(d) a sphere
Show Answer
Solution
(b) We know that, the shape of frustum of a cone is

So, the given figure is usually in the form of frustum of a cone.
5 The shape of a gilli, in the gilli-danda game (see figure) is a combination of

(a) two cylinders
(b) a cone and a cylinder
(c) two cones and a cylinder
(d) two cylinders and a cone
Show Answer
Solution
(c)
6 A shuttle cock used for playing badminton has the shape of the combination of
(a) a cylinder and a sphere
(b) a cylinder and a hemisphere
(c) a sphere and a cone
(d) frustum of a cone and a hemisphere
Show Answer
Solution
(d) Because the shape of the shuttle cock is equal to sum of frustum of a cone and hemisphere.
7 A cone is cut through a plane parallel to its base and then the cone that is formed on one side of that plane is removed. The new part that is left over on the other side of the plane is called
(a) a frustum of a cone
(b) cone
(c) cylinder
(d) sphere
Show Answer
Solution
(a)
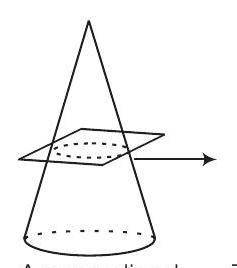
A cone sliced by a plane parallel to base
The two parts separated
Frustum of a cone
[when we remove the upper portion of the cone cut off by plane, we get frustum of a cone]
8 If a hollow cube of internal edge is filled with spherical marbles of diameter and it is assumed that space of the cube remains unfilled. Then, the number of marbles that the cube can accomodate is
(a) 142244
(b) 142344
(c) 142444
(d) 142544
Show Answer
Thinking Process
If we divide the total volume filled by marbles in a cube by volume of a marble, then we get the required number of marbles.
Solution
(a) Given, edge of the cube
Volume of the cube volume of cube
Also, given diameter of marble
Radius of a marble, diameter radius
Volume of one marble
Filled space of cube Volume of the cube Volume of cube
Required number of marbles
Hence, the number of marbles that the cube can accomodate is 142244 .
9 A metallic spherical shell of internal and external diameters and , respectively is melted and recast into the form a cone of base diameter . The height of the cone is
(a)
(b)
(c)
(d)
Show Answer
Thinking Process
When a solid shape is melted and recast into the form other solid shape, then volume of both shapes are equal.
Solution
(b) Given, internal diameter of spherical shell and external diameter of shell
Internal radius of spherical shell, diameter xradius and external radius of shell, diameter xradius
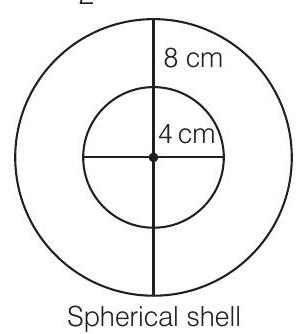
Now, volume of the spherical shell
volume of the spherical shell
Let height of the cone
Diameter of the base of cone
Radius of the base of cone diameter xradius
According to the question,
Volume of cone Volume of spherical shell
volume of cone height
Hence, the height of the cone is .
10 If a solid piece of iron in the form of a cuboid of dimensions , is moulded to form a solid sphere. Then, radius of the sphere is
(a)
(b)
(c)
(d)
Show Answer
Solution
(a) Given, dimensions of the cuboid
Volume of the cuboid
volume of cuboid length breadth height
Let the radius of the sphere is , then
Volume of the sphere voulme of the sphere
According to the question,
Volume of the sphere Volume of the cuboid
Hence, the radius of the sphere is .
11 A mason constructs a wall of dimensions with the bricks each of size and it is assumed that space is covered by the mortar. Then, the number of bricks used to construct the wall is
(a) 11100
(b) 11200
(c) 11000
(d) 11300
Show Answer
Thinking Process
If we divide the volume of the wall except the volume of mortar are used on wall by the volume of one brick, then we get the required number of bricks used to construct the wall.
Solution
(b) Volume of the wall
volume of cuboid length breadth height
Since, space of wall is covered by mortar.
So, remaining space of wall Volume of wall - Volume of mortar
Now, volume of one brick
volume of cuboid length breadth height
Required number of bricks (approx)
Hence, the number of bricks used to construct the wall is 11200 .
12 Twelve solid spheres of the same size are made by melting a solid metallic cylinder of base diameter and height . The diameter of each sphere is
(a)
(b)
(c)
(d)
Show Answer
Solution
(c) Given, diameter of the cylinder
Radius and height of the cylinder
Volume of the cylinder
volume of cylinder height
Now, let the radius of solid sphere
Then, its volume volume of sphere
According to the question,
Volume of the twelve solid sphere Volume of cylinder
Diameter of each sphere,
Hence, the required diameter of each sphere is .
13 The radii of the top and bottom of a bucket of slant height are and , respectively. The curved surface area of the bucket is
(a)
(b)
(c)
(d)
Show Answer
Solution
(a) Given, the radius of the top of the bucket, and the radius of the bottom of the bucket,
Slant height of the bucket,
Since, bucket is in the form of frustum of a cone.
Curved surface area of the bucket
14 A medicine-capsule is in the shape of a cylinder of diameter with two hemispheres stuck to each of its ends. The length of entire capsule is . The capacity of the capsule is
(a)
(b)
(c)
(d)
Show Answer
Solution
(a) Given, diameter of cylinder Diameter of hemisphere
[since, both hemispheres are attach with cylinder]
Radius of cylinder radius of hemisphere
diameter xradius
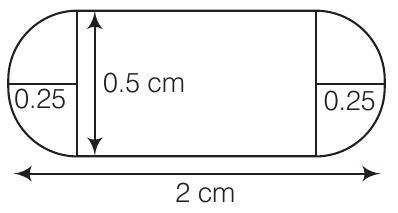
and total length of capsule
Length of cylindrical part of capsule,
Now, capacity of capsule Volume of cylindrical part Volume of hemisphere
volume of cylinder height and volume of hemispere
Hence, the capacity of capsule is .
15 If two solid hemispheres of same base radius are joined together along their bases, then curved surface area of this new solid is
(a)
(b)
(c)
(d)
Show Answer
Solution
(a) Because curved surface area of a hemisphere is and here, we join two solid hemispheres along their bases of radius , from which we get a solid sphere. Hence, the curved surface area of new solid
16 A right circular cylinder of radius and height (where, ) just encloses a sphere of diameter
(a)
(b)
(c)
(d)
Show Answer
Solution
(b) Because the sphere encloses in the cylinder, therefore the diameter of sphere is equal to diameter of cylinder which is .
17 During conversion of a solid from one shape to another, the volume of the new shape will
(a) increase
(b) decrease
(c) remain unaltered
(d) be doubled
Show Answer
Solution
(c) During conversion of a solid from one shape to another, the volume of the new shape will remain unaltered.
18 The diameters of the two circular ends of the bucket are and . The height of the bucket is . The capacity of the bucket is
(a)
(b)
(c)
(d)
Show Answer
Solution
(a) Given, diameter of one end of the bucket,
and diameter of the other end,
Height of the bucket,
Since, the shape of bucket is look like as frustum of a cone.
Capacity of the bucket Volume of the frustum of the cone
Hence, the capacity of bucket is .
19 In a right circular cone, the cross-section made by a plane parallel to the base is a
(a) circle
(b) frustum of a cone
(c) sphere
(d) hemisphere
Show Answer
Solution
(b) We know that, if a cone is cut by a plane parallel to the base of the cone, then the portion between the plane and base is called the frustum of the cone.
20 If volumes of two spheres are in the ratio , then the ratio of their surface areas is
(a)
(b)
(c)
(d)
Show Answer
Solution
(d) Let the radii of the two spheres are and , respectively.
Volume of the sphere of radius,
and volume of the sphere of radius,
Given, ratio of volumes [using Eqs. (i) and (ii)]
Now, ratio of surface area surface area of a sphere
[using Eq. (iii)]
Hence, the required ratio of their surface area is .
Very Short Answer Type Questions
Write whether True or False and justify your answer.
1 Two identical solid hemispheres of equal base radius are stuck together along their bases. The total surface area of the combination is .
Show Answer
Solution
False
Curved surface area of a hemisphere
Here, two identical soild hemispheres of equal radius are stuck together. So, base of both hemispheres is common.
Total surface area of the combination
2 A solid cylinder of radius and height is placed over other cylinder of same height and radius. The total surface area of the shape so formed is .
Show Answer
Solution
False
Since, the total surface area of cylinder of radius, and height,
When one cylinder is placed over the other cylinder of same height and radius, then height of the new cylinder
and radius of the new cylinder
Total surface area of the new cylinder
3 A solid cone of radius and height is placed over a solid cylinder having same base radius and height as that of a cone The total surface area of the combined solid is .
Show Answer
Solution
False
We know that, total surface area of a cone of radius,
and
height, Curved surface Area + area of base
where,
and total surface area of a cylinder of base radius, and height,
Here, when we placed a cone over a cylinder, then one base is common for both.
So, total surface area of the combined solid
4 A solid ball is exactly fitted inside the cubical box of side a. The volume of the ball is .
Show Answer
Solution
False
Because solid ball is exactly fitted inside the cubical box of side . So, is the diameter for the solid ball.
5 The volume of the frustum of a cone is , where is vertical height of the frustum and are the radii of the ends.
Show Answer
Solution
False
Since, the volume of the frustum of a cone is , where is vertical height of the frustum and are the radii of the ends.
6 The capacity of a cylindrical vessel with a hemispherical portion raised upward at the bottom as shown in the figure is .
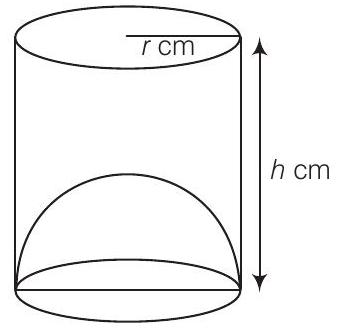
Show Answer
Solution
True
We know that, capacity of cylindrical vessel
and capacity of hemisphere
From the figure, capacity of the cylindrical vessel
7 The curved surface area of a frustum of a cone is , where and are the radii of the two ends of the frustum and is the vertical height.
Show Answer
Solution
False
We know that, if and are the radii of the two ends of the frustum and is the vertical height, then curved surface area of a frustum is , where .
8 An open metallic bucket is in the shape of a frustum of a cone, mounted on a hollow cylindrical base made of the same metallic sheet. The surface area of the metallic sheet used is equal to curved surface area of frustum of a cone + area of circular base + curved surface area of cylinder.
Show Answer
Solution
True
Because the resulting figure is
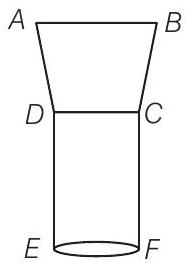
Here, is a frustum of a cone and is a hollow cylinder.
Short Answer Type Questions
1 Three metallic solid cubes whose edges are and are melted and formed into a single cube. Find the edge of the cube so formed.
Show Answer
Solution
Given, edges of three solid cubes are and , respectively.
Volume of first cube
Volume of second cube
and
volume of third cube
Sum of volume of three cubes
Let the edge of the resulting cube
Then, volume of the resulting cube,
2 How many shots each having diameter can be made from a cuboidal lead solid of dimensions ?
Show Answer
Solution
Given, dimensions of cuboidal
Volume of cuboidal
and diameter of shot
3 A bucket is in the form of a frustum of a cone and holds of water. The radii of the top and bottom are and , respectively. Find the height of the bucket.
Show Answer
Solution
Given, volume of the frustum
and radius of the top
radius of the bottom
Let height of the bucket
Now, volume of the bucket
[given]
4. cone of radius and height is divided into two parts by a plane through the mid-point of its axis parallel to its base. Find the ratio of the volumes of two parts.
Show Answer
Solution
Let ORN be the cone then given, radius of the base of the cone .
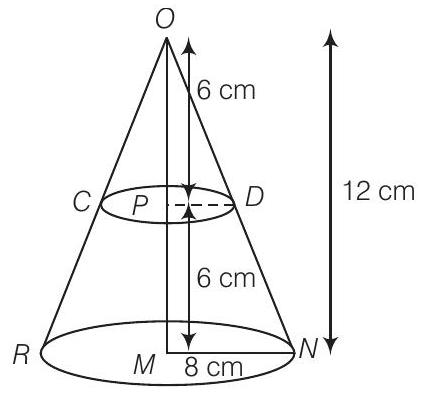
and height of the cone, (h)
Let be the mid-point of , then
The plane along divides the cone into two parts, namely
(i) a smaller cone of radius and height and (ii) frustum of a cone for which
Radius of the top of the frustum,
Radius of the bottom,
Required ratio Volume of frustum : Volume of cone
5 Two identical cubes each of volume are joined together end to end. What is the surface area of the resulting cuboid?
Show Answer
Solution
Let the length of a side of a cube
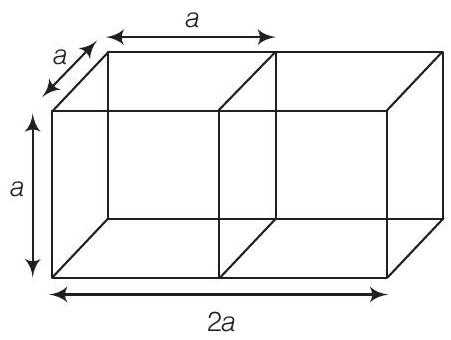
Given, volume of the cube,
On joining two cubes, we get a cuboid whose
6 From a solid cube of side , a conical cavity of height and radius is hollowed out. Find the volume of the remaining solid.
Show Answer
Solution
Given that, side of a solid cube
Height of conical cavity i.e., cone,
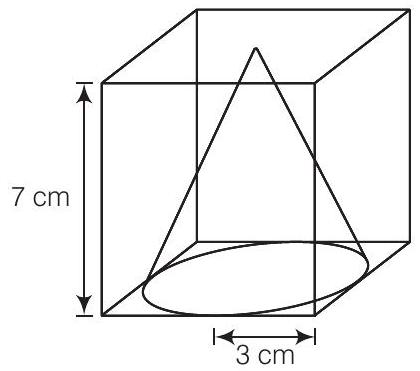
Since, the height of conical cavity and the side of cube is equal that means the conical cavity fit vertically in the cube.
Radius of conical cavity i.e., cone,
Diameter
Since, the diameter is less than the side of a cube that means the base of a conical cavity is not fit inhorizontal face of cube.
Now, volume of cube
and volume of conical cavity i.e., cone
Volume of remaining solid Volume of cube - Volume of conical cavity
Hence, the required volume of solid is .
7 Two cones with same base radius and height are joined together along their bases. Find the surface area of the shape so formed.
Show Answer
Solution
If two cones with same base and height are joined together along their bases, then the shape so formed is look like as figure shown.
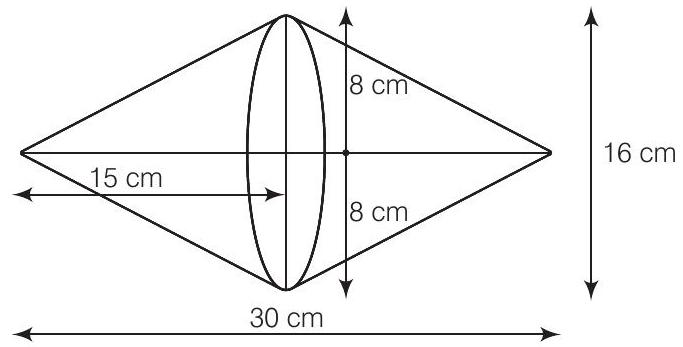
Given that, radius of cone, and height of cone,
So, surface area of the shape so formed
Curved area of first cone + Curved surface area of second cone
Surface area of cone
[since, both cones are identical]
(approx)
Hence, the surface area of shape so formed is .
8 Two solid cones and are placed in a cylindrical tube as shown in the figure. The ratio of their capacities is . Find the heights and capacities of cones. Also, find the volume of the remaining portion of the cylinder.
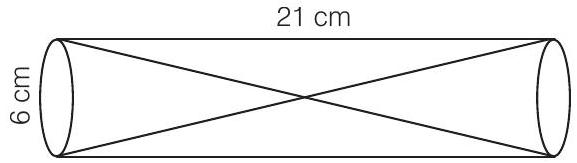
Show Answer
Solution
Let volume of cone be and volume of cone be . Again, let height of the cone , then height of cone
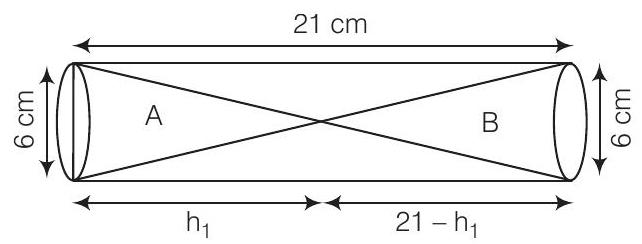
Given, diameter of the cone
Radius of the cone
Now, volume of the cone,
and volume of the cone,
From Eqs. (i) and (ii),
Required volume of the remaining portion Volume of the cylinder
9 An ice-cream cone full of ice-cream having radius and height as shown in figure
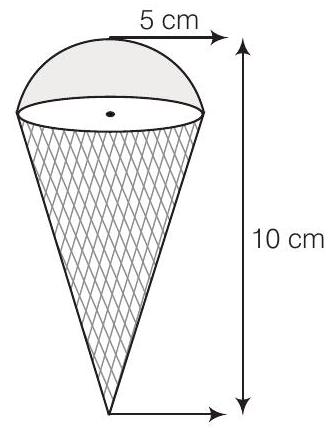
Calculate the volume of ice-cream, provided that its part is left unfilled with ice-cream.
Show Answer
Solution
Given, ice-cream cone is the combination of a hemisphere and a cone.
Also, radius of hemisphere
Volume of hemisphere
Now, radius of the cone
and height of the cone
Volume of the cone
Now, total volume of ice-cream cone
Since, part is left unfilled with ice-cream.
Required volume of ice-cream
10 Marbles of diameter are dropped into a cylindrical beaker of diameter containing some water. Find the number of marbles that should be dropped into the beaker, so that the water level rises by .
Show Answer
Solution
Given, diameter of a marble
Also, given diameter of beaker
Radius of beaker
Height of water level raised
Volume of the raised water in beaker
Now, required number of marbles
11 How many spherical lead shots each of diameter can be obtained from a solid rectangular lead piece with dimensions and ?
Show Answer
Solution
Given that, lots of spherical lead shots made from a solid rectangular lead piece.
Number of spherical lead shots
Also, given that diameter of a spherical lead shot , sphere
Radius of a spherical lead shot, radius diameter
So, volume of a spherical lead shot i.e., sphere
Now, length of rectangular lead piece,
Breadth of rectangular lead piece,
Height of rectangular lead piece,
Volume of a solid rectangular lead piece i.e., cuboid
From Eq. (i),
Number of spherical lead shots
Hence, the required number of spherical lead shots is 1500 .
12 How many spherical lead shots of diameter can be made out of a solid cube of lead whose edge measures .
Show Answer
Solution
Given that, lots of spherical lead shots made out of a solid cube of lead.
Number of spherical lead shots
Given that, diameter of a spherical lead shot i.e., sphere
Radius of a spherical lead shot
So, volume of a spherical lead shot i.e., sphere
Now, since edge of a solid cube
So, volume of a solid cube
From Eq. (i),
Number of spherical lead shots
Hence, the required number of spherical lead shots is 2541.
13. wall long, thick and high is constructed with the bricks each of dimensions . If the mortar occupies th of the volume of the wall, then find the number of bricks used in constructing the wall.
Show Answer
Solution
Given that, a wall is constructed with the help of bricks and mortar.
From Eq. (i),
Hence, the required number of bricks used in constructing the wall is 12960 .
14 Find the number of metallic circular disc with base diameter and of height to be melted to form a right circular cylinder of height and diameter .
Show Answer
Solution
Given that, lots of metallic circular disc to be melted to form a right circular cylinder. Here, a circular disc work as a circular cylinder.
Base diameter of metallic circular disc
Radius of metallic circular disc diameter radius and height of metallic circular disc i.e.,
Volume of a circular disc Height
Now, height of a right circular cylinder
and diameter of a right circular cylinder
Radius of a right circular cylinder
Volume of right circular cylinder
Number of metallic circular disc
Hence, the required number of metallic circular disc is 450 .
Long Answer Type Questions
1 A solid metallic hemisphere of radius is melted and recasted into a right circular cone of base radius . Determine the height of the cone.
Show Answer
Solution
Let height of the cone be .
Given, radius of the base of the cone
Volume of circular cone
Also, given radius of the hemisphere
Volume of the hemisphere
According to the question,
Volume of the cone Volume of the hemisphere
2 A rectangular water tank of base contains water upto a height of . If the water in the tank is transferred to a cylindrical tank of radius , find the height of the water level in the tank.
Show Answer
Solution
Given, dimensions of base of rectangular tank and height of water
Volume of the water in rectangular tank
Also, given radius of the cylindrical tank
Let height of water level in cylindrical tank be .
Then, volume of the water in cylindrical tank
According to the question,
Hence, the height of water level in cylindrical tank is .
3 How many cubic centimetres of iron is required to construct an open box whose external dimensions are and provided the thickness of the iron is . If one cubic centimetre of iron weights , then find the weight of the box.
Show Answer
Solution
Let the length , breadth (b) and height ( ) be the external dimension of an open box and thickness be .
Given that,
external length of an open box
external breadth of an open box
and external height of an open box
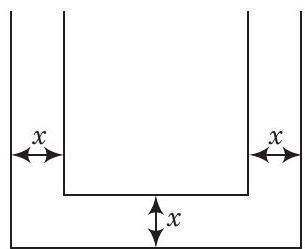
External volume of an open box bh
Since, the thickness of the iron
So, internal length of an open box
Therefore, internal breadth of an open box
and internal height of an open box
So, internal volume of an open box
Therefore, required iron to construct an open box
Hence, required iron to construct an open box is .
Given that, of iron weights
of iron weights
4 The barrel of a fountain pen, cylindrical in shape, is long and in diameter. A full barrel of ink in the pin is used up on writing 3300 words on an average. How many words can be written in a bottle of ink containing one-fifth of a litre?
Show Answer
Solution
Given, length of the barrel of a fountain pen and diameter
Radius of the barrel
Volume of the barrel [since, its shape is cylindrical]
Also, given volume of ink in the bottle of litre
Now, ink is used for writing number of words
ink is used for writing number of words
ink is used for writing number of words
5 Water flows at the rate of through a cylindrical pipe in diameter. How long would it take to fill a conical vessel whose diameter at the base is and depth ?
Show Answer
Solution
Given, speed of water flow
and diameter of the pipe
Radius of the pipe
Area of the face of pipe
Also, given diameter of the conical vessel
Radius of the conical vessel
and depth of the conical vessel
Volume of conical vessel
6 A heap of rice is in the form of a cone of diameter and height . Find the volume of the rice. How much canvas cloth is required to just cover heap?
Show Answer
Solution
Given that, a heap of rice is in the form of a cone.
Height of a heap of rice i.e., cone
and diameter of a heap of rice i.e., cone
Radius of a heap of rice i.e., cone
So,
Now, canvas cloth required to just cover heap of rice
Surface area of a heap of rice
Hence, canvas cloth is required to just cover heap.
7 A factory manufactures 120000 pencils daily. The pencils are cylindrical in shape each of length and circumference of base as . Determine the cost of colouring the curved surfaces of the pencils manufactured in one day at ₹ 0.05 per .
Show Answer
Solution
Given, pencils are cylindrical in shape.
Length of one pencil
and circumference of base
Now, curved surface area of one pencil
Curved surface area of 120000 pencils
Now, cost of colouring curved surface of the pencils manufactured in one day
Cost of colouring curved surface
8 Water is flowing at the rate of through a pipe of diameter into a cuboidal pond which is long and wide. In what time will the level of water in pond rise by ?
Show Answer
Solution
Given, length of the pond and width of the pond
Volume of water in the pond
Also, given radius of the pipe
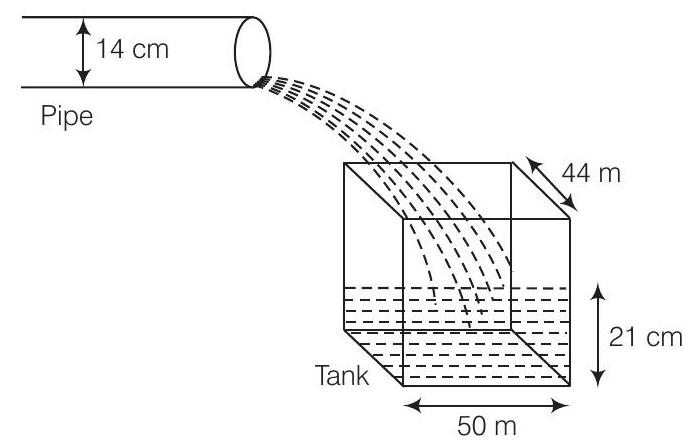
and speed of water flowing through the pipe
Now, volume of water flow in
Since, of water falls in the pond in .
So, water falls in the pond in .
Also, of water falls in the pond in
Hence, the required time is .
9 A solid iron cuboidal block of dimensions is recast into a hollow cylindrical pipe of internal radius and thickness . Find the length of the pipe.
Show Answer
Solution
Given that, a solid iron cuboidal block is recast into a hollow cylindrical pipe.
Length of cuboidal pipe
Breadth of cuboidal pipe and height of cuboidal pipe
So, volume of a solid iron cuboidal block
Also, internal radius of hollow cylindrical pipe
and thickness of hollow cylindrical pipe
So, external radius of hollow cylindrical pipe Thickness
Volume of hollow cylindrical pipe Volume of cylindrical pipe with external radius
-Volume of cylindrical pipe with internal radius
where, be the length of the hollow cylindrical pipe.
Now, by given condition,
Volume of solid iron cuboidal block Volume of hollow cylindrical pipe
Hence, required length of pipe is .
10500 persons are taking a dip into a cuboidal pond which is long and broad. What is the rise of water level in the pond, if the average displacement of the water by a person is ?
Show Answer
Solution
Let the rise of water level in the pond be , when 500 persons are taking a dip into a cuboidal pond.
Given that,
Length of the cuboidal pond
Breadth of the cuboidal pond
Now, volume for the rise of water level in the pond
and the average displacement of the water by a person
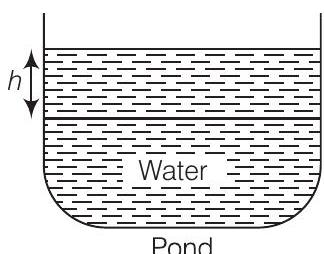
So, the average displacement of the water by 500 persons
Now, by given condition,
Volume for the rise of water level in the pond =Average displacement of the water by
500 persons
Hence, the required rise of water level in the pond is .
1116 glass spheres each of radius are packed into a cuboidal box of internal dimensions and then the box is filled with water. Find the volume of water filled in the box.
Show Answer
Solution
Given, dimensions of the cuboidal
Volume of the cuboidal
Also, given radius of one glass sphere
Volume of one glass sphere
Now, volume of 16 glass spheres
Required volume of water Volume of cuboidal - Volume of 16 glass spheres
12 A milk container of height is made of metal sheet in the form of a frustum of a cone with radii of its lower and upper ends as and , respectively. Find the cost of milk at the rate of ₹ 22 per which the container can hold.
Show Answer
Solution
Given that, height of milk container ,
Radius of lower end of milk container
and radius of upper end of milk container
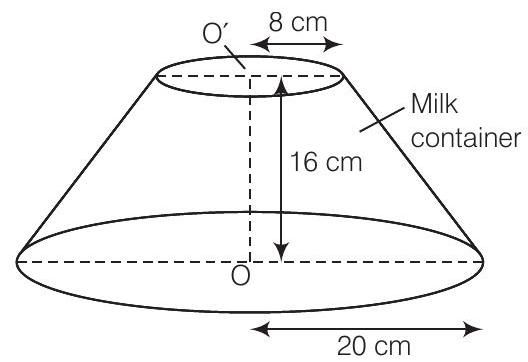
Volume of the milk container made of metal sheet in the form of a frustum of a cone
So, volume of the milk container is .
Cost of milk ₹ 22
Cost of milk
Hence, the required cost of milk is ₹ 230.12
13 A cylindrical bucket of height and base radius is filled with sand. This bucket is emptied on the ground and a conical heap of sand is formed. If the height of the conical heap is , find the radius and slant height of the heap.
Show Answer
Solution
Given, radius of the base of the bucket
Height of the bucket
So, volume of the sand in cylindrical bucket
Also, given height of the conical heap
Let radius of heap be .
Then, volume of the sand in the heap
According to the question,
Volume of the sand in cylindrical bucket Volume of the sand in conical heap
Again, let the slant height of the conical heap
Now,
Hence, radius of conical heap of sand
and slant height of conical heap
14 A rocket is in the form of a right circular cylinder closed at the lower end and surmounted by a cone with the same radius as that of the cylinder. The diameter and height of the cylinder are and , respectively. If the slant height of the conical portion is , then find the total surface area and volume of the rocket. (use )
Show Answer
Solution
Since, rocket is the combination of a right circular cylinder and a cone.
Given, diameter of the cylinder
Radius of the cylinder
and height of the cylinder
Volume of the cylinder
and curved surface area
Now, in right angled ,
Height of the cone,
Radius of the cone,
Now, volume of the cone
and curved surface area
Hence, total volume of the rocket

and total surface area of the rocket CSA of cone + CSA of cylinder
15 A building is in the form of a cylinder surmounted by a hemispherical vaulted dome and contains of air. If the internal diameter of dome is equal to its total height above the floor, find the height of the building?
Show Answer
Solution
Let total height of the building Internal diameter of the dome

Radius of building (or dome)
Height of cylinder
Volume of the cylinder
and volume of hemispherical dome cylinder
Total volume of the building Volume of the cylinder + Volume of hemispherical dome
According to the question,
16 A hemispherical bowl of internal radius is full of liquid. The liquid is to be filled into cylindrical shaped bottles each of radius and height . How many bottles are needed to empty the bowl?
Show Answer
Solution
Given, radius of hemispherical bowl, and radius of cylindrical bottles, and height,
Number of required cylindrical bottles
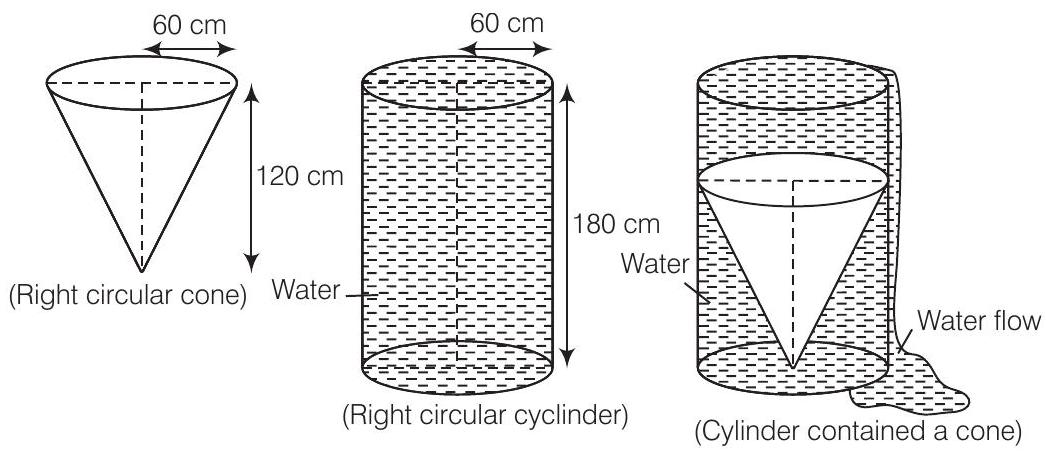
17 A solid right circular cone of height and radius is placed in a right circular cylinder full of water of height . Such that it touches the bottom. Find the volume of water left in the cylinder, if the radius of the cylinder is equal to the radius to the cone.
Show Answer
Solution
(i) Whenever we placed a solid right circular cone in a right circular cylinder with full of water, then volume of a solid right circular cone is equal to the volume of water falled from the cylinder.
(ii) Total volume of water in a cylinder is equal to the volume of the cylinder.
(iii) Volume of water left in the cylinder Volume of the right circular cylinder -volume of a right circular cone.
Now, given that
Height of a right circular cone
Radius of a right circular cone
Volume of a right circular cone
Volume of a right circular cone Volume of water falled from the cylinder
[from point (i)]
Given that, height of a right circular cylinder
and radius of a right circular cylinder Radius of a right circular cone
Volume of a right circular cylinder
So, volume of a right circular cylinder Total volume of water in a cylinder
From point (iii),
Volume of water left in the cylinder
the cylinder when solid cone is placed in it
Hence, the required volume of water left in the cylinder is .
18 Water flows through a cylindrical pipe, whose inner radius is , at the rate of in an empty cylindrical tank, the radius of whose base is . What is the rise of water level in tank in half an hour?
Show Answer
Solution
Given, radius of tank,
Let height of water level in tank in half an hour
Also, given internal radius of cylindrical pipe,
and speed of water i.e., in 1 water flow
In water flow
According to the question,
Volume of water in cylindrical tank =Volume of water flow from the circular pipe
in half an hour
Hence, the level of water in cylindrical tank rises in half an hour.
19 The rain water from a roof of dimensions drains into a cylindrical vessel having diameter of base and height . If the rain water collected from the roof just fill the cylindrical vessel, then find the rainfall (in ).
Show Answer
Solution
Given, length of roof and breadth of roof
Let the rainfall be .
Volume of water on the roof
Also, we have radius of base of the cylindrical vessel
and height of the cylindrical vessel
Volume of water in the cylindrical vessel when it is just full
Now, volume of water on the roof Volume of water in the vessel
Hence, the rainfall is .
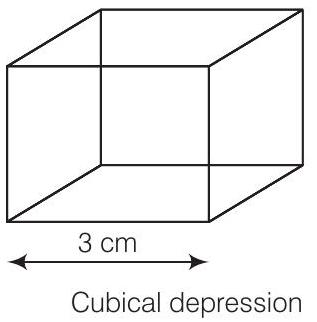
20 A pen stand made of wood is in the shape of a cuboid with four conical depressions and a cubical depression to hold the pens and pins, respectively. The dimensions of cubiod are and . The radius of each of the conical depressions is and the depth is . The edge of the cubical depression is . Find the volume of the wood in the entire stand.
Show Answer
Solution
Given that, length of cuboid pen stand
Breadth of cubiod pen stand
and height of cuboid pen stand

(Pen)
with conical base
(Pin)

Cuboid
with cubical base
Volume of cuboid pen stand
Also, radius of conical depression
and height (depth) of a conical depression
Volume of a conical depression
Also, given
Edge of cubical depression (a)
Volume of cubical depression
So, volume of 4 conical depressions
Volume of a conical depression
Hence, the volume of wood in the entire pen stand
Volume of cuboid pen stand - Volume of 4 conical
depressions - volume of a cubical depressions
So, the required volume of the wood in the entire stand is
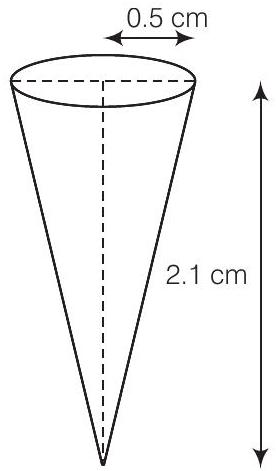
Conical depression
 .
.