Chapter 8 Application Of Integrals Miscellaneous Exercise
Miscellaneous Exercise on Chapter 8
1. Find the area under the given curves and given lines:
$\quad\quad$(i) $y=x^{2}, x=1, x=2$ and $x$-axis
$\quad\quad$(ii) $y=x^{4}, x=1, x=5$ and $x$-axis
Show Answer
Solution
i. The required area is represented by the shaded area ADCBA as
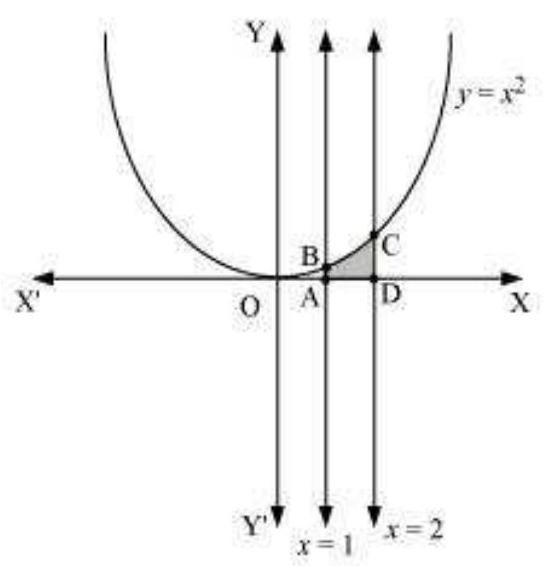
$ \begin{aligned} \text{ Area ADCBA } & =\int_1^{2} y d x \\ & =\int_1^{2} x^{2} d x \\ & =[\frac{x^{3}}{3}]_1^{2} \\ & =\frac{8}{3}-\frac{1}{3} \\ & =\frac{7}{3} \text{ units } \end{aligned} $
ii. The required area is represented by the shaded area ADCBA as

$ \begin{aligned} \text{ Area ADCBA } & =\int_1^{5} x^{4} d x \\ & =[\frac{x^{5}}{5}]_1^{5} \\ & =\frac{(5)^{5}}{5}-\frac{1}{5} \\ & =(5)^{4}-\frac{1}{5} \\ & =625-\frac{1}{5} \\ & =624.8 \text{ units } \end{aligned} $
2. Sketch the graph of $y=|x+3|$ and evaluate $\int _{-6}^{0}|x+3| d x$.
Show Answer
Solution
The given equation is $y=|x+3|$
The corresponding values of $x$ and $y$ are given in the following table.
| $x$ | -6 | -5 | -4 | -3 | -2 | -1 | 0 |
|---|---|---|---|---|---|---|---|
| $y$ | 3 | 2 | 1 | 0 | 1 | 2 | 3 |
On plotting these points, we obtain the graph of $y=|x+3|$ as follows.
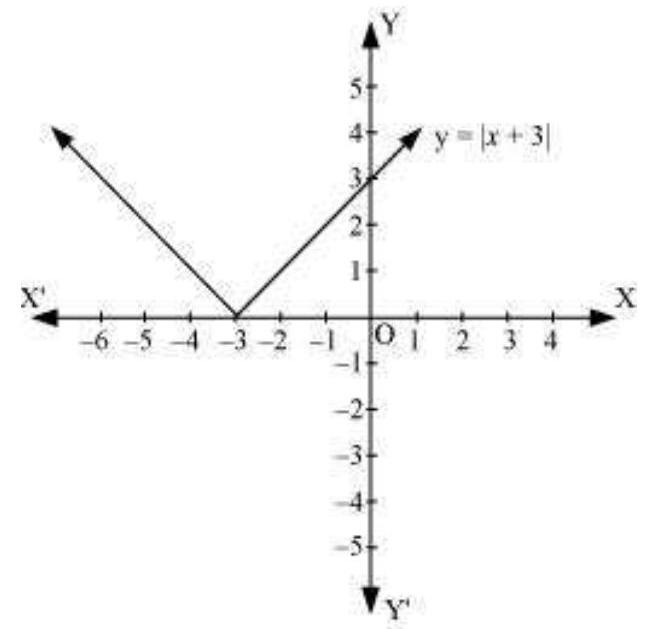
It is known that, $(x+3) \leq 0$ for $-6 \leq x \leq-3$ and $(x+3) \geq 0$ for $-3 \leq x \leq 0$
$ \begin{aligned} \therefore \int _{-6}^{0}|(x+3)| d x & =-\int _{-6}^{-3}(x+3) d x+\int _{-3}^{0}(x+3) d x \\ & =-[\frac{x^{2}}{2}+3 x] _{-6}^{-3}+[\frac{x^{2}}{2}+3 x] _{-3}^{0} \\ & =-[(\frac{(-3)^{2}}{2}+3(-3))-(\frac{(-6)^{2}}{2}+3(-6))]+[0-(\frac{(-3)^{2}}{2}+3(-3)]] \\ & =-[-\frac{9}{2}]-[-\frac{9}{2}] \\ & =9 \end{aligned} $
3. Find the area bounded by the curve $y=\sin x$ between $x=0$ and $x=2 \pi$.
Show Answer
Solution
The graph of $y=\sin x$ can be drawn as
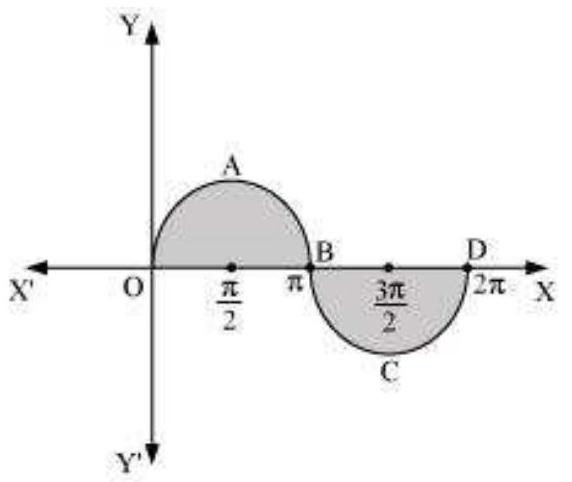
$\therefore$ Required area $=$ Area $OABO+$ Area $BCDB$
$ \begin{aligned} & =\int_0^{\pi} \sin x d x+|\int _{\pi}^{2 \pi} \sin x d x| \\ & =[-\cos x]_0^{\pi}+|[-\cos x] _{\pi}^{2 \pi}| \\ & =[-\cos \pi+\cos 0]+|-\cos 2 \pi+\cos \pi| \\ & =1+1+|(-1-1)| \\ & =2+|-2| \\ & =2+2=4 \text{ units } \end{aligned} $
Choose the correct answer in the following Exercises from 4 to 5.
4. Area bounded by the curve $y=x^{3}$, the $x$-axis and the ordinates $x=-2$ and $x=1$ is
$\quad\quad$(A) -9
$\quad\quad$(B) $\frac{-15}{4}$
$\quad\quad$(C) $\frac{15}{4}$
$\quad\quad$(D) $\frac{17}{4}$
Show Answer
Solution
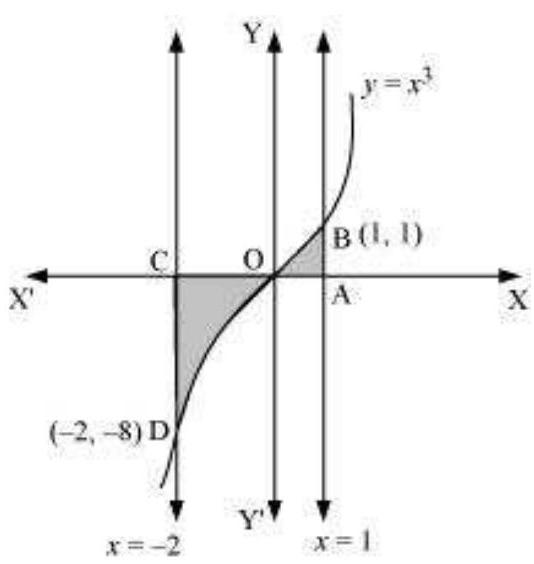
Required area $=\int _{-2}^{1} y d x$
1- Identify positive and negative areas:
- We know the curve dips below the x-axis between x = -2 and x = 0 (roughly at the point where x = -1).
- So, we’ll calculate the area under the curve in two sections:
- Area above the x-axis: from x = 0 to x = 1 (positive)
- Area below the x-axis: from x = -2 to x = 0 (negative)
2- Set up definite integrals:
- Area above the x-axis: ∫₀¹ (x^3) dx
- Area below the x-axis: ∫-20 (x^3) dx (notice the negative sign here to account for the area below the x-axis)
3- Evaluate the integrals: Following the same steps as before using the power rule of integration:
- Area above the x-axis: [(x^4)/4]₀¹ = (1/4)
- Area below the x-axis: |[(-x^4)/4]₋²⁰| = (16/4) (absolute value to consider the positive area enclosed below the x-axis)
4- Calculate the net enclosed area:
- Net Enclosed Area = Area above the x-axis+ Area below the x-axis = (1/4) + (16/4) = 17/4
The correct option is D.
5. The area bounded by the curve $y=x|x|, x$-axis and the ordinates $x=-1$ and $x=1$ is given by
$\quad\quad$(A) 0
$\quad\quad$(B) $\frac{1}{3}$
$\quad\quad$(C) $\frac{2}{3}$
$\quad\quad$(D) $\frac{4}{3}$
[Hint : $y=x^{2}$ if $x>0$ and $y=-x^{2}$ if $.x<0$].
Show Answer
Solution

Required area $=\int _{-1}^{1} y d x$
$=-\int _{-1}^{1} x|x| d x$
$=-\int _{-1}^{0} x^{2} d x+\int_0^{1} x^{2} d x$
$=-[\frac{x^{3}}{3}] _{-1}^{0}+[\frac{x^{3}}{3}]_0^{1}$
$=-(-\frac{1}{3})+\frac{1}{3}$
$=\frac{2}{3}$ units
Thus, the correct answer is C.










