Chapter 8 Application Of Integrals EXERCISE 8.1
EXERCISE 8.1
1. Find the area of the region bounded by the ellipse $\frac{x^{2}}{16}+\frac{y^{2}}{9}=1$.
Show Answer
Solution
The given equation of the ellipse, $\frac{x^{2}}{16}+\frac{y^{2}}{9}=1$, can be represented as
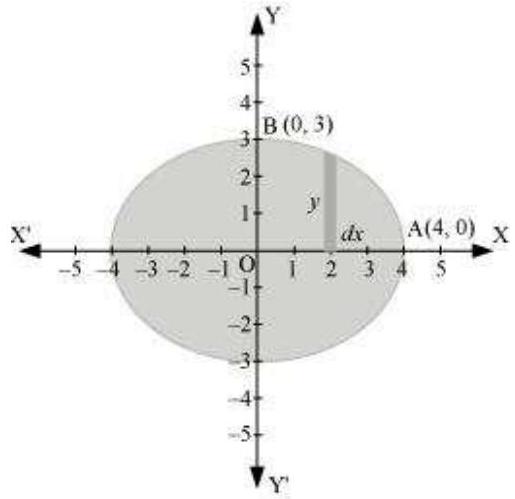
It can be observed that the ellipse is symmetrical about $x$-axis and $y$-axis.
$\therefore$ Area bounded by ellipse $=4 \times$ Area of OAB
$ \begin{aligned} \text{ Area of } OAB & =\int_0^{4} y d x \\ & =\int_0^{4} 3 \sqrt{1-\frac{x^{2}}{16}} d x \\ & =\frac{3}{4} \int_0^{4} \sqrt{16-x^{2}} d x \\ & =\frac{3}{4}[\frac{x}{2} \sqrt{16-x^{2}}+\frac{16}{2} \sin ^{-1} \frac{x}{4}]_0^{4} \\ & =\frac{3}{4}[2 \sqrt{16-16}+8 \sin ^{-1}(1)-0-8 \sin ^{-1}(0)] \\ & =\frac{3}{4}[\frac{8 \pi}{2}] \\ & =\frac{3}{4}[4 \pi] \\ & =3 \pi \end{aligned} $
Therefore, area bounded by the ellipse $=4 \times 3\pi =12 \pi$ units
2. Find the area of the region bounded by the ellipse $\frac{x^{2}}{4}+\frac{y^{2}}{9}=1$.
Show Answer
Solution
The given equation of the ellipse can be represented as
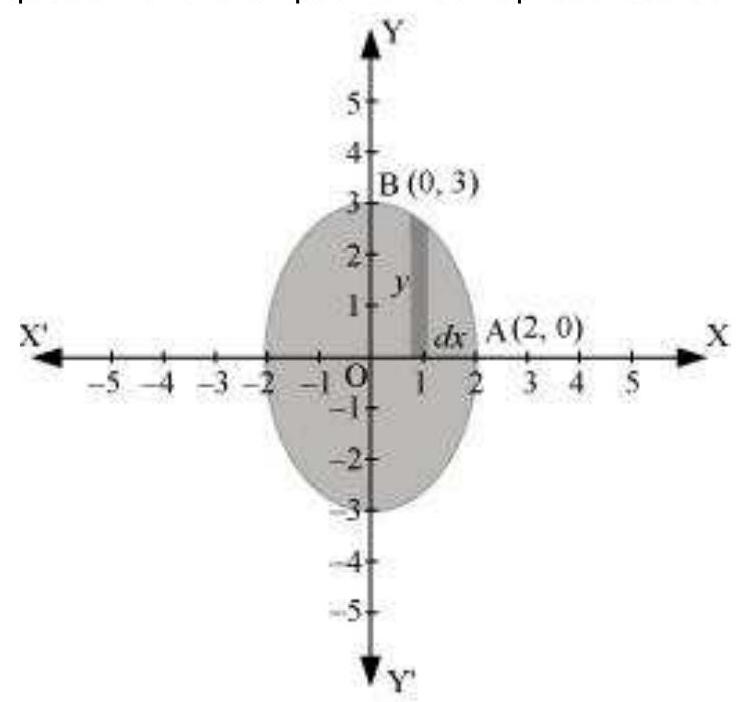
$\frac{x^{2}}{4}+\frac{y^{2}}{9}=1$
$\Rightarrow y=3 \sqrt{1-\frac{x^{2}}{4}}$
It can be observed that the ellipse is symmetrical about $x$-axis and $y$-axis.
$\therefore$ Area bounded by ellipse $=4 \times$ Area OAB $\therefore$ Area of $OAB=\int_0^{2} y d x$
$ \begin{aligned} & =\int_0^{2} 3 \sqrt{1-\frac{x^{2}}{4}} d x \quad \text{ [Using (1)] } \\ & =\frac{3}{2} \int_0^{2} \sqrt{4-x^{2}} d x \\ & =\frac{3}{2}[\frac{x}{2} \sqrt{4-x^{2}}+\frac{4}{2} \sin ^{-} \frac{x}{2}]_0^{2} \\ & =\frac{3}{2}[\frac{2 \pi}{2}] \\ & =\frac{3 \pi}{2} \end{aligned} $
Therefore, area bounded by the ellipse $=4 \times \frac{3 \pi}{2}=6 \pi$ units
Choose the correct answer in the following Exercises 3 and 4.
3. Area lying in the first quadrant and bounded by the circle $x^{2}+y^{2}=4$ and the lines $x=0$ and $x=2$ is
$\quad\quad$(A) $\pi$
$\quad\quad$(B) $\frac{\pi}{2}$
$\quad\quad$(C) $\frac{\pi}{3}$
$\quad\quad$(D) $\frac{\pi}{4}$
Show Answer
Solution
The area bounded by the circle and the lines, $x=0$ and $x=2$, in the first quadrant is represented as
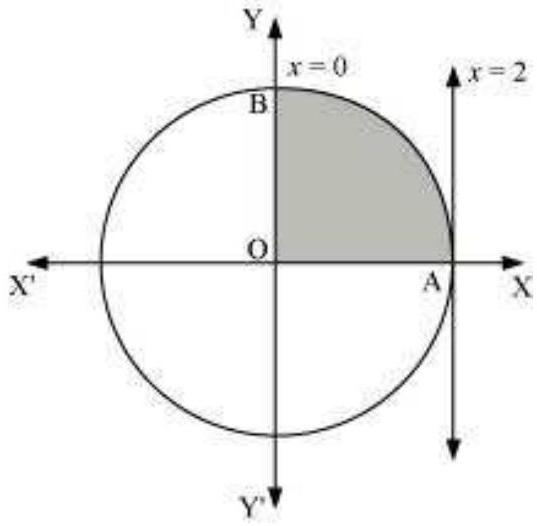
$ \begin{aligned} \therefore \text{ Area OAB } & =\int_0^{2} y d x \\ & =\int_0^{2} \sqrt{4-x^{2}} d x \\ & =[\frac{x}{2} \sqrt{4-x^{2}}+\frac{4}{2} \sin ^{-1} \frac{x}{2}]_0^{2} \\ & =2(\frac{\pi}{2}) \\ & =\pi \text{ units } \end{aligned} $
Thus, the correct answer is $A$.
4. Area of the region bounded by the curve $y^{2}=4 x, y$-axis and the line $y=3$ is
$\quad\quad$(A) 2
$\quad\quad$(B) $\frac{9}{4}$
$\quad\quad$(C) $\frac{9}{3}$
$\quad\quad$(D) $\frac{9}{2}$
Show Answer
Solution
The area bounded by the curve, $y^{2}=4 x, y$-axis, and $y=3$ is represented as

$\therefore$ Area $OAB=\int_0^{3} x d y$
$ =\int_0^{3} \frac{y^{2}}{4} d y $
$ \begin{aligned} & =\frac{1}{4}[\frac{y^{3}}{3}]_0^{3} \\ & =\frac{1}{12}(27) \\ & =\frac{9}{4} \text{ units } \end{aligned} $
Thus, the correct answer is $B$.










